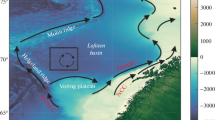
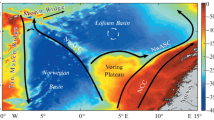
Abstract
Mean radial distributions of various dynamic characteristics of the permanently existing anticyclonic Lofoten vortex (LV) in the Norwegian Sea are obtained from an eddy-permitting regional hydrodynamic MIT general circulation model. It is shown that the model adequately reproduces the observed 3D thermohaline and dynamic structure of the vortex. The obtained radial distribution of the mean vertical velocity is found to form a complex structure: with the upward fluxes along the axis in and above the anticyclonically rotating LV core, compensated by the downward fluxes in the vortex skirt. These vertical motions maintain the vortex potential energy anomaly against dissipation. This secondary circulation is generated by the centrifugal force and, to a lesser extent, by the horizontal dispersion of the vortex energy, both intensified towards the sea surface. Below the vortex core, the maximum downward vertical velocity converges towards the vortex axis with depth. At these depth levels, the secondary circulation is forced by Ekman divergence in the bottom mixed layer. The theory of columnar vortices with helical structure, applied to the LV, relate the radial profiles of the vertical velocity with those of the horizontal circulation. The theoretically predicted the radial patterns of the mean vertical velocity in the LV were close to those, obtained from the primitive equation ocean model, when approximating the radial patterns of the azimuthal velocity with the Rayleigh profile.








Similar content being viewed by others
References
Adcroft A, Campin JM, Dutkiewicz S, Evangelinos C, Ferreira D, Follows M, ..., Hill E (2018) MITgcm Documentation: 1–306
Alekseenko SV, Kuibin PA, Okulov VL, Shtork SI (1999) Helical vortices in swirl flow. J Fluid Mech 382:195–243
Alekseenko SV, Kuibin PA, Okulov VL (2007) Theory of concentrated vortices. An introduction. Springer, Berlin 506 p
Alekseev GV, Bagryantsev MV, Bogorodskiy PV, Vasin VV, Shirokov PE (1991) Structure and circulation of water in the area of anticyclonic eddy in the northeastern Norwegian Sea [in Russian]. Probl Arctic Antarct 65:14–23
Arbic BK, Scott RB, Chelton DB, Richman JG, Shriver JF (2012) Effects of stencil width on surface ocean geostrophic velocity and vorticity estimation from gridded satellite altimeter data. J Geophys Res 117: C03029, https://doi.org/10.1029/2011JC007367
Barcelo-Llull B, Sangrà P, Pallàs-Sanz E, Barton ED, Estrada-Allis SN, Martínez-Marrero A et al (2017) Anatomy of a subtropical intrathermocline eddy. Deep Sea Res I 124:126–139
Bashmachnikov IL (2017) Seasonal and interannual variability of the position of the dynamic and thermal fronts of the Barents, Norwegian and Greenland seas [in Russian]. Conference materials “The Seas of Russia: science, security, resources”, 3–7 October 2017: 29–30
Bashmachnikov I, Boutov D, Dias J (2013a) Manifestation of two meddies in altimetry and sea-surface temperature. Ocean Sci 9(2):249–259
Bashmachnikov I, Loureiro C, Martins A (2013b) Topographically induced circulation patterns and mixing over condor seamount. Deep Sea Res II 98:38–51
Bashmachnikov I, Carton X, Belonenko T (2014) Characteristics of surface signatures of Mediterranean water eddies. J Geophys Res C119:1–22. https://doi.org/10.1002/2014JC010244
Bashmachnikov I, Neves F, Calheiros T, Carton X (2015) Properties and pathways of Mediterranean water eddies in the Atlantic. Prog Oceanogr 137:149–172
Bashmachnikov IL, Belonenko TV, Kuibin PA (2017a) The application of the theory of the columnar Q-vortex with helical structure to the description of the dynamic characteristics of the Lofoten vortex of the Norwegian sea [in Russian]Vestn St Petersburg Un-ta Ser.7 62(3):221-336. https://doi.org/10.21638/11701/spbu07.2017.301
Bashmachnikov IL, Sokolovskiy MA, Belonenko TV, Volkov DL, Isachsen PE, Carton X (2017b) On the vertical structure and stability of the Lofoten vortex in the Norwegian Sea. Deep Sea Res I 128:1–27. https://doi.org/10.1016/j.dsr.2017.08.001
Batchelor GK (1964) Axial flow in trailing line vortices. J Fluid Mech 20:645–658
Belonenko TV, Volkov DL, Ozhigin VK, Norden YuE (2014) Circulation of waters in the Lofoten Basin of the Norwegian Sea, [in Russian]. Vestn S. Petersbur. Un-ta, Ser.7. 2:108-121
Belonenko TV, Bashmachnikov IL, Koldunov AV, Kuibin PA (2017) On the vertical component of velocity in the Lofoten vortex of the Norwegian Sea [in Russian]. Izvestiya Atmos Ocean Phys 53(6):641–649. https://doi.org/10.1134/S0001433817060032
Bowden KF (1983) Physical oceanography of coastal waters. Ellis Horwood Limited, Chichester, p 302
Capet A, Mason E, Rossi V, Troupin C, Faugère Y, Pujol I, Pascual A (2014) Implications of refined altimetry on estimates of mesoscale activity and eddy‐driven offshore transport in the Eastern Boundary Upwelling Systems. Geophys Res Lett 41(21): 7602-7610
Carton X (2001) Hydrodynamical modelling of oceanic vortices. Surv Geophys 22:179–263
Chelton DB, Schlax MG, Samelson RM, de Szoeke RA (2007) Global observations of large oceanic eddies. Geophys Res Lett 34:L15606. https://doi.org/10.1029/2007GL030812
Chelton DB, Schlax MG, Samelson RM (2011) Global observations of nonlinear mesoscale eddies. Prog Oceanogr 91:167–216
Ciani D, Carton X, Bashmachnikov I, Chapron B, Perrot X (2015) Influence of deep vortices on the ocean surface, discontinuity, nonlinearity, and complexity. 4(3):281–311. https://doi.org/10.5890/DNC.2015.09.006
Ciani D, Carton X, Aguiar AB, Peliz A, Bashmachnikov I, Ienna F, Charron R, Santoleri R (2017) Surface signature of Mediterranean water eddies in a long-term high-resolution simulation. Deep-Sea Res I Oceanogr Res Pap 130:12–29
Fer I, Bosse A, Ferron B, Bouruet-Aubertot P (2018) The dissipation of kinetic energy in the Lofoten Basin Eddy. J Phys Oceanogr 48(6):1299–1316
Gaube P, Chelton DB, Strutton PG, Behrenfeld MJ (2013) Satellite observations of chlorophyll, phytoplankton biomass, and Ekman pumping in nonlinear mesoscale eddies. J Geophys Res C118. https://doi.org/10.1002/2013JC009027
Good SA, Martin MJ, Rayner NA (2013) EN4: Quality controlled ocean temperature and salinity profiles and monthly objective analyses with uncertainty estimates. J Geophys Res Oceans 118(12): 6704-6716
Golivets SV, Koshlyakov MN (2003) Cyclonic vortices of the subantarctic front and formation of Antarctic intermediate water. Oceanology [in Russian] 43(3):325–338
Hansen C, Kvaleberg E, Samuelsen A (2010) Anticyclonic eddies in the Norwegian Sea; their generation, evolution and impact on primary production. Deep Sea Res I 57(9):1079–1091
Isachsen PE (2015) Baroclinic instability and the mesoscale eddy field around the Lofoten Basin. J Geophys Res 120(4):2884–2903
Ivanov VV, Korablev AA (1995a) Formation and regeneration of the pycnocline lens in the Norwegian Sea, [in Russian]. Russ Meteorol Hydrol 9:62–69
Ivanov VV, Korablev AA (1995b) Dynamics of pycnocline lens in the Norwegian sea, [in Russian]. Russ Meteorol Hydrol 10:55–62
Klein P, Lapeyre G (2009) The oceanic vertical pump induced by mesoscale and submesoscale turbulence. Annu Rev Mar Sci 1:351–375
Kohl A (2007) Generation and stability of a quasi-permanent vortex in the Lofoten Basin. J Phys Oceanogr 37:2637–2651
Kuibin PA, Okulov VL (1996) One-dimensional solutions a flow with a helical symmetry. Thermophys Aeromech 4:297–301
Lavelle JW (2006) Flow, hydrography, turbulent mixing, and dissipation at Fieberling Guyot examined with a primitive equation model. J Geophys Res 111:C07014. https://doi.org/10.1029/2005JC003224
Lozier MS (2010) Destructing the conveyor belt. Science 328:1507–1511. https://doi.org/10.1126/science.1189250
Luo D, Lu Y (2000) The influence of negative viscosity on wind-driven, barotropic ocean circulation in a subtropical basin. J Phys Oceanogr 30(5):916–932
Mahdinia M, Hassanzadeh P, Marcus PS, Jiang CH (2016) Stability of 3D Gaussian vortices in rotating stratified Boussinesq flows: linear analysis. J Fluid Mech 824:97–134. https://doi.org/10.1017/jfm.2017.303
Maze JP, Arhan M, Mercier H (1997) Volume budjet of the eastern boundary layer off the Iberian Peninsula. Deep Sea Res I 44(9–10):1543–1574
McGillicuddy DJ, Anderson LA, Bates NR, Bibby T, Buesseler KO, Carlson CA et al (2007) Eddy/wind interactions stimulate extraordinary mid-ocean plankton blooms. Science 316(5827):1021–1026
Mullineaux LS, Mills S (1997) A test of the larval retention hypothesis in seamount-generated flows. Deep Sea Res 44:745–770
Nguyen AT, Menemenlis D, Kwok R (2011) Arctic ice-ocean simulation with optimized model parameters: approach and assessment. J Geophys Res 116:C04025. https://doi.org/10.1029/2010JC006573
Nof D (1993) Generation of ringlets. Tellus A 45(4):299–310
Ozmidov RV (1986) Diffusion of an impurity in the ocean, [in Russian]. Leningrad, Gidrometeoizdat, 280 p
Paldor N (1999) Linear instability of barotropic submesoscale coherent vortices observed in the ocean. J Phys Oceanogr 29(7):1442–1452
Pedlosky J (1987) Geophysical fluid dynamics. Springer Verlag, 710 p
Pereskokov AI (1999) On the physical nature of large-scale counter-cyclical cycle in the water column of the Norwegian Sea, [in Russian]. Rep Acad Sci 364(4):549–552
Pilo GS, Oke PR, Coleman R, Rykova T, Ridgway K (2018) Patterns of vertical velocity induced by eddy distortion in an ocean model. J Geophys Res Oceans 123(3):2274–2292
Raj RP, Chafik L, Nilsen JEØ, Eldevik T, Halo I (2015) The Lofoten vortex of the Nordic seas. Deep-Sea Res I I96:1–14
Romantcev VA (1991) Large-scale structure and characteristics of the average circulation of the water, [in Russian]. Probl Arctic Antarc 65:75–97
Scully MP (1975) Computation of helicopter rotor wake geometry and its influence on rotor harmonic airloads. Massachusetts Inst. of Technology, Publ. ARSL TR 152–1, Cambridge
Siedler G, Church J, Gould J (eds) (2001) Ocean circulation and climate: observing and modelling the global ocean, International Geophysics Series. Academic Press, San Diego. 77, ISBN 0–12–641351-7. XIX, p 715
Søiland H, Rossby T (2013) On the structure of the Lofoten Basin Eddy. J Geophys Res Oceans 118(9):4201–4212
Søiland H, Chafik L, Rossby T (2016) On the long-term stability of the Lofoten Basin Eddy. J Geophys Res Oceans 121(7):4438–4449
Vaillancourt RD, Marra J, Seki MP, Parsons ML, Bidigare RR (2003) Impact of a cyclonic eddy on phytoplankton community structure and photosynthetic competency in the subtropical North Pacific Ocean. Deep Sea Res I 50:829–847
Volkov DL, Lee T, Fu LL (2008) Eddy-induced meridional heat transport in the ocean. Geoph Res Lett 35(20)
Volkov DL, Belonenko TV, Foux VR (2013) Puzzling over the dynamics of the Lofoten Basin—a sub-Arctic hot spot of ocean variability. Geophys Res Lett 40(4):738–743. https://doi.org/10.1002/grl.50126
Volkov DL, Kubryakov AA, Lumpkin R (2015) Formation and variability of the Lofoten basin vortex in a high-resolution ocean model. Deep Sea Res I 105:142–157. https://doi.org/10.1016/j.dsr.2015.09.001
Walsh D, Richardson PL, Lynch J (1997) Observations of tilting meddies. Oceanogr Lit Rev 2(44):84
White M, Bashmachnikov I, Aristegui J, Martins A (2007) Physical processes and seamount productivity. In: Pitcher TJ, Morato T, PJB H, Clark MR, Haggan N, Santos RS (eds) Seamounts: ecology, conservation and management. Fish and Aquatic Resources Series, Blackwell, Oxford, Chapter 4, pp 65–84
Wunsch C, Ferrari R (2004) Vertical mixing, energy, and the general circulation of the oceans. Annu Rev Fluid Mech 36:281–314
Yu LS, Bosse A, Fer I, Orvik KA, Bruvik EM, Hessevik I, Kvalsund K (2017) The Lofoten Basin eddy: three years of evolution as observed by Seagliders. J Geophys Res Oceans 122:6814–6834. https://doi.org/10.1002/2017JC012982
Zhmur VV (2011) Mesoscale vortices of the ocean, [in Russian]. GEOS, Moscow, 384 p
Acknowledgements
The authors acknowledge support of Russian Science Foundation (RSF, project No. 18-17-00027). D. Volkov was supported by the NASA Physical Oceanography program (Grant NNX11AE27G) and by the base funds of NOAA Atlantic Oceanographic and Meteorological Laboratory.
Author information
Authors and Affiliations
Corresponding author
Additional information
Responsible Editor: Sergey Prants
This article is part of the Topical Collection on the International Conference “Vortices and coherent structures: from ocean to microfluids”, Vladivostok, Russia, 28-31 August 2017
Rights and permissions
About this article
Cite this article
Bashmachnikov, I., Belonenko, T., Kuibin, P. et al. Pattern of vertical velocity in the Lofoten vortex (the Norwegian Sea). Ocean Dynamics 68, 1711–1725 (2018). https://doi.org/10.1007/s10236-018-1213-1
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10236-018-1213-1