Skip to main content
SpringerLink
Log in

On a Randomized Backward Euler Method for Nonlinear Evolution Equations with Time-Irregular Coefficients

  • Published:
Foundations of Computational Mathematics Aims and scope Submit manuscript

Abstract

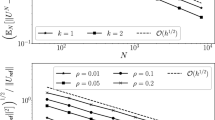
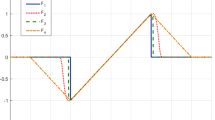
In this paper, we introduce a randomized version of the backward Euler method that is applicable to stiff ordinary differential equations and nonlinear evolution equations with time-irregular coefficients. In the finite-dimensional case, we consider Carathéodory-type functions satisfying a one-sided Lipschitz condition. After investigating the well-posedness and the stability properties of the randomized scheme, we prove the convergence to the exact solution with a rate of 0.5 in the root-mean-square norm assuming only that the coefficient function is square integrable with respect to the temporal parameter. These results are then extended to the approximation of infinite-dimensional evolution equations under monotonicity and Lipschitz conditions. Here, we consider a combination of the randomized backward Euler scheme with a Galerkin finite element method. We obtain error estimates that correspond to the regularity of the exact solution. The practicability of the randomized scheme is also illustrated through several numerical experiments.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

References

  1. H. Amann. Linear and Quasilinear Parabolic Problems. Vol. I: Abstract Linear Theory, volume 89 of Monographs in Mathematics. Birkhäuser Boston, Inc., Boston, MA, 1995.

  2. H. Amann. Compact embeddings of Sobolev and Besov spaces. Glasnik Matematicki, 35(55):161–177, 2000.

    MathSciNet  MATH  Google Scholar 

  3. A. Andersson and R. Kruse. Mean-square convergence of the BDF2-Maruyama and backward Euler schemes for SDE satisfying a global monotonicity condition. BIT Numer. Math., 57(1):21–53, 2017.

    Article  MathSciNet  Google Scholar 

  4. W. Arendt and M. Duelli. Maximal \(L^p\)-regularity for parabolic and elliptic equations on the line. J. Evol. Equ., 6(4):773–790, 2006.

    Article  MathSciNet  Google Scholar 

  5. C. Baiocchi and F. Brezzi. Optimal error estimates for linear parabolic problems under minimal regularity assumptions. Calcolo, 20(2):143–176, 1983.

    Article  MathSciNet  Google Scholar 

  6. R. E. Bank and H. Yserentant. On the \(H^1\)-stability of the \(L_2\)-projection onto finite element spaces. Numer. Math., 126(2):361–381, 2014.

    Article  MathSciNet  Google Scholar 

  7. C. Carstensen. Merging the Bramble-Pasciak-Steinbach and the Crouzeix-Thomée criterion for \(H^{1}\)-stability of the \(L^{2}\)-projection onto finite element spaces. Math. Comp., 71(237):157–163 (electronic), 2002.

  8. C. Carstensen. An adaptive mesh-refining algorithm allowing for an \(H^{1}\) stable \(L^{2}\) projection onto Courant finite element spaces. Constr. Approx., 20(4):549–564, 2004.

    Article  MathSciNet  Google Scholar 

  9. K. Chrysafinos and L. S. Hou. Error estimates for semidiscrete finite element approximations of linear and semilinear parabolic equations under minimal regularity assumptions. SIAM J. Numer. Anal., 40(1):282–306, 2002.

    Article  MathSciNet  Google Scholar 

  10. D. S. Clark. Short proof of a discrete Gronwall inequality. Discrete Appl. Math., 16(3):279–281, 1987.

    Article  MathSciNet  Google Scholar 

  11. M. Crouzeix and V. Thomée. The stability in \(L_{p}\) and \(W^{1}_{p}\) of the \(L_{2}\)-projection onto finite element function spaces. Math. Comp., 48(178):521–532, 1987.

    MathSciNet  MATH  Google Scholar 

  12. T. Daun. On the randomized solution of initial value problems. J. Complexity, 27(3-4):300–311, 2011.

    Article  MathSciNet  Google Scholar 

  13. M. Dindoš and V. Toma. Filippov implicit function theorem for quasi-Carathéodory functions. J. Math. Anal. Appl., 214(2):475–481, 1997.

    Article  MathSciNet  Google Scholar 

  14. E. Emmrich. Gewöhnliche und Operator-Differentialgleichungen. Vieweg, Wiesbaden, 2004.

    Book  Google Scholar 

  15. E. Emmrich. Two-step BDF time discretisation of nonlinear evolution problems governed by monotone operators with strongly continuous perturbations. Comput. Methods Appl. Math., 9(1):37–62, 2009.

    Article  MathSciNet  Google Scholar 

  16. L. C. Evans. Partial Differential Equations. Graduate studies in mathematics ; 19. American Mathematical Society, 1998.

  17. I. Gyöngy. On stochastic equations with respect to semimartingales. III. Stochastics, 7(4):231–254, 1982.

    Article  MathSciNet  Google Scholar 

  18. B. H. Haak and E. M. Ouhabaz. Maximal regularity for non-autonomous evolution equations. Math. Ann., 363(3-4):1117–1145, 2015.

    Article  MathSciNet  Google Scholar 

  19. S. Haber. A modified Monte-Carlo quadrature. Math. Comp., 20:361–368, 1966.

    Article  MathSciNet  Google Scholar 

  20. S. Haber. A modified Monte-Carlo quadrature. II. Math. Comp., 21:388–397, 1967.

    Article  MathSciNet  Google Scholar 

  21. W. Hackbusch. Optimal \(H^{p,\,p/2}\) error estimates for a parabolic Galerkin method. SIAM J. Numer. Anal., 18(4):681–692, 1981.

    Article  MathSciNet  Google Scholar 

  22. E. Hairer and G. Wanner. Solving Ordinary Differential Equations. Vol. II: Stiff and Differential-Algebraic Problems, volume 14 of Springer Series in Computational Mathematics. Springer-Verlag, Berlin, 2010. Second revised edition, paperback.

  23. J. K. Hale. Ordinary Differential Equations. Robert E. Krieger Publishing Co., Inc., Huntington, N.Y., second edition, 1980.

    MATH  Google Scholar 

  24. S. Heinrich and B. Milla. The randomized complexity of initial value problems. J. Complexity, 24(2):77–88, 2008.

    Article  MathSciNet  Google Scholar 

  25. L. S. Hou and W. Zhu. Error estimates under minimal regularity for single step finite element approximations of parabolic partial differential equations. Int. J. Numer. Anal. Model., 3(4):504–524, 2006.

    MathSciNet  MATH  Google Scholar 

  26. A. Jentzen and A. Neuenkirch. A random Euler scheme for Carathéodory differential equations. J. Comput. Appl. Math., 224(1):346–359, 2009.

    Article  MathSciNet  Google Scholar 

  27. B. Z. Kacewicz. Optimal solution of ordinary differential equations. J. Complexity, 3(4):451–465, 1987.

    Article  MathSciNet  Google Scholar 

  28. B. Z. Kacewicz. Almost optimal solution of initial-value problems by randomized and quantum algorithms. J. Complexity, 22(5):676–690, 2006.

    Article  MathSciNet  Google Scholar 

  29. A. Klenke. Probability Theory. A Comprehensive Course. Springer, London, 2nd ed. edition, 2014.

    Book  Google Scholar 

  30. R. Kruse and Y. Wu. Error analysis of randomized Runge-Kutta methods for differential equations with time-irregular coefficients. Comput. Methods Appl. Math., 17(3):479–498, 2017.

    Article  MathSciNet  Google Scholar 

  31. A. Logg, K.-A. Mardal, and G. N. Wells. Automated Solution of Differential Equations by the Finite Element Method: The FEniCS book. Lecture Notes in Computational Science and Engineering; 84. Springer, Berlin, Heidelberg, 2012 edition, 2012.

  32. A. Lunardi. Analytic Semigroups and Optimal Regularity in Parabolic Problems. Birkhäuser, Basel, 1995.

    Book  Google Scholar 

  33. D. Meidner and B. Vexler. Optimal error estimates for fully discrete Galerkin approximations of semilinear parabolic equations. ArXiv preprint, arXiv:1707.07889v1, 2017.

  34. E. Novak. Deterministic and Stochastic Error Bounds in Numerical Analysis, volume 1349 of Lecture Notes in Mathematics. Springer-Verlag, Berlin, 1988.

  35. A. Ostermann and M. Thalhammer. Convergence of Runge-Kutta methods for nonlinear parabolic equations. Appl. Numer. Math., 42(1-3):367–380, 2002. Ninth Seminar on Numerical Solution of Differential and Differential-Algebraic Equations (Halle, 2000).

  36. A. Prothero and A. Robinson. On the stability and accuracy of one-step methods for solving stiff systems of ordinary differential equations. Math. Comp., 28:145–162, 1974.

    Article  MathSciNet  Google Scholar 

  37. T. Roubíček. Nonlinear Partial Differential Equations with Applications, volume 153 of International Series of Numerical Mathematics. Birkhäuser/Springer Basel AG, Basel, second edition, 2013.

  38. G. Stengle. Numerical methods for systems with measurable coefficients. Appl. Math. Lett., 3(4):25–29, 1990.

    Article  MathSciNet  Google Scholar 

  39. G. Stengle. Error analysis of a randomized numerical method. Numer. Math., 70(1):119–128, 1995.

    Article  MathSciNet  Google Scholar 

  40. J. F. Traub, G. W. Wasilkowski, and H. Woźniakowski. Information-Based Complexity. Computer Science and Scientific Computing. Academic Press, Inc., Boston, MA, 1988. With contributions by A. G. Werschulz and T. Boult.

  41. H. Triebel. Interpolation Theory, Function Spaces, Differential Operators. North-Holland Publishing Company, Amsterdam-New York, 1978.

    MATH  Google Scholar 

  42. E. Zeidler. Nonlinear Functional Analysis and its Applications. 2/A, Linear Monotone Operators. Springer-Verlag, New York, 1990.

    Book  Google Scholar 

  43. E. Zeidler. Nonlinear Functional Analysis and its Applications. 2/B, Nonlinear Monotone Operators. Springer-Verlag, New York, 1990.

    Book  Google Scholar 

Download references

Acknowledgements

The authors like to thank Wolf-Jürgen Beyn for very helpful comments on non-autonomous evolution equations and Rico Weiske for good advice on programming. Also we like to thank two anonymous referees for their valuable suggestions. This research was partially carried out in the framework of Matheon supported by Einstein Foundation Berlin. ME would like to thank the Berlin Mathematical School for the financial support. RK also gratefully acknowledges financial support by the German Research Foundation (DFG) through the research unit FOR 2402—Rough paths, stochastic partial differential equations and related topics—at TU Berlin.

Author information

Authors and Affiliations

  1. Institut für Mathematik, Technische Universität Berlin, Secr. MA 5-3, Straße des 17. Juni 136, 10623, Berlin, Germany

    Monika Eisenmann & Raphael Kruse

  2. Department of Mathematical Sciences, Chalmers University of Technology and University of Gothenburg, 412 96, Gothenburg, Sweden

    Mihály Kovács & Stig Larsson

Authors
  1. Monika Eisenmann
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mihály Kovács
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Raphael Kruse
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Stig Larsson
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Raphael Kruse.

Additional information

Communicated by Arieh Iserles.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Eisenmann, M., Kovács, M., Kruse, R. et al. On a Randomized Backward Euler Method for Nonlinear Evolution Equations with Time-Irregular Coefficients. Found Comput Math 19, 1387–1430 (2019). https://doi.org/10.1007/s10208-018-09412-w

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10208-018-09412-w

Keywords

Mathematics Subject Classification

Search

Navigation