ISS and integral-ISS of switched systems with nonlinear supply functions

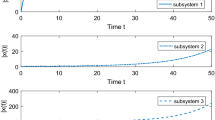
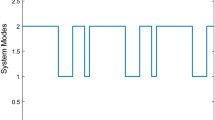
Abstract

The problem of input-to-state stability (ISS) and its integral version (iISS) are considered for switched nonlinear systems with inputs, resets and possibly unstable subsystems. For the dissipation inequalities associated with the Lyapunov function of each subsystem, it is assumed that the supply functions, which characterize the decay rate and ISS/iISS gains of the subsystems, are nonlinear. The change in the value of Lyapunov functions at switching instants is described by a sum of growth and gain functions, which are also nonlinear. Using the notion of average dwell-time (ADT) to limit the number of switching instants on an interval, and the notion of average activation time (AAT) to limit the activation time for unstable systems, a formula relating ADT and AAT is derived to guarantee ISS/iISS of the switched system. Case studies of switched systems with saturating dynamics and switched bilinear systems are included for illustration of the results.

1 Introduction

Switched systems—comprising a family of dynamical subsystems orchestrated by a piecewise constant switching signal—provide a mathematical framework for modeling systems where the state trajectories exhibit sudden transitions, either due to instant change in the vector field or due to jumps in some of the state variables [16, 17]. A canonical approach for stability analysis of such systems is to develop characterizations based on the stability properties of the individual subsystems, and the effects observed at switching times. When all subsystems are stable, the undesired instability effects may occur at switching times. In such cases, if one restricts the frequency of switching times over an interval, then the decay in the norm of state trajectories can overcome the growth at switching times. Such slow switching results are usually obtained by imposing lower bounds on the dwell-time or average dwell-time (ADT) between switching instances [13]. In the case when some subsystems are unstable, one needs to restrict the activation time or average activation time (AAT) of these unstable subsystems in order for the resulting switched system to be stable, and this has been studied in [21, 34, 35]. For switched linear systems, stability conditions based on matrix measure can also be used to determine stability when the switching signals are periodic [23], and this criterion is later extended to switching signals satisfying asymptotic AAT constraints [33]. Meanwhile, it is also possible to consider cases where the continuous dynamics of all the individual subsystems are unstable, but the impulsive effects from switching between these subsystems stabilize the system [30]. Conditions describing how fast such stabilizing impulsive effects must occur are captured under the notion of reverse average dwell-time (r-ADT), introduced in [12].

An additional important element that we incorporate in our stability analysis of switched systems is the presence of inputs or disturbances. For nonlinear systems, in general, quantitative relationships between the norm of the state trajectories and the magnitude of the inputs are appropriately captured by the notion of input-to-state stability (ISS), or its integral variant, integral input-to-state stability (iISS). These notions have been pioneered in [26] and [28], and since their inception, these tools have been used for analysis and design purposes in many control-related problems. In the context of switched systems under arbitrary switching, converse results regarding the existence of ISS and iISS Lyapunov functions first appeared in [19], and some implications relating ISS and iISS for switched systems have been developed in [8]. Switching dynamics with reset maps can also be captured by the framework of hybrid systems [7], and ISS characterizations via Lyapunov functions for hybrid systems have been developed in [3, 4]. Relaxing certain assumptions in these works, along with some developments based on converse results, iISS characterizations via Lyapunov functions for hybrid systems appear in [22]. However, these results do not explicitly work out the stability conditions for switched systems in terms of the data associated with individual subsystems.

Combining the aforementioned two directions of research, it is rather natural to ask whether the Lyapunov characterizations for individual subsystems can be combined with ADT and AAT notions to obtain sufficient conditions for ISS/iISS of switched systems with inputs. This question has indeed been addressed in the literature in different settings. When a switched system has only one mode, it becomes an impulsive system, for which the ISS and iISS properties are studied in [12, 20]. While the result [12, Theorem 6] provides conditions for the impulsive system to be iISS under arbitrary switching even if the decay rate is nonlinear, growth in the Lyapunov function when impulses occur is not allowed in that result. On the other hand, the work [20] relaxes both the decay and growth rates to be nonlinear, and concludes sufficient conditions on the impulse sequence so that the time-varying impulsive system is ISS. For switched systems with multiple modes, the paper [31] provides a lower bound on the dwell-time and the paper [29] provides a lower bound on the ADT in terms of the data associated with individual ISS subsystems so that the switched system is ISS. Later the paper [21] relaxes the assumption of all ISS subsystems and allows possible unstable subsystems. A result on ISS switched system is concluded in that work when the switching signal satisfies both ADT and AAT conditions. Nevertheless, a major restriction of these works on switched systems is that the results require the assumption that both the decay rates in the inequalities associated with the derivative of Lyapunov functions and the growth rate in the value of the Lyapunov functions at switching instants are linear. This assumption becomes overly restrictive when studying iISS.

Compared to the aforementioned references, the major contribution of this work is to study ISS and iISS of switched systems with nonlinear decay rate in the dissipation inequalities associated with the Lyapunov functions of individual subsystems. In other words, using the terminology from [27], we consider Lyapunov functions for each subsystem with nonlinear supply functions. Moreover, the change in the value of Lyapunov function at switching times is also described by nonlinear growth function. This setup was, in particular, adopted in the conference version of this paper [18], and the previous independent work of the authors [25, 36]. While [36] provides the construction of an ISS Lyapunov function to find the lower bounds on ADT for stability of cascade interconnections, the present work uses a conceptually similar approach for a broader class of switched systems and extends the study to iISS. In contrast to [25], where the analysis is carried out using trajectory-based methods with nested comparison functions, this paper makes transition from dwell-time conditions to a more quantitative ADT estimate. In our conference paper [18], we only study switched systems whose subsystems are all (i)ISS and the growth of the Lyapunov functions at the switching times is not allowed to depend on the input. Such assumptions have been relaxed in this work and consequently, a generalization of the results in [21] is obtained with a Lyapunov-function-based approach that allows us to handle additional nonlinearities in the supply functions.

The rest of the paper is organized as follows. After some necessary preliminaries about switched systems given in Sect. 2, our main result is stated in Sect. 3 with some discussions about the validity of its assumptions. Section 4 contains the technical tools used to prove our main result. Case studies of switched systems with saturating dynamics and switched bilinear systems are provided in Sect. 5 for illustration of the results, followed by the conclusion in Sect. 6.

2 Preliminaries

In this section, we introduce the basic notation of comparison functions, the definition of switched systems and switching signals, and the definitions of ISS and iISS of switched systems.

Comparison functions A function $\alpha :\mathbb {R}_{\ge 0} \rightarrow \mathbb {R}_{\ge 0}$ is said to be positive definite if it is continuous, $\alpha (0) = 0$ and $\alpha (s)>0$ for all $s>0$. If $\alpha$ is also strictly increasing, then it is said to be of class $\mathcal {K}$. In addition if $\alpha$ is also unbounded, then it is said to be of class $\mathcal {K}_\infty$. A function $\beta : \mathbb {R}_{\ge 0} \times \mathbb {R}_{\ge 0} \rightarrow \mathbb {R}_{\ge 0}$ is said to be of class $\mathcal {K}\mathcal {L}$ if $\beta (\cdot ,t)$ is of class $\mathcal {K}$ for each $t \in \mathbb {R}_{\ge 0}$, $\beta (s,\cdot )$ is continuously decreasing and $\beta (s,t) \rightarrow 0$ as $t \rightarrow \infty$ for each fixed $s \in \mathbb {R}_{\ge 0}$; see [14, Chapter 4] for their use in formulation of common stability notions. In addition, we require class $\mathcal {K}\mathcal {L}\mathcal {L}$ functions: A function $\beta :\mathbb {R}_{\ge 0} \times \mathbb {R}_{\ge 0} \times \mathbb {R}_{\ge 0} \rightarrow \mathbb {R}_{\ge 0}$ is a class $\mathcal {K}\mathcal {L}\mathcal {L}$ function if $\beta (\cdot ,\cdot ,j)$ is a class $\mathcal {K}\mathcal {L}$ function for each $j \ge 0$ and $\beta (\cdot ,t,\cdot )$ is a class $\mathcal {K}\mathcal {L}$ function for each $s \ge 0$.

Switched systems Let $\mathcal {P}\subset {\mathbb {N}}$. For each $p\in \mathcal {P}$, there is a vector field $f_p:\mathbb {R}^n\times \mathbb {R}^m\rightarrow \mathbb {R}^n$, bluewhich is jointly locally Lipschitz in its arguments. The differential equations

$$\begin{aligned} \dot{x}=f_p(x,\omega ),\quad p\in \mathcal {P}\end{aligned}$$

(1)

are the dynamics of the subsystems or modes of the switched system with input $\omega :\mathbb {R}_{\ge 0} \rightarrow \mathbb {R}^m$. For each pair $(p,q)\in \mathcal {P}\times \mathcal {P}$, there is also a continuous jump map $g_{p,q}:\mathbb {R}^n\times \mathbb {R}^m\rightarrow \mathbb {R}^n$. It is assumed that for each $p \in \mathcal {P}$, $x\in \mathbb {R}^n$ and $\omega \in \mathbb {R}^d$, the set $G_p(x,\omega ):= \{g_{p,q}(x,\omega ) \, : \, q \in \mathcal {P}\}\subset \mathbb {R}^n$ is closed. Let ${\overline{\varSigma }}$ be the set of all right-continuous, piecewise constant mappings from $\mathbb {R}_{\ge 0}$ to $\mathcal {P}$ with a locally finite number of discontinuities, called switching signals. For each switching signal $\sigma \in {\overline{\varSigma }}$, define

$$\begin{aligned} \mathcal {T}(\sigma ):=\{t> 0:\sigma (t)\ne \sigma (t^-)\} \end{aligned}$$

(2)

In other words, $\mathcal {T}(\sigma )$ is the collection of switching instants. With these data, we consider switched dynamical systems with inputs and resets, described by

$$\begin{aligned} \dot{x}(t)= & {} f_{\sigma (t)}(x(t), \omega (t))\quad \qquad \text{ if } t\not \in \mathcal {T}(\sigma ), \end{aligned}$$

(3a)

$$\begin{aligned} x(t)= & {} g_{\sigma (t^-),\sigma (t)}(x(t^-), \omega (t))\ \text{ if } t\in \mathcal {T}(\sigma ), \end{aligned}$$

(3b)

where $\omega :\mathbb {R}_{\ge 0} \rightarrow \mathbb {R}^m$ is locally essentially bounded on $\mathbb {R}_{\ge 0}$ and bounded on $\mathcal {T}(\sigma )$. We note that, over an interval $[t_0,t_1)$ where $\sigma$ is constant, (3a) is seen as a time-varying differential equation with possible discontinuities due to inputs. The solution of (3a) is, therefore, interpreted in Carathéodory sense over such an interval $[t_0,t_1)$, see [10, Sect. I.5]. With $f_p(\cdot ,\cdot )$ locally Lipschitz in both arguments, the conditions for existence of Carathéodory solutions hold [10, Theorem 5.1, Page 28]. We call (3a) the continuous flow and (3b) the discrete jumps. We denote the solution of (3) with initial state $x_0$, input $\omega$ and switching signal $\sigma$ by $x(\cdot ;x_0,\omega ,\sigma )$. When $x_0,\omega ,\sigma$ are clear from the context, the solution is also abbreviated by $x(\cdot )$.

Switching signals We now specify the class of switching signals for which we study the stability of the system (3). We write the set $\mathcal {P}$ as a disjoint union $\mathcal {P}=\mathcal {P}_{\text {s}}\cup \mathcal {P}_{\text {u}}$, and introduce two different notions which constrain the class of switching signals. Conceptually, the class of switching signals with ADT constraints puts an upper bound on the average number of switches over any time interval. According to [13], we say that a switching signal $\sigma$ satisfies average dwell-time (ADT) condition with parameters $\tau _a>0$ (average dwell-time) and $N_0\ge 1$ if

$$\begin{aligned} \forall t_2\ge t_1\ge 0:\quad N_{\sigma }(t_1,t_2)\le N_0+\frac{t_2-t_1}{\tau _a}, \end{aligned}$$

(4)

where $N_{\sigma }(t_1,t_2)$ is the number of switches occurring in the interval $(t_1,t_2]$: $N_{\sigma }(t_1,t_2):=|(t_1,t_2]\cap \mathcal {T}(\sigma )|$. We denote $\varSigma _{\text {ADT}}(\tau _a,N_0)$ to be the collection of all ADT switching signals with parameters $\tau _a,N_0$.

The second constraint provides a bound on the activation time for the modes contained in $\mathcal {P}_{\mathrm{u}}$. Following the definition in [21], we say that a switching signal $\sigma$ satisfies average activation time (AAT) condition with parameters $\eta \in [0,1]$ (percentage activation time), $T_0\ge 0$, if

$$\begin{aligned} \forall t_2\ge t_1\ge 0:\quad T_{\sigma ,\mathcal {P}_{\text {u}}}(t_1,t_2)\le T_0+\eta (t_2-t_1), \end{aligned}$$

(5)

where $T_{\sigma ,\mathcal {P}_{\text {u}}}(t_1,t_2)$ is the activation time of modes in $\mathcal {P}_{\text {u}}$ over the interval $(t_1,t_2]$. More precisely, if we consider the function $\mathbf{1} _{\mathcal {P}_{\text {u}}} : \mathcal {P}\rightarrow \{0,1\}$, defined as

$$\begin{aligned} \mathbf{1} _{\mathcal {P}_{\text {u}}}(\sigma ) = {\left\{ \begin{array}{ll} 1, &{} \text { if } \sigma \in \mathcal {P}_{\text {u}}, \\ 0, &{} \text { otherwise}, \end{array}\right. } \end{aligned}$$

(6)

then $T_{\sigma ,\mathcal {P}_{\text {u}}}(t_1,t_2):= \int _{t_1}^{t_2}{} \mathbf{1} _{\mathcal {P}_{\text {u}}} (\sigma (\tau )) d\tau$. We denote $\varSigma _{\text {AAT}}(\mathcal {P}_{\text {u}},\eta ,T_0)$ to be the collection of all AAT switching signals of modes in $\mathcal {P}_{\text {u}}$ with parameters $\eta ,T_0$. Notice that the AAT condition is always with respect to the set $\mathcal {P}_{\text {u}}$, which we later associate with the collection of subsystems with not necessarily stable dynamics. In addition, in the special case when $\eta =1$, (5) imposes no constraints on the activation time and hence the switching signal can be arbitrary. On the other hand, when $T_0=0$ and $\eta <1$, (5) implies that modes in $\mathcal {P}_{\text {u}}$ are not activated at all.

Stability definitions Let $\varSigma \subset {\overline{\varSigma }}$ be a set of switching signals. In this paper, we will focus on the following two uniform external stability properties of switched systems with respect to all switching signals in $\varSigma$. In what follows, the essential supremum norm of $\omega$ over a set I (that is, the supremum of $\omega$ on I except for a set of measure zero) is denoted by ${{\,\mathrm{ess\,sup}\,}}_{s\in I} \vert \omega (s) \vert$.

Definition 1

A switched system (3) is uniformly input-to-state stable (ISS) over $\varSigma$ if there exist $\beta \in \mathcal {KL},\gamma _1,\gamma _2\in {\mathcal {K}}$ such that

$$\begin{aligned}&|x(t;x_0,\omega ,\sigma )|\le \beta (|x_0|,t)\nonumber \\&\quad +\gamma _1({{\,\mathrm{ess\,sup}\,}}_{s\in [0,t]\backslash \mathcal {T}(\sigma )} |\omega (s)|)+\gamma _2\left( \sup _{s\in [0,t]\cap \mathcal {T}(\sigma )}|\omega (s)|\right) \end{aligned}$$

(7)

for all $t\ge 0,x_0\in \mathbb {R}^n$ and $\sigma \in \varSigma$.

Definition 2

A switched system (3) is uniformly integral input-to-state stable (iISS) over $\varSigma$ if there exist $\alpha _0\in {\mathcal {K}}_{\infty },\beta \in \mathcal {KL},\gamma _1,\gamma _2\in {\mathcal {K}}$ such that

$$\begin{aligned} \alpha _0(|x(t;x_0,\omega ,\sigma )|)\le \beta (|x_0|,t)+\int _0^t \gamma _1(|\omega (s)|)\hbox {d}s +\sum _{s\in [0,t]\cap \mathcal {T}(\sigma )} \gamma _2 (\vert \omega (s)\vert ) \end{aligned}$$

(8)

for all $t\ge 0,x_0\in \mathbb {R}^n$, and $\sigma \in \varSigma$.

Our definitions of ISS and iISS are adopted from [3] and [22]. Notice that the last terms in (7), and in (8) do not appear in standard definitions of ISS and iISS for non-switched systems; these additional terms are needed in our framework to capture the growth of state trajectories at switching instants due to the presence of inputs in the jump dynamics (3b). We also remark here that both ISS and iISS can be defined in an even stronger way that the magnitude of the solution is in addition required to decrease with respect to the total number of switches so far. However, such stronger definitions are not necessary for the development of our results. Some discussion around this issue appears later in Remark 5.

3 Assumptions and main results

We now state some assumptions on the data of system (3) required for the statement of the main result.

Assumption 1

There exist $\mathcal {C}^1$ Lyapunov functions $V_p:\mathbb {R}^n\rightarrow \mathbb {R}_{\ge 0}$, $p\in \mathcal {P}$, satisfying the conditions:

• (L1) There exist ${\underline{\alpha }},{\overline{\alpha }}\in \mathcal {K}_\infty$ such that

  $$\begin{aligned} {\underline{\alpha }}(|x|)\le V_p(x)\le {\overline{\alpha }}(|x|),\quad \forall x\in \mathbb {R}^n,p\in \mathcal {P}. \end{aligned}$$

  (9)

• (L2) There exist a disjoint partition $\mathcal {P}=\mathcal {P}_{\text {s}}\cup \mathcal {P}_{\text {u}}$, two positive definite functions $\alpha _{\text {s}},\alpha _{\text {u}}$, and $\gamma \in \mathcal {K}$ such that

  $$\begin{aligned} \Big \langle \frac{\partial }{\partial x} V_p(x),f_p(x,\omega ) \Big \rangle \le -\alpha _{\text {s}}(V_p(x))+\gamma (|\omega |)\quad \forall x\in \mathbb {R}^n,\omega \in \mathbb {R}^m, p\in \mathcal {P}_{\text {s}},\nonumber \\ \end{aligned}$$

  (10a)

  and

  $$\begin{aligned} \Big \langle \frac{\partial }{\partial x} V_p(x),f_p(x,\omega ) \Big \rangle \le \alpha _{\text {u}}(V_p(x))+\gamma (|\omega |)\quad \forall x\in \mathbb {R}^n,\omega \in \mathbb {R}^m, p\in \mathcal {P}_{\text {u}}.\nonumber \\ \end{aligned}$$

  (10b)

• (L3) There exist $\chi \in \mathcal {K}_\infty ,\rho \in \mathcal {K}$ such that

  $$\begin{aligned} V_q(g_{p,q}(x,\omega ))\le \chi (V_p(x))+\rho (|\omega |) \quad \forall x\in \mathbb {R}^n,\omega \in \mathbb {R}^m,p,q\in \mathcal {P}. \end{aligned}$$

  (11)

Remark 1

For each subsystem in $\mathcal {P}_{\text {s}}$, (L1) and the condition (10a) in (L2) provide a necessary and sufficient condition for it to be iISS [2]. In addition, if it is assumed that $\alpha _{\text {s}}$ in (10a) is $\mathcal {K}_{\infty }$, then each subsystem in $\mathcal {P}_{\text {s}}$ is ISS.

Remark 2

The partition $\mathcal {P}_{\text {s}},\mathcal {P}_{\text {u}}$ is not necessarily an indicator whether a subsystem is iISS or not. Indeed, when a subsystem is not iISS, (10a) will not hold for any choice of $V_p$ but (10b) may still hold. However, even if a subsystem is iISS, it may still lead to the inequality (10b) due to the improper choice of $V_p$. In this case this subsystem needs to be categorized to the set $\mathcal {P}_{\text {u}}$.

Based on Assumption 1, we introduce the function $\psi :\mathbb {R}_{\ge 0} \rightarrow \mathbb {R}_{\ge 0}$ as

$$\begin{aligned} \psi (t):=\min _{r\in [0,t]}\{\alpha _{\text {s}}(r)+c(t-r)\} \end{aligned}$$

(12)

where $c >0$ is some constant. The next assumption bounds the divergence rate for the subsystems in $\mathcal {P}_{\text {u}}$:

Assumption 2

The supremum

$$\begin{aligned} \kappa :=\sup _{r>0}\frac{\alpha _{\text {u}}(r)}{\psi (r)} \end{aligned}$$

(13)

is finite, where $\alpha _{\text {u}}$ comes from (L2) in Assumption 1 and $\psi$ is defined in (12).

Our last assumption will be instrumental in deriving a lower bound for ADT in a similar fashion as in [36]:

Assumption 3

The supremum

$$\begin{aligned} \zeta ^*:=\sup _{s> 0}\int _s^{\chi (s)}\frac{1}{\psi (r)}\hbox {d}r \end{aligned}$$

(14)

is finite, where $\chi$ comes from (L3) in Assumption 1 and $\psi$ is defined in (12).

We are now ready to state the main theorem of this paper:

Theorem 1

Consider the switched system (3) and suppose that Assumptions 1, 2, and 3 hold with the function $\psi$ constructed via (12) for some $c>0$. If $\tau _a>\zeta ^*$, then for every $\eta \in [0,1]$ satisfying the inequality

$$\begin{aligned} (1+\kappa )\eta +\frac{\zeta ^*}{\tau _a}<1, \end{aligned}$$

(15)

the system (3) is uniformly iISS over $\varSigma _{\text {ADT}}(\tau _a,N_0) \cap \varSigma _{\text {AAT}}(\mathcal {P}_{\text {u}},\eta ,T_0)$ for any $N_0\ge 1$ and $T_0\ge 0$. Moreover, if in (L2) $\alpha _{\text {s}}\in \mathcal {K}_\infty$, and $\tau _a > \zeta ^*$, then system (3) is uniformly ISS over $\varSigma _{\text {ADT}}(\tau _a,N_0)\cap \varSigma _{\text {AAT}}(\mathcal {P}_{\text {u}},\eta ,T_0)$ for every $\eta \in [0,1]$ satisfying (15) and any $N_0\ge 1,T_0\ge 0$.

To provide some insight about Theorem 1, let us consider its application to some special types of switched systems. We first consider the case when all the subsystems are ISS/iISS. In this case, we can always find $V_p$ satisfying (10a) in (L2) for all $p\in \mathcal {P}$ so that $\mathcal {P}_{\text {u}}=\emptyset$. Hence, the AAT condition vanishes and we could simply set $\eta =0$. As a result, (15) reduces to $\tau _a>\zeta ^*$. In other words, in this case the switched system (3) is uniformly ISS/iISS over $\varSigma _{\text {ADT}}(\tau _a,N_0)$ for any $\tau _a>\zeta ^*,N_0\ge 1$.

We next consider the case when the switching does not introduce jumps in the Lyapunov functions evaluated along the solutions of the unforced system, so that $\chi =\text {id}$. We then conclude from (14) that $\zeta ^*=0$, so by Theorem 1, the switched system is ISS/iISS with arbitrarily small values of the average dwell-time $\tau _a$. In addition, (15) results in $\eta <\frac{1}{1+\kappa }$ and the switched system (3) is uniformly ISS/iISS over $\big (\cup _{\tau _a>0}\varSigma _{\text {ADT}}(\tau _a,N_0)\big )\cap \varSigma _{\text {AAT}}(\mathcal {P}_{\text {u}},\eta ,T_0)$.

Lastly, we consider the case when the decaying rates and growth rates in (L2), (L3) of Assumption 1 are all linear; that is, there exist $\lambda _{\text {s}},\lambda _{\text {u}},\mu >0$ such that $\alpha _{\text {s}}(r)=\lambda _{\text {s}}r,\alpha _{\text {u}}(r)=\lambda _{\text {u}}r,\chi (r)=\mu r$ for all $r\ge 0$. In this case, the formula (14) yields

$$\begin{aligned} \zeta ^*=\int _s^{\mu s}\frac{1}{\lambda _{\text {s}}r}\hbox {d}r=\frac{\ln \mu }{\lambda _{\text {s}}} \end{aligned}$$

and consequently the inequality (15) becomes

$$\begin{aligned} \left( 1+\frac{\lambda _{\text {u}}}{\lambda _{\text {s}}}\right) \eta + \frac{\ln \mu }{\lambda _{\text {s}}\tau _a}<1. \end{aligned}$$

After rearranging the terms, we recover the conditions on $\tau _a$ and $\eta$ which guarantee ISS switched system as stated in [21, Theorem 2]. Thus, our work turns out to be a generalization from linear to nonlinear setting of the known results in the literature.

Discussion of the assumptions Once we impose some characterizations of the stability or instability of individual subsystems and the reset maps in Assumption 1, it is seen that the stability condition in (15) primarily depends on finiteness of $\kappa$ in Assumption 2 and $\zeta ^*$ in Assumption 3. In both the assumptions, the function $\psi$ constructed in (12) plays a key role.

• 1. Note that (12) immediately implies that $\psi (t) \le {\overline{\psi }}(t) := \min \{\alpha _{\text {s}}(t), ct\}$. In [18], we proposed stability conditions using the function ${\overline{\psi }}$ while assuming that it is globally one-sided Lipschitz. That assumption is necessary in [18]; for example, take $\alpha _{\text {s}}(r)=re^{-r}+1-\cos (r^2)$, then ${\overline{\psi }}(t)$ is not one-sided Lipschitz for any $c>0$. In this work, the Lipschitzness assumption is no longer required because the function $\psi$ in (12) has this property by construction, which will be shown later by Lemma 7.

• 2. The function $\psi$ is essentially a global one-sided Lipschitz modification of $\alpha _{\text {s}}$. In case $\alpha _{\text {s}}$ is already globally Lipschitz, $\psi$ can be chosen equal to $\alpha _{\text {s}}$ by setting c larger than its Lipschitz constant. Functions like $\alpha _{\text {s}}(r)=r, \alpha _{\text {s}}(r) = \ln (1+r)$ and $\alpha _{\text {s}}(r)=re^{-r}$ are examples of such a case.

• 3. Combining (12) and (13), we conclude from (10b) that for all $p\in \mathcal {P}_{\text {u}}$, $\Big \langle \frac{\partial }{\partial x} V_p(x),f_p(x,\omega ) \Big \rangle \le \kappa cV_p(x)+\gamma (|\omega |)$. According to [1, Theorem 1], this property in turn implies that these subsystems are forward complete, which is a very reasonable assumption on the subsystems as one cannot expect the switched system to be ISS/iISS if an active subsystem has finite escape time.

• 4. In order to get a qualitative answer to the question of when the switched system is ISS/iISS under slow switching, we need to better understand Assumption 3. The next lemma states that in order to show that $\zeta ^*$ is finite, we only need to examine the values of $\psi (s),\chi (s)$ for small and large s without computing the integral in (14). To this end, we say a positive definite function $\alpha :\mathbb {R}_{\ge 0}\rightarrow \mathbb {R}_{\ge 0}$ is initially increasing if there exists $r>0$ such that $\alpha (s)\le \alpha (t)$ for all $0\le s\le t\le r$, and eventually increasing (resp. eventually decreasing) if there exists $R>0$ such that $\alpha (s)\le \alpha (t)$ (resp. $\alpha (s)\ge \alpha (t)$) for all $R\le s\le t$.

Lemma 2

The value $\zeta ^*$ defined in (14) is finite when both conditions (a) and (b) hold:

• (a) $\psi$ is initially increasing and $\limsup _{s\rightarrow 0^+}\frac{\chi (s)-s}{\psi (s)}<\infty$, and

• (b) $\psi$ is eventually increasing and $\limsup _{s\rightarrow \infty }\frac{\chi (s)-s}{\psi (s)}<\infty$, or $\psi$ is eventually decreasing and $\limsup _{s\rightarrow \infty }\frac{\chi (s)-s}{\psi (\chi (s))}< \infty$.

Proof

We only study the non-trivial case when $\chi (s)>s$ for all $s>0$. By inspecting the definition of $\zeta ^*$ in (14), we see that it suffices to show that $\limsup _s \int _s^{\chi (s)}\frac{1}{\psi (r)}\hbox {d}r$ is finite when s approaches both $0^+$ and infinity in order for $\zeta ^*$ to be finite. When $\psi$ is initially increasing, we have ${\overline{r}}>0$ such that for all $s>0$ with $\chi (s)\le {\overline{r}}$,

$$\begin{aligned} \int _s^{\chi (s)}\frac{1}{\psi (r)}\hbox {d}r\le \int _s^{\chi (s)}\frac{1}{\psi (s)}\hbox {d}r=\frac{\chi (s)-s}{\psi (s)}. \end{aligned}$$

Take the limit as $s\rightarrow 0^+$, and it follows from the first condition in Lemma 2 that $\limsup _{s\rightarrow 0^+} \int _s^{\chi (s)}\frac{1}{\psi (r)}\hbox {d}r$ is bounded. Similarly, the second condition results in $\limsup _{s\rightarrow \infty } \int _s^{\chi (s)}\frac{1}{\psi (r)}\hbox {d}r$ being bounded. $\square$

It is not hard to see that $\zeta ^*$ is finite for the linear functions when $\psi (s)=\lambda s, \chi (s)=\mu s$. In addition, using Lemma 2, it is straightforward to verify that the nonlinear pairs such as $(\psi (s),\chi (s))=(1-e^{-s},s+1-e^{-s})$, $(e^{-s}(1-e^{-s}),\ln (e^s+1))$ also ensure finite $\zeta ^*$.

4 Technical tools and proofs

Our proof of Theorem 1 relies on rewriting the switched system into a hybrid system in the framework of [7] and then showing ISS/iISS of that hybrid system. In this section, we provide a brief description of hybrid systems, followed by some supporting lemmas and the proof of Theorem 1.

4.1 Hybrid systems with inputs

Consider the hybrid dynamical system with inputs, which is described as:

$$\begin{aligned} {\left\{ \begin{array}{ll} {\dot{\xi }}\in {\mathcal {F}}(\xi ,d), &{} \ (\xi ,d)\in {\mathcal {C}}, \\ \xi ^+\in {\mathcal {G}}(\xi ,d), &{} \ (\xi ,d)\in {\mathcal {D}}, \end{array}\right. } \end{aligned}$$

(16)

where the state trajectory $\xi$ evolves in $\mathcal {X}\subset \mathbb {R}^n$, and the disturbance d takes values in $\mathbb {R}^m$. The sets $\mathcal {C}$, $\mathcal {D}$ are subsets of $\mathcal {X}\times \mathbb {R}^m$ and are called flow and jump sets, respectively. The evolution of the state is thus described by $\mathcal {F}$ (during flows) and by $\mathcal {G}$ (at jump instants), which are set-valued mappings from $\mathcal {X}\times \mathbb {R}^m$ to $\mathcal {X}$. The solutions of the hybrid system (16) are defined on hybrid time domains. A set $I \subset \mathbb {R}_{\ge 0} \times \mathbb {Z}_{\ge 0}$ is called a compact hybrid time domain if $I = \cup _{j = 0}^{J-1}([t_j,t_{j+1}],j)$ for some finite sequence of times $0 = t_0 \le t_1 \cdots \le t_{J}$; and I is a hybrid time domain if for all $(T,J) \in I$, $I \cap ([0,T] \times \{0, 1, \dots , J\})$ is a compact hybrid time domain. The domain of the hybrid input d in (16) is a hybrid time domain, and $d : {{\,\mathrm{dom}\,}}d \rightarrow \mathbb {R}^m$ is such that $d(\cdot , j)$ is Lebesgue measurable and locally essentially bounded for each $j \in \mathbb {Z}_{\ge 0}$. When the system data $(\mathcal {F}, \mathcal {G}, \mathcal {C}, \mathcal {D})$ satisfies certain basic assumptions [7, Assumption 6.5], [3, Page 48], it holds that, for each input $d:{{\,\mathrm{dom}\,}}d \rightarrow \mathbb {R}^m$, the system (16) admits a local solution, called hybrid arc, $\xi : {{\,\mathrm{dom}\,}}\xi \rightarrow \mathcal {X}$. A hybrid arc $\xi : {{\,\mathrm{dom}\,}}\xi \rightarrow \mathcal {X}$ and a hybrid input $d :{{\,\mathrm{dom}\,}}d \rightarrow \mathbb {R}^m$ are a solution pair to the hybrid model (16) if:

• ${{\,\mathrm{dom}\,}}\xi = {{\,\mathrm{dom}\,}}d$;

• for all $j \in Z_{\ge 0}$ and almost all $t\in \mathbb {R}_{\ge 0}$ such that $(t, j)\in {{\,\mathrm{dom}\,}}\xi$, we have $(\xi (t, j), d(t, j)) \in \mathcal {C}$ and ${\dot{\xi }} (t, j) \in \mathcal {F}(\xi (t, j), d(t, j))$; and

• for $(t, j) \in {{\,\mathrm{dom}\,}}\xi$ such that $(t,j + 1) \in {{\,\mathrm{dom}\,}}\xi$ we have $(\xi (t,j),d(t,j)) \in \mathcal {D}$ and $x(t,j + 1) \in \mathcal {G}(\xi (t, j), d(t, j))$.

For an initial state $\xi _0$ and an input d, a hybrid arc of (16) is denoted by $\xi (\cdot ,\cdot ;\xi _0,d)$. In what follows, we denote the distance between a vector $\xi \in \mathcal {X}$ and a compact set $\mathcal {A}\subset \mathcal {X}$ by $\vert \xi \vert _{\mathcal {A}}$, that is, $\vert \xi \vert := \inf _{\zeta \in \mathcal {A}} \vert \xi - \zeta \vert$. Following [3], for a hybrid signal d, we use the notation $\Vert d \Vert _{(t, j)}$ to denote the maximum between $\displaystyle {{\,\mathrm{ess\,sup}\,}}_{({{\hat{t}}}, {\hat{j}}+1) \not \in {{\,\mathrm{dom}\,}}d, {\hat{t}}+ {\hat{j}} \le t + j} \vert d({\hat{t}}, {\hat{j}}) \vert$ and $\displaystyle \sup \nolimits _{({\hat{t}}, {\hat{j}}+1) \in {{\,\mathrm{dom}\,}}d, {\hat{t}}+ {\hat{j}}\le t+ j} \vert d({\hat{t}}, {\hat{j}}) \vert$.

Definition 3

A hybrid system (16) is said to be input-to-state stable (ISS) with respect to $\mathcal {A}$ if there exist $\beta _h\in \mathcal {KLL}$ and $\gamma _h\in {\mathcal {K}}$ such that for all $\xi _0\in {\mathcal {X}}$ and all $(t,j)\in {{\,\mathrm{dom}\,}}\xi$, each solution pair $(\xi ,d)$ satisfies

$$\begin{aligned} |\xi (t,j;\xi _0,d)|_{\mathcal {A}}\le \beta _h(|\xi _0|_{\mathcal {A}},t,j)+\gamma _h(\Vert d \Vert _{(t, j)}). \end{aligned}$$

(17)

Definition 4

A hybrid system (16) is said to be integral input-to-state stable (iISS) with respect to $\mathcal {A}$ if there exist $\alpha _h\in {\mathcal {K}}_\infty , \beta _h\in \mathcal {KLL}$ and $\gamma _h\in {\mathcal {K}}$ such that for all $\xi _0\in {\mathcal {X}}$ and all $(t,j)\in \text{ dom } \xi$, each solution pair $(\xi ,d)$ satisfies

$$\begin{aligned} \alpha _h(|\xi (t,j;\xi _0,d)|_{\mathcal {A}})\le & {} \beta _h(|\xi _0|_{\mathcal {A}},t,j)+\int _0^t\gamma _h(|d(s,j_{d,s})|)\hbox {d}s \nonumber \\&+ \sum _{\begin{array}{c} ({\hat{t}}, {\hat{j}}+1) \in {{\,\mathrm{dom}\,}}d \\ {\hat{t}}+ {\hat{j}}\le t+ j \end{array}} \gamma _h (\vert d ({\hat{t}}, {\hat{j}}) \vert ) \end{aligned}$$

(18)

where $j_{d,s} := \max \{ i \in \mathbb {N}\, \vert \, (s,i) \in {{\,\mathrm{dom}\,}}d\}$.

We can also characterize ISS and iISS using Lyapunov functions. The following result provides sufficient conditions in terms of existence of Lyapunov functions with certain properties which guarantee ISS/iISS.

Lemma 3

[3, 22] A hybrid system (16) is iISS with respect to $\mathcal {A}$ if there exists a smooth function $V:{\mathcal {X}}\rightarrow {\mathbb {R}}_{\ge 0}$, positive definite functions $\alpha _c,\alpha _d$, functions $\alpha _1,\alpha _2\in {\mathcal {K}}_\infty$ and $\gamma _c,\gamma _d\in {\mathcal {K}}$ such that

$$\begin{aligned} \alpha _1(|\xi |_{\mathcal {A}})\le V(\xi )\le \alpha _2(|\xi |_{\mathcal {A}}) \end{aligned}$$

(19)

for all $\xi \in {\mathcal {X}}$,

$$\begin{aligned} \left\langle \frac{\partial }{\partial \xi } V(\xi ),{\overline{f}} \right\rangle \le -\alpha _c(|\xi |_{\mathcal {A}})+ \gamma _c(|d|) \end{aligned}$$

(20)

for all $(\xi ,d)\in {\mathcal {C}}, {\overline{f}}\in {\mathcal {F}}(\xi ,d)$, and

$$\begin{aligned} V({\overline{g}})-V(\xi )\le -\alpha _d(|\xi |_{\mathcal {A}}) + \gamma _d(|d|) \end{aligned}$$

(21)

for all $(\xi ,d)\in {\mathcal {D}},{\overline{g}}\in {\mathcal {G}}(\xi ,d)$. In addition if $\alpha _c,\alpha _d\in {\mathcal {K}}_\infty$, then the hybrid system (16) is ISS with respect to $\mathcal {A}$.

We call a function V satisfying the inequalities in Lemma 3 with positive definite functions $\alpha _c,\alpha _d$ (resp. $\alpha _c,\alpha _d\in {\mathcal {K}}_\infty$) a hybrid iISS (resp. ISS) Lyapunov function.

Remark 3

In papers [3, 22], the authors work with a single-valued version of (16), that is, $\mathcal {F}(\xi ,d) = f(\xi ,d)$ and $\mathcal {G}(\xi ,d) = g(\xi ,d)$. The statements in Lemma 3 are obvious extensions of those results. Additionally, we see a condition on system data in the results of [3, 22], which says that, for each $\xi \in \mathcal {X}$ and each $\epsilon \ge 0$, the set $\{f(\xi ,d) \, \vert \, d \in \mathbb {R}^m \cap \epsilon \overline{{\mathbb {B}}}\}$ is convex. However, this condition is only required for proving the converse implication that ISS/iISS implies the existence of an appropriate Lyapunov function, and is not needed for our purposes.

4.2 Hybrid models for ADT and AAT

We now model the switched systems with ADT and AAT constraints as a hybrid system (16). It is assumed that $\mathcal {P}= \mathcal {P}_{\text {u}} \cup \mathcal {P}_{\text {s}}$, and we recall that $\mathbf{1} _{\mathcal {P}_{\text {u}}} (\cdot )$, defined in (6), is the indicator function associated with unstable modes indexed by $\mathcal {P}_{\text {u}}$. Recall that the definitions of ADT and AAT constrained switching signals in (4) and (5) depend on the positive integer $N_0$ and the scalar $T_0$. We consider an autonomous hybrid system evolving on $\mathcal {X}:=\mathcal {P}\times [0,N_0] \times [0,T_0]$ with state denoted by $(p, \tau , \tau _u )$. The dynamics of this hybrid system is given by

$$\begin{aligned}&{\left\{ \begin{array}{ll} \dot{p} = 0, \\ {\dot{\tau }} \in [0,\frac{1}{\tau _a}], \\ {\dot{\tau }}_u \in [\mathbf{1} _{\mathcal {P}_{\text {u}}}(p)-\eta ,\mathbf{1} _{\mathcal {P}_{\text {u}}}(p)], \end{array}\right. }&\xi \in \mathcal {C}, \end{aligned}$$

(22a)

$$\begin{aligned}&{\left\{ \begin{array}{ll} p^+ \in \mathcal {P}, \\ \tau ^+ = \tau -1,\\ \tau _u^+ = \tau _u, \end{array}\right. }&\xi \in \mathcal {D}\end{aligned}$$

(22b)

where the parameters $\tau _a,\eta$ come from (4), (5) and the flow and jump sets are given by $\mathcal {C}:=\mathcal {P}\times [0,N_0] \times [0,T_0]$, $\mathcal {D}:=\mathcal {P}\times [1,N_0] \times [0,T_0]$, respectively. Essentially, in our model (22), we are introducing two timers: $\tau$ and $\tau _u$. This idea of using two timers can be found in the work [24], where it is shown that while the timer $\tau$ confines the switching signals with ADT constraint, the timer $\tau _u$ confines the switching signals with AAT constraint. Recall the definition of $\mathcal {T}(\sigma )$ in (2) and denote $\mathcal {T}(\sigma )=:\{t_1,t_2,\cdots \}$ with $t_1< t_2< \cdots$ and denote $t_0:=0$. In addition we abuse the notation that when $|\mathcal {T}(\sigma )|<\infty$, $[t_{|\mathcal {T}(\sigma )|},t_{|\mathcal {T}(\sigma )+1|}]:=[t_{|\mathcal {T}(\sigma )|},\infty )$. The next proposition formalizes the relation between the complete solutions of the hybrid system (22) and the switching signals satisfying both ADT and AAT conditions.

Proposition 4

For every switching signal $\sigma \in \varSigma _{\text {ADT}}(\tau _a,N_0)\cap \varSigma _{\text {AAT}}(\mathcal {P}_{\text {u}},\eta ,T_0)$, there exists a complete solution $\xi =(p,\tau ,\tau _u)$ to the hybrid system (22) with the hybrid domain ${{\,\mathrm{dom}\,}}\xi =\cup _{j=0}^{|\mathcal {T}(\sigma )|}[t_j,t_{j+1}]\times \{j\}$ and it satisfies that

$$\begin{aligned} p(t,j)=\sigma (t_j) \quad \forall (t,j)\in {{\,\mathrm{dom}\,}}\xi . \end{aligned}$$

(23)

On the other hand, for every complete solution $\xi =(p,\tau ,\tau _u)$ to the hybrid system (22), denote its hybrid domain by ${{\,\mathrm{dom}\,}}\xi :=\cup _{j=0}^{J}[t_j,t_{j+1}]\times \{j\}$, where $[t_J, t_{J+1}] = [t_J,\infty )$ if $J < \infty$. Define a switching signal

$$\begin{aligned} \sigma (t):=p(t,j)\quad \forall \, t\in [t_j,t_{j+1}),\,j=1,\cdots ,J \end{aligned}$$

(24)

then $\sigma \in \varSigma _{\text {ADT}}(\tau _a,N_0)\cap \varSigma _{\text {AAT}}(\mathcal {P}_{\text {u}},\eta ,T_0)$.

Proof

As shown in [7, Example 2.15], the dynamics of p and $\tau$ guarantees the equivalence between complete solutions of the hybrid system (22) and switching signals $\sigma \in \varSigma _{\text {ADT}}(\tau _a,N_0)$ such that $p(t,j)=\sigma (t)$. It remains to show that the dynamics on $\tau _u$ confines p(t, j) to be equivalent to an AAT switching signal, which is given by [24, Lemma 7]. $\square$

Remark 4

We make a comparison between our model with two independent timers and the one proposed in [32], where a single timer is developed in the context of a small-gain theorem in [32] which imitates a switching signal under both ADT and AAT constraints. We acknowledge here that although the models are different, Proposition 1 in [32] is analogous to Proposition 4 in our paper. It turns out that for our purpose (see Sect. 4.4), using two individual timers makes the argument fairly intuitive and straightforward.

Using the result of Proposition 4, one can write the switched system (3), along with the ADT and AAT constraints on the switching signal, more naturally in the form of (16). To do so, we let

$$\begin{aligned} \xi :=\begin{pmatrix}y\\ p\\ \tau \\ \tau _u \end{pmatrix}\in \mathbb {R}^n\times \mathcal {P}\times [0,N_0] \times [0,T_0]=:\mathcal {X}. \end{aligned}$$

(25)

The hybrid model capturing the dynamics of the switched system, driven by an $\mathbb {R}^m$-valued disturbance d and a switching signal $\sigma \in \varSigma _{\text {ADT}}(\tau _a,N_0)\cap \varSigma _{\text {AAT}}(\mathcal {P}_{\text {u}}, \eta , T_0)$, is compactly written as:

$$\begin{aligned} \dot{\xi }\in {\mathcal {F}}(\xi ,d):&\left\{ \begin{array}{l} \dot{y}=f_p(y,d),\\ \dot{p}=0,\\ {\dot{\tau }}\in [0,\frac{1}{\tau _a}], \\ {\dot{\tau }}_u \in [{\mathbf {1}}_{\mathcal {P}_{\text {u}}}(p)-\eta ,{\mathbf {1}}_{\mathcal {P}_{\text {u}}}(p)] , \end{array}\right. \quad (\xi ,d)\in {\mathcal {C}}, \end{aligned}$$

(26a)

$$\begin{aligned} \xi ^+\in {\mathcal {G}}(\xi ,d):&\left\{ \begin{array}{l} y^+\in G_p(y,d),\\ p^+\in \mathcal {P},\\ \tau ^+=\tau -1,\\ \tau _u^+ = \tau _u, \end{array}\right. \qquad \qquad \quad (\xi ,d)\in {\mathcal {D}}, \end{aligned}$$

(26b)

where ${\mathcal {C}}:=\mathcal {X}\times {\mathbb {R}}^m,{\mathcal {D}}:={\mathbb {R}}^n\times \mathcal {P}\times [1,N_0]\times [0,T_0] \times {\mathbb {R}}^m$, and $G_p(y,d) = \bigcup _{q \in \mathcal {P}} g_{p,q} (y,d)$. For the system (26), it is of interest to study whether the compact set

$$\begin{aligned} \mathcal {A}:=\{0\}^n\times \mathcal {P}\times [0,N_0] \times [0,T_0] \end{aligned}$$

(27)

is an attractor. We observe that system (26) satisfies basic assumptions listed in [7, Assumption 6.5]:

• The sets $\mathcal {C}$ and $\mathcal {D}$ are closed.

• The mapping $\mathcal {F}(\cdot ,\cdot )$ is outer semi-continuous, convex-valued and locally bounded relative to $\mathcal {C}$, and $\mathcal {C}\subseteq {{\,\mathrm{dom}\,}}(\mathcal {F})$. Here, we used the fact that, for each $p \in \mathcal {P}$, the mapping $(y,d) \mapsto f_p(y,d)$ is locally Lipschitz continuous by assumption, and as already noted in (22), the set-valued dynamics for $(p,\tau ,\tau _u)$ are outer semi-continuous.

• The mapping $\mathcal {G}(\cdot ,\cdot )$ is outer semi-continuous and locally bounded relative to $\mathcal {D}$. This is because, for each $p \in \mathcal {P}$, $y \in \mathbb {R}^n$, $d\in \mathbb {R}^m$, we assumed that $G_p(y,d)$ is closed and hence, the mapping $(y,d) \mapsto G_p(y,d)$ is outer semi-continuous. Because of the discrete topology of the set $\mathcal {P}$, it then follows that $(p,y,d)\mapsto G_p(y,d)$, as a subset of $\mathcal {P}\times \mathbb {R}^n \times \mathbb {R}^m$, is closed, and outer semi-continuity of $\mathcal {G}(\cdot ,\cdot )$ follows by checking that its graph is closed.

Comparing (17) and (18) with (7) and (8), respectively, we have the following straightforward result, which shows that studying ISS (resp. iISS) for system (3) is equivalent to studying ISS (resp. iISS) for system (26).

Corollary 5

The switched system (3) is uniformly ISS (resp. iISS) over the class of switching signals $\varSigma _{\text {ADT}}(\tau _a,N_0) \cap \varSigma _{\text {AAT}}(\mathcal {P}_{\text {u}}, \eta , T_0)$ if the hybrid system (26), with augmented state variable defined in (25), is ISS (resp. iISS) with respect to $\mathcal {A}$.

Proof

We show that a solution of the switched system (3) satisfies the estimate (7) if the hybrid system (26) is ISS with respect to $\mathcal {A}$. The proof for iISS follows by similar arguments. Let $x(\cdot ; x_0, \omega ,\sigma )$ be a solution of the switched system. From Proposition 4, we see that x is equivalent to a complete solution of the hybrid system (26) with a hybrid input signal d defined by $d(0,0) := \omega (0)$, $d(t,j) := \omega (t)$ for $t \in [t_j, t_{j+1})$, where we recall that $\{t_1,t_2,\cdots \}=\mathcal {T}(\sigma )$ is the set of switching instants, in the sense that (23) holds and $y(0,0)=x_0,y(t,j)=x(t;x_0,\omega ,\sigma )$ for all $t\in [t_j,t_{j+1})$. With the hybrid system (26) being ISS, ${{\,\mathrm{dom}\,}}\xi = {{\,\mathrm{dom}\,}}d$ and the pair $(\xi ,d)$ satisfies the estimate (17). Therefore, by observing that $|x(t;x_0,\omega ,\sigma )|=|y(t,j)|=|\xi (t,j)|_{\mathcal {A}}$ for all $t\in [t_j,t_{j+1})$, and

$$\begin{aligned} \Vert d\Vert _{(t,j)} = \max \left\{ {{\,\mathrm{ess\,sup}\,}}_{s\in [0,t]\backslash \mathcal {T}(\sigma )} |\omega (s)|, \sup _{s\in [0,t]\cap \mathcal {T}(\sigma )}|\omega (s)|\right\} , \end{aligned}$$

the estimate in (7) is now obtained by taking $\beta (s,t) = \beta _h(s, t, 0)$ and $\gamma _1=\gamma _2=\gamma _h$.

$\square$

Remark 5

Towards the end of the proof of Corollary 5, if we define a class $\mathcal {KLL}$ function ${\widetilde{\beta }}(s,t,j):=\beta _h(s,t,j)$ instead of taking the class $\mathcal {KL}$ function $\beta$, then we can actually conclude

$$\begin{aligned} |x(t;x_0,\omega ,\sigma )|\le & {} {\widetilde{\beta }}(|x_0|,t,N_{\sigma }(0,t))+\gamma _1({{\,\mathrm{ess\,sup}\,}}_{s\in [0,t]\backslash \mathcal {T}(\sigma )} |\omega (s)|)\nonumber \\&+\gamma _2\left( \sup _{s\in [0,t]\cap \mathcal {T}(\sigma )}|\omega (s)|\right) . \end{aligned}$$

(28)

Compared to (7), in (28) not only the magnitude of the solution decreases with respect to t, but it also decreases with respect to the total number of switches up to t. We can also conclude a similar stronger estimate on the solution for iISS. Such properties are termed as strong ISS/iISS, which appeared in [9, 12]. Nevertheless, we do not work with the strong ISS/iISS notions in this paper. This is because it is inferred from [9, Proposition 2.3] that strong ISS/iISS and ISS/iISS are equivalent for switched systems with switching signals satisfying the ADT condition, which is the class of switching signals addressed in our results.

4.3 Supporting lemmas

In this subsection, we provide a couple of supporting lemmas which will be used in our proof of Theorem 1. We start with an observation on $\psi$:

Lemma 6

The function $\psi$ constructed in (12) is positive definite. It is also of class $\mathcal {K}_\infty$ if $\alpha _{\text {s}}$ is of class $\mathcal {K}_\infty$.

Proof

By construction it is clear that $\psi$ is positive definite. To show that $\psi \in \mathcal {K}_{\infty }$ when $\alpha _{\text {s}}\in \mathcal {K}_{\infty }$, notice that $\psi$ is continuous, $\psi (0)=0$ so we are left to show that $\psi$ is strictly increasing and unbounded. To show $\psi$ is strictly increasing, we take arbitrary $t_2> t_1\ge 0$. Suppose that $\alpha _{\text {s}}(r)+c(t_2-r)$ achieves minimum at some $t^*\in [0,t_2]$, that is, $\psi (t_2)=\alpha _{\text {s}}(t^*)+c(t_2-t^*)$. We consider two cases by comparing $t^*$ and $t_1$. If $t^*\le t_1$, then

$$\begin{aligned} \psi (t_2)= & {} \alpha _{\text {s}}(t^*)+c(t_1-t^*)+c(t_2-t_1)>\alpha _{\text {s}}(t^*)+c(t_1-t^*)\\\ge & {} \min _{r\in [0,t_1]}\{\alpha _{\text {s}}(r)+c(t_1-r)\}=\psi (t_1). \end{aligned}$$

Otherwise, if $t^*>t_1$, then

$$\begin{aligned} \psi (t_2)= & {} \alpha _{\text {s}}(t_1)+(\alpha _{\text {s}}(t^*)-\alpha _{\text {s}}(t_1))+c(t_2-t^*)>\alpha _{\text {s}}(t_1) \\\ge & {} \min _{r\in [0,t_1]}\{\alpha _{\text {s}}(r)+c(t_1-r)\}=\psi (t_1). \end{aligned}$$

Therefore, we conclude that $\psi (t_2)>\psi (t_1)$. Next we show that $\lim _{t\rightarrow \infty }\psi (t)=\infty$. If this is not true, there exists $K>0$ such that $\psi (t)\le K$ for all $t\ge 0$. Because $\alpha _{\text {s}}\in \mathcal {K}_{\infty }$, there exists $r>0$ such that $\alpha _{\text {s}}(r)=2K$. Then, for any $r'\in [0,r+\frac{2K}{c}]$, either $r'\in [0,r]$ so that $c(r+\frac{2K}{c}-r')\ge 2K$, or $r'\in (r,r+\frac{2K}{c}]$ so that $\alpha _{\text {s}}(r') >2K$. Thus,

$$\begin{aligned} \psi \left( r+\frac{2K}{c}\right) =\min _{r'\in [0,r+\frac{2K}{c}]}\left\{ \alpha _{\text {s}}(r')+c\left( r+\frac{2K}{c}-r'\right) \right\} \ge 2K>K, \end{aligned}$$

which is a contradiction. This completes the proof. $\square$

The next lemma shows that $\psi$ is globally one-sided Lipschitz. One-sided Lipschitzness often appears in the study of differential inclusions (see, e.g., [6]).

Lemma 7

The function $\psi$ constructed in (12) is a globally one-sided Lipschitz function on $\mathbb {R}_{\ge 0}$ with constant c; that is, for any $t_2\ge t_1\ge 0$, $\psi (t_2)-\psi (t_1)\le c(t_2-t_1)$.

Proof

For any $t_2\ge t_1\ge 0$,

$$\begin{aligned} \psi (t_2)&=\min _{r\in [0,t_2]}\{\alpha _{\text {s}}(r)+c(t_2-r)\}\\&\le \min _{r\in [0,t_1]}\{\alpha _{\text {s}}(r)+c(t_2-r)\}\\&=\min _{r\in [0,t_1]}\{\alpha _{\text {s}}(r)+c(t_1-r)\}+c(t_2-t_1)\\&=\psi (t_1)+c(t_2-t_1) \end{aligned}$$

which is the desired inequality. $\square$

Next we define a function which will be used in the construction of the (i)ISS Lyapunov function for the hybrid system. Define the function $\varphi :\mathbb {R}_{\ge 0} \rightarrow \mathbb {R}_{\ge 0}$ by

$$\begin{aligned} \varphi (s):={\left\{ \begin{array}{ll} \exp \left( \int _1^s\frac{2 c}{\psi (r)}\hbox {d}r\right) , &{} \text { if } s>0, \\ 0, &{} \text { if } s=0. \end{array}\right. } \end{aligned}$$

(29)

where we recall c and $\psi$ are introduced in (12). We state two properties associated with $\varphi$.

Lemma 8

It holds that $\varphi \in \mathcal {K}_\infty$.

Proof

By definition, $\varphi (s)$ is strictly increasing and continuous for all $s>0$. It remains to show that $\varphi$ is continuous at 0 and $\lim _{s\rightarrow \infty }\varphi (s)=\infty$. It follows from (12) that $\psi (r)\le cr$ for all $r\ge 0$ so $\frac{2c}{\psi (r)}\ge \frac{2}{r}$. Therefore, when $s>1$, $\int _1^s\frac{2c}{\psi (r)}\hbox {d}r\ge \int _1^s\frac{2c}{cr}\hbox {d}r= 2\ln s$ so $\lim _{s\rightarrow \infty }\varphi (s)=\infty$. On the other hand, when $s<1$, $\int _1^s\frac{2c}{\psi (r)}\hbox {d}r\le \int _1^s\frac{2c}{cr}\hbox {d}r= 2\ln s$ so $\lim _{s\rightarrow 0^+}\varphi (s)=0$ and thus $\varphi$ is continuous at 0. $\square$

Lemma 9

Let $c_0>0$ and $s\ge t>0$ such that $\varphi (s)\le c_0\varphi (t)$. It follows that $\frac{\psi (s)}{\psi (t)}\le \sqrt{c_0}$.

Proof

Fix $s \ge t > 0$. Then, the inequality $\varphi (s)\le c_0\varphi (t)$ implies that

$$\begin{aligned} \exp \left( \int _1^s\frac{2c}{\psi (r)}\hbox {d}r\right) \le c_0\exp \left( \int _1^t\frac{2c}{\psi (r)}\hbox {d}r\right) . \end{aligned}$$

Take logarithm on both sides and subtract the integral on the right from the left, to get

$$\begin{aligned} \int _t^s\frac{2c}{\psi (r)}\hbox {d}r\le \ln (c_0). \end{aligned}$$

(30)

Define ${\widetilde{\psi }}_t:[t,\infty )\rightarrow \mathbb {R}_{+}$ by ${\widetilde{\psi }}_t(r):=\psi (t)+c(r-t)$. Recall from Lemma 7 that c is also the global one-sided Lipschitz constant of $\psi$, so we have ${\widetilde{\psi }}_t(r)\ge \psi (r)$ for all $r\ge t$. Hence, continuing from (30), we have

$$\begin{aligned} \ln (c_0)\ge & {} \int _t^s\frac{2c}{\psi (r)}\hbox {d}r \ge \int _t^s\frac{2c}{{\widetilde{\psi }}_t(r)}\hbox {d}r=\int _{t}^{s}\frac{2c}{\psi (t)+c(r-t)}\hbox {d}r\\= & {} 2\ln \big (\psi (t)+c(r-t)\big )\big |_{r=t}^{r=s}=2\ln \frac{\psi (t)+c(s-t)}{\psi (t)}\ge 2\ln \frac{\psi (s)}{\psi (t)}, \end{aligned}$$

which results in $\frac{\psi (s)}{\psi (t)}\le \sqrt{c_0}$. $\square$

The next lemma tells that a special transformation of function $\varphi$ inherits the global one-sided Lipschitzness from $\psi$.

Lemma 10

The function $\theta :\mathbb {R}_{\ge 0}\rightarrow \mathbb {R}_{\ge 0}$ defined by $\theta (s):=\varphi ^{-1}(c_0\varphi (s))$ with any $c_0\ge 1$ is globally one-sided Lipschitz with constant $\sqrt{c_0}$, where $\varphi$ is defined by (29).

Proof

Before we start the proof, we note that $\varphi ^{-1}$ in the definition of $\theta$ is well defined because $\varphi \in \mathcal {K}_{\infty }$, stated by Lemma 8. To show $\theta$ is globally one-sided Lipschitz with constant $\sqrt{c}_0$, it suffices to show that

$$\begin{aligned} \theta (t_2)-\theta (t_1)\le \sqrt{c}_0(t_2-t_1) \end{aligned}$$

for all $t_2\ge t_1\ge 0$; in other words, we need to show that $\theta (t)-t\sqrt{c}_0$ is a non-increasing function on $\mathbb {R}_{\ge 0}$. To this end, we first observe that it follows from the definition of $\varphi$ in (29) that the derivatives

$$\begin{aligned} \varphi '(s)=\frac{2c\varphi (s)}{\psi (s)} \end{aligned}$$

(31)

and

$$\begin{aligned} (\varphi ^{-1})'(s)=\frac{1}{\varphi '(\varphi ^{-1}(s))}=\frac{\psi (\varphi ^{-1}(s))}{2cs}. \end{aligned}$$

(32)

Hence, we conclude that for any $t\in \mathbb {R}_{\ge 0}$,

$$\begin{aligned} \frac{\hbox {d}}{\hbox {d}t}\theta (t)=\frac{\hbox {d}}{\hbox {d}t}\Big (\varphi ^{-1}\big (c_0\varphi (t)\big )\Big )=\frac{\psi \big (\varphi ^{-1}(c_0\varphi (t))\big )}{2cc_0\varphi (t)}\frac{2cc_0\varphi (t)}{\psi (t)}=\frac{\psi (\theta (t))}{\psi (t)}. \end{aligned}$$

Let $s:=\theta (t)$, so that $\varphi (s)=c_0\varphi (t)$. Since $c_0\ge 1$, $s\ge t\ge 0$, Lemma 9 yields $\frac{\psi (s)}{\psi (t)}\le \sqrt{c}_0$, and

$$\begin{aligned} \frac{\hbox {d}}{\hbox {d}t}\big (\theta (t)-t\sqrt{c}_0\big )=\frac{\psi (s)}{\psi (t)}- \sqrt{c}_0\le 0. \end{aligned}$$

Therefore, $\theta (t)-t\sqrt{c}_0$ is non-increasing and this completes the proof. $\square$

4.4 Proof of the main result

We now use the developments carried out in Sects. 4.1, 4.2, and 4.3 to prove Theorem 1.

Proof of Theorem 1

Since $\tau _a>\zeta ^*$ and (15) holds, we pick $\zeta \in (\zeta ^*,\tau _a)$ which is sufficiently close to $\zeta ^*$ so that

$$\begin{aligned} (\kappa +1)\eta +\frac{\zeta }{\tau _a}<1. \end{aligned}$$

(33)

We prove the theorem by showing that

$$\begin{aligned} V(\xi ):=\varphi ^{-1}\Big (e^{2c\big (\zeta \tau +(\kappa +1)(T_0-\tau _u)\big )}\varphi \big (V_p(x)\big )\Big ) \end{aligned}$$

(34)

is an (i)ISS Lyapunov function for the hybrid system (26) corresponding to the switched system (3) with ADT and AAT constrained switching signals.

Firstly, to verify the condition (19), we set

$$\begin{aligned} \alpha _1(s)={\underline{\alpha }}(s),\quad \text {and} \quad \alpha _2(s)=\varphi ^{-1}\Big (e^{2c\big (\zeta N_0+(\kappa +1)T_0\big )}\varphi \big ({\overline{\alpha }}(s)\big )\Big ) \end{aligned}$$

and the bounds in (19) clearly hold.

We next verify the conditions (20) and (21) . We recall from the proof of [2, Theorem 1] that the inequality (20) holds for some positive definite function $\alpha _c$ if there exists a positive definite function ${\tilde{\alpha }}_c$ such that

$$\begin{aligned} \left\langle \frac{\partial }{\partial \xi } V(\xi ),{\overline{f}} \right\rangle \le -{\tilde{\alpha }}_c(V(\xi ))+ \gamma _c(|d|). \end{aligned}$$

(35)

Moreover, $\alpha _c\in \mathcal {K}_{\infty }$ if ${\tilde{\alpha }}_c\in \mathcal {K}_{\infty }$, by setting $\alpha _c:={\tilde{\alpha }}_c\circ \alpha _1$. Similarly (21) holds for some positive definite function $\alpha _d$ if there exists a positive definite function ${\tilde{\alpha }}_d$ such that

$$\begin{aligned} V({\overline{g}})-V(\xi )\le -{\tilde{\alpha }}_d(V(\xi )) + \gamma _d(|d|) \end{aligned}$$

(36)

and $\alpha _d\in \mathcal {K}_{\infty }$ if ${\tilde{\alpha }}_d\in \mathcal {K}_{\infty }$. To verify the condition (35) (and therefore (20)) for the continuous flow, we define

$$\begin{aligned} W(\xi ):=e^{2c \big (\zeta \tau +(\kappa +1)(T_0-\tau _u)\big )}\varphi \big (V_p(x)\big ). \end{aligned}$$

(37)

Picking $(\xi ,d)\in {\mathcal {C}}$ and ${\overline{f}}\in {\mathcal {F}}(\xi ,d)$, we have

$$\begin{aligned} \left\langle \frac{\partial }{\partial \xi } W(\xi ), {\overline{f}} \right\rangle&= 2c\zeta W(\xi ){\dot{\tau }}-2c(\kappa +1)W(\xi ){\dot{\tau }}_u \\&\quad + \left\langle e^{2c (\zeta \tau +(\kappa +1)(T_0-\tau _u))}\varphi '(V_p(x))\frac{\partial }{\partial x} V_p(x),f_p(x,d) \right\rangle \\&=2c\left( \zeta {\dot{\tau }}-(\kappa +1){\dot{\tau }}_u+\frac{1}{\psi (V_p(x))}\left\langle \frac{\partial }{\partial x} V_p(x),f_p(x,d) \right\rangle \right) W(\xi ) \end{aligned}$$

where we have used (31) for $\varphi '$. When $p\in \mathcal {P}_{\text {s}}$, it follows from (10a) and (12) that $\left\langle \frac{\partial }{\partial x} V_p(x),f_p(x,d) \right\rangle \le -\psi (V_p(x))+\gamma (|d|)$. In addition ${\dot{\tau }}\in [0,\frac{1}{\tau _a}]$ and ${\dot{\tau }}_u\in [-\eta ,0]$, so

$$\begin{aligned} \left\langle \frac{\partial }{\partial \xi } W(\xi ), {\overline{f}} \right\rangle \le 2c\left( \frac{\zeta }{\tau _a}+(\kappa +1)\eta -1\right) W(\xi )+\frac{2cW(\xi )}{\psi (V_p(x))}\gamma (|d|). \end{aligned}$$

When $p\in \mathcal {P}_{\text {u}}$, it follows from (10b) and (13) that $\left\langle \frac{\partial }{\partial x} V_p(x),f_p \right\rangle \le \kappa \psi (V_p(x))+\gamma (|d|)$. In addition ${\dot{\tau }}\in [0,\frac{1}{\tau _a}]$ and ${\dot{\tau }}_u\in [1-\eta ,1]$, so

$$\begin{aligned} \left\langle \frac{\partial }{\partial \xi } W(\xi ), {\overline{f}} \right\rangle \le 2c\left( \frac{\zeta }{\tau _a}+(\kappa +1)(\eta -1)+\kappa \right) W(\xi )+\frac{2cW(\xi )}{\psi (V_p(x))}\gamma (|d|) \end{aligned}$$

and we reach the same upper bound as in the first case. Appealing to (33), we conclude that

$$\begin{aligned} \left\langle \frac{\partial }{\partial \xi } W(\xi ), {\overline{f}} \right\rangle \le -2caW(\xi )+\frac{2cW(\xi )}{\psi (V_p(x))}\gamma (|d|) \end{aligned}$$

where

$$\begin{aligned} a:=1-\frac{\zeta }{\tau _a}-(\kappa +1)\eta >0. \end{aligned}$$

(38)

Consequently, it can be computed that along the differential inclusion (26a),

$$\begin{aligned} \left\langle \frac{\partial }{\partial \xi } V(\xi ),{\overline{f}} \right\rangle&= (\varphi ^{-1})'(W(\xi )) \left\langle \frac{\partial }{\partial \xi } W(\xi ), {\overline{f}} \right\rangle \\&\le \left( \frac{\psi \big (\varphi ^{-1}(W(\xi ))\big )}{2cW(\xi )}\right) \left( -2caW(\xi )+\frac{2c W(\xi )}{\psi (V_p(x))}\gamma (|d|)\right) \\&=-a\psi \big (\varphi ^{-1}(W(\xi ))\big )+\frac{\psi (\varphi ^{-1}(W(\xi )))}{\psi (V_p(x))}\gamma (|d|)\\&=-a\psi (V(\xi ))+\frac{\psi (V(\xi ))}{\psi (V_p(x))}\gamma (|d|). \end{aligned}$$

To further simplify the right-hand side, let $v:=V_p(x)$, $w:=V(\xi )$. Then, we have $w\ge v\ge 0$ and $\varphi (w)=W(\xi )=e^{2c\big (\zeta \tau +(\kappa +1)(T_0-\tau _u)\big )}\varphi (V_p(x))\le e^{2c\big (\zeta N_0+(\kappa +1)T_0\big )}\varphi (v)$. Hence, from Lemma 9,

$$\begin{aligned} \left\langle \frac{\partial }{\partial \xi } V(\xi ),{\overline{f}}\right\rangle \le -a\psi (V(\xi ))+e^{c\big (\zeta N_0+(\kappa +1)T_0\big )}\gamma (|d|) \end{aligned}$$

and (35) holds with

$$\begin{aligned} {\tilde{\alpha }}_c(s)&:=a\psi (s) \end{aligned}$$

(39a)

$$\begin{aligned} \gamma _c(s)&:=e^{c\big (\zeta N_0+(\kappa +1)T_0\big )}\gamma (s). \end{aligned}$$

(39b)

It follows from Lemma 6 that if $\alpha _{\text {s}}$ is positive definite (resp. $\alpha _{\text {s}}\in {\mathcal {K}}_{\infty }$), then ${\tilde{\alpha }}_c$ is also positive definite (resp. $\alpha _c\in {\mathcal {K}}_{\infty }$) and so is $\alpha _c$. In addition, $\gamma _c\in {\mathcal {K}}$.

Lastly, to verify the condition (36) (and therefore (21)) for the discrete jumps, we pick $(\xi ,d)\in \mathcal {D}$ and ${\bar{g}}\in \mathcal {G}(\xi ,d)$. Notice that

$$\begin{aligned} \varphi \big (\chi (V_p(x))\big )= & {} \exp \Big (\int _1^{\chi (V_p(x))}\frac{2c}{\psi (r)}\hbox {d}r\Big )\\= & {} \exp \Big (\int _{V_p(x)}^{\chi (V_p(x))}\frac{2c}{\psi (r)}\hbox {d}r\Big )\exp \Big (\int _1^{V_p(x)}\frac{2c}{\psi (r)}\hbox {d}r\Big ) \le e^{2c\zeta ^*}\varphi (V_p(x)) \end{aligned}$$

where we recall that $\zeta ^*$ is defined in (14). Hence, if we define

$$\begin{aligned} \theta (s):=\varphi ^{-1}\left( e^{2c (\zeta (\tau -1)+(\kappa +1)(T_0-\tau _u))}\varphi (s)\right) , \end{aligned}$$

and recall the definition of W from (37), we have the estimate that $\theta \big (\chi (V_p(x))\big )\le \varphi ^{-1}\left( e^{2c (\zeta (\tau -1)+(\kappa +1)(T_0-\tau _u))+2c \zeta ^*}\varphi \big (V_p(x)\big ) \right) =\varphi ^{-1}\left( e^{2c(\zeta ^*-\zeta )}W(\xi )\right)$. Therefore, using the assumption (L3) and Lemma 10, we get

$$\begin{aligned} V({\overline{g}})&=\varphi ^{-1}\left( e^{2c (\zeta \tau ^++(\kappa +1)(T_0-\tau _u^+))}\varphi (V_{p^+}(x^+))\right) \\&=\varphi ^{-1}\left( e^{2c (\zeta (\tau -1)+(\kappa +1)(T_0-\tau _u))}\varphi \big (V_{q}(g_{p,q}(x,d))\big )\right) \\&\le \varphi ^{-1}\left( e^{2c (\zeta (\tau -1)+(\kappa +1)(T_0-\tau _u))}\varphi \big (\chi (V_p(x))+\rho (|d|)\big ) \right) \\&=\theta \big (\chi (V_p(x))+\rho (|d|)\big )\\&\le \theta \big (\chi (V_p(x)\big )+e^{c(\zeta (\tau -1)+(\kappa +1)(T_0-\tau _u))}\rho (|d|)\\&\le \varphi ^{-1}\left( e^{2c(\zeta ^*-\zeta )}W(\xi )\right) +e^{c(\zeta (N_0-1)+(\kappa +1)T_0)}\rho (|d|)\\&= \varphi ^{-1}\left( e^{2c(\zeta ^*-\zeta )}\varphi (V(\xi ))\right) +e^{c(\zeta (N_0-1)+(\kappa +1)T_0)}\rho (|d|). \end{aligned}$$

Thus, the inequality (36) is seen to hold with

$$\begin{aligned} {\tilde{\alpha }}_d(s)&:=s-\varphi ^{-1}\left( e^{2c(\zeta ^*-\zeta )}\varphi (s)\right) \end{aligned}$$

(40a)

$$\begin{aligned} \gamma _d(s)&:=e^{c(\zeta (N_0-1)+(\kappa +1)T_0)}\rho (s). \end{aligned}$$

(40b)

Recall that $\zeta >\zeta ^*$ and $\varphi \in \mathcal {K}_{\infty }$; thus by construction ${\tilde{\alpha }}_d$ is positive definite. In addition, $\gamma _d\in \mathcal {K}$. Lastly, we show that ${\tilde{\alpha }}_d\in \mathcal {K}_\infty$ when $\alpha _{\text {s}}\in \mathcal {K}_\infty$. It follows from (40a) that $\varphi \big ((s-{\tilde{\alpha }}_d(s)\big )=e^{2c(\zeta ^*-\zeta )}\varphi (s)$. Using the definition of $\varphi$ in (29), and taking logarithm, it follows that

$$\begin{aligned} \int _1^{s-{\tilde{\alpha }}_d(s)}\frac{2c}{\psi (r)}\hbox {d}r=2c(\zeta ^*-\zeta )+\int _1^{s}\frac{2c}{\psi (r)}\hbox {d}r. \end{aligned}$$

Hence, we have

$$\begin{aligned} \int _{s-{\tilde{\alpha }}_d(s)}^s\frac{1}{\psi (r)}\hbox {d}r=\zeta -\zeta ^* >0 . \end{aligned}$$

(41)

By Lemma 6, when $\alpha _{\text {s}}\in \mathcal {K}_\infty$, $\psi \in \mathcal {K}_\infty$ as well and thus $\frac{1}{\psi (r)}$ decreases to 0 as r increases to infinity. Therefore, (41) holds for all $s>0$ only when the length of the interval of integration grows to infinity as $s\rightarrow \infty$. In other words, we conclude that ${\tilde{\alpha }}_d\in \mathcal {K}_\infty$.

With all the three conditions (19), (20) and (21) verified, we appeal to Lemma 3 and conclude that the hybrid system (26) is ISS (resp. iISS) when $\alpha _s\in \mathcal {K}_\infty$ (resp. $\alpha _s$ is only positive definite). Then, by Corollary 5, the switched system (3) is uniformly ISS (resp. uniformly iISS) over the set of signals $\varSigma _{\text {ADT}}(\tau _a,N_0)\cap \varSigma _{\text {AAT}}(\mathcal {P}_{\text {u}},\eta ,T_0)$. $\square$

Remark 6

It is observed that the formulas for $\gamma _c,\gamma _d$ in (39b), (40b) only depend on the parameters $N_0,T_0$ but not $\tau _a,\eta$. Since it is known in [3, 22] that the (i)ISS gain is related to $\gamma _c,\gamma _d$, we conclude that the average dwell-time or percentage activation time of the unstable modes do not affect the (i)ISS gain. On the other hand, it is seen that the functions ${\tilde{\alpha }}_c,{\tilde{\alpha }}_d$ depend on $\tau _a,\eta$. Thus, we conclude that the convergence rate of the unforced switched system depends on the average dwell-time and percentage activation time of the unstable modes. In particular, when the left-hand side of (15) is close to 1, the convergence rate of the unforced switched system may become extremely slow and when it is larger than 1, the unforced switched system may become unstable and thus the switched system with inputs is not (i)ISS. This observation is consistent with the known results in [11].

5 Case studies

In this section, we will demonstrate how our results can be applied to determining stability of several switched nonlinear systems. The subsystems of these switched systems will be constructed using the matrices:

$$\begin{aligned} A_1=\begin{pmatrix}-0.1&{}-1\\ 2&{}-0.1\end{pmatrix},\ A_2=\begin{pmatrix}-0.1&{}-2\\ 1&{}-0.1\end{pmatrix},\ A_3=\begin{pmatrix}0.1&{}-1\\ 2&{}0.1\end{pmatrix}. \end{aligned}$$

(42)

Notice that $A_1$ and $A_2$ are both Hurwitz and they have the same eigenvalues; $A_3$ is not Hurwitz. In addition, it is discussed in [16, Sect. 3.2] that the switched linear system with modes $\mathcal {P}=\{1,2\}$

$$\begin{aligned} \dot{x}= & {} A_\sigma x \text{ if } t\not \in \mathcal {T}(\sigma ), \end{aligned}$$

(43a)

$$\begin{aligned} x= & {} x^-\ \text{ if } t\in \mathcal {T}(\sigma ). \end{aligned}$$

(43b)

has no common Lyapunov function and for some particular switching signals the solution will diverge.

5.1 Switched system with saturation-like scaling

Consider the switched system with modes $\mathcal {P}=\{1,2\}$

$$\begin{aligned} \dot{x}= & {} \frac{1}{1+|x|}A_\sigma x+\omega \quad \text{ if } t\not \in \mathcal {T}(\sigma ), \end{aligned}$$

(44a)

$$\begin{aligned} x= & {} x^-\qquad \qquad \qquad \text{ if } t\in \mathcal {T}(\sigma ) \end{aligned}$$

(44b)

where $\omega \in \mathbb {R}^2$ is the input. The coefficient $\frac{1}{1+|x|}$ mimics the effect of saturation such that $\dot{x}\approx A_{\sigma }x+\omega$ when |x| is small and $\dot{x}\approx A_{\sigma }\frac{x}{|x|}+\omega$ when |x| is large. The subsystems of (44) are both iISS, which can be verified by picking the iISS-Lyapunov functions

$$\begin{aligned} V_p(x)=\sqrt{1+x^\top M_p x}-1 \end{aligned}$$

(45)

where $M_p$ are the solutions to the Lyapunov equations

$$\begin{aligned} M_pA_p+A_p^\top M_p+I=0. \end{aligned}$$

(46)

However, this switched system is not iISS under slow switching. To see this, notice that when $u\equiv 0$, the solutions of the switched system (44) are the same as the solutions of (43) after a time re-parameterization. By similar arguments as discussed in [25], for any $\tau _a>0$ and $N_0\ge 1$, we can pick an initial state far away enough from the origin and a switching signal from $\varSigma _{\text {ADT}}(\tau _a,N_0)$, yet the solution diverges. Hence, the switched system (44) is not uniformly iISS over $\varSigma _{\text {ADT}}(\tau _a,N_0)$ for any $\tau _a>0$. Nevertheless, we show that the switched system (44) is iISS over AAT switching signals. To do this, let $V_1$ be defined as in (45) with $p=1$ and set $V_2=V_1$. We first see that (9) is verified with ${\underline{\alpha }}(s):=\sqrt{1+{\underline{\sigma }}(M_1)s^2}-1$ and ${\bar{\alpha }}(s):=\sqrt{1+{\bar{\sigma }}(M_1)s^2}-1$, where ${\bar{\sigma }}(\cdot ),{\underline{\sigma }}(\cdot )$ denote the largest and smallest singular values, respectively. We further denote $Q_1:=-I, Q_2:=M_1A_2+A_2^\top M_1$. Notice that because $V_2$ is not a Lyapunov function for the subsystem $p=2$, $Q_2$ defined in this way is sign indefinite. It can be computed that

$$\begin{aligned} \Big \langle \frac{\partial }{\partial x} V_p(x),f_p(x,\omega ) \Big \rangle&=\frac{1}{\sqrt{1+x^\top M_1 x}}\left( \dot{x}^\top M_1 x+x^\top M_1 \dot{x}\right) \\&=\frac{x^\top \left( A_p^\top M_1+M_1A_p\right) x}{(1+|x|)\sqrt{1+x^\top M_1 x}} +\frac{2x^\top M_p}{\sqrt{1+x^\top M_p x}}\omega \\&=\frac{x^\top Q_p x}{(1+|x|)\sqrt{1+x^\top M_1 x}} +\frac{2x^\top M_1}{\sqrt{1+x^\top M_1 x}}\omega \\&=\frac{x^\top Q_p x}{(1+|x|)(V_p(x)+1)} +\frac{2x^\top M_1}{\sqrt{1+x^\top M_1 x}}\omega . \end{aligned}$$

It is observed that the function $h(s)=\frac{s^2}{1+s}$ is increasing on $\mathbb {R}_{\ge 0}$; in addition, from (45) we have $\sqrt{\frac{V_p^2+2V_p}{{\bar{\sigma }}(M_1)}}\le |x|\le \sqrt{\frac{V_p^2+2V_p}{{\underline{\sigma }}(M_1)}}$. Hence, we conclude that

$$\begin{aligned} \Big \langle \frac{\partial }{\partial x} V_1(x),f_1(x,\omega ) \Big \rangle \le -\frac{V_1^2+2V_1}{\left( {\bar{\sigma }}(M_1)+\sqrt{{\bar{\sigma }}(M_1)(V_1^2+2V_1)}\right) (V_1+1)}+\frac{2{\bar{\sigma }}(M_1)}{\sqrt{{\underline{\sigma }}(M_1)}}|\omega |, \end{aligned}$$

which fits the form (10a) with $\alpha _{\text {s}}(s):=\frac{s^2+2s}{\big ({\bar{\sigma }}(M_1)+\sqrt{{\bar{\sigma }}(M_1)(s^2+2s)}\big )(s+1)}$ and $\gamma (s):=\frac{2{\bar{\sigma }}(M_1)}{\sqrt{{\underline{\sigma }}(M_1)}}s$. It can be verified that $\alpha _{\text {s}}$ is globally Lipschitz so as discussed in Sect. 3, we can set $\psi =\alpha _{\text {s}}$. For $p=2$,

$$\begin{aligned} \Big \langle \frac{\partial }{\partial x} V_2(x),f_2(x,\omega ) \Big \rangle \le \frac{{\bar{\sigma }}(Q_2)(V_2^2+2V_2)}{\left( {\underline{\sigma }}(M_1)+\sqrt{{\underline{\sigma }}(M_1)(V_2^2+2V_2)}\right) (V_2+1)}+\frac{2{\bar{\sigma }}(M_1)}{\sqrt{{\underline{\sigma }}(M_1)}}|\omega |, \end{aligned}$$

which fits the form (10b) with $\alpha _{\text {u}}(s):=\frac{{\bar{\sigma }}(Q_2)(s^2+2s)}{\big ({\underline{\sigma }}(M_1)+\sqrt{{\underline{\sigma }}(M_1)(s^2+2s)}\big )(s+1)}$. The above computations imply that Assumption 1 holds. Plugging the numerical values (42) for $A_1,A_2$ into the formulas for $\psi ,\alpha _{\text {u}}$, it is computed that $\kappa =\sup _{s\ge 0}\frac{\alpha _{\text {u}}(s)}{\psi (s)}={\bar{\sigma }}(Q_2)\frac{{\bar{\sigma }}(M_1)}{{\underline{\sigma }}(M_1)}=24.9436$ and $\frac{1}{1+\kappa }=0.0385$. Hence, Assumption 2 also holds. In addition, because we have chosen $V_1=V_2$ and the switched system has no resets at switches, $\zeta ^*=0$ and Assumption 3 holds as well. Therefore, by Theorem 1 and the discussion after the theorem statement, we conclude that the switched system (44) is uniformly ISS over $\varSigma _{\text {ADT}}(\tau _a,N_0)\cap \varSigma _{\text {AAT}}(\{2\},\eta ,T_0)$ for any $\tau _a>0,N_0\ge 1,\eta <0.0385,T_0\ge 0$.

5.2 Switched bilinear system with resets and stable modes

Consider the switched system with modes $\mathcal {P}=\{1,2\}$

$$\begin{aligned} \dot{x}= & {} A_\sigma x+\omega Bx\qquad \text{ if } t\not \in \mathcal {T}(\sigma ), \end{aligned}$$

(47a)

$$\begin{aligned} x= & {} Dx^-+\omega Ex^-\quad \text{ if } t\in \mathcal {T}(\sigma ), \end{aligned}$$

(47b)

where $\omega \in \mathbb {R}$ is the input, $A_p$ are given in (42) and $B,D,E\in \mathbb {R}^{2\times 2}$. Each subsystem is shown to be iISS (see, e.g., [5]) by picking the iISS-Lyapunov function $V_p$ as

$$\begin{aligned} V_p(x):=\ln (1+x^\top M_px) \end{aligned}$$

(48)

where again $M_p$ are the solutions of (46). We have

$$\begin{aligned} {\underline{\alpha }}(|x|):= & {} \ln \left( 1+\min _{p\in \mathcal {P}}{\underline{\sigma }}(M_p)|x|^2\right) \le V_p(x)\nonumber \\\le & {} \ln \left( 1+\max _{p\in \mathcal {P}}{\bar{\sigma }}(M_p)|x|^2\right) =:{\bar{\alpha }}(|x|). \end{aligned}$$

(49)

For the continuous flow, it can be computed that

$$\begin{aligned} \Big \langle \frac{\partial }{\partial x} V_p(x),f_p(x,\omega ) \Big \rangle&=\frac{1}{1+x^\top M_px}\left( \dot{x}^\top M_p x+x^\top M_p \dot{x}\right) \\&=\frac{1}{1+x^\top M_px}x^\top (A_p^\top M_p+M_pA_p)x+\frac{2x^\top M_pBx}{1+x^\top M_px}\omega \\&=-\frac{|x|^2}{1+x^\top M_px}+\frac{2x^\top M_pBx}{1+x^\top M_px}\omega \\&\le -\frac{(e^{V_p}-1)}{{\bar{\sigma }}(M_p)e^{V_p}}+\frac{{\bar{\sigma }}(M_pB)}{{\underline{\sigma }}(M_p)}|\omega | \end{aligned}$$

Hence, (10a) is satisfied for all $p\in \mathcal {P}_{\text {s}}=\mathcal {P}$ with $\alpha _{\text {s}}(s):=\frac{1-e^{-s}}{\sigma _{\max }}$, $\gamma (s):=c_1s$ where $\sigma _{\max }:=\max _{p\in \mathcal {P}}{\bar{\sigma }}(M_p),c_1:=\max _{p\in \mathcal {P}}\frac{{\bar{\sigma }}(M_pB)}{{\underline{\sigma }}(M_p)}$. Now consider the discrete jumps and denote $g(x,\omega ):=Dx+\omega Ex$. Let $\mu >0$ be such that $x^\top D^\top M_p Dx\le \mu x^\top M_q x$ for all $x\in \mathbb {R}^n$ and $p,q\in \mathcal {P}$, then we have the inequality that

$$\begin{aligned} 1+g(x,\omega )^\top&M g(x,\omega )=1+(Dx+uEx)^\top M_p(Dx+uEx)\\&=1+x^\top D^\top M_p Dx+x^\top (2uE^\top M_pD+\omega ^2E^\top M_p E)x\\&\le 1+\mu x^\top M_q x+x^\top (2uE^\top M_pD+\omega ^2E^\top M_p E)x\\&=(1+\mu x^\top M_q x)\left( 1+\frac{x^\top (2uE^\top M_pD+\omega ^2E^\top M_p E)x}{1+\mu x^\top M_q x}\right) \\&\le (1+\mu x^\top M_q x)\left( 1+\frac{2{\bar{\sigma }}(E^\top M_pD)|\omega |+{\bar{\sigma }}(E^\top M_p E)|\omega |^2}{\mu {\underline{\sigma }}(M_q)}\right) \end{aligned}$$

Hence, when a switch occurs,

$$\begin{aligned} V_p(g(x,\omega ))&=\ln (1+g(x,\omega )^\top M g(x,\omega ))\\&\le \ln \left( (1+\mu x^\top M_q x)\left( 1+\frac{2{\bar{\sigma }}(E^\top M_pD)|\omega |+{\bar{\sigma }}(E^\top M_p E)|\omega |^2}{\mu {\underline{\sigma }}(M_q)}\right) \right) \\&=\ln (1+\mu x^\top M_q x)+\ln \left( 1+\frac{2{\bar{\sigma }}(E^\top M_pD)|\omega |+{\bar{\sigma }}(E^\top M_p E)|\omega |^2}{\mu {\underline{\sigma }}(M_q)}\right) \\&=\ln \left( 1+\mu \big (e^{V_q(x)}-1\big )\right) +\ln \left( 1+\frac{2{\bar{\sigma }}(E^\top M_pD)|\omega |+{\bar{\sigma }}(E^\top M_p E)|\omega |^2}{\mu {\underline{\sigma }}(M_q)}\right) \end{aligned}$$

Hence, (11) is satisfied with $\chi (s):=\ln \left( 1+\mu \big (e^{s}-1\big )\right)$ and $\rho (s):=\ln (1+c_2s+c_3s^2)$, where $c_2:=\frac{2\max _{p\in \mathcal {P}}{\bar{\sigma }}(E^\top M_pD)}{\mu \min _{q\in \mathcal {P}}{\underline{\sigma }}(M_q )},c_3:=\frac{2\max _{p\in \mathcal {P}}{\bar{\sigma }}(E^\top M_p E)}{\mu \min _{q\in \mathcal {P}}{\underline{\sigma }}(M_p)}$. Therefore, Assumption 1 is verified and since both modes are stable, Assumption 2 is omitted. Again because $\alpha _{\text {s}}$ is globally Lipschitz, we let $\psi =\alpha _{\text {s}}$ and it can be computed that

$$\begin{aligned} \zeta ^*&=\sup _{s>0}\int _s^{\chi (s)}\frac{1}{\psi (r)}\hbox {d}r =\sup _{s>0}\int _s^{\chi (s)}\frac{\sigma _{\max }e^r}{(e^r-1)}\hbox {d}r\\&=\sigma _{\max }\sup _{s>0}\ln (e^r-1)\Big |_{s}^{\ln \left( \mu (e^s-1)+1\right) }\\&=\sigma _{\max }\sup _{s>0}\ln \frac{\left( \mu (e^s-1)+1\right) -1}{e^s-1}\\&=\sigma _{\max }\ln \mu \end{aligned}$$

and Assumption 3 is verified. Hence, we conclude from the special case of Theorem 1 that the switched system (47) with modes $\mathcal {P}=\{1,2\}$ is uniformly iISS over $\varSigma _{\text {ADT}}(\tau _a,N_0)$ for all $\tau _a>\sigma _{\max }\ln \mu ,N_0\ge 1$. In particular, if $D=I$, it can be numerically computed that $\sigma _{\max }\ln \mu =5.1804$.

The same arguments also work for switched bilinear systems with resets and stable modes in general, in the form

$$\begin{aligned} \dot{x}= & {} A_\sigma x+\sum _{j=1}^{m_c}B_{\sigma ,j}x\omega _j+C_\sigma \omega ,\qquad \qquad \qquad \qquad \text{ if } t\in \mathcal {T}(\sigma ), \end{aligned}$$

(50a)

$$\begin{aligned} x= & {} D_{(\sigma ^-,\sigma )}x^-+\sum _{k=1}^{m_d}E_{(\sigma ^-,\sigma ),k}x^-\omega _k+F_{(\sigma ^-,\sigma )}\omega \quad \text{ if } t\in \mathcal {T}(\sigma ), \end{aligned}$$

(50b)

where $x\in \mathbb {R}^n$ is the state, $\omega \in \mathbb {R}^m$ is the input and $A_p,B_{p,j},D_{(p,q)},E_{(p,q),k}\in \mathbb {R}^{n\times n}$, $C_p,F_{(p,q)}\in \mathbb {R}^{n\times m}$ for all $p,q\in \mathcal {P},j=1,\cdots m_c, k=1,\cdots m_d$.

Proposition 11

Consider the switched system (50) with finitely many modes and assume that $A_p$ are Hurwitz for all $p\in \mathcal {P}$ so that there exist positive definite symmetric matrices $M_p,Q_p\in \mathbb {R}^{n\times n}$ for which $A_p^\top M_p+M_pA_p+Q_p=0$ hold. Let $\lambda >0$ be such that $x^\top Q_p x\ge \lambda x^\top M_p x$ and $\mu >0$ be such that $x^\top D_{(p,q)}^\top M_q D_{(p,q)}x\le \mu x^\top M_p x$ for all $x\in \mathbb {R}^n$ and $p,q\in \mathcal {P}$. Then, (50) is uniformly iISS over $\varSigma _{\text {ADT}}(\tau _a,N_0)$ for all $\tau _a>\frac{\ln \mu }{\lambda }$ and $N_0\ge 1$.

The proof is similar to the one carried out for system (47) and is provided in Appendix.

Remark 7

Notice that $\lambda ,\mu$ defined in Proposition 11 are exactly the linear decay rate in the continuous flow and linear growth rate in the discrete jumps, respectively, for the unforced switched system (50). It is known that in this case, $\frac{\ln \mu }{\lambda }$ is the lower bound on ADT such that the unforced switched linear system is globally asymptotically stable (see [13]). It follows that the bilinear input does not affect the inheritance of the stability properties from the subsystems to the switched system when it is under slow switching. In addition, when there exists a common Lyapunov function, all $M_p$’s are the same and further when $D=I$, we conclude $\zeta ^*=0$ so $\tau _a$ can be as small as possible. This is indeed the case because such a switched system is iISS with arbitrary switching [19].

5.3 Switched bilinear system with unstable modes

For the last example we still consider the switched system with dynamics given by (47) but now the set of modes is $\mathcal {P}=\{1,3\}$. Recall that $A_3$ given in (42) is non-Hurwitz so this switched system is not uniformly iISS over $\varSigma _{\text {ADT}}(\tau _a,N_0)$ for any $\tau _a>0$. We also let $D=2I$, that is, whenever a switch occurs the state is scaled by 2 and hence this switched system is also not uniformly iISS over $\varSigma _{\text {AAT}}(\mathcal {P}_{\text {u}},\eta ,T_0)$ for any $\eta \in [0,1]$. However, if we pick $V_3=V_1=\ln (1+x^\top M_1 x)$, it can be computed that

$$\begin{aligned} \Big \langle \frac{\partial }{\partial x} V_3(x),f_3(x,\omega ) \Big \rangle&=\frac{1}{1+x^\top M_1x}x^\top (A_3^\top M_1+M_1A_3)x+\frac{2x^\top M_1Bx}{1+x^\top M_1x}\omega \\&\le \frac{{\bar{\sigma }}(A_3^\top M_1+M_1A_3)|x|^2}{1+x^\top M_1x}+\frac{2x^\top M_1Bx}{1+x^\top M_1x}\omega \\&\le \frac{{\bar{\sigma }}(A_3^\top M_1+M_1A_3)}{{\underline{\sigma }}(M_1)}\big (1-e^{V_p(x)}\big )+\frac{{\bar{\sigma }}(M_1B)}{{\underline{\sigma }}(M_1)}\omega \end{aligned}$$

So we have $\mathcal {P}_{\text {u}}=\{3\}$ with $\alpha _{\text {u}}(s):=\frac{{\bar{\sigma }}(A_3^\top M_1+M_1A_3)}{{\underline{\sigma }}(M_1)}\big (1-e^s\big )$. Thus, Assumption 2 holds with $\kappa =\frac{\alpha _{\text {u}}}{\psi }={\bar{\sigma }}(A_3^\top M_1+M_1A_3)\frac{{\bar{\sigma }}(M_1)}{{\underline{\sigma }}(M_1)}=3.9868$. On the other hand, $V_p$ being the same and $D=2I$ imply $\mu =4$. Thus, Assumption 3 holds with $\zeta ^*=\sigma _{\max }\ln \mu =10.3857$. Therefore, by Theorem 1 we conclude that (47) is uniformly iISS over $\varSigma _{\text {ADT}}(\tau _a,N_0)\cap \varSigma _{\text {AAT}}(\{3\},\eta ,T_0)$ for any $N_0\ge 1,T_0\ge 0$ and all $\tau _a>0,\eta \in [0,1]$ satisfying $3.9868\eta +\frac{10.3857}{\tau _a}<1$.

6 Conclusion

Within the context of literature on stability of switched systems, this paper provided conditions on the switching signals such that a switched nonlinear system with inputs, resets and unstable modes is ISS or iISS. In particular, we considered the case where the decay or growth rates of all subsystems are allowed to be nonlinear, and the changes of Lyapunov function values at switching instants are also assumed to be nonlinear. Under some mild assumptions on the switched system, and using the hybrid system framework, we derived a mixed ADT and AAT condition which guarantees ISS or iISS of the switched system. Using the main result of our work, switched systems with saturating dynamics and switched bilinear systems were shown to be uniformly iISS over switching signals satisfying this mixed ADT and AAT condition.

From a broader viewpoint, in this paper, we restricted ourselves to a certain class of switching signals, and we proposed techniques which relate the parameterization of these switching signals with the nonlinear rates associated with the Lyapunov functions of individual subsystems. There are other classes of switching signals which can be studied. For example, in [15], the notion of ADT is generalized by replacing the affine function of time in the definition of ADT by a more generic function. Similar generalization is also seen in the recent work [9]. Meanwhile, in [20], the authors propose impulse sequences whose impulse frequencies are eventually uniformly bounded, but iISS-related questions have not been investigated for such a class of signals. Thus, a natural question that originates from these works is to find conditions for ISS/iISS of switched systems in the nonlinear setting with different classes of switching signals, and whether the tools proposed in this paper could be used in making such generalizations.

Appendix

Proof of Proposition 11

First of all, each subsystem is shown to be iISS (see, e.g., [5]) by picking the iISS-Lyapunov function $V_p$ as

$$\begin{aligned} V_p(x):=\ln (1+x^\top M_px). \end{aligned}$$

(51)

We have

$$\begin{aligned} {\underline{\alpha }}(|x|):=\ln \left( 1+\min _{p\in \mathcal {P}}{\underline{\sigma }}(M_p)|x|^2\right) \le V_p(x)\le \ln \left( 1+\max _{p\in \mathcal {P}}{\bar{\sigma }}(M_p)|x|^2\right) =:{\bar{\alpha }}(|x|).\nonumber \\ \end{aligned}$$

(52)

So the condition (L1) is verified. For the continuous flow, it can be computed that

$$\begin{aligned}&\Big \langle \frac{\partial }{\partial x} V_p(x),f_p(x,\omega ) \Big \rangle =\frac{1}{1+x^\top M_px}\left( \dot{x}^\top M_p x+x^\top M_p \dot{x}\right) \\&\quad =\frac{1}{1+x^\top M_px}x^\top (A_p^\top M_p+M_pA_p)x+\sum _{j=1}^{m_c}\frac{2x^\top M_pB_{p,j}x}{1+x^\top M_px}\omega _j+\frac{2x^\top M_pC_p}{1+x^\top M_p x}\omega \\&\quad \le -\frac{x^\top Q_p x}{1+x^\top M_px}+\sqrt{\sum _{j=1}^{m_c}\left( \frac{2x^\top M_pB_{p,j}x}{1+x^\top M_px}\right) ^2}|\omega |+\frac{2|x^\top M_pC_p|}{1+x^\top M_p x}|\omega |\\&\quad \le -\frac{\lambda x^\top M_p x}{1+x^\top M_p x}+2\sqrt{n}\frac{\max _{j}{\bar{\sigma }}(M_pB_{p,j})}{{\underline{\sigma }}(M_p)}|\omega |+\frac{{\bar{\sigma }}(M_pC_p)}{\sqrt{{\underline{\sigma }}(M_p)}}|\omega |\\&\quad = -\lambda (1-e^{-V_p(x)})+\left( 2\sqrt{n}\frac{\max _{j}{\bar{\sigma }}(M_pB_{p,j})}{{\underline{\sigma }}(M_p)}+\frac{{\bar{\sigma }}(M_pC_p)}{\sqrt{{\underline{\sigma }}(M_p)}}\right) |\omega |. \end{aligned}$$

Hence, (10a) is satisfied for all $p\in \mathcal {P}_{\text {s}}=\mathcal {P}$ with

$$\begin{aligned} \alpha _{\text {s}}(r):=\lambda (1-e^{-r}),\gamma (r):=\left( \max _p\big (2\sqrt{n}\frac{\max _{j}{\bar{\sigma }}(M_pB_{p,j})}{{\underline{\sigma }}(M_p)}+\frac{{\bar{\sigma }}(M_pC_p)}{\sqrt{{\underline{\sigma }}(M_p)}}\big )\right) r. \end{aligned}$$

Now consider the discrete jumps. We start by defining the terms:

$$\begin{aligned} K_{DD}&:=\left( D_{(p,q)}x^-\right) ^\top M_q D_{(p,q)}x^-,\\ K_{EE}&:=\left( \sum _{k=1}^{m_d}E_{(p,q),k}x^-\omega _k\right) ^\top M_q\big (\sum _{k=1}^{m_d}E_{(p,q),k}x^-\omega _k\big ),\\ K_{FF}&:=\left( F_{(p,q)}\omega \right) ^\top M_q(F_{(p,q)}\omega ),\\ K_{DE}&:=(D_{(p,q)}x^-)^\top M_q\left( \sum _{k=1}^{m_d}E_{(p,q),k}x^-\omega _k\right) ,\\ K_{DF}&:=(D_{(p,q)}x^-)^\top M_q(F_{(p,q)}\omega ),\\ K_{EF}&:=\left( \sum _{k=1}^{m_d}E_{(p,q),k}x^-\omega _k\right) ^\top M_q(F_{(p,q)}\omega ). \end{aligned}$$

These are the terms when expanding the product

$$\begin{aligned}&\left( D_{(p,q)}x^-+\sum _{k=1}^{m_d}E_{(p,q),k}x^-\omega _k+F_{(p,q)}\omega \right) ^\top M_q\\&\quad \left( D_{(p,q)}x^-+\sum _{k=1}^{m_d}E_{(p,q),k}x^-\omega _k+F_{(p,q)}\omega \right) . \end{aligned}$$

From the hypothesis of Proposition (11), we have $K_{DD}\le \mu (x^-)^\top M_p x^-$. Meanwhile, use the fact that $y^\top M y\le {\bar{\sigma }}(M)|y|^2$ for any matrix $M\in \mathbb {R}^{n\times n}$ and any vectors $y\in \mathbb {R}^y$, in addition to the inequality that

$$\begin{aligned} \left| \sum _{k=1}^{m_d}E_{(p,q),k}x\omega _k\right| \le \left| \sum _{k=1}^{m_d}\omega _kE_{(p,q),k}\right| |x|\le \sqrt{\sum _{k=1}^{m_d}{\bar{\sigma }}(E_{(p,q),k})^2}|\omega ||x|, \end{aligned}$$

we conclude that $K_{EE}\le k_{EE}|x^-|^2|\omega |^2$, $K_{FF}\le k_{FF}|\omega |^2$, $K_{DE}\le k_{DE}|x^-|^2|\omega |$, $K_{DF}\le k_{DF}|x^-||\omega |$ and $K_{EF}\le k_{EF}|x^-||\omega |^2$, where

$$\begin{aligned} k_{EE}&:={\bar{\sigma }}(M_q)\sum _{k=1}^{m_d}{\bar{\sigma }}(E_{(p,q),k})^2,\quad k_{FF}:={\bar{\sigma }}(F_{(p,q)}^\top M_q F_{(p,q)}),\\ k_{DE}&:={\bar{\sigma }}(M_qD_{(p,q)})\left( \sum _{k=1}^{m_d}{\bar{\sigma }}(E_{(p,q),k})^2\right) ^{\frac{1}{2}},\quad k_{DF}:={\bar{\sigma }}(D_{(p,q)}^\top M_qF_{(p,q)}),\\ k_{EF}&:={\bar{\sigma }}(M_qF_{(p,q)})\left( \sum _{k=1}^{m_d}{\bar{\sigma }}(E_{(p,q),k})^2\right) ^{\frac{1}{2}}. \end{aligned}$$

Therefore, when a switch occurs,

$$\begin{aligned} V_q(x)&=\ln \big (1+K_{DD}+K_{EE}+K_{FF}+2K_{DE}+2K_{DF}+2K_{EF}\big )\\&\le \ln \big (1+\mu (x^-)^\top M_p x^-+(2k_{DE}|\omega |+k_{EE}|\omega |^2)|x^-|^2\\&\quad +(2k_{DF}|\omega |+2k_{EF}|\omega |^2)|x^-|+k_{FF}|\omega |^2\big )\\&=\ln \Big ((1+\mu (x^-)^\top M_p x^-)\\&\quad \times \big (1+\frac{(2k_{DE}|\omega |+k_{EE}|\omega |^2)|x^-|^2+(2k_{DF}|\omega |+2k_{EF}|\omega |^2)|x^-|+k_{FF}|\omega |^2}{1+\mu (x^-)^\top M_p x^-}\big )\Big )\\&=\ln (1+\mu (x^-)^\top M_p x^-)\\&\quad +\ln \Big (1+\frac{(2k_{DE}|\omega |+k_{EE}|\omega |^2)|x^-|^2+(2k_{DF}|\omega |+2k_{EF}|\omega |^2)|x^-|+k_{FF}|\omega |^2}{1+\mu (x^-)^\top M_p x^-}\Big )\\&\le \ln (1+\mu (x^-)^\top M_p x^-)\\&\quad +\ln \Big (1+\frac{(2k_{DE}|\omega |+k_{EE}|\omega |^2)|x^-|^2}{\mu (x^-)^\top M_p x^-}+\frac{(2k_{DF}|\omega |+2k_{EF}|\omega |^2)|x^-|}{1+\mu (x^-)^\top M_p x^-}+k_{FF}|\omega |^2\Big )\\&\le \ln (1+\mu (e^{V_p(x^-)}-1))\\&\quad +\ln \Big (1+\frac{2k_{DE}|\omega |+k_{EE}|\omega |^2}{\mu {\underline{\sigma }}(M_p)}+\frac{k_{DF}|\omega |+k_{EF}|\omega |^2}{\sqrt{\mu {\underline{\sigma }}(M_p)}}+k_{FF}|\omega |^2\Big ) \end{aligned}$$

Hence, (11) is satisfied with $\chi (s):=\ln \left( 1+\mu \big (e^{s}-1\big )\right)$ and $\rho (s):=\ln \Big (1+\big (\frac{2k_{DE}}{\mu {\underline{\sigma }}(M_p)}+\frac{k_{DF}}{\sqrt{\mu {\underline{\sigma }}(M_p)}}\big )s+\big (\frac{k_{EE}}{\mu {\underline{\sigma }}(M_p)}+\frac{k_{EF}}{\sqrt{\mu {\underline{\sigma }}(M_p)}}+k_{FF}\big )s^2\Big )$. Therefore, Assumption 1 is verified. Because $\alpha _{\text {s}}$ is globally Lipschitz, we let $\psi =\alpha _{\text {s}}$ and it can be computed that

$$\begin{aligned} \zeta ^*&=\sup _{s>0}\int _s^{\chi (s)}\frac{1}{\psi (r)}\hbox {d}r =\sup _{s>0}\int _s^{\chi (s)}\frac{e^r}{\lambda (e^r-1)}\hbox {d}r\\&=\frac{1}{\lambda }\sup _{s>0}\ln (e^r-1)\Big |_{s}^{\ln \left( \mu (e^s-1)+1\right) }\\&=\frac{1}{\lambda }\sup _{s>0}\ln \frac{\left( \mu (e^s-1)+1\right) -1}{e^s-1}\\&=\frac{\ln \mu }{\lambda }. \end{aligned}$$

Thus, Assumption 3 is verified and Proposition 11 is proven by Theorem 1. $\square$