Skip to main content
SpringerLink
Log in

Bifurcation analysis of a rigid-rotor squeeze film damper system with unsymmetrical stiffness supports

  • Original
  • Published:
Archive of Applied Mechanics Aims and scope Submit manuscript

Abstract

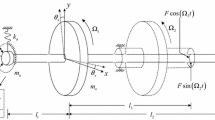
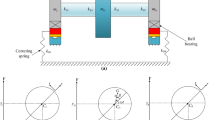
This paper is focused on the relationship between rigid body translation and rigid body precession in a squeeze film damper–rigid-rotor system with unsymmetrical stiffness supports. Two cases are considered: the precession motion non-resonance and internal resonance when translation motion occur primary resonant. In the first case, the amplitudes of translation and precession can be connected with an integration parameter about rotor parameters such as geometric size, stiffness, and mass. Some combination of system parameters will make the amplitudes of precession motion reach the same magnitude of the amplitudes of translation motion, so this integration parameter become an index to reflect the precessional motion degree, it is can be used to judge the feasibility of neglecting processional motion and simplifying asymmetry rotor as reasonable symmetry model. In the second case, the translation motion and precessional motion are strongly coupled, the vibration energy transfer between two kind of motion and the system occur internal resonant, which is possible appear in the rotor with cantilever disk. Each of case may appear nonlinear phenomenon, which is closely related with system parameters. The bifurcation analysis by using singularity methods is carried out to delimit the range of applicative operation parameters to avoid harmful phenomenon in unsymmetrical rotor system. The results of this paper provide a theoretical foundation for the convenient model simplification judgment and parameters optimization of the squeeze film damper-unsymmetrical rotor systems.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10

Similar content being viewed by others

Abbreviations

\(a_{1,2}\) :

Non-dimensional amplitudes in complex coordinates

\(\bar{{a}}_{1,2} \) :

Non-dimensional amplitudes in principal coordinates

B :

Non-dimensional bearing parameter

\({B}'\) :

Non-dimensional bearing parameter

c :

Clearance of squeeze film damper (m)

\({c}'\) :

Damping of the supports (N s/m)

e :

Radial displacement of journal (m)

\(F_{cx}\) :

Oil film force in x-direction (N)

\(F_{cy}\) :

Oil film force in y-direction (N)

\(F_{r}\) :

Oil film force in radial direction (N)

\(F_{t}\) :

Oil film force in tangential direction (N)

\(\bar{{F}}_r \) :

Non-dimensional oil film force in radial direction

\(\bar{{F}}_t \) :

Non-dimensional oil film force in tangential direction

\(J_{d}\) :

Equivalent equatorial moment of inertia (\(\hbox {kg m}^{2}\))

\(J_{p}\) :

Equivalent polar moment of inertia (\(\hbox {kg m}^{2}\))

K :

Ratio of stiffness coefficient

\(k_{1}\) :

Equivalent stiffness coefficient of the left support (N/m)

\(k_{2}\) :

Equivalent stiffness coefficient of the right support (N/m)

L :

Length of squeeze film damper (m)

\(l_{1}\) :

Distance from the disk to the left support (m)

\(l_{2}\) :

Distance from the disk to the right support (m)

m :

Equivalent mass of the rotor (kg)

\(p_{1,2}\) :

Elements of modal matrix

\(Q_{1,2}\) :

Non-dimensional displacements in complex coordinates

\(\bar{{Q}}_{1,2} \) :

Non-dimensional displacements in principal coordinates

\(q_{11,22}\) :

Elements of inverse matrix of modal matrix

R :

Radius of the journal (m)

r :

Non-dimensional radial displacement of journal

t :

Time (s)

U :

Non-dimensional integrated unbalance value

\(\alpha _{1,2} \) :

Non-dimensional stiffness parameters

\(\gamma \) :

Length parameter

\(\delta \) :

Unbalance value (kg m)

\(\zeta \) :

Non-dimensional damping parameter

\(\eta \) :

Non-dimensional moment of inertia parameter

\(\theta _{1,2} \) :

Phase angles of vibration responses

\(\kappa \) :

Non-dimensional stiffness parameters

\(\mu \) :

Kinetic viscosity coefficient of oil (\(\hbox {N s/m}^{2}\))

\(\xi _{1,2} \) :

Non-dimensional damping parameters

\(\tau \) :

Non-dimensional time

\(\psi \) :

Angular displacement of journal (rad)

\(\omega \) :

Rotor speed (rad/s)

\(\omega _{1,2}\) :

Principal frequencies

\(\omega _c \) :

Natural frequency of rigid rotor on retainer springs (rad/s)

\(\varOmega \) :

Speed parameter

\(\bar{{\varOmega }}\) :

Ratio of rotor speed

References

  1. Cooper, S.: Preliminary investigation of oil films for control of vibration. Lubr. Wear Conven. 305–315 (1963)

  2. Della Pietra, L., Adiletta, G.: The squeeze film damper over four decades of investigations. Part II : rotordynamic analyses with rigid and flexible rotors. Shock Vib. Dig. 34(2), 97–126 (2002)

    Google Scholar 

  3. Jiao, Y., Chen, Z., Xia, S., et al.: Period solution bifurcation and stability analysis of nonlinear squeeze film damper rotor system. Mech. Sci. Technol. 23(7), 879–882 (2004)

    Google Scholar 

  4. Zhu, C.S., Robb, D.A., Ewins, D.J.: Analysis of the multiple-solution response of a flexible rotor supported on non-linear squeeze film dampers. J. Sound Vib. 252, 389–408 (2002)

    Article  Google Scholar 

  5. Qin, W., Zhang, J., Ren, X.: Response and bifurcation of rotor with squeeze film damper on elastic support. Chaos Solitons Fractals 39, 188–195 (2009)

    Article  Google Scholar 

  6. Bonello, P., Brennan, M.J., Holmes, R.: Non-linear modeling of rotor dynamic systems with squeeze film dampers—an efficient integrated approach. J. Sound Vib. 249(4), 743–773 (2002)

    Article  Google Scholar 

  7. Zhao, J.Y., Hahn, E.J.: Subharmonic, quasi-periodic and chaotic motions of a rigid rotor supported by an eccentric squeeze film damper. Proc. Inst. Mech.Eng. Part C J. Mech. Eng. Sci. 207, 383–392 (1993)

    Article  Google Scholar 

  8. Chu, F., Holmes, R.: Efficient computation on nonlinear responses of a rotating assembly incorporating the squeeze-film damper. Comput. Methods Appl. Mech. Eng. 164, 363–373 (1998)

    Article  MATH  Google Scholar 

  9. Meng, G., Xia, N.: Bifurcation and chaos responses of a flexible rotor-squeeze film damper system. Acta Aeronautica et Astronautica Sinica 24(1), 42–45 (2003)

    Google Scholar 

  10. He, E., Ren, X., Qin, W.: Chaotic response of cracked rotor supported on squeeze film damper and the routes to chaos. Chin. J. Aeronaut. 15(3), 145–149 (2002)

    Article  Google Scholar 

  11. Lin, F., Zhang, T., Meng, G.: Nonlinear characteristics of the rub-impact response of a rotor system supported on squeeze film dampers. J. Vib. Shock 23(1), 12–16 (2004)

    Google Scholar 

  12. Inayat-Hussain, J.I., Kanki, H., Mureithi, N.W.: Stability and bifurcation of a rigid rotor in cavitated squeeze-film dampers without centering springs. Tribol. Int. 34, 689–702 (2001)

    Article  MATH  Google Scholar 

  13. Inayat-Hussain, J.I.: Bifurcations of a flexible rotor response in squeeze-film dampers without centering springs. Chaos Solitons Fractals 24, 583–596 (2005)

    Article  MATH  Google Scholar 

  14. Inayat-Hussain, J.I.: Bifurcations in the response of a flexible rotor in squeeze-film dampers with retainer springs. Chaos Solitons Fractals 39, 519–532 (2009)

    Article  Google Scholar 

  15. Cai-Wan, C.-J., Chao-Kuang, C.: Chaos and bifurcation of a flexible rub-impact rotor supported by oil film bearings with nonlinear suspension. Mech. Mach. Theory 42, 312–333 (2007)

    Article  MATH  Google Scholar 

  16. Cao, D., Wang, L., Chen, Y., Huang, W.: Bifurcation and chaos of the bladed overhang rotor system with squeeze film dampers. Sci. China Ser. E Technol. Sci. 52(3), 709–720 (2009)

    Article  MATH  Google Scholar 

  17. Zhong, Y.E., He, Y.Z., Wang, Z., et al.: Rotordynamics. Tsinghua University Press, Beijing (1987)

    Google Scholar 

  18. Vlajic, N., Liu, X., Karki, H., et al.: Torsional oscillations of a rotor with continuous stator contact. Int. J. Mech. Sci. 83, 65–75 (2014)

    Article  Google Scholar 

  19. Yushu, C., Andrew, Y.L.T.: Bifurcation and Chaos in Engineering. Springer, London (1998)

    MATH  Google Scholar 

  20. Zhang, H., Chen, Y., Li, J.: Bifurcation on the synchronous full annular rub of a rigid-rotor elastic-support system. Appl. Math. Mech. (Engl. Ed.) 33(7), 865–880 (2012)

    Article  MathSciNet  Google Scholar 

  21. Chen, H., Chen, Y.: Analysis on bifurcation of a vibration jumping and engineering control in aero-engine. J. Aerosp. Power 28, 2781–2789 (2013)

    Google Scholar 

  22. Qin, Z.H., Chen, Y.S., Li, J.: Singularity analysis of a two-dimensional elastic cable with 1:1 internal resonance. Appl. Math. Mech. (Engl. Ed.) 31(2), 143–150 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  23. Li, J., Chen, Y.S.: Transition sets of bifurcation of dynamical system with two state variables with constraints. Appl. Math. Mech. (Engl. Ed.) 33(2), 139–154 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  24. Zhang, H., Chen, Y.: Bifurcation analysis on full annular rub of a nonlinear rotor system. Sci. China Technol. Sci. 54(8), 1977–1985 (2011)

    Article  MATH  Google Scholar 

  25. Qin, Z.-H.: Singularity Method for Nonlinear Dynamical Analysis of Systems with Two Parameters and Its Application in Engineering. Dissertation for the Doctoral Degree. Harbin Institute of Technology, Harbin (2010)

  26. Zhang, Z., Chen, Y., Cao, Q.: Bifurcations and hysteresis of varying compliance vibrations in the primary parametric resonance for a ball bearing. J. Sound Vib. 350, 171–184 (2015)

    Article  Google Scholar 

Download references

Acknowledgements

The authors would like to acknowledge the financial supports from the National Basic Research Program (973 Program) of China (Grant No. 2015CB057400). Funding was provided by China Postdoctoral Science Foundation (Grant No. 2016M590277), National Natural Science Foundation of China (Grant No. 11602070).

Author information

Authors and Affiliations

  1. School of Astronautics, Harbin Institute of Technology, Harbin, 150001, People’s Republic of China

    Huizheng Chen, Lei Hou & Yushu Chen

  2. School of Energy Science and Engineering, Harbin Institute of Technology, Harbin, 150001, People’s Republic of China

    Lei Hou

Authors
  1. Huizheng Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Lei Hou
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Yushu Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Lei Hou.

Appendix

Appendix

$$\begin{aligned} X_1= & {} \left[ {-2p_1 \zeta -\zeta \left( {1-\gamma } \right) +\frac{p_1 \left( {q_{22} -1} \right) }{q_{11} }\left( {\xi _{1} -\xi _{2} } \right) +\frac{(q_{22} -1)}{q_{11} }\left( {\xi _{1} +\gamma \xi _{2} } \right) } \right] \frac{\omega _1 a_1 }{p_1 +1}\\&+\left( {\frac{q_{22} -1}{q_{11} }{B}'-B} \right) \bar{{F}}_t\\ X_2= & {} \frac{2\left( {1-\bar{{\varOmega }}} \right) }{q_{11} }\frac{\omega _1 ^{2}}{p_1 }\frac{a_1 }{p_1 +1}+\frac{\left( {q_{22} -1} \right) }{q_{11} }\eta \bar{{\varOmega }}\frac{a_1 }{p_1 +1}\omega _1 ^{2}\\&+\left[ {B-\frac{(q_{22} -1)}{q_{11} }{B}'} \right] \bar{{F}}_r\\ X_3= & {} \left[ {-2p_1 \zeta -\zeta \left( {1-\gamma } \right) +\frac{p_1 \left( {q_{22} -1} \right) }{q_{11} }\left( {\xi _{1} -\xi _{2} } \right) +\frac{(q_{22} -1)}{q_{11} }\left( {\xi _{1} +\gamma \xi _{2} } \right) } \right] \frac{\omega _1 }{p_1 +1}\\&+\left( {\frac{q_{22} -1}{q_{11} }{B}'-B} \right) \frac{\partial \bar{{F}}_t }{\partial a_1 }\\ X_4= & {} \frac{2\left( {1-\bar{{\varOmega }}} \right) }{p_1 q_{11} }\frac{\omega _1 ^{2}}{p_1 +1}+\frac{\left( {q_{22} -1} \right) }{q_{11} }\eta \bar{{\varOmega }}\frac{\omega _1 ^{2}}{p_1 +1}+\left[ {B-\frac{(q_{22} -1)}{q_{11} }{B}'} \right] \frac{\partial \bar{{F}}_r }{\partial a_1 }\\ S_1= & {} -2 \omega _2 a_2 \left( {\sigma _1 +\sigma _2 } \right) +\eta \sigma _1 a_2 +2 \sigma _2 \omega _2 a_2 + \frac{2{B}' \left( {a_1 +a_2 } \right) a_2 }{\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{2}}, S_2 =\left( {\xi _1 -\xi _2 } \right) \omega _1 a_1 \\&- \frac{\pi {B}'a_1 }{2\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{3/2}}\\ S_3= & {} \left( {\alpha _1 -\alpha _2 } \right) a_1 + \frac{2{B}' \left( {a_1 +a_2 } \right) a_1 }{\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{2}},\\ S_4= & {} \left( {\xi _1 +\gamma \xi _2 } \right) \omega _2 a_2 -\frac{\pi {B}'a_2 }{2\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{3/2}},\\ S_5= & {} \left( {\xi _1 -\xi _2 } \right) \omega _1 a_1 + \frac{\pi {B}'a_1 }{2\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{3/2}}, S_6 = \frac{2{B}' a_2 }{\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{2}}+ \frac{8{B}' \left( {a_1 +a_2 } \right) ^{2}a_2 }{\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{3}},\\ S_7= & {} \left( {\xi _1 -\xi _2 } \right) \omega _1 - \frac{\pi {B}'}{2\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{3/2}}- \frac{3\pi {B}' \left( {a_1 + a_2 } \right) a_1 }{2\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{5/2}},\\ S_8= & {} \alpha _1 -\alpha _2 + \frac{2{B}'\left( {2a_1 +a_2 } \right) }{\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{2}}+ \frac{8{B}' \left( {a_1 +a_2 } \right) ^{2}a_1 }{\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{3}},\\ S_9= & {} \frac{3\pi {B}'a_2 \left( { a_1 + a_2 } \right) }{2\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{5/2}},\\ S_{10}= & {} \left( {\xi _1 -\xi _2 } \right) \omega _1 + \frac{\pi {B}'}{2\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{3/2}}+ \frac{3\pi {B}' \left( {a_1 + a_2 } \right) a_1 }{2\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{5/2}},\\ S_{11}= & {} -2\omega 2 \left( {\sigma _1 +\sigma _2 } \right) +\eta \sigma _1 +2 \sigma _2 \omega _2 + \frac{2{B}' \left( {a_1 +2a_2 } \right) }{\left( {1-\left( {a1+a2} \right) ^{2}} \right) ^{2}}+ \frac{8{B}' \left( {a_1 +a_2 } \right) ^{2}a_2 }{\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{3}},\\ S_{12}= & {} -\frac{3\pi {B}'a_1 \left( {a_1 + a_2 } \right) }{2\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{5/2}},\\ S_{13}= & {} \frac{2{B}' a_1 }{\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{2}}+ \frac{8{B}' \left( {a_1 +a_2 } \right) ^{2}a_1 }{\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{3}},\\ S_{14}= & {} \left( {\xi _1 +\gamma \xi _2 } \right) \omega _2 - \frac{\pi {B}' }{2\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{3/2}}-\frac{3\pi {B}'a_2 \left( { a_1 + a_2 } \right) }{2\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{5/2}}, \end{aligned}$$
$$\begin{aligned} Q_1= & {} 2 \zeta \omega _1 a_1 +\zeta \left( {1-\gamma } \right) \omega _2 a_2 +\frac{B\pi \left( {a_1 +a_2 } \right) }{2\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{3/2}},\\ Q_2= & {} 2 \zeta \omega _1 +\frac{3\pi B \left( {a_1 +a_2 } \right) ^{2}}{2\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{5/2}}+\frac{B\pi }{2\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{3/2}},\\ Q_3= & {} 2 \omega _1 a_1 \sigma _1 -\kappa a_2 - \frac{2B\left( {a_1 +a_2 } \right) ^{2}}{\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{2}},\\ Q_4= & {} 2 \omega _1 \sigma _1 - \frac{4B\left( {a_1 +a_2 } \right) }{\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{2}}- \frac{8B\left( {a_1 +a_2 } \right) ^{3}}{\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{3}},\\ Q_5= & {} \left( {1-\gamma } \right) \zeta \omega _2 + \frac{B\pi }{2\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{3/2}}+ \frac{3B\pi \left( {a_1 +a_2 } \right) ^{2}}{2\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{5/2}},\\ Q_6= & {} -\kappa - \frac{4B\left( {a_1 +a_2 } \right) }{\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{2}}- \frac{8B\left( {a_1 +a_2 } \right) ^{3}}{\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{3}}.\\ G_{1x}= & {} 2 Q_1 Q_2 +2 Q_3 Q_4 ,\quad G_{1y} =2 Q_1 Q_5 +2 Q_3 Q_6 ,\\ G_{2x}= & {} 2\left( {S_1 S_2 +S_3 S_4 } \right) \left( {S_2 S_6 +S_1 S_7 +S_4 S_8 +S_3 S_9 } \right) +2\left( {-S_4 S_5 +S_1 S_3 } \right) \\&\times \left( {-S_5 S_9 +S_4 S_{10} +S_3 S_6 +S_1 S_8 } \right) \\&+\,2\left( {S_2 S_5 -S_3 ^{2}} \right) \left( {S_5 S_7 +S_2 S_{10} -2S_3 S_8 } \right) \\ G_{2y}= & {} 2\left( {S_1 S_2 +S_3 S_4 } \right) \left( {S_2 S_{11} +S_1 S_{12} +S_4 S_{13} +S_3 S_{14} } \right) +2\left( {-S_4 S_5 +S_1 S_3 } \right) \\&\times \left( {-S_5 S_{14} -S_4 S_{12} +S_3 S_{11} +S_1 S_{13} } \right) \\&+\,2\left( {S_2 S_5 -S_3 ^{2}} \right) \left( {S_5 S_{12} -S_2 S_{12} -2S_3 S_{13} } \right) \\ G_{1\varOmega }= & {} -4 U^{2}\left( {1+\sigma _1 } \right) ^{3}+4 \left( {2 \omega _1 a_1 \sigma _1 -\kappa a_2 -2 \frac{B\left( {a_1 +a_2 } \right) ^{2}}{\left( {1-\left( {a_1 +a_2 } \right) ^{2}} \right) ^{2}}} \right) \omega _1 a_1 ,\\ G_{2\varOmega }= & {} 2\left( {-2 \omega _2 a_2 +\eta a_2 } \right) \left[ { \left( {S_1 S_2 } \right. \left. {+S_3 S_4 } \right) S_2 +S_3 \left( {-S_4 S_5 } \right. \left. {+S_1 S_3 } \right) } \right] ,\\ f_1= & {} G_{1xx} {a}'_1 {a}'_1 +2G_{1xy} {a}'_1 {a}'_2 +G_{1yy} {a}'_2 {a}'_2 , \quad f_2 =G_{2xx} {a}'_1 {a}'_1 +2G_{2xy} {a}'_1 {a}'_2 +G_{2yy} {a}'_2 {a}'_2 ,\\ G_{1xx}= & {} \frac{\partial G_{1x} }{\partial a_1 }, \quad G_{1xy} =\frac{\partial G_{1x} }{\partial a_2 }, \quad G_{1yy} =\frac{\partial G_{1y} }{\partial a_2 }, \quad G_{2xx} =\frac{\partial G_{2x} }{\partial a_1 }, \quad G_{2xy} =\frac{\partial G_{2x} }{\partial a_2 }\\ G_{2yy}= & {} \frac{\partial G_{2y} }{\partial a_2 },\hbox { and} {a}'_1, {a}'_2\hbox { satisfies }G_{1x} {a}'_1 +G_{1y} {a}'_2 =0 \end{aligned}$$

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chen, H., Hou, L. & Chen, Y. Bifurcation analysis of a rigid-rotor squeeze film damper system with unsymmetrical stiffness supports. Arch Appl Mech 87, 1347–1364 (2017). https://doi.org/10.1007/s00419-017-1254-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00419-017-1254-9

Keywords

Search

Navigation