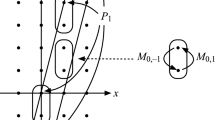
Abstract
The subtle interplay between local and global charges for topological semimetals exactly parallels that for singular vector fields. Part of this story is the relationship between cohomological semimetal invariants, Euler structures, and ambiguities in the connections between Weyl points. Dually, a topological semimetal can be represented by Euler chains from which its surface Fermi arc connectivity can be deduced. These dual pictures, and the link to topological invariants of insulators, are organised using geometric exact sequences. We go beyond Dirac-type Hamiltonians and introduce new classes of semimetals whose local charges are subtle Atiyah–Dupont–Thomas invariants globally constrained by the Kervaire semicharacteristic, leading to the prediction of torsion Fermi arcs.
Similar content being viewed by others
References
Atiyah, M.F.: Vector fields on manifolds. Arbeitsgemeinschaft für Forschung des Landes Nordrhein-Westfalen, vol. 200, pp. 7–26. VS, Cologne (1970)
Atiyah M.F., Dupont J.L.: Vector fields with finite singularities. Acta Math. 128(1), 1–40 (1972)
Atiyah M.F., Rees E.: Vector bundles on projective 3-space. Invent. Math. 35, 131–153 (1976)
Avila J.C., Schulz-Baldes H., Villegas-Blas C.: Topological invariants of edge states for periodic two-dimensional models. Math. Phys. Anal. Geom. 16(2), 137–170 (2013)
Avron J.E., Sadun L., Segert J., Simon B.: Topological invariants in Fermi systems with time-reversal invariance. Phys. Rev. Lett. 61, 1329 (1988)
Avron J.E., Sadun L., Segert J., Simon B.: Chern numbers, quaternions, and Berry’s phases in Fermi systems. Commun. Math. Phys. 124(4), 595–627 (1989)
Borel A., Hirzebruch F.: Characteristic classes and homogeneous spaces, I. Am. J. Math. 80(2), 458–538 (1958)
Borel A., Moore J.C.: Homology theory for locally compact spaces. Mich. Math. J. 7(2), 137–159 (1960)
Bott R., Tu L.W.: Differential Forms in Algebraic Topology. Grad. Texts in Math. 82. Springer, New York (1982)
Bradlyn, B., Cano, J., Wang, Z., Vergniory, M.G., Felser, C., Cava, R.J., Bernevig, B.A.: Beyond Dirac and Weyl fermions: unconventional quasiparticles in conventional crystals. Science 353(6299) (2016)
Brylinski J-L.: Loop Spaces, Characteristic Classes and Geometric Quantization. Progress in Mathematics, 107. Birkhauser Boston, Inc., Boston (1993)
Burghelea D., Haller S.: Euler structures, the variety of representations and the Milnor–Turaev torsion. Geom. Topol. 10, 1185–1238 (2006)
Chriss N., Ginzburg V.: Representation Theory and Complex Geometry. Birkhäuser, Boston (1997)
Carpentier D., Delplace P., Fruchart M., Gawędzki K.: Topological index for periodically driven time-reversal invariant 2D systems. Phys. Rev. Lett. 114(10), 106806 (2015)
De Nittis G., Gomi K.: Classification of “Quaternionic” Bloch-bundles topological quantum Systems of type AII. Commun. Math. Phys. 339, 1–55 (2015)
Dupont J.L.: Symplectic bundles and KR-theory. Math. Scand. 24(1), 27–30 (1969)
Dwivedi V., Chua V.: Of bulk and boundaries: generalized transfer matrices for tight-binding models. Phys. Rev. B 93, 134304 (2016)
Dwivedi V., Ramamurthy S.T.: Connecting the dots: time-reversal symmetric Weyl semimetals with tunable Fermi arcs. Phys. Rev. B 94, 245143 (2016)
Freed D.S., Moore G.W.: Twisted equivariant matter. Ann. Henri Poincaré 14(8), 1927–2023 (2013)
Fu L., Kane C.L., Mele E.J.: Topological insulators in three dimensions. Phys. Rev. Lett. 98(10), 106803 (2007)
Gawędzki K.: 2d Fu–Kane–Mele invariant as Wess–Zumino action of the sewing matrix. Lett. Math. Phys. 107(4), 733–755 (2017)
Hatsugai Y.: Edge states in the integer quantum Hall effect and the Riemann surface of the Bloch function. Phys. Rev. B 48(16), 11851–11862 (1993)
Hatsugai Y.: Symmetry-protected \({{\mathbb{Z}}_2}\) -quantization and quaternionic Berry connection with Kramers degeneracy. New J. Phys. 12, 065004 (2010)
Herring C.: Accidental degeneracy in the energy bands of crystals. Phys. Rev. 52, 365–373 (1937)
Huang S.-M. et al.: New type of Weyl semimetal with quadratic double Weyl fermions. Proc. Natl. Acad. Sci. USA 113(5), 1180–1185 (2016)
Hutchings M.: Reidemeister torsion in generalized Morse theory. Forum Math. 14, 209–244 (2002)
Iversen B.: Cohomology of Sheaves. Springer, Berlin (1986)
Kato T.: Perturbation Theory for Linear Operators. Classics in Mathematics. Springer, Berlin (1995)
Kaufmann R.M., Li D., Wehefritz-Kaufmann B.: Notes on topological insulators. Rev. Math. Phys. 28(10), 1630003 (2016)
Korbaš J.: Distributions, vector distributions, and immersions of manifolds in Euclidean spaces. In: Krupka, D., Saunders, D. (eds) Handbook of Global Analysis, pp. 665–724. Elsevier Science, Amsterdam (2008)
Kraus Y.E., Lahini Y., Ringel Z., Verbin M., Zilberberg O.: Topological states and adiabatic pumping in quasicrystals. Phys. Rev. Lett. 109, 106402 (2012)
Kuchment P.: An overview of periodic elliptic operators. Bull. Am. Math. Soc. 53, 343–414 (2016)
Lawson H.B., Michelsohn M.-L.: Spin Geometry. Princeton University Press, Princeton (1989)
Lin H., Yau S.-T.: On exotic sphere fibrations, topological phases, and edge states in physical systems. Int. J. Modern Phys. B 27(19), 1350107 (2013)
Lindner N.H., Refael G., Galitski V.: Floquet topological insulator in semiconductor quantum wells. Nat. Phys. 7, 490–495 (2011)
Liu, J., Fang, C., Fu, L.: Tunable Weyl fermions and Fermi arcs in magnetized topological crystalline insulators. arXiv:1604.03947
Lv B.Q. et al.: Experimental discovery of Weyl semimetal TaAs. Phys. Rev. X 5, 031013 (2015)
Mathai V., Thiang G.C.: T-duality of topological insulators. J. Phys. A: Math. Theor. 48(42), 42FT02. (2015) arXiv:1503.01206
Mathai V., Thiang G.C.: T-duality simplifies bulk-boundary correspondence. Commun. Math. Phys. 345(2), 675–701. (2016) arXiv:1505.05250
Mathai V., Thiang G.C.: T-duality simplifies bulk-boundary correspondence: some higher dimensional cases. Ann. Henri Poincaré 17(12), 3399–3424. (2016) arXiv:1506.04492
Mathai V., Thiang G.C.: Global topology of Weyl semimetals and Fermi arcs. J. Phys. A: Math. Theor. (Letter) 50(11), 11LT01 (2017) arXiv:1607.02242
Milnor J.: On manifolds homeomorphic to the 7-sphere. Ann. Math. (2) 64(2), 399–405 (1956)
Milnor J.: Topology from the Differentiable Viewpoint. Based on Notes by David W. Weaver. The University Press of Virginia, Charlottesville (1965)
Molina O.M.: Co-Euler structures on bordisms. Topol. Appl. 193, 51–76 (2015)
Murray M., Stevenson D.: The basic bundle gerbe on unitary groups. J. Geom. Phys. 58(11), 1571–1590 (2008)
Murray M., Stevenson D.: Bundle gerbes: stable isomorphism and local theory. J. Lond. Math. Soc. (2) 62(3), 925–937 (2000)
Nielsen H.B., Ninomiya M.: Absence of neutrinos on a lattice: (II). Intuitive topological proof. Nucl. Phys. B 193, 173–194 (1981)
Paechter G.F.: The groups π r (V n,m ). Q. J. Math. 7(1), 249–268 (1956)
Polyakov A.M.: Particle spectrum in quantum field theory. Pisma. Zh. Eksp. Theor. Fiz. 20, 430 (1974) [JETP Lett. 20 194 (1974)]
Prodan E.: Virtual topological insulators with real quantized physics. Phys. Rev. B 91, 245104 (2015)
Read N.: Compactly-supported Wannier functions and algebraic K-theory. Phys. Rev. B 95, 115309 (2017)
Rechtsman M.C.: Photonic Floquet topological insulators. Nature 496, 196–200 (2013)
Reed M., Simon B.: Methods of Modern Mathematica Physics. Vol. IV: Analysis of Operators. Elsevier, Amsterdam (1978)
Simon B.: Holonomy, the quantum adiabatic theorem, and Berry’s phase. Phys. Rev. Lett. 51(24), 2167 (1983)
Simons, J., Sullivan, D.: The Mayer–Vietoris property in differential cohomology. arXiv:1010.5269
Soluyanov A.A., Gresch D., Wang Z., Wu Q., Troyer M., Dai X., Bernevig B.A.: Type-II Weyl semimetals. Nature 527, 495–498 (2015)
Shaw R., Lever J.: Irreducible multiplier corepresentations of the extended Poincaré group. Commun. Math. Phys. 38(4), 279–297 (1974)
t’ Hooft G.: Magnetic monopoles in unified gauge theories. Nucl Phys. B 79(2), 276–284 (1974)
Tang Z., Zhang W.: A generalization of the Atiyah–Dupont vector fields theory. Commun. Contemp. Math. 4(4), 777–796 (2002)
Thiang G.C.: On the K-theoretic classification of topological phases of matter. Ann. Henri Poincaré. 17(4), 757–794 (2016)
Thomas E.: The index of a tangent 2-field. Comment. Math. Helv. 42(1), 86–110 (1967)
Thomas E.: Vector fields on manifolds. Bull. Am. Math. Soc. 75(4), 643–683 (1969)
Turaev V.: Euler structures, nonsingular vector fields, and torsions of Reidemeister type. Izv. Math. 34(3), 627–662 (1990)
Turaev V.: Torsion invariants of Spin c-structures on 3-manifolds. Math. Res. Lett. 4, 679–695 (1997)
Turner A.M., Vishwanath A.: Beyond band insulators: topology of semimetals and interacting phases. In: Franz, M., Molenkamp, L. (eds) Contemp. Concepts Cond. Mat. Sci. 6, Topological Insulators, pp. 293–324. Elsevier, Amsterdam (2013)
Varadarajan, V.S.: Supersymmetry for Mathematicians: An Introduction, vol. 11. Courant Institute of Mathematical Sciences at New York University, New York (2004)
von Neumann J., Wigner E.P.: Über merkwürdige diskrete Eigenwerte. Physik. Zeits. 30, 467–470 (1929)
Wan X., Turner A.M., Vishwanath A., Savrasov S.Y.: Topological semimetal and Fermi-arc surface states in the electronic structure of pyrochlore iridates. Phys. Rev. B 83, 205101 (2011)
Wigner E.P.: Unitary representations of the inhomogeneous Lorentz group including reflections. In: Gürsey, F. (eds) Group Theoretical Concepts in Elementary Particle Physics, vol. 1., pp. 37–80. Gordon and Breach, New York (1964)
Witten E.: Three lectures on topological phases of matter. La Rivista del Nuovo Cimento 39(7), 313–370 (2016)
Xu S.-Y. et al.: Discovery of a Weyl fermion semimetal and topological Fermi arcs. Science 349, 613–617 (2015)
Xu S.-Y. et al.: Discovery of a Weyl fermion state with Fermi arcs in niobium arsenide. Nat. Phys. 11, 748–754 (2015)
Xu Y., Zhang F., Zhang C.: Structured Weyl points in Spin-orbit coupled fermionic superfluids. Phys. Rev. Lett. 115, 265304 (2015)
Zhang C. et al.: Signatures of the Adler–Bell–Jackiw chiral anomaly in a Weyl fermion semimetal. Nat. Commun. 7, 10735 (2016)
Zhang S.-C., Lian B.: Five-dimensional generalization of the topological Weyl semimetal. Phys. Rev. B 94, 041105(R) (2016)
Zhao Y.X., Wang Z.D.: Topological classification and stability of Fermi surfaces. Phys. Rev. Lett 110, 240404 (2013)
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by M. Salmhofer
Rights and permissions
About this article
Cite this article
Mathai, V., Thiang, G.C. Differential Topology of Semimetals. Commun. Math. Phys. 355, 561–602 (2017). https://doi.org/10.1007/s00220-017-2965-z
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00220-017-2965-z