Skip to main content
SpringerLink
Log in

Solution of the Inverse Spectral Problem for a Rod Weakened by Transverse Cracks by the Levenberg—Marquardt Optimization Algorithm

  • Published:
Mechanics of Solids Aims and scope Submit manuscript

Abstract

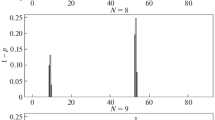
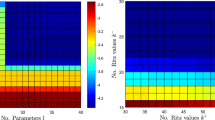
The longitudinal vibrations of a rod weakened by transverse cracks are considered. Cracks are assumed to be open and modeled by translational springs. The stiffness of the springs corresponds to the size of the cracks. A method has been developed for identifying the number and position of transverse cracks, as well as the stiffness of the corresponding springs according to two spectra that correspond to two types of conditions at the ends of the rod: free—free and fixed—free. The developed method is based on minimizing the objective function that characterizes the difference between the given (measured) natural frequencies and natural frequencies calculated during the implementation of the algorithm. The objective function is minimized using the Levenberg—Marquardt algorithm. Numerical examples are considered. The stability of the obtained results with respect to noise in the initial data is investigated.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. A. D. Dimarogonas, “Vibration of Cracked Structures: a State of the Art Review,” Engng Fract. Mech. 55, 831–857 (1996).

    Article  Google Scholar 

  2. Y. Narkis, “Identification of Crack Location in Vibrating Simply Supported Beams,” J. Sound Vib. 172 (4), 549–558 (1994).

    Article  ADS  MATH  Google Scholar 

  3. A. Morassi, “A Uniqueness Result on Crack Location in Vibrating Rods,” Inverse Prob. Engng 4, 231–254 (1997).

    Article  Google Scholar 

  4. G. Biscontin, A. Morassi, and P. Wendel, “Asymptotic Separation of the Spectrum in Notched Rods,” J. Vibr. Cont. 4, 237–251 (1998).

    Article  Google Scholar 

  5. A. Morassi, “Identification of a Crack in a Rod Based on Changes in a Pair of Natural Frequencies,” J. Sound Vibr. 242, 577–596 (2001).

    Article  ADS  Google Scholar 

  6. R. Ruotolo and C. Surace, “Natural Frequencies of a Bar with Multiple Cracks,” J. Sound Vibr. 272, 301–316 (2004).

    Article  ADS  Google Scholar 

  7. M. Dilena and A. Morassi, “The Use of Antiresonances for Crack Detection in Beams,” J. Sound Vibr. 276, 195–214 (2004).

    Article  ADS  Google Scholar 

  8. K. V. Singh, “Transcendental Inverse Eigenvalue Problems in Damage Parameter Estimation,” Mech. Syst. Sign. Proc. 23, 1870–1883 (2009).

    Article  Google Scholar 

  9. A. M. Akhtyamov and M. A. Il’gamov, “Flexural Model for a Notched Beam: Direct and Inverse Problems,” Prikl. Mat. Teor. Phiz. 54(1), 152–162 (2013)

    MATH  Google Scholar 

  10. A. M. Akhtyamov and M. A. Il’gamov, [J. Appl. Mech. Tech. Phys. (Engl. Transl.) 54(1), 132–141 (2013)].

    Article  ADS  Google Scholar 

  11. L. D. Akulenko and S. V. Nesterov, “Mass Defect Influence on the Longitudinal Vibration Frequencies and Mode Shapes of a Beam,” Izv. Ros. Akad. Nauk. Mekh. Tv. Tela, No. 1, 135–144. (2014)

  12. L. D. Akulenko and S. V. Nesterov, [Mech. Sol. (Engl. Transl.) 49 (1), 104–111 (2014)].

    Google Scholar 

  13. M. A. Il’gamov, “Longitudinal Vibrations of a Bar with Incipient Transverse Cracks,” Izv. Ros. Akad. Nauk. Mekh. Tv. Tela, No. 1, 23–31 (2017)

  14. M. A. Il’gamov, [Mech. Sol. (Engl. Transl.) 52 (1), 18–24 (2017)].

    Google Scholar 

  15. L. D. Akulenko, V. G. Baidulov, D. V. Georgievskii, and S. V. Nesterov, “Evolution of Natural Frequencies of Longitudinal Vibrations of a Bar as Its Cross-Section Defect Increases,” Izv. Ros. Akad. Nauk. Mekh. Tv. Tela, No. 6, 136–144 (2017)

  16. L. D. Akulenko, V. G. Baidulov, D. V. Georgievskii, and S. V. Nesterov, [Mech. Sol. (Engl. Transl.) 52(6), 708–714 (2017)].

    Google Scholar 

  17. A. Morassi and M. Rollo, “Identification of Two Cracks in a Simply Supported Beam from Minimal Frequency Measurements,” J. Vibr. Contr. 7, 729–739 (2001).

    Article  MATH  Google Scholar 

  18. L. Rubio, J. Fernandez-Saez, and A. Morassi, “Identification of Two Cracks in a Rod by Minimal Resonant and Antiresonant Frequency Data,” Mech. Syst. Sign. Proc. 60–61, 1–13 (2015).

    Google Scholar 

  19. L. Rubio, J. Fernandez-Saez, and A. Morassi, “Identification of Two Cracks with Different Severity in Beams and Rods from Minimal Frequency Data,” J. Vibr. Cont. 22 (13), 3102–3117 (2016).

    Article  MathSciNet  Google Scholar 

  20. N. T. Khiem and L. K. Toan, “A Novel Method for Crack Detection in Beam-Like Structures by Measurements of Natural Frequencies,” J. Sound Vibr. 333, 4084–4103 (2014).

    Article  ADS  Google Scholar 

  21. E. I. Shifrin, “Identification of a Finite Number of Small Cracks in a Rod Using Natural Frequencies,” Mech. Sys. Sign. Proc. 70–71, 613–624 (2016).

    Article  Google Scholar 

  22. K. V. Singh, “Transcendental Inverse Eigenvalue Problems in Damage Parameter Estimation,” Mech. Sys. Sign. Proc. 23, 1870–1883 (2009).

    Article  Google Scholar 

  23. J. Lee, “Identification of Multiple Cracks in a Beam Using Natural Frequencies,” J. Sound Vibr. 320, 482–490 (2009).

    Article  ADS  Google Scholar 

  24. J. Lee, “Identification of a Crack in a Beam by the Boundary Element Method,” J. Mech. Sci. Techn. 24 (3), 801–804 (2010).

    Article  Google Scholar 

  25. S. Casciati, “Stiffness Identification and Damage Localization Via Differential Evolution Algorithms,” Struct. Cont. Health Mon. 15, 436–449 (2008).

    Article  Google Scholar 

  26. N. Khaji and M. Mehrjoo, “Crack Detection in a Beam with an Arbitrary Number of Transverse Cracks Using Genetic Algorithms,” J. Mech. Sci. Techn. 28(3), 823–836 (2014).

    Article  Google Scholar 

  27. U. Eroglu and E. Tufekci, “Exact Solution Based Finite Element Formulation of Cracked Beams for Crack Detection,” Int. J. Sol. Struct. 96, 240–253 (2016).

    Article  Google Scholar 

  28. S. Sandesh and K. Shankar, “Application of a Hybrid of Particle Swarm and Genetic Algorithm for Structural Damage Detection,” Inv. Prob. Sci. Engng. 18 (7), 997–1021 (2010).

    Article  MATH  Google Scholar 

  29. L. Rubio, J. Fernandez-Saez, and A. Morassi, “The Full Nonlinear Crack Detection Problem in Uniform Vibrating Rods,” J. Sound Vibr. 339, 99–111 (2015).

    Article  ADS  Google Scholar 

  30. J. Fernandez-Saez, A. Morassi, M. Pressacco, and L. Rubio, “Unique Determination of a Single Crack in a Uniform Simply Supported Beam in Bending Vibration,” J. Sound Vibr. 371, 94–109 (2016).

    Article  ADS  Google Scholar 

  31. E. I. Shifrin, “Inverse Spectral Problem for a Rod with Multiple Cracks,” Mech. Syst. Sig. Proc. 56–57, 181–196 (2015).

    Article  Google Scholar 

  32. E. I. Shifrin, “Inverse Spectral Problem for a Non-Uniform Rod with Multiple Cracks,” Mech. Syst. Sign. Proc. 96, 348–365 (2017).

    Article  Google Scholar 

  33. J. M. Melenk and I. Babuska, “The Partition of Unity Finite Element Method: Basic Theory and Applications,” Comp. Met. Appl. Mech. Engng 139, 289–314 (1996).

    Article  MathSciNet  MATH  Google Scholar 

  34. I. Babuska and J. M. Melenk, “The Partition of Unity Method,” Int. J. Num. Met. Engng 40, 727–758 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  35. M. Arndt, R. D. Machado, and A. Scremin, “An Adaptive Generalized Finite Element Method aapplied to Free Vibration Analysis of Straight Bars and Trusses,” J. Sound Vibr. 329, 659–672 (2010).

    Article  ADS  Google Scholar 

  36. K. Levenberg, “A Method for the Solution of Certain Non-Linear Problems in Least Squares,” Quart. Appl. Math. 2 (2), 164–168 (1944).

    Article  MathSciNet  MATH  Google Scholar 

  37. D. W. Marquardt, “An Algorithm for the Least-Squares Estimation of Nonlinear Parameters,” SIAM J. Appl. Math. 11 (2), 431–441 (1963).

    Article  MathSciNet  MATH  Google Scholar 

  38. M. Lampton, “Damping-Undamping Strategies for Levenberg-Marquardt Nonlinear Least-Squares Method,” Comp. Phys. 11 (1), 110–115 (1997).

    Article  Google Scholar 

  39. M. I. A. Lourakis, “A Brief Description of the Levenberg-Marquardt Algorithm Implemented by Levmar,” Found. Res. Techn. 4 (1), 1–6 (2005).

    Google Scholar 

  40. J. Pujol, “The Solution of Nonlinear Inverse Problems and the Levenberg-Marquardt Method,” Geophys. 72(4), W1–W16 (2007).

    Article  MathSciNet  Google Scholar 

  41. F. Sarvi, S. Shojaee, and P. Torkzadeh, “Damage Identification of Trusses by Finite Element Model Updating Using an Enhanced Levenberg-Marquardt Algorithm,” Int. J. Opt. Civil Engng. 4 (2), 207–231 (2014).

    Google Scholar 

  42. R. L. Fox and M. P. Kapoor, “Rates of Change Eigenvalues and Eigenvectors,” AIAA J. 6 (12), 2426–2429 (1968).

    Article  ADS  MATH  Google Scholar 

  43. R. B. Nelson, “Simplified Calculation of Eigenvector Derivatives,” AIAA J. 14 (9), 1201–1205 (1976).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  44. N. P. Van Der Aa, H. G. Ter Morsche, and R. R. M. Mattheij, “Computation of eigenvalue and eigenvector derivatives for a general complex-valued eigensystem,” Electron. J. Lin. Alg. 16(1), 300–314 (2007).

    MathSciNet  MATH  Google Scholar 

  45. E. I. Shifrin and R. Ruotolo, “Natural Frequencies of a beam with an Arbitrary Number of Cracks,” J. Sound Vibr. 222 (3), 409–423 (1999).

    Article  ADS  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Ishlinsky Institute for Problems in Mechanics of the Russian Academy of Sciences, pr. Vernadskogo 101, str. 1, Moscow, 119526, Russia

    I. M. Lebedev & E. I. Shifrin

Authors
  1. I. M. Lebedev
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. E. I. Shifrin
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to E. I. Shifrin.

Additional information

Russian Text © The Author(s), 2019, published in Izvestiya Akademii Nauk, Mekhanika Tverdogo Tela, 2019, No. 4, pp. 8–26.

Acknowledgement

This work was carried out on the subject of state assignment No. of state registration AAAA-A17-117021310386-3 and with the support of the RFBR (project No. 19-01-00100).

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Lebedev, I.M., Shifrin, E.I. Solution of the Inverse Spectral Problem for a Rod Weakened by Transverse Cracks by the Levenberg—Marquardt Optimization Algorithm. Mech. Solids 54, 857–872 (2019). https://doi.org/10.3103/S0025654419060025

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.3103/S0025654419060025

Keywords

Search

Navigation