Skip to main content
SpringerLink
Log in

Convergence results of iterative algorithms for the sum of two monotone operators in reflexive Banach spaces

  • Published:
Applications of Mathematics Aims and scope Submit manuscript

Abstract

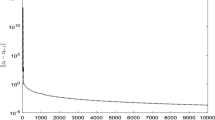
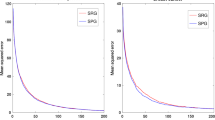
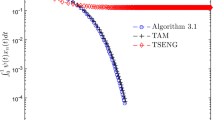
The aim of this paper is to propose two modified forward-backward splitting algorithms for zeros of the sum of a maximal monotone operator and a Bregman inverse strongly monotone operator in reflexive Banach spaces. We prove weak and strong convergence theorems of the generated sequences by the proposed methods under some suitable conditions. We apply our results to study the variational inequality problem and the equilibrium problem. Finally, a numerical example is given to illustrate the proposed methods. The results presented in this paper improve and generalize many known results in recent literature.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. R. P. Agarwal, D. O’Regan, D. R. Sahu: Fixed Point Theory for Lipschitzian-type Mappings with Applications. Topological Fixed Point Theory and Its Applications 6. Springer, New York, 2009.

    MATH  Google Scholar 

  2. Y. I. Alber: Generalized projection operators in Banach spaces: Properties and applications. Funct. Differ. Equ. 1 (1993), 1–21.

    MathSciNet  MATH  Google Scholar 

  3. Y. I. Alber: Metric and generalized projection operators in Banach spaces: Properties and applications. Theory and Applications of Nonlinear Operator of Accretive and Monotone type. Lecture Notes in Pure and Applied Mathematics 178. Marcel Dekker, New York, 1996, pp. 15–50.

    MATH  Google Scholar 

  4. V. Barbu, T. Precupanu: Convexity and Optimization in Banach Spaces. Springer Monographs in Mathematics. Springer, Dordrecht, 2012.

    Book  MATH  Google Scholar 

  5. H. H. Bauschke, J. M. Borwein, P. L. Combettes: Essential smoothness, essential strict convexity, and Legendre functions in Banach spaces. Commun. Contemp. Math. 3 (2001), 615–647.

    Article  MathSciNet  MATH  Google Scholar 

  6. H. H. Bauschke, X. Wang, L. Yao: General resolvents for monotone operators: Characterization and extension. Biomedical Mathematics: Promising Directions in Imagine, Therapy Planning and Inverse Problem (Huangguoshu 2008) (Y. Censor, M. Jiang, G. Wang, eds.). Medical Physics Publishing, Madison, 2010, pp. 57–74.

  7. A. Beck: First-Order Methods in Optimization. MOS-SIAM Series on Optimization 25. SIAM, Philadelphia, 2017.

    Book  MATH  Google Scholar 

  8. D. P. Bertsekas, J. N. Tsitsiklis: Parallel and Distributed Computation: Numerical Methods. Athena Scientific, Belmont, 1997.

    MATH  Google Scholar 

  9. J. F. Bonnans, A. Shapiro: Perturbation Analysis of Optimization Problems. Springer Series in Operations Research. Springer, New York, 2000.

    Book  MATH  Google Scholar 

  10. J. M. Borwein, S. Reich, S. Sabach: A characterization of Bregman firmly nonexpansive operators using a new monotonicity concept. J. Nonlinear Convex Anal. 12 (2011), 161–184.

    MathSciNet  MATH  Google Scholar 

  11. L. M. Bregman: The relaxation method of finding the common point of convex sets and its application to the solution of problems in convex programming. U.S.S.R. Comput. Math. Math. Phys. 7 (1967), 200–217; translation from Zh. Vychisl. Mat. Mat. Fiz. 7 (1967), 620–631.

    Article  MathSciNet  MATH  Google Scholar 

  12. D. Butnariu, E. Resmerita: Bregman distances, totally convex functions, and a method for solving operator equations in Banach spaces. Abstr. Appl. Anal. 2006 (2006), Article ID 84919, 39 pages.

  13. S.-S. Chang, C.-F. Wen, J.-C. Yao: Generalized viscosity implicit rules for solving quasi-inclusion problems of accretive operators in Banach spaces. Optimization 66 (2017), 1105–1117.

    Article  MathSciNet  MATH  Google Scholar 

  14. S.-S. Chang, C.-F. Wen, J.-C. Yao: Common zero point for a finite family of inclusion problems of accretive mappings in Banach spaces. Optimization 67 (2018), 183–1196.

    Article  MathSciNet  MATH  Google Scholar 

  15. S.-S. Chang, C.-F. Wen, J.-C. Yao: A generalized forward-backward splitting method for solving a system of quasi variational inclusions in Banach spaces. Rev. R. Acad. Cienc. Exactas Fís. Nat., Ser. A Mat., RACSAM 113 (2019), 729–747.

    Article  MathSciNet  MATH  Google Scholar 

  16. S.-S. Chang, C.-F. Wen, J.-C. Yao: Zero point problem of accretive operators in Banach spaces. Bull. Malays. Math. Sci. Soc. (2) 42 (2019), 105–118.

    Article  MathSciNet  MATH  Google Scholar 

  17. G. H.-G. Chen, R. T. Rockafellar: Convergence rates in forward-backward splitting. SIAM J. Optim. 7 (1997), 421–444.

    Article  MathSciNet  MATH  Google Scholar 

  18. P. Cholamjiak, N. Pholasa, S. Suantai, P. Sunthrayuth: The generalized viscosity explicit rules for solving variational inclusion problems in Banach spaces. To appear in Optimization.

  19. P. L. Combettes, V. R. Wajs: Signal recovery by proximal forward-backward splitting. Multiscale Model. Simul. 4 (2005), 1168–1200.

    Article  MathSciNet  MATH  Google Scholar 

  20. J. C. Dunn: Convexity, monotonicity, and gradient processes in Hilbert space. J. Math. Anal. Appl. 53 (1976), 145–158.

    Article  MathSciNet  MATH  Google Scholar 

  21. O. Güler: On the convergence of the proximal point algorithm for convex minimization. SIAM J. Control Optim. 29 (1991), 403–419.

    Article  MathSciNet  MATH  Google Scholar 

  22. S. He, C. Yang: Solving the variational inequality problem defined on intersection of finite level sets. Abstr. Appl. Anal. 2013 (2013), Article ID 942315, 8 pages.

  23. R. Iyer, J. A. Bilmes: Submodular-Bregman and the Lovász-Bregman divergences with applications. Advances in Neural Information Processing Systems 25 (NIPS 2012). MIT Press, Cambridge, 2012, 9 pages.

    Google Scholar 

  24. Y. Kimura, K. Nakajo: Strong convergence for a modified forward-backward splitting method in Banach spaces. J. Nonlinear Var. Anal. 3 (2019), 5–18.

    MATH  Google Scholar 

  25. P.-L. Lions, B. Mercier: Splitting algorithms for the sum of two nonlinear operators. SIAM J. Numer. Anal. 16 (1979), 964–979.

    Article  MathSciNet  MATH  Google Scholar 

  26. G. López, V. Martín-Márquez, F. Wang, H.-K. Xu: Forward-backward splitting methods for accretive operators in Banach spaces. Abstr. Appl. Anal. 2012 (2012), Article ID 109236, pages 25 pages.

  27. P. E. Maingé: Approximation methods for common fixed points of nonexpansive mappings in Hilbert spaces. J. Math. Anal. Appl. 325 (2007), 469–479.

    Article  MathSciNet  MATH  Google Scholar 

  28. V. Martín-Márquez, S. Reich, S. Sabach: Iterative Methods for approximating fixed points of Bregman nonexpansive operators. Discrete Contin. Dyn. Syst., Ser. S 6 (2013), 1043–1063.

    Article  MathSciNet  MATH  Google Scholar 

  29. E. Naraghirad: Halpern’s iteration for Bregman relatively nonexpansive mappings in Banach spaces. Numer. Funct. Anal. Optim. 34 (2013), 1129–1155.

    Article  MathSciNet  MATH  Google Scholar 

  30. E. Naraghirad, J.-C. Yao: Bregman weak relatively nonexpansive mappings in Banach space. Fixed Point Theory Appl. 2013 (2013), Article ID 141, 43 pages.

  31. F. Nielsen, S. Boltz: The Burbea-Rao and Bhattacharyya centroids. IEEE Trans. Inf. Theory 57 (2011), 5455–5466.

    Article  MathSciNet  MATH  Google Scholar 

  32. F. Nielsen, R. Nock: Generalizing skew Jensen divergences and Bregman divergences with comparative convexity. IEEE Signal Process. Lett. 24 (2017), 1123–1127.

    Article  MATH  Google Scholar 

  33. F. U. Ogbuisi, C. Izuchukwu: Approximating a zero of sum of two monotone operators which solves a fixed point problem in reflexive Banach spaces. Numer. Funct. Anal. Optim. 41 (2020), 322–343.

    Article  MathSciNet  MATH  Google Scholar 

  34. H. K. Pathak: An Introduction to Nonlinear Analysis and Fixed Point Theory. Springer, Singapore, 2018.

    Book  MATH  Google Scholar 

  35. S. Reich: A weak convergence theorem for the alternating method with Bregman distances. Theory and Applications of Nonlinear Operators of Accretive and Monotone Type. Lecture Notes in Pure and Applied Mathematics 178. Marcel Dekker, New York, 1996, pp. 313–318.

    Google Scholar 

  36. S. Reich, S. Sabach: Two strong convergence theorems for a proximal method in reflexive Banach spaces. Numer. Funct. Anal. Optim. 31 (2010), 22–44.

    Article  MathSciNet  MATH  Google Scholar 

  37. S. Reich, S. Sabach: Two strong convergence theorems for Bregman strongly nonexpansive operators in reflexive Banach spaces. Nonlinear Anal., Theory Methods Appl., Ser. A 73 (2010), 122–135.

    Article  MathSciNet  MATH  Google Scholar 

  38. S. Reich, S. Sabach: Existence and approximation of fixed points of Bregman firmly nonexpansive mappings in reflexive Banach spaces. Fixed-Point Algorithms for Inverse Problems in Science and Engineering. Springer Optimization and Its Applications 49. Springer, New York, 2011, pp. 301–316.

    MATH  Google Scholar 

  39. R. T. Rockafellar: Convex Analysis. Princeton University Press, Princeton, 1970.

    Book  MATH  Google Scholar 

  40. R. T. Rockafellar: On the maximal monotonicity of subdifferential mappings. Pac. J. Math. 33 (1970), 209–216.

    Article  MathSciNet  MATH  Google Scholar 

  41. R. T. Rockafellar: Monotone operators and the proximal point algorithm. SIAM J. Control Optim. 14 (1976), 877–898.

    Article  MathSciNet  MATH  Google Scholar 

  42. S. Sabach: Products of finitely many resolvents of maximal monotone mappings in reflexive Banach spaces. SIAM J. Optim. 21 (2011), 1289–1308.

    Article  MathSciNet  MATH  Google Scholar 

  43. Y. Shehu: Convergence results of forward-backward algorithms for sum of monotone operators in Banach spaces. Result. Math. 74 (2019), Article ID 138, 24 pages.

  44. Y. Shehu, G. Cai: Strong convergence result of forward-backward splitting methods for accretive operators in Banach spaces with applications. Rev. R. Acad. Cienc. Exactas Fís. Nat., Ser. A Mat., RACSAM 112 (2018), 71–87.

    Article  MathSciNet  MATH  Google Scholar 

  45. S. Suantai, P. Cholamjiak, P. Sunthrayuth: Iterative methods with perturbations for the sum of two accretive operators in q-uniformly smooth Banach spaces. Rev. R. Acad. Cienc. Exactas Fís. Nat., Ser. A Mat., RACSAM 113 (2019), 203–223.

    Article  MathSciNet  MATH  Google Scholar 

  46. P. Sunthrayuth, P. Cholamjiak: Iterative methods for solving quasi-variational inclusion and fixed point problem in q-uniformly smooth Banach spaces. Numer. Algorithms 78 (2018), 1019–1044.

    Article  MathSciNet  MATH  Google Scholar 

  47. W. Takahashi, N.-C. Wong, J.-C. Yao: Two generalized strong convergence theorems of Halpern’s type in Hilbert spaces and applications. Taiwanese J. Math. 16 (2012), 1151–1172.

    Article  MathSciNet  MATH  Google Scholar 

  48. P. Tseng: A modified forward-backward splitting method for maximal monotone mappings. SIAM J. Control Optim. 38 (2000), 431–446.

    Article  MathSciNet  MATH  Google Scholar 

  49. Y. Wang, F. Wang: Strong convergence of the forward-backward splitting method with multiple parameters in Hilbert spaces. Optimization 67 (2018), 493–505.

    Article  MathSciNet  MATH  Google Scholar 

  50. H.-K. Xu: Inequalities in Banach spaces with applications. Nonlinear Anal., Theory Methods Appl. 16 (1991), 1127–1138.

    Article  MathSciNet  MATH  Google Scholar 

  51. C. Zălinescu: Convex Analysis in General Vector Spaces. World Scientific, Singapore, 2002.

    Book  MATH  Google Scholar 

  52. H. Zegeye, N. Shahzad: An algorithm for finding a common point of the solution set of a variational inequality and the fixed point set of a Bregman relatively nonexpansive mapping. Appl. Math. Comput. 248 (2014), 225–234.

    MathSciNet  MATH  Google Scholar 

  53. J.-H. Zhu, S.-S. Chang: Halpern-Mann’s iterations for Bregman strongly nonexpansive mappings in reflexive Banach spaces with applications. J. Inequal. Appl. 2013 (2013), Article ID 146, 14 pages.

Download references

Acknowledgements

The authors express their deep gratitude to the referee and the editor, their valuable comments and suggestions helped tremendously in improving the quality of this paper and made it suitable for publication.

Author information

Authors and Affiliations

  1. College of Mathematics and Statistics, Chongqing Technology and Business University, Chongqing, 400067, P. R. China

    Yan Tang

  2. Department of Mathematics and Computer Science, Faculty of Science and Technology, Rajamangala University of Technology Thanyaburi (RMUTT), Pathumthani, 12110, Thailand

    Ratthaprom Promkam

  3. School of Science, University of Phayao, Phayao, 56000, Thailand

    Prasit Cholamjiak

  4. Department of Mathematics and Computer Science, Faculty of Science and Technology, Rajamangala University of Technology Thanyaburi (RMUTT), Pathumthani, 12110, Thailand

    Pongsakorn Sunthrayuth

Authors
  1. Yan Tang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ratthaprom Promkam
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Prasit Cholamjiak
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Pongsakorn Sunthrayuth
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Pongsakorn Sunthrayuth.

Additional information

Y. Tang was funded by the Natural Science Foundation of Chongqing (CSTC2019JCYJmsxmX0661), the Science and Technology Research Project of Chongqing Municipal Education Commission (KJQN 201900804) and the Research Project of Chongqing Technology and Business University (KFJJ1952007). P. Cholamjiak was supported by Thailand Science Research and Innovation under the project IRN62W0007. P. Sunthrayuth was supported by the RMUTT Research Grant for New Scholar under Grant NSF62D0602.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Tang, Y., Promkam, R., Cholamjiak, P. et al. Convergence results of iterative algorithms for the sum of two monotone operators in reflexive Banach spaces. Appl Math 67, 129–152 (2022). https://doi.org/10.21136/AM.2021.0108-20

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.21136/AM.2021.0108-20

Keywords

MSC 2020

Search

Navigation