Short-range nanoregions and nanosized defects in LiFeO$_2$ compound deduced from Mössbauer spectroscopy and Rietveld analysis

Abstract

We examined the crystal and magnetic properties of the polymorphic LiFeO$_2$ compound using Mössbauer spectra (MS), X-ray diffraction data (XRD), and magnetic measurements. X-ray diffraction analysis of $\alpha$-LiFeO$_2$ and $\beta '$-LiFeO$_2$ phases reveals that short-range ordering observed in the $\alpha$-LiFeO$_2$ phase is potentially linked to the $\beta '$-LiFeO$_2$ phase nanoregions. Low-temperature MS of the $\alpha$-LiFeO$_2$ phase reveal a complicated disordered magnetic state below 90 K. Rietveld analysis of the XRD data of the $\gamma$-LiFeO$_2$ phase reveals a defect microstructure. These defects produce a complicated distribution of the hyperfine magnetic field estimated from MS. While it is not possible to produce the $\beta '$-LiFeO$_2$ phase in pure form, extended annealing of the $\alpha$-LiFeO$_2$ phase at 400 $^\circ$C yields a nanocomposite material comprising nanoregions of both $\beta '$-LiFeO$_2$ and $\gamma$-LiFeO$_2$ phases.

Graphical abstract

Introduction

Ternary oxides LiMO$_2$ (M = Ti, Mn, Fe, Ni, and Co) display interesting crystal and physical properties [1] and can be used in several applications, among the most current being cathodic materials in lithium-ion batteries. The polymorphic LiFeO$_2$ compound has been studied in the past by several researchers [2,3,4,5,6,7,8,9,10,11,12,13]. Its crystal structure depends on temperature and pressure. At high temperatures $750\,^\circ {\text{C}}\le T\le 1100^\circ {\text{C}}$ and ambient pressures, LiFeO$_2$ crystalizes in the cubic NaCl-type structure [4] (space group $Fm\bar{3}m$ (No. 225)). This is the $\alpha \text {-LiFeO}_2$ phase. Since this phase is metastable below $700\,^\circ$C it can be prepared by quenching or slow cooling from high temperatures due to slow kinetics in transforming to more stable forms, $\gamma$, $\beta$, or $\beta '$ (see Supplementary information (SI) Fig. S1). This compound has been studied in the past with transmission electron microscopy [6, 14,15,16] neutron and Mössbauer spectroscopy [4, 17,18,19]. Moreover, Layek et al. [11] discovered a pressure-induced spin crossover of the Fe ion from high to low spin in $\alpha$-LiFeO$_2$. Du et al. [19] observed peculiar magnetic ac-susceptibility data in $\alpha$-LiFeO$_2$ phase, attributing this behavior to iron-rich nano-scale clusters with short-range order.

The earlier neutron diffraction data of Cox et al. [4] revealed that at $T=4.2$ K, in addition to the diffraction peaks predicted from the cubic $\alpha$-LiFeO$_2$ crystal structure, broad diffraction peaks with half-integer indices appear, attributed to sort-range magnetic correlations of the Fe$^{3+}$ magnetic moment. These peaks can be interpreted assuming an MnO-type magnetic structure for the iron magnetic moments. To account for the relative intensity of the magnetic Bragg peaks (1/2, 1/2, 1/2) and (3/2, 1/2, 1/2) Cox et al. [4] argued that the spin axis lies within the (111) plane. These broad peaks are present and above 90 K, implying that their origin has to do also with some short-range order. The high-temperature diffuse scattering is also observed in the X-ray [6, 7] and electron diffraction patterns [15, 20], indicating that it may arise from Fe and Li clustering (short-range order). For the $\beta$, $\beta '$, and $\gamma$ phases, there are only a few studies available [4, 9, 18, 21,22,23]. Some of them pertain to crystallographic studies of samples prepared using hydrothermal synthesis [18, 21, 22]. The works of Brunel et al. [23] and Famery et al. [9] on single crystal and powder samples attempt to explain the defect structure of these compounds using antiphase structural models. Mössbauer spectra have been published sporadically, for selected temperatures without any direct connection to the X-ray and magnetization data.

As previously noted, apart from the interesting crystal chemistry properties of the LiFeO$_2$ compound, these lithium double oxides may also prove to be useful in lithium battery technology. Hu et al. [24] demonstrated that nanosized $\alpha$-LiFeO$_2$ can be a cathode material with excellent electrochemical performance. Zhang et al. [25] found that a polypyrrole coating to $\alpha$-LiFeO$_2$ nanoparticles enhanced their capacity and cycling stability in lithium batteries. It was found [26, 27] that nanosized $\alpha$-LiFeO$_2$ electrodes provide good capacity during the first cycles and show adequate cycling life. Rahman et al. [12] found that a thin carbon layer on a nanosized $\alpha$-LiFeO$_2$ anode electrode enhances capacity and cycle stability. Morales et al. [28] studied $\alpha$-LiFeO$_2$ electrodes during cycling, and they confirmed that Fe ions change valence during this procedure and that after the first cycle, a layer of solid electrolyte interface begins to form. With $\alpha$-LiFeO$_2$ as the cathode material, a 150 mAh/g stabilized capacity is achievable. Hirayama et al. [29] found that reducing the particle size and disorder in the cation layers enhances the electrochemical activity of LiFeO$_2$ particles. Lee et al. [30] observed that an orthorhombic form of LiFeO$_2$ collapses into the spinel structure during cycling, with a significant impact on capacity. Wu et al. [31] found that there are no structural changes to the cubic lattice during cycling. Suresh et al. [32] found support that the solution-combustion production of LiFeO$_2$ leads to enhanced initial capacity. The $\gamma$-LiFeO$_2$ phase has also been studied electrochemically as an anode material for lithium-ion batteries [33].

Here, we present a detailed crystallographic and Mössbauer study of three of the polymorphic phases of the LiFeO$_2$ compound, prepared by solid-state reaction of Li$_2$CO$_3$ and Fe$_2$O$_3$ reagents in an air atmosphere. We also use magnetic measurements to study the magnetic properties and the magnetic transitions. Our MS, specific heat and magnetization data indicate that the $\alpha \text {-LiFeO}_2$ exhibit a disordered magnetic state below 90 K. The $\gamma \text {-LiFeO}_2$ phase is produced in pure form after prolonged annealing at $600\,^\circ$C for 30 days. Fitting of the MS and Rietveld analysis shows that in this phase, extended defects, possible anti-phase domains (inherent to the ordering structural transition) produce selective broadening of the XRD Bragg peaks and a distribution of the magnetic effective hyperfine magnetic fields seen by iron nuclei. The $\beta '\text {-LiFeO}_2$ phase cannot be produced as a single-phase material. Prolonged annealing of the $\alpha \text {-LiFeO}_2$ phase at $400\,^\circ$C produces a nanocomposite material consisting of nanoregions of $\beta '\text {-LiFeO}_2$ and $\gamma \text {-LiFeO}_2$ phases. A small amount ($\approx 5\%$) of $\alpha \text {-LiFeO}_2$ phase remains untransformed. From the time evolution of the $\alpha \text {-LiFeO}_2$ phase at $400\,^\circ$C, we can conclude that the short-range ordering observed in the $\alpha \text {-LiFeO}_2$ phase is related to nanoregions of the $\beta '\text {-LiFeO}_2$ phase.

Rietveld analysis of the X-ray diffraction data

X-ray diffraction data of $\alpha \text{-LiFeO}_2$ phase

The x-ray diffraction data of of $\alpha \text{-LiFeO}_2$ phase were analyzed with the Rietveld method (see SI Fig. S2 for the Rietveld plot). The Bragg peaks are described with a pseudo-Voigt function ($\# 7$, see FullProf user guide [34]). The angular dependence of the full width at the half maximum (FWHM) of the Gaussian and Lorentzian parts are given by the formulas $\Gamma _G^2 = U\tan ^2\theta + V\tan \theta + W + IG/\cos ^2\theta$ and $\Gamma _L = X\tan \theta + Y/\cos \theta$, respectively. The instrumental FWHM parameters are estimated using the NIST 640C standard LiB$_6$ sample. The parameters reported here represent the additional broadening with respect to the LiB$_6$ sample. Only the X, Y parameters are refined. The others were held to be zero, ($W=U=V=IG=0$). We refine the crystal structure (see SI Fig. S1 (upper panel)) with a cubic face-centered space group $Fm\overline{3}m$. In that case, the only structural parameters are the cell constants, the relative occupancy of Li and Fe at the 4a, (0, 0, 0) (site symmetry $m\overline{3}m$) and the thermal parameters. The estimated cell constant is found to be $a=4.159(1)$Å  and the relative occupancy ratio of Li:Fe was estimated to be nearly 1:1, within the standard deviations ($5\%$ for iron and $10\%$ for lithium). The Lorentzian isotropic strain parameter $X=0.017(1)$ deg., and the Lorentzian isotropic size parameter $Y\approx 0$ (giving a resolution limited apparent size $\langle D \rangle \sim 1\mu \text {m}$). The refinement converged, giving profile, weight profile, and Bragg factors: $R_p=6\%$, $R_{wp}=7\%$, and $R_B=4.8\%$, respectively. By a close inspection of the background, one can see significant diffuse scattering around 20°, 30° and 60°. The intensity around 30° arises mainly from the glass sample holder. Similar diffuse scattering has been observed in the neutron diffraction data of Cox et al. [4]. As we see below, the diffuse scattering may be related to the $\beta '-\text{ LiFeO}_2$ nanoregions.

X-ray diffraction data of $\gamma \text{-LiFeO}_2$ phase

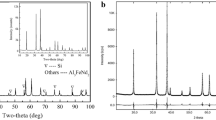
Figure 1 shows the Rietveld plot of the refined XRD data of the $\gamma \text{-LiFeO}_2$ phase. $\gamma \text{-LiFeO}_2$ is a tetragonal distorted superstructure form of the $\alpha \text{-LiFeO}_2$ compound with (see SI, Fig. S1 (middle panel)) $a_t<a_c$, $c_t>2a_c$, where $a_t,c_t,a_c$ are the cell constants of the tetragonal and cubic phase, respectively. The crystal structure was refined using the space group $I 4_1/a m d$ (No. 141). The Li and Fe cations occupy the special positions 4b and 4a with coordinates (0, 1/4, 3/8) and (0, 3/4, 1/8), respectively, both with point symmetry $-\,4m2$. The oxygen anions occupy the special position 8e (0, 1/4, z). The Rietveld method was applied to the XRD measurements of the $\gamma \text{-LiFeO}_2$ compound, adopting the following strategy. Similar to the $\alpha$ phase case, we set $U=V=IG=0$ and $W=0$. During the refinement, the global strain and size parameters X, Y, respectively, are left free to vary. The free parameters in the refinement were the cell parameters, the oxygen z coordinate, the Li, Fe, and O temperature factors, and the Fe and Li relative occupancy in the special positions 4a and 4b. With this model, the refinement converged, giving the following agreement parameters $R_p=11.8\%$, $R_{wp}=16.1\%$, $R_B=3.85\%$. Although the obtained $R_B$ has an acceptable value, the profile agreement factors $R_p, R_{wp}$ are rather high.

By close inspection of the refined X-ray diffraction patterns, we find that several peaks, mainly describing the ordering of lithium and iron ions, are broader than in comparison to those of the disordered state. This selective broadening observed in the Bragg peaks with $\ell =2n+1$ (arising from Li/Fe ordering) leads us to model these peaks with a different size parameter (IsizeModel$\,=-\,1$, see Fullprof user guide [34], p. 22), significantly improving the Rietveld agreement parameters. Convergence was achieved with the following agreement parameters $R_p=8.9$, $R_{wp}=13.2$, $\chi ^2=1.84$ and $R_{B}=2.46$. These parameters yielding the $\chi ^2$ minimum are: $a=4.0469(1)$Å, $c=8.7489(1)$Å, $z(O) = 0.1052(2)$, $o(\text{4a }) = 0.247(\text{ Li})+0.003(\text{ Fe})$, $o(\text{4b }) = 0.247(\text{ Fe})+0.003(\text{ Li})$, $B(\text{ Li})=1.4(3)$Å$^2$, $B(\text{ Fe})=0.31(2)$Å$^2$, $B(\text{ O})=0.27(5)$Å$^2$, $X = 0.085(4)$ (deg.), $Y = 0.029(2)$ (deg.) and Lorentzian size parameter, for Bragg peaks with $\ell =2n+1$, equal to 1.31(3). The significant finding from the Rietveld refinement is that the lithium and iron ions occupy the special positions 4b and 4a, respectively. The bond lengths and bond valence sums of the ferric iron and lithium ions, which are octahedrally coordinated with oxygen ions, are estimated to be $\text {Li--O}=2.360(3)\mathrm{\text{\AA }}\times 2$, $\text {Li--O}=2.0308(2)\mathrm{\text{\AA }}\times 4$, $BVS=0.881(1)$ and $\text {Fe--O}=2.015(3)\mathrm{\text{\AA }}\times 2$, $\text {Fe--O}=2.0308(2)\mathrm{\text{\AA }}\times 4$, $BVS=2.961(5)$, respectively. The Fe–O bond lengths exhibit minimal variation for iron, leading to an approximately regular octahedron. In contrast, for lithium, two out of the six Li–O bond lengths are greater than the remaining four, resulting in an elongated octahedron. The bond valence sums closely approach the ideal values of 1 for Li$^{1+}$ and 3 for Fe$^{3+}$. The refined crystal structure results obtained at this stage agree well with neutron data from samples prepared using solid-state reaction and hydrothermal synthesis methods [4, 18, 21].

Figure 1

(Upper panel) Rietveld plot of $\gamma$-LiFeO$_2$ phase. The open dots correspond to the experimental X-ray diffraction pattern. The red solid line is the theoretical x-ray diffraction pattern, calculated from the structural and microstructural parameters. The short vertical lines indicate the position of the expected Bragg peaks. The black solid line represents the difference between the experimental and theoretical calculated patterns. The lower panel shows Rietveld plots of selected reflections.

Figure 2

Williamson–Hall plot of $\gamma \text{-LiFeO}_2$ phase.

Let us next discuss what information on the microstructure can be obtained from the selective broadening of the Bragg peaks. Figure 2 illustrates the Williamson–Hall (WH) diagram [35] constructed using FWHM parameters, which describe both the average and superstructure Bragg peaks ($\ell =2n+1$). In this diagram, we plot the effective FWHM parameter $\beta ^*_{hk\ell }=\beta _{hk\ell }\sin \theta /\lambda$ versus the inverse distance $d^*_{hk\ell }=1/d_{hk\ell }$ for all the Bragg peaks. The points on the WH diagram are categorized into two groups with distinct size parameters characteristic of the superstructure and the peaks associated with the disordered structure. According to the WH relation $\beta ^{*} = 2\epsilon d^{*} + 1/\langle L \rangle$, the data points should lie on two straight lines. The intersections of these lines with the y-axis yield an estimation of the inverse average crystalline size, while the slope provides the $2\epsilon$ parameter which is related to strain. As the two categories of peaks possess distinct size parameters, the Williamson–Hall graph is expected to exhibit two parallel lines, as it does. From the slope of the lines, we calculate the maximum strain [36] parameter $\epsilon = 5.88(4)\times 10^{-4}$. Furthermore, the y-axis intersection points allow an estimate of the average crystalline size for both categories of Bragg peaks. Specifically, the average size of the diffraction domains associated with the Li/Fe ordering measures $\langle L \rangle = 54$ nm, while the average size deduced from the Bragg peaks of the disordered structure $\langle L \rangle = 188$ nm. This microstructure nay result from antiphase domains.

The differential temperature analysis (DTA) measurements versus temperature (see SI, Fig. S2) for the $\gamma$-LiFeO$_2$ phase show on heating, a clear endothermic peak is observed with onset temperature $T_\text{on}=687$ °C and local minimum temperature $T_\text{min}=709$ °C. This negative peak is related to the endothermic structural transformation from the ordered to the disordered state ($\gamma \text{-LiFeO}_2\rightarrow \alpha \text{-LiFeO}_2$). The area of the endothermic negative peak represents the latent heat that must be absorbed by $\gamma \text{-LiFeO}_2$ (ordered state) to be transformed into $\alpha \text{-LiFeO}_2$ (disordered state). While cooling, one may expect an exothermic peak due to the anticipated transition from a disordered to an ordered state. However, the kinetics of this structural transformation are very slow (in comparison to the time needed to cool the sample in the DTA apparatus, a few hours), which does not allow the $\alpha$ to $\gamma$ phase transformation at this specific cooling rate.

X-ray diffraction data of the nominal $\beta '\text{-LiFeO}_2$ sample

Despite using various heating protocols, we were unable to successfully synthesize the $\beta '\text{-LiFeO}_2$ phase in pure form through a solid-state reaction in an air atmosphere. Figure 3 illustrates the time evolution of the XRD patterns from our attempts to prepare the $\beta '\text{-LiFeO}_2$ phase.

Figure 3

(Panels a–f) Evolution with annealing time at $\sim 400\,^\circ$C for 37 days of the XRD patterns of a sample which initial has the $\alpha \text {-LiFeO}_2$ structure. The panels (a)–(f) display the powder XRD patterns after 3, 10, 16, 23, 30, and 37 days of annealing, respectively. (lower panel) Rietveld plot of LiFeO$_2$, sample annealed at 400 °C for a month. In the lower panel, the intensity scale is linear, whereas in the upper panel is logarithmic.

Initially, we began with a sample containing only $\alpha \text{-LiFeO}_2$ phase. As the annealing time increased part of $\alpha \text{-LiFeO}_2$ phase transforms into the $\beta '\text{-LiFeO}_2$ phase (see panels (a)–(c) of Fig. 3).

It is interesting to note that at the locations where diffuse scattering is observed in the XRD data of the $\alpha \text{-LiFeO}_2$ phase, the low-angle Bragg peaks of the $\beta '\text{-LiFeO}_2$ phase develop. Therefore, it is reasonable to relate the diffuse scattering observed in the $\alpha$-phase to $\beta '$‘-phase nanodomains. Furthermore, for more extended annealing periods, the XRD patterns show that the weight percentage of the $\gamma \text{-LiFeO}_2$ phase tends to increase, whereas the amount of $\alpha \text{-LiFeO}_2$ phase is reduced by about $5\%$ (for an annealing period of 30 days). It is known that the pure $\beta '\text{-LiFeO}_2$ phase can only be prepared using hydrothermal synthesis methods [21, 22].

Figure 3 (lower panel) shows the Rietveld plots of the sample annealed for 30 days at $400\,^{\circ }$C. The Rietveld refinement is performed using a three-phase model, consisting of $\alpha \text{-LiFeO}_2$, $\beta '\text{-LiFeO}_2$, and $\gamma \text{-LiFeO}_2$ phases. By employing the structural models of the $\gamma \text{-LiFeO}_2$ and $\alpha \text{-LiFeO}_2$ phases (“X-ray diffraction data of $\alpha \text{-LiFeO}_2$ phase” and “X-ray diffraction data of $\gamma \text{-LiFeO}_2$ phase” sections), as well as the structural model of the $\beta '\text{-LiFeO}_2$ phase reported by Barre and Catti [21], and applying the same methodology used in the case of the $\gamma \text{-LiFeO}_2$ phase to address the selective broadening (see “X-ray diffraction data of $\gamma \text{-LiFeO}_2$ phase” section), we were able to reproduce the experimental XRD patterns satisfactorily. The estimated weight percentages for the three LiFeO$_2$ phases are $54\%$ for $\beta '$, $41\%$ for $\gamma$ and $5\%$ for $\alpha$. The estimated cell constants for the three phases are as follows: $a=4.1570(1)$Å for the cubic $\alpha \text{-LiFeO}_2$ phase, $a=4.071(1)$Å and $c=8.657(2)$Å for the tetragonal $\gamma \text{-LiFeO}_2$ phase, and $a=8.581(1)$Å, $b=11.561(2)$Å, $c=5.202(1)$Å and $\beta =146.13(1)^{\circ }$ for the monoclinic $\beta '\text{-LiFeO}_2$ phase. The diffraction peaks of the $\beta '$ phase with $k=2n+1$ display selective broadening. The size parameter for these Bragg peaks was estimated as $SP=10(1)$, whereas the Lorentzian size parameter for the remaining Bragg peaks was estimated as $Y=0.26(1)$. These values correspond to diffraction domain sizes of approximately 6.1 nm and 21 nm, respectively. Here, the reflections of the $\gamma$ phase display severe selective broadening, especially those with $\ell =2n+1$. The size parameter estimated through Rietveld refinement for the Bragg peaks with $\ell =2n+1$ was $SP=26(1)$, corresponding to a diffraction domain size of about 3(1) nm. The Lorentzian size parameter for the Bragg peaks with $\ell \ne 2n+1$ was estimated to be $Y=0.23(1)$, indicating a domain size of 24(1) nm. What is interesting here is that both $\beta '\text{-LiFeO}_2$ and $\gamma \text{-LiFeO}_2$ phases show a severe broadening of the diffraction peaks, indicating that these phases consist of nanoregions possible within the original crystallites of the $\alpha \text{-LiFeO}_2$ phase. In practice, the prolonged annealing of the $\alpha \text{-LiFeO}_2$ phase at this temperature produces a nanocomposite material via a meta-stable phase. It is important to emphasize that the remaining small amount of $\alpha \text{-LiFeO}_2$ phase has narrow diffraction peaks. The phase identification mentioned above is based only on Rietveld analysis of the powder XRD patterns. The knowledge of the XRD pattern of the $\alpha$-phase and the XRD patterns observed during the $\alpha$ to $\gamma$ transformation it leaves little doubt about the correctness of our model. However, future electron transmission diffraction data may also provide an additional check about the phase indication and the spatial arrangement of the observed nanophases.

Mössbauer spectra

$\alpha$-LiFeO$_2$

Figure 4 shows the paramagnetic Mössbauer spectra of the $\alpha$-LiFeO$_2$ compound, measured at $T=290$ K. The spectrum consists of a symmetric, doublet, with half-width at half maximum (HWHM, $\Gamma /2$) larger than the instrumental minimum of our spectrometer ($\Gamma /2\approx 0.14$ mm/s).

Figure 4

(upper panel) Experimental Mössbauer spectrum (points) of $\alpha$-LiFeO$_2$, compound at $T=290$ K. The red solid line represents the theoretical spectrum, calculated with the hyperfine parameters obtained by least squares fitting using two doublets depicted with green and magenta colored solid lines. The (middle panel) shows the same spectrum as the upper panel. The theoretical spectrum (red solid line) is calculated using the quadrupole splitting distribution (see lower panel) estimated with the Le Caer–Dubois method. The blue lines represent the difference between the experimental and theoretical calculated spectra.

Figure 5

(left panel) Magnetically split MS of $\alpha$-LiFeO$_2$ phase, fitted with two sextets, supposing an asymmetric Gaussian distribution of the effective hyperfine magnetic field and a symmetric distribution of the quadrupole parameter. The line positions were calculated with the first-order perturbation approximation for the quadrupole interaction. (right panel) Distributions of the hyperfine magnetic field extracted from the fitting of the MS, using a two-sites model.

An initial attempt to fit the spectra with only one doublet ($\Gamma /2 = 0.3$ mm/s, $\delta = 0.4$ mm/s, and $\Delta E_Q = 0.7$ mm/s) was unsatisfactory. By adding a second doublet, the fitting results were significantly improved. The estimated hyperfine parameters for the two-site model are: $\Gamma _1/2 = 0.18$ mm/s, $\delta _1 = 0.38(1)$ mm/s, $\Delta E_{Q,1} = 0.48(1)$ mm/s, $\Gamma _2/2 = 0.17(1)$ mm/s, $\delta _2 = 0.38(1)$ mm/s, and $\Delta E_{Q,2} = 0.87(1)$ mm/s. The relative spectral areas of the two components were found to be $A_1:A_2 = 63:37$. Similar paramagnetic spectra were observed in previous studies [4, 17].

The fitting result can be significantly improved if we assume a continuous distribution of the $\Delta E_Q$ values. This task can be accomplished with the help of the Le Caer–Dubois method [37]. In this method, if some hyperfine parameter, denoted as $x = H, \Delta E_Q$, is randomly distributed and can be described by a probability density function, it can be determined by minimizing the following quantity:

$$\begin{aligned} \tilde{\chi }^2=\chi ^2+\lambda \int _{0}^{\infty }\left( \frac{d^2p(x)}{dx^2}\right) ^2dx, \end{aligned}$$

(1)

where $\lambda$ is the smoothing parameter, $\chi ^2 = \sum _{i=1}^{N} w_i(Y_e(i) - Y(i))^2$ (the classical Chi-squared statistic), and $Y_e(i)$, Y(i) represent the experimental and theoretical relative absorptions in the i-channel.

The shape of the calculated distribution depends on the smoothing parameter and the FWHM $(\Gamma )$. To avoid introducing artificial peaks and features in the $\Delta E_Q$-distribution, we have chosen (using trial end error methodology, see [37]) $\lambda = 85$ and $\Gamma /2 = 0.14$ mm/s as the values for the smoothing parameter and the HWHM, respectively. The isomer shift was estimated from the centroid of the MS. The value which gives the minimum $\tilde{\chi }^2=504$ is estimated $\delta =0.38$ mm/s in perfect agreement with the earlier, simpler, two-component model. This value of the isomer shift indicates high spin ferric iron in an octahedral environment comprising oxygen atoms [38]. The isomer shift value also agrees very well with values estimated by Knop et al. [17] (0.364(8) mm/s), Tabuchi et al. [18] (0.37 mm/s), Cox et al. [4] (graphical estimation 0.381 mm/s) and Du et al. [19] (0.37 mm/s doublet I and 0.36 mm/s doublet II). The fitted spectrum and the extracted distribution are shown in Fig. 4 (middle and lower panels). Importantly, the salient features of the estimated $p(\Delta E_Q)$ distribution, including peaks and shoulders, can be directly associated with the two components utilized in the simplified model. The non-zero value of $\Delta E_Q$ and, more importantly, the existence of at least two zones in the distribution of $\Delta E_Q$ is an interesting finding. The $\alpha \text{-LiFeO}_2$ phase crystallizes in the cubic crystallographic system, where iron ions occupy the sites with point group symmetry $m\overline{3}m$. A zero average $\langle \Delta E_Q\rangle =0$ is expected. The peculiarities of the $\Delta E_Q$ distribution (or the two doublet model used to fit the MS spectra) imply that some short-range order exists in the $\alpha \text{-LiFeO}_2$ phase. The Mössbauer spectra from room temperature to $T=95$ K remain paramagnetic without significant changes. Only the isomer shift, and the area slightly increase as the temperature decreases.

Below $T=90$ K, the spectra further broaden, indicating the onset of a magnetic transition. As the temperature decreases, the splitting of the absorption Mössbauer lines increases while their broadening decreases (see Fig. 5). At $T=4.2$ K, a well-defined sextet is observed, albeit with noticeable line broadening. Additionally, the asymmetry in the shapes of lines 1 and 6, combined with the narrow width of lines 3 and 4, reveals that the spectrum consists of at least two components (two sextets). The spectrum cannot be satisfactorily fitted without using two distinct quadrupole shift values, in agreement with the earlier finding of distinct quadrupole splitting values at higher temperatures. Thus, the magnetic MS are fitted with two sextets with distributions of quadrupole shift (u) and hyperfine magnetic field (H). To avoid over-parametrization of the fitting procedure, we have used a constrained model by supposing that the two components have the same half-width at the half maximum ($\Gamma _1/2=\Gamma _2/2$) and the same isomer shift ($\delta _1=\delta _2$). Considering the quadrupole interaction as a first-order perturbation, the velocities of the six absorption lines are given from the relations (in velocity units) $v_i=\gamma _iH+\beta _iu+\delta$, where $\beta _1=\beta _6=1$, $\beta _2=\beta _3=\beta _4=\beta _5=-\,1$, $\gamma _1=-\gamma _6= -\,0.016035$ (mm/s)/(kG), $\gamma _2=-\gamma _5= -\,0.00928$ (mm/s)/(kG), and $\gamma _3=-\gamma _4= -\,0.002535$ (mm/s)/(kG). The parameter u represents the first-order correction of the excited nuclear levels and is given by the formula: $u=(3/2)[3\cos \theta ^2-1+\eta \sin \theta ^2\cos 2\phi ](e^2Qq/12)$, where $\theta$ and $\phi$ are the polar angles of the H to the principal axes of the EFG tensor of the $^{57}$Fe nucleus. In addition, the MS are convoluted with a symmetric Gaussian distribution of the u parameter, $p(u)=1/(\sqrt{2\pi }\sigma _u)\exp [(u-\bar{u})^2/2\sigma _u^2]$, and an asymmetric distribution of the H,

$$\begin{aligned} p(H)= \frac{2/\sqrt{2\pi }}{\sigma _l+\sigma _h}\left\{ \begin{matrix} e^{-(H-\bar{H})^2/2\sigma _l^2}, \text{ for } H\le \bar{H}\\ e^{-(H-\bar{H})^2/2\sigma _h^2}, \text{ for } H\ge \bar{H} \end{matrix} \right. \end{aligned}$$

(2)

for each site. Practically, H takes values within a finite interval. Therefore, in the fitting routine, a truncated asymmetric distribution of H has been implemented, where the magnetic field is restricted to a specific range (0 or $\bar{H} - n_l\sigma _l \le H \le \bar{H} + n_h\sigma _h$), with $n_h$ and $n_l$ both equal to 5. The parameters $\bar{u}$ and $\sigma _u$ represent the mean and standard deviation of the variable u, respectively. Similarly, $\bar{H}$, $\sigma _l$, and $\sigma _h$ represent respectively the mean, low-field, and high-field standard deviations of the variable H. The left panel of Fig. 5 shows the experimental MS together with two sextets, which the hyperfine parameters give the lower $\chi ^2$. The right panel depicts the hyperfine field distributions calculated from the parameters we obtained from the fitting process. The hyperfine parameters obtained from fitting of the MS at $T=4.2$ K are: $\Gamma _1/2=\Gamma _2/2=0.14$ mm/s (fixed for both sites), $\delta _1=\delta _2=0.482(1)$ mm/s, (are constrained to be equal) $H_1=500(1)$ kOe, $u_1=0.035(1)$ mm/s, $\sigma _{u,1}=0.125$ mm/s (fixed), $\sigma _{l,1}=20(1)$ kOe, $\sigma _{h,1}=11(1)$ kOe and $A_1=63\%$, $H_2=487(1)$ kOe, $u_2=-\,0.0565(2)$ mm/s, $\sigma _{u,2}=0.125$ mm/s (fixed), $\sigma _{l,2}=33(1)$ kOe, $\sigma _{h,2}=6(1)$ kOe and $A_2=37\%$.

Figure 6

(upper panel) Temperature variation of the peak of the hyperfine magnetic field distributions for the two sextets, derived from least-squares fitting of the magnetically split Mössbauer spectra. The solid line with pink color represents the p(H) distribution at $T=85$ K. (middle panel) Temperature variation of the dc-magnetic susceptibility, measured in zero-field-cooling and field-cooling protocols under an external magnetic field 1 kOe. (lower panel) Temperature variation of the experimental isomer shift (solid circles) of the $\alpha$-LiFeO$_2$ phase. The solid lines are calculated by using the equation $\delta (T)=\delta _C+\delta _{\text{SOS}}(T)$, with $\Theta _{D}=400-600$ K.

The upper panel of Fig. 6 show the temperature evolution of the peaks of the hyperfine magnetic fields distributions for the two sextets. The middle panel of Fig. 6 illustrates the temperature variation of the dc-magnetic susceptibility, $\chi _\text{dc}(T)$, as measured using the standard zero-field-cooling (ZFC) and field-cooling (FC) protocols under an external magnetic field of 1 kOe. A distinct difference between the ZFC and FC branches is evident below the temperature at which the MS exhibits magnetic splitting. This behavior has been observed in numerous systems with magnetic disorder, where the peak in the ZFC branch of $\chi _\text{dc}(T)$ has been attributed to a magnetic glass transition [39].

Figure 6 (lower panel) shows the temperature variation of the experimental isomer shift, obtained from the MS fitting, using a two-site model. From the temperature variation of isomer shift, the Debye temperature can be obtained. The isomer shift, $\delta$ can be written [38] as the sum of the chemical isomer shift, $\delta _\text{C}$ (which is practically temperature independent) and second-order Doppler shift, $\delta _\text{SOS}$ (temperature dependent), $\delta =\delta _\text{C}+\delta _\text{SOS}$.

The second-order Doppler shift is given (in velocity units) by the formula $\delta _\text{SOD}(T) = -\langle \upsilon ^2\rangle /(2c)$, where $\langle \upsilon ^2\rangle$ represents the mean square velocity of the Mössbauer atom, and c stands for the speed of light. When considering a Debye phonon spectrum, then (see Shenoy [40]):

$$\begin{aligned} \langle \upsilon ^2\rangle =\frac{9k_\text{B}\Theta _D}{8M}+\frac{9k_\text{B} T}{M} \left( \frac{T}{\Theta _D}\right) ^3\int _0^{\Theta _\text{D}/T}\frac{x^3}{\exp x-1}dx, \end{aligned}$$

(3)

where $k_\text{B}$ represents the Boltzmann constant, M stands for the mass of the $^{57}$Fe isotope, $\Theta _D$ denotes the Debye temperature, and T indicates the absolute temperature. Based on experimental data, $\delta _C+\delta _\text{SOS}(0)$ is estimated to be approximately 0.49 mm/s. In Fig. 6 (lower panel), the solid lines depict theoretical $\delta (T)=\delta _{C}+\delta _\text{SOS}(T)$ curves calculated numerically using the Debye model, for Debye temperatures ranging from 400 K to 600 K in increments of 50 K. The curve that closely passes through the experimental data corresponds to a Debye temperature of $\Theta _D=550\pm 50$ K.

Heat capacity measurements of $\alpha \text{-LiFeO}_2$

The upper and middle panels of Fig. 7 depict the temperature variation of the heat capacity under constant pressure $C_p$ and the ratio $C_p/T$ of the $\alpha$-LiFeO$_2$ phase, respectively. Our data exhibit good agreement with those $(80 \text{ K }\le T\le 300 \text{ K})$ of King [41]. The higher-temperature region of $C_p\approx 40$ J mol$^{-1}$K$^{-1}$ remains beneath the Dulong-Petit limit, $C_\text{DP}\approx 3(n=2)R\approx 49.4$ J mol$^{-1}$K$^{-1}$, where $R=8.314$ J mol$^{-1}$K$^{-1}$ is the gas constant and $n=2$ signifies the number of atoms per formula unit Li$_{0.5}$Fe$_{0.5}$O [42]. This finding suggests that the Debye temperature significantly exceeds 300 K. Unlike the clear peak observed in the ZFC branch of the $\chi _\text{dc}(T)$ curve (see middle panel of Fig. 6), the specific heat does not display any anomaly as expected for a paramagnetic-antiferromagnetic phase transition. The absence of a lambda-like peak in the specific heat suggests a broad distribution of Néel’s temperatures due to numerous configurations existing in a mixed crystal, where in addition, one of the two atoms is diamagnetic. A wide Néel temperature distribution effectively blurs the anticipated lambda-type magnetic transition in the specific heat data. The broad distribution of the effective hyperfine magnetic field (starting from zero magnetic field), observed within the temperature range of [70 K, 85 K], further supports this explanation for the absence of a lambda-type magnetic transition.

Figure 7

(upper panel) Temperature variation of the experimental heat capacity of the $\alpha \text{-LiFeO}_2$ phase, measured under constant pressure. The solid lines represent the theoretical specific heat ( magenta line) resulting from the superposition of three isotropic acoustic modes (blue line, $\Theta _D=430$ K, “Debye contribution”) and three optical modes (red line, $\Theta _{E1}=550$ K, $\Theta _{E2}=\Theta _{E3}=690$ K, “Einstein contribution”). (middle panel) The same data set as presented in panel (a), but plotted as $C_p/T$ versus T. (lower panel) Variation of $C_p/T$ with $T^2$ at low temperatures. The red colored line represents the curve $C_p = B_2T^2 + B_3T^3$ (refer to the main text).

Over a broad temperature range, the phonon component of the specific heat can be effectively described using a hybrid Debye-Einstein model (as outlined in [43], p. 77).

$$\begin{aligned} \begin{aligned} C_\text{DE}=&9R\left( \frac{T}{\Theta _\text{D}}\right) ^3 \int _{0}^{\Theta _\text{D}/T} \frac{x^4e^x}{(e^x-1)^2}dx+\\&+3R\sum _{i=1}^{3}\frac{(\Theta _{E,i}/T)^2 e^{\Theta _{E,i}/T}}{(e^{\Theta _{E,i}/T}-1)} \end{aligned} \end{aligned}$$

(4)

The first term represents the specific heat contribution from three degenerate acoustic phonons. The second term approximates the contribution of optical phonons using three Einstein modes with Einstein temperatures $\Theta _{E1}=550$ K and $\Theta _{E2}=\Theta _{E3}=690$ K. The Debye, Einstein contributions and their sum are depicted with solid lines in the upper and middle panels of Fig. 7. The additional specific heat relative to the total, occurring below 90 K, is probably related to the contribution of the disordered magnetic state. The lower panel of Fig. 7 displays the low-temperature variation of the $C_p/T$ as a function of $T^2$. The experimental data can be reproduced with cubic and quadratic temperature terms $C_p=B_2T^2+B_3 T^3$, with $B_2=1.91^{-3}$ J mol$^{-1}$K$^{-3}$ and $B_3=1.872\times 10^{-5}$ J mol$^{-1}$K$^{-4}$. The low-temperature specific heat for a magnetic insulator can be approximated by the relation [44]

$$\begin{aligned} C_p=C_\text{ph}+C_\text{sw}. \end{aligned}$$

The first term $C_\text{ph}=B_3T^3$, represents the acoustic phonons contribution to the specific heat with $B_3=(12\pi ^4nR /5\Theta _\text{D}^3)$. $n=2$ is the number of atoms per chemical formula [42] and $\Theta _\text{D}$ is the Debye temperature. From the $B_3$ constant considering three degenerate acoustic modes, we deduce a Debye temperature $\Theta _\text{D}=470$ K. The conventional Debye temperature for four atoms per molecule (e.g. LiFeO$_2$) is $2^{1/3}\Theta _\text{D}=592$ K. This value agrees with the one we estimated from the temperature dependence of the isomer shift. The second term is possibly related to magnon excitations. Kubo [45] predicted a quadratic low-temperature variation of the specific heat for a 2D Heisenberg antiferromagnet in the temperature interval $E_a< k_\text{B}T < k_\text{B}T_\text{N}$, where $E_a$ represents the magnetic anisotropy energy in the spin wave spectrum.

It is helpful to compare our specific heat data with those of LiCoO$_2$ [46, 47]. The existence, in effect, of only one cubic term in low-temperature data [47] has been explained as a signature of the fully stoichiometric LiCoO$_2$. From the low-temperature cubic term a Debye temperature $\Theta _D=834\pm 28$ K (or $834(2/4)^{1/3}=589$ K for a Li$_{0.5}$Co$_{0.5}$O formula unit) is estimated. This value is higher that deduced for Li$_{0.5}$Fe$_{0.5}$O compound.

$\gamma$-LiFeO$_2$ phase

Figure 8 illustrates the MS of $\gamma \text{-LiFeO}_2$ phase from $T=4.2$ K up to 312 K. In the same figure, we plotted the distributions of the effective hyperfine magnetic field, estimated with the Le Caer–Dubois method. We found that the Le Caer–Dubois method is the best strategy to fit the spectra, yielding effective magnetic field distributions across the full range of temperatures. In this code [37], the absorption line positions are calculated from the first-order perturbation approximation, $v_i=\gamma _i H+\delta +\beta _i u$ (see “$\alpha$-LiFeO$_2$” section for the meaning of symbols). Since this code does not fit the isomer shift $\delta$, the quadrupole shift parameter u, and the HWHM parameter $\Gamma /2$, we estimated these parameters by a trial and error procedure to minimize the $\chi ^2$. We used a single value for the quadrupole shift parameter for all temperatures, $u=-\,0.115$ mm/s. If we take into account that the principal axis of the EFG tensor is parallel to the tetragonal crystallographic c-axis ($\theta =0$) and the asymmetry parameter is $\eta =0$ (due to the tetragonal symmetry), we can estimate the quadrupole coupling constant $e^2q_{zz}Q/2=2u=-\,0.24$ mm/s. The value of the quadrupole coupling constant estimated in the present study agrees very well with the one reported in the earlier study of Cox et al. [4]. Because e and Q are positive constants, the $V_{zz}$ is a negative number. For reference, we quote the hyperfine parameters used to estimate the distribution of the effective magnetic field at $T=4.2$ K, $\Gamma /2=0.15$ mm/s (fixed), $u=-\,0.115$ mm/s (deduced by trial and error), $\delta =0.546$ mm/s (deduced by trial and error). From the estimated magnetic field distribution, a mean value of $\langle H\rangle =525$ kOe with standard deviation 8 kOe was calculated. The isomer shift, the u, and $\langle H\rangle$ values are in good agreement with those found by Tabuchi et al. [18], at $T=4.2$ K in a $\gamma \text{-LiFeO}_2$ sample prepared hydrothermally.

Figure 8

(left panels) Mössbauer spectra of $\gamma \text{-LiFeO}_2$ with estimated effective magnetic field distributions (right panels) using the Le Caer–Dubois method at several temperatures.

The estimated isomer shift is plotted as a function of temperature in the lower panel of Fig. 9. Utilizing the same procedure as in the case of $\alpha \text{-LiFeO}_2$, we deduce a Debye temperature of $\Theta _D=300\pm 50$ K for the $\gamma \text{-LiFeO}_2$ sample. An important question arises: What is the physical explanation for a distribution of hyperfine magnetic fields in the MS? Ideally, only one sextet should exist, as only one crystallographic site within that particular crystal structure exists. A reasonable answer to this question could be based on the fact that a small percentage of iron occupies the Li site and vice versa. According to the results of the Rietveld analysis, this proportion amounts to a maximum of $2\%$. However, in our spectra, the proportion of the second sextet is approximately $16\%$, which is significantly greater than the Rietveld estimate. This leads us to speculate that the distribution may arise from iron at the boundaries of coherent Li:Fe ordered regions. The size of the coherently diffracting domains, deduced from the selective broadening of Bragg peaks that describe the Li/Fe ordering in the $\gamma$-phase, was estimated to be around 54 nm, or equivalently, about 62 cells. Since each cell contains four iron ions, the total count of Fe ions, denoted as $N_\text{Fe}$, would be $62\times 4=248$ Fe ions. If we also assume that the boundaries of the scattering regions align with a specific number of unit cells, for instance, 5 cells, then the disordered iron ions would amount to $5\times 4=20$, which corresponds to $20/248=8\%$ of the total. Thus, it is reasonable to suggest that a significant second component may arise from iron ions located at the boundaries of the coherently scattering regions, also known as antiphase boundaries. As the temperature rises, the overall splitting of the MS diminishes. This implies that the most probable value of H declines monotonically, as depicted in the upper panel of Fig. 9. Moreover, the distribution of hyperfine magnetic fields widens with increasing temperature. Around $T=300$ K, this distribution becomes quite broad, reaching its peak at the most probable value and extending to zero field. These findings reveal a pronounced disordering of the magnetic state.

Figure 9

Mössbauer and magnetic data of $\gamma \text{-LiFeO}_2$ compound. (upper panel) Local maxima of the effective hyperfine magnetic field distributions versus temperature estimated from Mössbauer spectra. The solid line represents the p(H) distribution at $T=295$ K. (middle panel) Temperature variation of the dc-magnetic susceptibility, $\chi _{\text{dc}}(T)$, and $d\chi _{\text{dc}}(T)/dT$. The measurements are collected in ZFC and FC modes under a dc magnetic field of 5kOe. (lower panel) Temperature variation of experimental and theoretical calculated isomer shift using calculated from second-order Doppler shift. The curves represent $\delta (T)$ curves for $\Theta _D=250,300,$ and 350 K.

The magnetic dc-susceptibility of this sample, measured under a DC-magnetic field of $H=5$ kOe using both ZFC and FC protocols, (see middle panel of Fig. 9) exhibits slight hysteresis between the ZFC and FC branches within the temperature range of 4.2–260 K. However, it does not display a clear signature of a transition from the antiferromagnetic to the paramagnetic state. Only when plotting the first-order derivative ($d\chi _\text{dc}/dT$, as shown in the middle panel of Fig. 9) can one identify an anomaly at approximately $T\approx 320$ K. The corresponding MS at $T=312$ K can be fitted with a highly broad distribution of the hyperfine field, featuring two peaks: one at around $\approx 310$ kOe and a second close to zero field at $\approx 25$ kOe (refer to Fig. 8, right column).

Nominally $\beta '\text{-LiFeO}_2$ sample

The MS of the nominally $\beta '\text{-LiFeO}_2$ sample, measured at temperatures of 4.2 K, 40 K, 80 K, 150 K, 200 K, and 295 K (see SI, Fig. S4). According to the results of the Rietveld analysis for this specific sample, the MS is expected to contain contributions from the $\beta '\text{-LiFeO}_2$, $\gamma \text{-LiFeO}_2$, and $\alpha \text{-LiFeO}_2$ phases. Since in $\gamma$ phase iron occupies predominately a single crystallographic site, the MS at $T=4.2$ K should manifest as a single sextet with a distribution of hyperfine fields H (as detailed in “$\gamma$-LiFeO$_2$ phase” section). Given that this phase comprises nanoregions, its MS spectrum is expected to be more complex. In the monoclinic $\beta '$-phase, iron and lithium ions are distributed into four distinct sites ($4e_i$, where $i=1,2,3$, and 4). Among these, $4e_1$ and $4e_3$ are predominantly occupied by iron, while the other two are occupied by lithium. It is reasonable expectation that the MS at $T=4.2$ K should be comprised two sextets. A cursory examination of lines 1 and 6 in the MS at $T=4.2$ K reveals structure within these lines. Drawing from the findings of “$\gamma$-LiFeO$_2$ phase” section, the outer regions of lines 1 and 6 can be accurately reproduced by a sextet featuring hyperfine parameters close to those of the $\gamma$ phase. The remaining spectral area can be mainly associated with the $\beta '$ phase.

An initial attempt to fit the MS at 4.2 K using three sextets, each with an asymmetric distribution of hyperfine fields, disclosed that a minimum of four sextets are necessary to replicate the experimental spectra. A broadened fourth sextet can be attributed to both the $\gamma$ phase and the $\alpha$ phase. Employing this model, we consistently simulated the 4.2 K, 40 K, and 80 K Mössbauer spectra. The hyperfine parameters used to reproduce this sextet are: $\delta _1=0.56$ mm/s, $H_1=520$ kOe, $u_1=-\,0.11$ mm/s, $\sigma _{l,1}=10$ kOe, $\sigma _{h,1}=5$ kOe and $A_1=23\%$. This sextet depicted in blue in Fig. S4 (see SI), can be attributed to the $\gamma$ phase. The hyperfine parameters of the sextets related with the $\beta '$ phase are: $\delta _2=0.43$ mm/s, $H_2=517$ kOe, $u_2=-\,0.04$ mm/s, $\sigma _{l,2}=23$ kOe, $\sigma _{h,2}=11$ kOe, and $A_2=25\%$ and $\delta _3=0.51$ mm/s, $H_3=473$ kOe, $u_3=-\,0.15$ mm/s, $\sigma _{l,3}=9$ kOe, $\sigma _{h,3}=16$ kOe and $A_3=26\%$ (sextets colored with magenta and brown colors, respectively in Fig S4 (see SI)). The hyperfine parameters of the fourth sextet related to the $\gamma$ (predominantly) and $\alpha$ phase are: $\delta _4=0.51$ mm/s, $H_4=482$ kOe, $u_4=0.05$ mm/s, $\sigma _{l,4}=8$ kOe, $\sigma _{h,4}=9$ kOe, and $A_4=26\%$ (shown with green color in Fig. S4 (see SI)). At 140 K, the MS consists of a magnetic sextet characterized by a very broad distribution of H, accompanied by a central unresolved region. At 200 K, the magnetic sextets are still present, albeit with a smaller spectral area in comparison to the 140 K spectrum. The central unresolved spectral region may be attributed to either paramagnetic/fast-relaxing entities or magnetically split sextets with small magnetic fields. At ambient temperature, the MS is composed of an unresolved spectral area resulting from the paramagnetic doublets of the $\alpha$ phase, $\beta '$ and $\gamma$ nanoregions. The temperature dependence of the dc-magnetic moment, (see Supplementary information Fig. S5), exhibits significant hysteresis between the ZFC and FC branches, indicating the presence of nanoregions (superparamagnetic behavior) within the sample. The peak observed at 150 K is reasonably attributed to the magnetic transition of the $\beta '$ phase. The convergence of the ZFC and FC branches observed around 250 K can be attributed to the superparamagnetic behavior of the $\gamma$ phase nanoregions.

Conclusions

We conducted a study on the crystal structure of the LiFeO$_2$ compound in its three polymorphic forms using powder XRD data and the local magnetic properties through MS and magnetization studies. The primary finding of our investigation on the disordered cubic $\alpha$-LiFeO$_2$ phase is that the MS exhibit broad spectral lines in both the paramagnetic and antiferromagnetic states. This indicates a distribution of the hyperfine parameters. The paramagnetic spectra can be accurately reproduced with a two-peak distribution of the $\Delta E_Q$ parameter, which we estimated using the Le Caer–Dubois method. For the magnetically split MS, we employed a model involving two sextets, convoluted with temperature-dependent asymmetric and temperature-independent symmetric distributions of the effective magnetic field $p_i(H)$ ($i=1,2$) and the quadrupole parameter $p_i(u)$, respectively. The broad, temperature-dependent distributions $p_i(H)$ calculated from MS indicate a wide distribution of the Néel temperature or spin glass behavior. This behavior is inherent to the disorder and the short-range ordering present in this mixed crystal.

The $\gamma$-LiFeO$_2$ phase was prepared through prolonged annealing of the $\alpha$-LiFeO$_2$ below the order–disorder transition temperature. Despite the extended annealing, Rietveld analysis indicates a selective broadening of the reflections with $\ell = 2n+1$. This broadening likely originates from antiphase domains. These defects significantly influence the magnetically split MS from low temperatures up to the Néel temperature. We calculated the effective hyperfine magnetic field distributions (p(H)) using the Le Caer–Dubois method. The temperature dependence of these distributions, particularly below the magnetic transition temperature, is linked to a broad distribution of the Néel temperature due to the presence of the antiphase defects. The hyperfine parameters extracted from the MS indicate that iron predominantly exists in a high spin ferric state.

Efforts to synthesize the $\beta '$-LiFeO$_2$ phase as a single-phase sample proved unsuccessful. Rietveld analysis of the XRD data of these samples revealed that they consist of nanoregions containing both $\beta '$-LiFeO$_2$ and $\gamma$-LiFeO$_2$ phases. According to the MS and magnetic measurements, the $\beta '$-LiFeO$_2$ phase exhibits a magnetic transition at 150 K.

Methods and experimental details

$\alpha$-LiFeO$_2$ polycrystalline samples are prepared by solid-state reaction of stoichiometric amounts of Li$_2$CO$_3$ and Fe$_2$O$_3$ powders ($99.99\%$ and $99.9\%$ purity, respectively). Initially, the Li$_2$CO$_3$ and Fe$_2$O$_3$ powders are thoroughly mixed in an agate mortar and pestle. Subsequently, the mixed powders are pressed (180 kg/cm$^2$) with a cylindrical die. The cylindrical pastilles were slowly heated to the reaction temperature in an alumina crucible. The reaction temperature is selected in the interval 800–950 °C. The grinding, pastille formation, and reaction process were repeated twice. Finally, the samples were either cooled slowly to ambient temperature or quenched from the reaction temperature into water. The $\gamma$-LiFeO$_2$ polycrystalline sample was prepared by annealing a $\alpha$-LiFeO$_2$ sample for 30 days, at 600°C. We attempted to prepare single phase $\beta ' \text{-LiFeO}_2$ by prolonged annealing an $\alpha \text{-LiFeO}_2$ in the temperature interval 250–500 °C, but without success (see below).

The magnetic ac-susceptibility, dc-magnetization, and specific heat measurements were carried out with a Physical Property Measurement System (PPMS, Quantum Design) using the ACMS and specific heat options and with a SQUID magnetometer (Quantum Design MPMS 5.5). Absorption Mössbauer spectra (MS) were measured using a conventional constant acceleration spectrometer with a $^{57}$Co(Rh) source oscillating at room temperature. The spectrometer was calibrated with a thin $\alpha$-Fe foil at ambient conditions. Isomer shifts are referred to $\alpha$-Fe at 295 K. The powder X-ray diffraction patterns of the polycrystalline samples were measured in the Bragg–Brentano geometry using Siemens/Bruker D5000 and smartlab Rigaku diffractometers, both operated at 40 kV and 35 mA, with CuK$_\alpha$ radiation ($\lambda _1 = 1.54184$Å). The powder XRD patterns were analyzed with the Rietveld method using the FULLPROF program suite [34, 48]. Thermogravimetric measurements were performed with a Perkin-Elmer (Pyris Diamond TG/TDA) apparatus.