Skip to main content
SpringerLink
Log in

Fundamental Solution of the Multi-Dimensional Time Fractional Telegraph Equation

  • Research Paper
  • Published:
Fractional Calculus and Applied Analysis Aims and scope Submit manuscript

Abstract

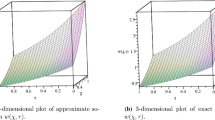
In this paper we study the fundamental solution (FS) of the multidimensional time-fractional telegraph equation where the time-fractional derivatives of orders α ∈]0,1] and β ∈]1,2] are in the Caputo sense. Using the Fourier transform we obtain an integral representation of the FS in the Fourier domain expressed in terms of a multivariate Mittag-Leffler function. The Fourier inversion leads to a double Mellin-Barnes type integral representation and consequently to a H-function of two variables. An explicit series representation of the FS, depending on the parity of the dimension, is also obtained. As an application, we study a telegraph process with Brownian time. Finally, we present some moments of integer order of the FS, and some plots of the FS for some particular values of the dimension and of the fractional parameters α and β.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. M. Abramowitz, I.A. Stegun, Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables. 10th printing. National Bureau of Standards, Wiley-Interscience Publication, John Wiley & Sons, New York etc. (1972).

    MATH  Google Scholar 

  2. R.G. Buschman, H-functions of two variables, III. Pure Appl. Math. Sci. 9 (1978), 13–18.

    MathSciNet  MATH  Google Scholar 

  3. R.G. Buschman, H-functions of two variables, I. Indian J. Math. 20 (1978), 132–153.

    MATH  Google Scholar 

  4. R.F. Camargo, A.O. Chiacchio, E.C. de Oliveira, Differentiation to fractional orders and the fractional telegraph equation. J. Math. Phys. 49, No 3 (2008), Article ID 033505, 12p; 10.1063/1.2890375.

    Google Scholar 

  5. R.C. Cascaval, E.C. Eckstein, L.C. Frota, J.A. Goldstein, Fractional telegraph equations. J. Math. Anal. Appl. 276, No 1 (2002), 145–159; 10.1016/S0022-247X(02)00394-3.

    Article  MathSciNet  Google Scholar 

  6. J. Chen, F. Liu, V. Anh, Analytical solution for the time-fractional telegraph equation by the method of separating variables. J. Math. Anal. Appl. 338, No 2 (2008), 1364–1377; 10.1016/j.jmaa.2007.06.023.

    Article  MathSciNet  Google Scholar 

  7. A. Erdélyi, W. Magnus, F. Oberhettinger, G. Tricomi, Tables of Integral Transforms, Vol. II. Bateman Manuscript Project, California Institute of Technology, McGraw-Hill Book Company, New York-Toronto-London (1954).

    MATH  Google Scholar 

  8. M. Ferreira, N. Vieira, Fundamental solutions of the time fractional diffusion-wave and parabolic Dirac operators. J. Math. Anal. Appl. 447, No 1 (2017), 329–353; 10.1016/j.jmaa.2016.08.052.

    Article  MathSciNet  Google Scholar 

  9. R. Gorenflo, A.A. Kilbas, F. Mainardi, S. Rogosin, Mittag-Leffler Functions. Theory and Applications. Springer Monographs in Mathematics, Springer, Berlin (2014).

    MATH  Google Scholar 

  10. H.J. Haubold, A.M. Mathai, R.K. Saxena, Mittag-Leffler functions and their applications. J. Appl. Math. (2011), Article ID 298628, 51p; 10.1155/2011/298628.

    Google Scholar 

  11. A. Kilbas, M. Saigo, H-transforms. Theory and Applications. Analytical Methods and Special Functions, Vol. 9, Chapman & Hall/CRC, Boca Raton, FL (2004).

    Book  Google Scholar 

  12. A. Kilbas, H.M. Srivastava, J.J. Trujillo, Theory and Applications of Fractional Differential Equations. North-Holland Mathematics Studies, Vol. 204, Elsevier, Amsterdam (2006).

    MATH  Google Scholar 

  13. Y. Luchko, R. Gorenflo, An operational method for solving fractional differential equations with the Caputo derivatives. Acta Math. Vietnam. 24, No 2 (1999), 207–233.

    MathSciNet  MATH  Google Scholar 

  14. J. Lundgren, Convergence acceleration of alternating series (https://www.mathworks.com/matlabcentral/fileexchange/25200-altsum). MATLAB Central File Exchange, 2011; Access: 12/09/2016.

    Google Scholar 

  15. M.O. Mamchuev, Solutions of the main boundary value problems for the time-fractional telegraph equation by the Green function method. Fract. Calc. Appl. Anal. 20, No 1, (2017), 190–211; 10.1515/fca-2017-0010; https://www.degruyter.com/view/j/fca.2017.20.issue-1/issue-files/fca.2017.20.issue-1.xml.

    Article  MathSciNet  Google Scholar 

  16. E. Orsingher, L. Beghin, Time-fractional telegraph equations and telegraph processes with Brownian time. Probab. Theory Relat. Fields 128, No 1 (2004), 141–160; 10.1007/s00440-003-0309-8.

    Article  MathSciNet  Google Scholar 

  17. I. Podlubny, Fractional Differential Equations. An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of their Solution and some of their Applications. Mathematics in Science and Engineering 198, Academic Press, San Diego (1999).

    MATH  Google Scholar 

  18. S.G. Samko, A.A. Kilbas, O.I. Marichev, Fractional Integrals and Derivatives: Theory and Applications. Gordon and Breach, New York, NY (1993).

  19. S. Yakubovich, M.M. Rodrigues, Fundamental solutions of the fractional two-parameter telegraph equation. Integral Transforms Spec. Funct. 23, No 7 (2012), 509–519, 10.1080/10652469.2011.608488.

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Technology and Management Polytechnic, Institute of Leiria, P-2411-901, Leiria, Portugal

    Milton Ferreira

  2. Center for Research and Development in Mathematics and Applications (CIDMA), Department of Mathematics, University of Aveiro, Campus Universitário de Santiago, 3810-193, Aveiro, Portugal

    Milton Ferreira, M. Manuela Rodrigues & Nelson Vieira

Authors
  1. Milton Ferreira
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. M. Manuela Rodrigues
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Nelson Vieira
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Milton Ferreira.

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ferreira, M., Rodrigues, M.M. & Vieira, N. Fundamental Solution of the Multi-Dimensional Time Fractional Telegraph Equation. FCAA 20, 868–894 (2017). https://doi.org/10.1515/fca-2017-0046

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1515/fca-2017-0046

MSC 2010

Key Words

Search

Navigation