1 Introduction

This paper is concerned with a \(2\times 2\) hyperbolic system of conservation laws with time-dependent damping

$$ \textstyle\begin{cases} u_{t}+ (\frac{u^{2}}{2} )_{x}=-\frac{\mu}{1+t} u, \\ v_{t}+(v u)_{x}=0, \end{cases} $$
(1.1)

where \(t\in R^{+}\) represents the time variable, \(x\in R\) stands for the space variable, \(u=u(x,t)\) is given smooth function, the sign of \(v=v(x,t)\) remains unchanged, and the external term \(-\frac{\mu}{1+t} u\) with physical parameter \(\mu >0\) is a time-gradually-degenerate damping [1, 2].

The first equation of system (1.1) with \(\mu =0\) is said to be the Burgers equation, which can be used to model various phenomena, such as shock waves in gas dynamics and hydrodynamics turbulence [3–6]. The homogeneous system (1.1) can model the evolution of density inhomogeneities in matter in the universe [7]. The Riemann solutions involving delta-shocks of the homogeneous case (1.1) were obtained using the vanishing viscosity approach [8, 9]. De la cruz [10] discussed the Riemann problem for the homogeneous system (1.1) with damping term \(-\alpha u\) (\(\alpha >0\)). De la cruz and Juajibioy [11] showed the existence of Riemann solutions involving delta-shock for a \(2\times 2\) system of conservation laws with linear damping by employing vanishing viscosity method. Beyond these, there are many studies about various nonlinear differential equations with damping. For example, Yang and Zhou [12] considered the degenerate fractional Kirchhoff wave equation with structural damping or strong damping and proved the well-posedness and the existence of global attractor in the natural energy space by the Faedo-Galerkin method and energy estimates. Di and Song [13] proved the global existence and non-global existence of the initial-boundary value problem for a quasilinear viscoelastic equation with strong damping and source terms. Yang, Ning, and Chen [14] proved the exponential stability of the nonlinear Schrödinger equation with locally distributed damping on a compact Riemannian manifold. As regards the delta-shocks, we refer the reader to papers [8, 15–30] for more details.

However, it is noticeable that the damping term is usually closely related to time. With this in mind, we first discuss the Riemann problem for (1.1) with initial data

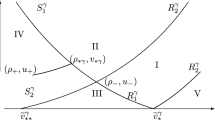
$$ (u,v)\vert _{t=0}=\bigl(u(x,0),v(x,0)\bigr)= \textstyle\begin{cases} (u_{-},v_{-})& x< 0, \\ (u_{+},v_{+})& x>0. \end{cases} $$
(1.2)

System (1.1) may be changed to a homogeneous conservative form through the transform \(u=\frac{w}{(1+t)^{\mu}}\). With the characteristic analysis, we obtain two kinds of solutions for the modified system (2.1). To be exact, the solution is made up of two contact discontinuities and vacuum provided that the initial data satisfy \(u_{-}< u_{+}\). The solution is in the form of a delta-shock when the initial data satisfy \(u_{-}>u_{+}\). By solving the generalized Rankine-Hugoniot relation under the over-compressive entropy condition, the position, strength, and propagation speed of the delta-shock are obtained. Then, we use the relation between variables u and w to construct two kinds of Riemann solutions for the original system (1.1) with initial data (1.2). Additionally, the generalized Rankine-Hugoniot relation is also verified. Under the impact of the time-dependent damping, the Riemann solutions are no longer self-similar. The contact discontinuities and delta-shock curves are monotone increasing (decreasing) and concave (convex).

Since the existence and uniqueness of the delta-shock solution are established, a natural question arises: is it stable? Taking a cue from the scalar conservation law with time-dependent viscosity

$$ U_{t}+\bigl(F(U)\bigr)_{x}= Q(t)U_{xx}, \quad Q(t)>0, $$

we introduce the time-dependent viscous perturbation

$$ \textstyle\begin{cases} u_{t}+ (\frac {u^{2}}{2} )_{x}= \varepsilon (\frac{1}{(1+t)^{\mu}} \int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds ) u_{xx}-\frac{\mu}{1+t} u, \\ v_{t}+(v u)_{x}=0, \end{cases} $$
(1.3)

to analyze the stability of the solutions involving delta-shock and vacuum to (1.1)-(1.2), where

$$ \int _{0}^{t}\frac{1}{(1+s)^{\mu}} \,ds=\textstyle\begin{cases} \ln (1+t),& \mu =1, \\ \frac{1}{1-\mu} (\frac{1}{(1+t)^{\mu -1}}-1 ),& \mu \neq 1. \end{cases} $$
(1.4)

The hyperbolic systems of conservation laws with time-dependent viscosity \(Q(t)=\varepsilon t\) were independently proposed by Tupciev [31] and Dafermos [32]. By applying this method, Tan, Zhang, and Zheng [21] initially studied the delta-shock for a nonstrictly hyperbolic system of conservation laws. Later, the method was used to study the delta-shock for various systems of conservation laws, see papers [20, 22, 33–37]. We refer to works [10, 11], for the nonhomogeneous triangular systems of conservation laws with nonlinear function \(Q(t)\).

As can easily be seen, although the solutions of (1.1)-(1.2) are non-self-similar, the solutions of (2.1)-(2.2) retain a similar structure if the initial data belong to a bounded total variation space. With the introduction of the transformation \(u=\frac{w}{(1+t)^{\mu}}\), (1.3) becomes the modified viscous system (4.1), which is a viscous approximation of the modified system (2.1). One can observe that if \((w,v)\) is the solution of the problem (4.1) and (2.2), then \((u,v)=(\frac{w}{(1+t)^{\mu}},v)\) is the solutions of the problem (1.3) with (1.2). Therefore, so long as we prove the stability of solutions to (2.1)-(2.2) under viscous perturbation, the stability of solutions of (1.1)-(1.2) to viscous perturbation can be obtained because ε is independent of t. We first establish the existence of the solutions depending on the similarity variable \(\xi =\frac{x}{\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds}\) for the modified viscous system (4.1) with (2.2). Then, we investigate the limits of the solutions to the system (4.1) with (2.2) as \(\varepsilon \rightarrow 0^{+}\). It is discovered that when \(u_{-}>u_{+}\), the limit of solutions to (4.1) with (2.2) is identical with the delta-shock solution of (2.1)-(2.2). The limit function of \(v^{\varepsilon}\) is a sum of a step function and a Dirac delta function. Apart from that, it is also shown that when \(u_{-}< u_{+}\), the vacuum solution of (2.1)-(2.2) is just the limit of the solution of the system (4.1) with (2.2) as \(\varepsilon \rightarrow 0^{+}\). So, the stability of solutions of (2.1)-(2.2) and (1.1)-(1.2) to viscous perturbation is proved.

This paper includes six parts. Section 2 discusses the Riemann problem for a modified homogeneous system. Section 3 constructs the Riemann solutions of (1.1)-(1.2). Section 4 proves the existence of solutions to the modified viscous system (4.1) with (2.2). Section 5 analyzes the limiting behavior of solutions of (4.1) and (2.2) as \(\varepsilon \rightarrow 0^{+}\). Section 6 gives the conclusion.

2 Riemann solutions for (2.1)

In this section, we construct the Riemann solutions to the modified homogeneous system (2.1) with the same Riemann initial data. For convenience, we assume that \(v\geq 0\) throughout the paper. Inserting \(u=\frac{w}{(1+t)^{\mu}}\) into (1.1), we obtain the following homogeneous conservative system, after some manipulation,

$$ \textstyle\begin{cases} w_{t}+ (\frac{w^{2}}{2(1+t)^{\mu}} )_{x}=0, \\ v_{t}+ (\frac{wv}{(1+t)^{\mu}} )_{x}=0. \end{cases} $$
(2.1)

Meanwhile, the initial data become

$$ \bigl(w(x,0),v(x,0)\bigr)= \textstyle\begin{cases} (u_{-},v_{-})& x< 0, \\ (u_{+},v_{+})& x>0. \end{cases} $$
(2.2)

The double eigenvalue of system (2.1) is \(\lambda =\frac{w}{(1+t)^{\mu}}\), and the corresponding right eigenvector is \(\overrightarrow{r}=(0,1)^{T}\) satisfying \(\nabla \lambda \cdot \overrightarrow{r}=0\). Consequently, (2.1) is nonstrictly hyperbolic, and λ is linearly degenerate. The linear degeneracy excludes the possibility of the solutions containing rarefaction and shock waves.

A bounded discontinuity at \(x=x(t)\) satisfies the Rankine-Hugoniot condition

$$ \textstyle\begin{cases} -\sigma (t)[w]+ [\frac{w^{2}}{2(1+t)^{\mu}} ]=0, \\ -\sigma (t)[v]+ [\frac{wv}{(1+t)^{\mu}} ]=0, \end{cases} $$
(2.3)

where \(\sigma (t)=x'(t)\), \([w]=w_{l}-w_{r}\) with \(w_{l}=w(x(t)-0,t)\), \(w_{r}=w(x(t)+0,t)\), in which \([w]\) denotes the jump of w across the discontinuity. Unlike the classical systems of conservation laws, the propagation speed of discontinuity is related to the time t.

Solving (2.3) yields the contact discontinuity

$$ J: \sigma (t)=\frac{w_{l}}{(1+t)^{\mu}}=\frac{w_{r}}{(1+t)^{\mu}}. $$
(2.4)

It stands to reason that if \(w_{l}=w_{r}\), the two states \((w_{l},v_{l})\) and \((w_{r},v_{r})\) can be connected using a contact discontinuity J.

We now construct the Riemann solutions by means of the constants, vacuum and contact discontinuity. When \(u_{-}< u_{+}\), the Riemann solution, which is composed of two contact discontinuities \(J_{1}\) and \(J_{2}\) with the vacuum state (denoted by Vac) between them, can be expressed as

$$ (w,v) (x,t)=\textstyle\begin{cases} (u_{-},v_{-}), & x< u_{-}\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds, \\ \text{Vac},& u_{-}\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds\leq x \leq u_{+}\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds, \\ (u_{+},v_{+}),& x>u_{+}\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds, \end{cases} $$
(2.5)

where the integral \(\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds\) is given explicitly by (1.4).

When \(u_{-}>u_{+}\), the singularity of solution must happen, which is caused by the superposition of linearly degenerate characteristics in some specific initial data. Motivated by [8, 20], the solution containing delta-shock should be kept in mind. For this purpose, the definitions of two-dimensional weighted delta function and delta-shock solution are introduced as follows.

Definition 2.1

A two-dimensional weighted delta function \(\eta (s)\delta _{S}\) supported on a smooth curve \(S=\{(x(s),t(s)): c\leq s\leq d\}\) is defined by

$$ \bigl\langle \eta (s)\delta _{S}, \phi (x,t) \bigr\rangle =\int _{c}^{d}\eta (s) \phi \bigl(x(s),t(s)\bigr) \,ds $$
(2.6)

for all test functions \(\phi \in C^{\infty}_{0}(R\times R^{+})\).

Definition 2.2

A pair \((w,v)\) is referred to as a delta-shock solution to (2.1) in the sense of distributions if there exists a smooth curve S and a weight \(\eta \in C^{1}(S)\) such that w and v are represented in the following form

$$ v(x,t)=v_{0}(x,t)+\eta (t)\delta _{S},\qquad w(x,t)=w_{0}(x,t),\qquad w(x,t)\vert _{S}=w_{\delta}(t), $$
(2.7)

where \(v_{0}(x,t)=v_{l}(x,t)-[v]H(x-x(t))\), \(w_{0}(x,t)=w_{l}(x,t)-[w]H(x-x(t))\), in which \((w_{l},v_{l})(x,t)\) and \((w_{r},v_{r})(x,t)\) are piecewise smooth solutions to (2.1), \(H(x)\) is the Heaviside function, which is equal to zero for \(x<0\) and to one for \(x>0\), and it satisfies

$$ \begin{gathered} \langle w,\phi _{t}\rangle + \biggl\langle \frac{w^{2}}{2(1+t)^{\mu}}, \phi _{x} \biggr\rangle =0,\qquad \langle v,\phi _{t}\rangle + \biggl\langle \frac{wv}{(1+t)^{\mu}}, \phi _{x} \biggr\rangle =0 \end{gathered} $$
(2.8)

for all test functions \(\phi \in C_{0}^{\infty}(R\times R^{+})\) in which

$$ \begin{gathered} \biggl\langle \frac{wv}{(1+t)^{\mu}}, \phi \biggr\rangle = \int _{0}^{+\infty} \int _{-\infty}^{+\infty} \frac{w_{0}v_{0}}{(1+t)^{\mu}}\phi \,dx \,dt+ \biggl\langle \frac{\eta (t)\omega _{\delta}(t)}{(1+t)^{\mu}}\delta _{S},\phi \biggr\rangle , \end{gathered} $$

and v has the similar integral identities as above.

In the light of these definitions, we seek a solution with the discontinuity \(x=x(t)\in C^{1}\) for system (2.1) of the form

$$ (w,v) (x,t)=\textstyle\begin{cases} (w_{l},v_{l})(x,t),& x< x(t), \\ ( w_{\delta}(t),\eta (t)\delta (x-x(t))),& x=x(t), \\ (w_{r},v_{r})(x,t),& x>x(t). \end{cases} $$
(2.9)

If a pair \((w,v)\) of the form (2.9) satisfies the relation

$$ \textstyle\begin{cases} \frac{dx(t)}{dt}=\sigma (t)=\frac{w_{\delta}(t)}{(1+t)^{\mu}}, \\ -[w]\sigma (t)+ [\frac{w^{2}}{2(1+t)^{\mu}} ]=0, \\ \frac {d\eta (t)}{dt}=-[ v]\sigma (t)+ [\frac{w v}{(1+t)^{\mu}} ], \end{cases} $$
(2.10)

then it is said to be a solution to system (2.1) in the sense of distributions. The proof is similar to that [20, 33], so we omit it.

System (2.10) reflecting the exact relationship among the limit states on two sides of the discontinuity, the weight, propagation speed and the location of the discontinuity is referred to as the generalized Rankine-Hugoniot relation.

Furthermore, to guarantee that such a discontinuity is unique, the delta entropy condition

$$ \lambda (w_{r},v_{r})< \frac{dx(t)}{dt}< \lambda (w_{l},v_{l}) $$
(2.11)

should be added. (2.11) tells us that all characteristics on both sides of the discontinuity are incoming.

A discontinuity of the form (2.9), satisfying (2.10) and (2.11), is known as a delta-shock of the system (2.1), symbolized by δ.

In the following, the generalized Rankine-Hugoniot relation will be applied to the case \(u_{-}>u_{+}\). At this time, the solution is a delta-shock in the following form

$$ (w,v) (x,t)=\textstyle\begin{cases} (u_{-},v_{-}),& x< x(t), \\ (w_{\delta}(t),\eta (t)\delta (x-x(t))),& x=x(t), \\ (u_{+},v_{+}),& x>x(t). \end{cases} $$
(2.12)

Under the delta entropy condition

$$ \frac{u_{+}}{(1+t)^{\mu}}< \frac{w_{\delta}(t)}{(1+t)^{\mu}}< \frac{u_{-}}{(1+t)^{\mu}}, $$
(2.13)

we solve the generalized Rankine-Hugoniot relation (2.10) with initial condition

$$ t=0: x(0)=0, \eta (0)=0 $$
(2.14)

to derive

$$ \textstyle\begin{cases} w_{\delta }=\frac{u_{-}+u_{+}}{2},\qquad \sigma (t)=\frac{u_{-}+u_{+}}{2(1+t)^{\mu}}, \\ x(t)=\frac{u_{-}+u_{+}}{2}\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds, \\ \eta (t)=\frac{ (u_{-}-u_{+})(v_{-}+v_{+})}{2}\int _{0}^{t} \frac{1}{(1+s)^{\mu}}\,ds, \end{cases} $$
(2.15)

where \(\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds\) is shown in (1.4).

3 Riemann solutions to (1.1)-(1.2)

In view of discussion in the above section, we construct the solutions of the original system (1.1) with initial data (1.2) in terms of \((u,v)(x,t)=(\frac{w}{(1+t)^{\mu}},v)(x,t)\).

When the initial data satisfy \(u_{-}< u_{+}\), the Riemann solution to (1.1) with (1.2) can be shown as

$$ (u,v) (x,t)=\textstyle\begin{cases} (\frac{u_{-}}{(1+t)^{\mu}},v_{-} ),& x< u_{-}\int _{0}^{t} \frac{1}{(1+s)^{\mu}}\,ds, \\ \text{Vac},& u_{-}\int _{0}^{t} \frac{1}{(1+s)^{\mu}}\,ds\leq x \leq u_{+}\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds, \\ (\frac{u_{+}}{(1+t)^{\mu}},v_{+} ),& x>u_{+}\int _{0}^{t} \frac{1}{(1+s)^{\mu}}\,ds. \end{cases} $$
(3.1)

In addition, we have

$$ \frac{d(u_{\pm}\int _{0}^{t} \frac{1}{(1+s)^{\mu}}\,ds)}{dt}=\frac{u_{\pm}}{(1+t)^{\mu}},\qquad \frac{d^{2}(u_{\pm}\int _{0}^{t} \frac{1}{(1+s)^{\mu}}\,ds)}{dt^{2}}=- \frac{\mu u_{\pm}}{(1+t)^{\mu +1}}. $$

According to the signs of \(u_{-}\) and \(u_{+}\), it can be concluded that the contact discontinuities curves are either monotone increasing and concave or monotone decreasing and convex.

Definition 3.1

If there exists a smooth curve S and a weight \(\eta \in C^{1}(S)\) such that u and v are represented in the following form

$$ v(x,t)=v_{0}(x,t)+\eta (t)\delta _{S},\qquad u(x,t)=u_{0}(x,t),\qquad u(x,t)\vert _{S}=u_{\delta}(t), $$
(3.2)

then a pair \((u,v)\) is called a delta-shock type solution to (1.1) in the sense of distributions, where \(v_{0}(x,t)=v_{l}(x,t)-[v]H(x-x(t))\), \(u_{0}(x,t)=u_{l}(x,t)-[u]H(x-x(t))\), in which \((u_{l},v_{l})(x,t)\) and \((u_{r},v_{r})(x,t)\) are piecewise smooth solutions to the system (1.1), and it satisfies

$$ \begin{gathered} \langle u,\phi _{t}\rangle +\biggl\langle \frac{u^{2}}{2}, \phi _{x} \biggr\rangle =\biggl\langle \frac{\mu}{1+t}u,\phi \biggr\rangle ,\qquad \langle v,\phi _{t}\rangle +\langle uv,\phi _{x}\rangle =0 \end{gathered} $$
(3.3)

for all \(\phi \in C_{0}^{\infty}( R\times R^{+})\), where \(\langle uv, \phi \rangle = \int _{0}^{+\infty}\int _{-\infty}^{+ \infty}u_{0}v_{0}\phi \,dx \,dt+\langle \eta (t)u_{\delta}(t)\delta _{S}, \phi \rangle \).

When the initial data satisfy \(u_{-}> u_{+}\), we look for the Riemann solution of (1.1)-(1.2) in the following form

$$ (u,v) (x,t)=\textstyle\begin{cases} (\frac{u_{-}}{(1+t)^{\mu}},v_{-} ),& x< x(t), \\ ( u_{\delta}(t),\eta (t)\delta (x-x(t))),& x=x(t), \\ (\frac{u_{+}}{(1+t)^{\mu}},v_{+} ),& x>x(t) \end{cases} $$
(3.4)

satisfying the generalized Rankine-Hugoniot relation

$$ \textstyle\begin{cases} \frac{dx(t)}{dt}=u_{\delta}(t), \\ -[u]u_{\delta}(t)+[\frac{u^{2}}{2}]=0, \\ \frac {d\eta (t)}{dt}=-[v]u_{\delta}(t)+[uv], \end{cases} $$
(3.5)

in which the jumps across the discontinuity are

$$ [u]=\frac{u_{-}}{(1+t)^{\mu}}-\frac{u_{+}}{(1+t)^{\mu}},\qquad [uv]=\frac{u_{-}v_{-}}{(1+t)^{\mu}}-\frac{u_{+}v_{+}}{(1+t)^{\mu}}. $$
(3.6)

Also, the over-compressive entropy condition

$$ \frac{u_{+}}{(1+t)^{\mu}}< u_{\delta}(t)< \frac{u_{-}}{(1+t)^{\mu}} $$
(3.7)

should be satisfied to ensure the uniqueness of the solution.

Considering (3.7), we solve the generalized Rankine-Hugoniot relation (3.5) taking into account the initial value (2.14) to obtain the \(x(t)\), \(u_{\delta}(t)\) and \(\eta (t)\).

Consequently, when \(u_{+}< u_{-}\), the solution is a delta-shock in the following form

$$ (u,v) (x,t)=\textstyle\begin{cases} (\frac{u_{-}}{(1+t)^{\mu}},v_{-} ),& x< \frac{(u_{-}+u_{+})}{2}\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds, \\ (\frac{(u_{-}+u_{+})}{2(1+t)^{\mu}},\eta (t)\delta (x-x(t)) ),& x=\frac{(u_{-}+u_{+})}{2}\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds, \\ (\frac{u_{+}}{(1+t)^{\mu}},v_{+} ),& x>\frac{(u_{-}+u_{+})}{2}\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds, \end{cases} $$
(3.8)

where \(\eta (t)\) is displayed in (2.15). Moreover, we have

$$ \frac{d(\frac{u_{-}+u_{+}}{2}\int _{0}^{t} \frac{1}{(1+s)^{\mu}}\,ds)}{dt}= \frac{u_{-}+u_{+}}{2(1+t)^{\mu}},\qquad \frac{d^{2}(\frac{u_{-}+u_{+}}{2}\int _{0}^{t} \frac{1}{(1+s)^{\mu}}\,ds)}{dt^{2}}=- \frac{\mu (u_{-}+u_{+})}{2(1+t)^{\mu +1}}, $$

which means that the delta-shock curve is either monotone increasing and concave or monotone decreasing and convex.

In the following context, we show that the delta-shock solution satisfies (1.1) in the sense of distributions. For any \(\phi \in C_{0}^{\infty}( R\times R^{+})\), by means of the integration by parts and Green’s formula, one can calculate

$$ \begin{aligned} &\langle u,\phi _{t}\rangle +\biggl\langle \frac{u^{2}}{2},\phi _{x} \biggr\rangle -\biggl\langle \frac{\mu u}{1+t},\phi \biggr\rangle \\ &\quad =\int ^{+\infty}_{0} \int ^{x(t)}_{-\infty} \frac{u_{-}}{(1+t)^{\mu}}\phi _{t} \,dx\,dt+\int ^{+\infty}_{0} \int _{x(t)}^{+ \infty} \frac{u_{+}}{(1+t)^{\mu}}\phi _{t} \,dx\,dt \\ &\qquad{} +\int ^{+\infty}_{0} \int ^{x(t)}_{-\infty} \frac{1}{2} \biggl( \frac{u_{-}}{(1+t)^{\mu}}\biggr)^{2}\phi _{x} \,dx \,dt+\int ^{+\infty}_{0} \int _{x(t)}^{+\infty} \frac{1}{2}\biggl( \frac{u_{+}}{(1+t)^{\mu}}\biggr)^{2} \phi _{x} \,dx\,dt \\ &\qquad{} -\int ^{+\infty}_{0} \int ^{x(t)}_{-\infty} \frac{\mu u_{-}}{(1+t)^{\mu +1}}\phi \,dx \,dt-\int ^{+\infty}_{0} \int _{x(t)}^{+\infty} \frac{\mu u_{+}}{(1+t)^{\mu +1}}\phi \,dx \,dt \\ &\quad =\int ^{+\infty}_{0} \int ^{x(t)}_{-\infty}\biggl( \frac{u_{-}}{(1+t)^{\mu}}\phi \biggr)_{t}\,dx\,dt+ \int ^{+\infty}_{0} \int _{x(t)}^{+\infty}\biggl(\frac{u_{+}}{(1+t)^{\mu}}\phi \biggr)_{t}\,dx\,dt \\ &\qquad{} +\int ^{+\infty}_{0} \int ^{x(t)}_{-\infty} \biggl(\frac{1}{2} \biggl( \frac{u_{-}}{(1+t)^{\mu}}\biggr)^{2}\phi \biggr)_{x} \,dx\,dt+ \int ^{+\infty}_{0} \int _{x(t)}^{+\infty} \biggl(\frac{1}{2} \biggl(\frac{u_{+}}{(1+t)^{\mu}}\biggr)^{2} \phi \biggr)_{x} \,dx\,dt \\ &\quad =\oint \biggl(-\frac{u_{-}}{(1+t)^{\mu}}\phi \,dx+\frac{1}{2} \biggl( \frac{u_{-}}{(1+t)^{\mu}}\biggr)^{2}\phi \,dt \biggr) \\ &\qquad{} + \oint \biggl(- \frac{u_{+}}{(1+t)^{\mu}}\phi \,dx+\frac{1}{2} \biggl( \frac{u_{+}}{(1+t)^{\mu}}\biggr)^{2}\phi \,dt \biggr) \\ &\quad =\int ^{+\infty}_{0} \biggl(-\frac{u_{-}}{(1+t)^{\mu}} \frac{dx}{dt}+\frac{u_{+}}{(1+t)^{\mu}} \frac{dx}{dt} +\frac{1}{2}\biggl(\frac{u_{-}}{(1+t)^{\mu}}\biggr)^{2}-\frac{1}{2}\biggl( \frac{u_{+}}{(1+t)^{\mu}}\biggr)^{2} \biggr) \phi \,dt \\ &\quad =0 \end{aligned} $$

and

$$ \begin{aligned} &\langle v,\phi _{t}\rangle +\langle v u, \phi _{x}\rangle \\ &\quad =\int ^{+\infty}_{0} \int ^{x(t)}_{-\infty}\biggl\{ (v_{-}\phi )_{t}+\biggl( \frac{v_{-}u_{-}}{(1+t)^{\mu}}\phi \biggr)_{x} \biggr\} \,dx\,dt \\ &\qquad{} +\int ^{+\infty}_{0} \int _{x(t)}^{+\infty}\biggl\{ (v_{+}\phi )_{t}+\biggl( \frac{v_{+}u_{+}}{(1+t)^{\mu}}\phi \biggr)_{x} \biggr\} \,dx\,dt +\int ^{+\infty}_{0} \eta (t)\,d\phi \\ &\quad =\oint \biggl\{ -v_{-}\phi \,dx+\frac{v_{-}u_{-}}{(1+t)^{\mu}}\phi \,dt \biggr\} +\oint \biggl\{ -v_{+}\phi \,dx + \frac{v_{+}u_{+}}{(1+t)^{\mu}}\phi \,dt \biggr\} -\int ^{+\infty}_{0} \phi \,d\eta (t) \\ &\quad =\int ^{+\infty}_{0} \biggl(-v_{-} \frac{dx}{dt}+ \frac{v_{-}u_{-}}{(1+t)^{\mu}} +v_{+}\frac{dx}{dt}- \frac{v_{+}u_{+}}{(1+t)^{\mu}}-\frac{d\eta (t)}{dt} \biggr)\phi \,dt \\ &\quad =0. \end{aligned} $$

4 Existence of solutions to the system (4.1) and (2.2)

This section shows the existence of solutions to the modified viscous system (4.1) and (2.2). Substituting \(u=\frac{w}{(1+t)^{\mu}}\) into the system (1.3) yields

$$ \textstyle\begin{cases} w_{t}+ (\frac {w^{2}}{2(1+t)^{\mu}} )_{x}= \varepsilon ( \frac {1}{(1+t)^{\mu}} \int _{0}^{t}\frac {1}{(1+s)^{\mu}}\,ds ) w_{xx}, \\ v_{t}+ (\frac {vw}{(1+t)^{\mu}} )_{x}=0. \end{cases} $$
(4.1)

Seeking the solutions depending on the variable \(\xi =\frac{x}{\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds}\), one acquires

$$ \textstyle\begin{cases} -\xi w_{\xi}+(\frac{w^{2}}{2})_{\xi}=\varepsilon w_{\xi \xi}, \\ -\xi v_{\xi}+(v w)_{\xi}=0 \end{cases} $$
(4.2)

and

$$ (w,v) (\pm \infty )=(u_{\pm},v_{\pm}). $$
(4.3)

Theorem 4.1

For every fixed \(\varepsilon >0\) and \(u_{-}\neq u_{+}\), there is a unique and monotonic smooth solution for the problem

$$ \textstyle\begin{cases} -\xi w_{\xi}+(\frac{w^{2}}{2})_{\xi}=\varepsilon w_{\xi \xi}, \\ w(\pm \infty )=u_{\pm}. \end{cases} $$
(4.4)

Proof

Motivated by [32], we deal with the altered problem

$$ \textstyle\begin{cases} -\xi w_{\xi}+\nu (\frac {w^{2}}{2})_{\xi}=\varepsilon w_{\xi \xi}, \\ w(\pm R)=u_{\pm}, \end{cases} $$
(4.5)

where \(\nu \in [0,1]\), R is a real number that is big enough. Integrating (4.5) over \((\xi _{0},\xi )\) yields

$$ \begin{gathered} w(\xi )-w(\xi _{0})=w'( \xi _{0})\int _{\xi _{0}}^{\xi } \operatorname{exp} \biggl(\int _{\xi _{0}}^{s}\frac{\nu w(r)-r}{\varepsilon}\,dr \biggr) \,ds. \end{gathered} $$
(4.6)

We assume that \(u_{-}>u_{+}\), the case \(u_{-}< u_{+}\) can be treated similarly. If \(\xi _{1}\) is a critical point of \(w(\xi )\) satisfying \(w'(\xi _{1})=0\), then we infer from (4.5) that \(w^{(n)}(\xi _{1})=0\) for \(n\geq 2\). Consequently, \(w(\xi )\) is constant in \([-R,R]\), which leads to a contradiction. It follows from (4.6) that the monotonicity of \(w(\xi )\) depends on \(w'(\xi _{0})\). If \(w'(\xi _{0})>0\), then we have \(w(\xi )>w(\xi _{0})\), which is impossible as \(u_{-}>u_{+}\). Hence, the solution of (4.5) is monotone decreasing. Considering \(w'(\xi )<0\), we estimate

$$ \bigl\Vert w(\xi ) \bigr\Vert _{L^{\infty}}=\sup_{\xi \in [-R,R]} \bigl\vert w(\xi ) \bigr\vert \leq \max \bigl\{ \vert u_{-} \vert , \vert u_{+} \vert \bigr\} , $$

which is independent of ν and R. By Theorem 3.1 in [32], the existence of a solution of (4.4) on \((-\infty ,+\infty )\) is obtained.

Now we plan to show the uniqueness of the solution. Assume that \(w_{1}(\xi )\) and \(w_{2}(\xi )\) are two smooth solutions of (4.4) and denote \(W(\xi )=w_{2}(\xi )-w_{1}(\xi )\). Then \(W(\xi )\) is a smooth solution of the boundary value problem

$$ \textstyle\begin{cases} -\xi W_{\xi}+(Wh)_{\xi}=\varepsilon W_{\xi \xi}, \\ W(\pm \infty )=0, \end{cases} $$
(4.7)

where \(h(\xi )=\frac{w_{2}(\xi )+w_{1}(\xi )}{2}\). Multiplying the first equation of (4.4) by \(\operatorname{exp}(\frac{\xi ^{2}}{2\varepsilon})\) gives

$$ \frac{d}{d\xi} \biggl(w'(\xi )\operatorname{exp} \biggl( \frac{\xi ^{2}}{2\varepsilon} \biggr) \biggr) =\frac{w(\xi )}{\varepsilon}w'( \xi )\operatorname{exp} \biggl( \frac{\xi ^{2}}{2\varepsilon} \biggr). $$

Integrating the above formula, we obtain

$$ \bigl\vert w'(\xi ) \bigr\vert \leq \bigl\vert w'(0) \bigr\vert \operatorname{exp} \biggl( \frac{2\max \{ \vert u_{-} \vert , \vert u_{+} \vert \} \vert \xi \vert -\xi ^{2}}{2\varepsilon} \biggr). $$

Hence, we deduce

$$ \bigl\vert W'(\xi ) \bigr\vert \leq \bigl( \bigl\vert w'_{1}(0) \bigr\vert + \bigl\vert w'_{2}(0) \bigr\vert \bigr)\operatorname{exp} \biggl( \frac{2\max \{ \vert u_{-} \vert , \vert u_{+} \vert \} \vert \xi \vert -\xi ^{2}}{2\varepsilon} \biggr). $$

This shows that \(W'(\xi )\) tends to zero when \(\vert \xi \vert \rightarrow \infty \) for any fixed \(\varepsilon >0\). As a result, we have \(\lim_{\xi \rightarrow \pm \infty}\xi W(\xi )=0\) if \(\lim_{\xi \rightarrow \pm \infty} W(\xi )=0\).

Suppose that \(W(\xi )\) is not a null function. Let α and β be two consecutive zeros of \(W(\xi )\) satisfying \(-\infty \leq \alpha <\beta \leq +\infty \). By means of the boundedness of \(h(\xi )\), we integrate (4.7) over \((\alpha ,\beta )\) to get

$$ \int _{\alpha}^{\beta }W(\xi )\,d\xi =\varepsilon \bigl(W'(\beta )-W'( \alpha )\bigr). $$

If \(W(\xi )>0\) for \(\xi \in (\alpha ,\beta )\), then we obtain \(W'(\beta )>W'(\alpha )\), which contradicts \(W'(\beta )\leq W'(\alpha )\). Similarly, if \(W(\xi )<0\), one can also derive a contradiction. Consequently, we arrive at \(W(\xi )=0\). □

Theorem 4.2

Assume that \(w(\xi )\) is the solution of (4.4), then the problem

$$ \textstyle\begin{cases} -\xi v_{\xi}+(vw)_{\xi}=0, \\ v(\pm \infty )=v_{\pm }\end{cases} $$
(4.8)

has a weak solution \(v\in L^{1}[-\infty ,\infty ]\)

$$ v(\xi )=\textstyle\begin{cases} v_{1}(\xi )=v_{-}\operatorname{exp} (\int _{-\infty}^{\xi} \frac{-w'(s)}{w(s)-s}\,ds ),& \xi < \xi _{\alpha}, \\ v_{2}(\xi )=v_{+}\operatorname{exp} (\int _{\xi}^{+\infty} \frac{w'(s)}{w(s)-s}\,ds ),& \xi >\xi _{\alpha}, \end{cases} $$
(4.9)

where \(\xi _{\alpha}\) satisfies \(\xi _{\alpha}=w(\xi _{\alpha})\).

Proof

Integrating (4.8) over \((-\infty ,\xi _{\alpha})\) and \((\xi _{\alpha},+\infty )\) yields (4.9). Furthermore, we have

$$ \lim_{\xi \rightarrow \xi _{\alpha}^{-}}v_{1}(\xi )=+\infty , \qquad \lim _{\xi \rightarrow \xi _{\alpha}^{+}}v_{2}(\xi )=+ \infty . $$

Next, we show that \(v(\xi )\in L^{1}[\xi _{1},\xi _{2}]\) for any interval \([\xi _{1},\xi _{2}]\) containing \(\xi _{\alpha}\). We integrate (4.8) over \([\xi _{1},\xi ]\) for \(\xi _{1}<\xi <\xi _{\alpha}\) to obtain

$$ v_{1}(\xi ) \bigl(w(\xi )-\xi \bigr)-v_{1}(\xi _{1}) \bigl(w(\xi _{1})-\xi _{1}\bigr)+ \int _{\xi _{1}}^{\xi}v_{1}(r)\,dr=0, $$
(4.10)

which may be written in the following form

$$ \textstyle\begin{cases} a(\xi )p'(\xi )+p(\xi )=A_{1}, \\ p(\xi _{1})=0 \end{cases} $$

by setting

$$ p(\xi )=\int _{\xi _{1}}^{\xi}v_{1}(r)\,dr,\qquad A_{1}=v_{1}( \xi ) \bigl(w(\xi _{1})-\xi _{1}\bigr),\qquad a(\xi )=w(\xi )-\xi . $$

Solving for \(p(\xi )\) from the above equation, we have

$$ p(\xi )=A_{1} \biggl\{ 1-\operatorname{exp} \biggl(-\int _{\xi _{1}}^{\xi} \frac{dr}{a(r)} \biggr) \biggr\} . $$

Noticing that \(a(\xi )>0\) and \(a(\xi )=O(\vert \xi -\xi _{\alpha}\vert )\) as \(\xi \rightarrow \xi _{\alpha}^{-}\), one deduces

$$ \lim_{\xi \rightarrow \xi _{\alpha}^{-}}\int _{\xi _{1}}^{ \xi}v_{1}(r) \,dr=A_{1}. $$
(4.11)

Therefore,

$$ \lim_{\xi \rightarrow \xi _{\alpha}^{-}}v_{1}(\xi ) \bigl(w(\xi )- \xi \bigr)=0. $$
(4.12)

Similarly, we have

$$ \lim_{\xi \rightarrow \xi _{\alpha}^{+}}\int _{\xi _{2}}^{ \xi}v_{2}(r)\,dr =v_{2}(\xi _{2}) \bigl(w(\xi _{2})-\xi _{2}\bigr) $$
(4.13)

and

$$ \lim_{\xi \rightarrow \xi _{\alpha}^{+}}v_{2}(\xi ) \bigl(w(\xi )- \xi \bigr)=0. $$
(4.14)

(4.11) and (4.13) show that \(v(\xi )\in L^{1}[\xi _{1},\xi _{2}]\).

We now show \(v(\xi )\) satisfying

$$ -\int _{\xi _{1}}^{\xi _{2}}\bigl(v w(\xi )-v\xi \bigr)\phi '\,d\xi +\int _{\xi _{1}}^{\xi _{2}}v\phi \,d\xi =0 $$
(4.15)

for every test function \(\phi \in C_{0}^{\infty}[\xi _{1},\xi _{2}]\). In fact, for \(\xi _{1}<\sigma _{1}<\xi _{\alpha}<\sigma _{2}<\xi _{2}\), we have

$$ \begin{aligned} I&=-\int _{\xi _{1}}^{\xi _{2}}\bigl(v w(\xi )-v\xi \bigr)\phi '\,d\xi +\int _{\xi _{1}}^{\xi _{2}}v\phi \,d\xi , \\ &= \biggl(\int _{\xi _{1}}^{\sigma _{1}}+\int _{\sigma _{1}}^{ \sigma _{2}}+ \int _{\sigma _{2}}^{\xi _{2}} \biggr) \bigl(v\phi -v\bigl( w( \xi )-\xi \bigr)\phi ' \bigr)\,d\xi \\ &=I_{1}+I_{2}+I_{3}. \end{aligned} $$
(4.16)

For the first term \(I_{1}\) and the third term \(I_{3}\), we have

$$\begin{aligned}& \begin{aligned} \vert I_{1} \vert &= \biggl\vert - \phi (\sigma _{1})v_{1}(\sigma _{1}) \bigl(w(\sigma _{1})- \sigma _{1}\bigr)+\int _{\xi _{1}}^{\sigma _{1}} \bigl(\bigl(v_{1}( \xi ) \bigl(w( \xi )-\xi \bigr)\bigr)'+v_{1}(\xi ) \bigr)\phi \,d\xi \biggr\vert \\ &= \bigl\vert \phi (\sigma _{1})v_{1}(\sigma _{1}) \bigl(w(\sigma _{1})- \sigma _{1} \bigr) \bigr\vert \rightarrow 0,\quad \text{as } \sigma _{1} \rightarrow \xi _{\alpha}^{-}, \end{aligned} \\& \vert I_{3} \vert = \bigl\vert \phi (\sigma _{2})v_{2}(\sigma _{2}) \bigl(w( \sigma _{2})- \sigma _{2}\bigr) \bigr\vert \rightarrow 0,\quad \text{as } \sigma _{2} \rightarrow \xi _{\alpha}^{+}. \end{aligned}$$

In virtue of \(v(\xi )\in L^{1}[\xi _{1},\xi _{2}]\), the second term \(I_{2}\) is estimated as follows

$$ \begin{aligned} \vert I_{2} \vert \leq \int _{\sigma _{1}}^{\sigma _{2}} \bigl\vert \phi -\bigl(w(\xi )- \xi \bigr)\phi ' \bigr\vert \vert v \vert \,d\xi \rightarrow 0,\quad \text{as } \sigma _{1} \rightarrow \xi _{\alpha}^{-}, \sigma _{2}\rightarrow \xi _{ \alpha}^{+}. \end{aligned} $$

By virtue of the fact that I is independent of \(\xi _{1}\) and \(\xi _{2}\), (4.15) holds. And so, \(v(\xi )\) is a weak solution of (4.8). This finishes the proof. □

5 Limit solutions of (4.1) and (2.2) as ε falls to zero

This section analyzes the limit of solutions of (4.1) and (2.2) as ε falls to zero. It is shown that the solutions of (2.1)-(2.2) are stable.

Case 1

\(u_{-}>u_{+}\).

Lemma 5.1

Let \(\xi _{\alpha}^{\varepsilon}\) be the unique point satisfying \(\xi _{\alpha}^{\varepsilon}=w^{\varepsilon}(\xi _{\alpha}^{ \varepsilon})\), \(\xi _{\alpha}=\lim_{\varepsilon \rightarrow {0^{+}}}\xi _{ \alpha}^{\varepsilon }\) (pass to a subsequence if necessary). Then, for any \(\beta >0\),

$$\begin{aligned}& \lim_{\varepsilon \rightarrow {0^{+}}}w_{\xi}^{\varepsilon}(\xi )=0, \quad \textit{for } \vert \xi -\xi _{\alpha} \vert \geq \beta ,\\& \lim_{\varepsilon \rightarrow {0^{+}}}w^{\varepsilon}(\xi )= \textstyle\begin{cases} u_{-},&\textit{for } \xi \leq \xi _{\alpha}-\beta , \\ u_{+},&\textit{for } \xi \geq \xi _{\alpha}+\beta \end{cases}\displaystyle \end{aligned}$$

uniformly in the above intervals.

Denote \((w^{\varepsilon},v^{\varepsilon})\) by \((w,v)\) when there is no confusion.

Proof

Take \(\xi _{3}=\xi _{\alpha}+\beta /2\), and let ε be so small that \(\xi _{\alpha}^{\varepsilon}<\xi _{3}-\beta /4\). Integrating the first equation of (4.2) twice over \((\xi _{3},\xi )\) provides

$$ \begin{aligned} w(\xi _{3})-w(\xi ) &=-w'( \xi _{3})\int _{\xi _{3}}^{\xi} \operatorname{exp} \biggl(\int _{\xi _{3}}^{r}\frac{w(s)-s}{\varepsilon}\,ds \biggr)\,dr \\ &\geq -w'(\xi _{3})\int ^{ \xi -\xi _{3}}_{0}\operatorname{exp} \biggl(\frac{2(u_{+}-\xi _{3})r-r^{2}}{2\varepsilon} \biggr)\,dr. \end{aligned} $$

Passing to the limit \(\xi \rightarrow +\infty \), we have

$$ \begin{aligned} u_{-}-u_{+} \geq -w'(\xi _{3})\int ^{+\infty}_{0}\operatorname{exp} \biggl( \frac{2(u_{+}-\xi _{3})r-r^{2}}{2\varepsilon} \biggr)\,dr \geq -w'( \xi _{3}) \varepsilon C_{1}, \end{aligned} $$

in which \(C_{1}\) is a positive constant independent of ε. Thus, we get

$$ \bigl\vert w'(\xi _{3}) \bigr\vert \leq \frac{u_{-}-u_{+}}{\varepsilon C_{1}} $$

and

$$ \bigl\vert w'(\xi ) \bigr\vert \leq \frac{u_{-}-u_{+}}{\varepsilon C_{1}}\operatorname{exp} \biggl(\int _{\xi _{3}}^{\xi} \frac{w(s)-s}{\varepsilon}\,ds \biggr). $$

Additionally, as

$$ w(s)-s=w(s)-w\bigl(\xi _{\alpha}^{\varepsilon}\bigr)+\xi _{\alpha}^{\varepsilon}-s=\bigl(w'( \nu )-1\bigr) \bigl(s-\xi _{\alpha}^{\varepsilon}\bigr)\leq -\beta /4,\quad \xi _{\alpha}^{\varepsilon}< \nu < s, $$

we have

$$ \bigl\vert w'(\xi ) \bigr\vert \leq \frac{u_{-}-u_{+}}{\varepsilon C_{1}}\operatorname{exp} \biggl(-\frac{\beta}{4\varepsilon} (\xi - \xi _{3}) \biggr), $$

which shows that

$$ \lim_{\varepsilon \rightarrow{0^{+}}}w_{\xi}^{\varepsilon}(\xi )=0, \quad \text{uniformly for } \xi \geq \xi _{\alpha}+\beta . $$

When \(\xi >\xi _{4}\geq \xi _{\alpha}+\beta \), we have

$$ \begin{aligned} w(\xi _{4})-w(\xi ) =-w'( \xi _{4})\int _{\xi _{4}}^{\xi} \operatorname{exp} \biggl(\int _{\xi _{4}}^{r}\frac{w(s)-s}{\varepsilon}\,ds \biggr)\,dr \end{aligned} $$

yielding

$$ \begin{aligned} \bigl\vert w(\xi )-w(\xi _{4}) \bigr\vert \leq \bigl\vert w'(\xi _{4}) \bigr\vert \int _{\xi _{4}}^{\xi} \operatorname{exp} \biggl(\int _{\xi _{4}}^{r}\frac{-\beta}{4\varepsilon}\,ds \biggr)\,dr =\frac{4\varepsilon}{\beta} \bigl\vert w'( \xi _{4}) \bigr\vert \biggl\{ 1-\operatorname{exp} \biggl(\frac{\beta}{4\varepsilon}(\xi _{4}- \xi ) \biggr) \biggr\} . \end{aligned} $$

If we pass to the limit \(\xi \rightarrow +\infty \), we conclude

$$ \bigl\vert u_{+}-w(\xi _{4}) \bigr\vert \leq \frac{4\varepsilon}{\beta} \bigl\vert w'(\xi _{4}) \bigr\vert , $$

which shows that

$$ \lim_{\varepsilon \rightarrow{0^{+}}}w^{\varepsilon}(\xi )=u_{+}, \quad \text{uniformly for } \xi \geq \xi _{\alpha}+\beta . $$

By a similar reasoning, we can obtain the result for \(\xi \leq \xi _{\alpha}-\beta \). The Lemma 5.1 is proved. □

Lemma 5.2

For any \(\beta >0\),

$$ \lim_{\varepsilon \rightarrow {0^{+}}}v^{\varepsilon}(\xi )= \textstyle\begin{cases} v_{-},&\textit{for } \xi < \xi _{\alpha}-\beta , \\ v_{+},&\textit{for } \xi >\xi _{\alpha}+\beta \end{cases} $$

uniformly.

Proof

Take \(\varepsilon _{0}>0\) so small that \(\vert \xi _{\alpha}^{\varepsilon}-\xi _{\alpha}\vert <\beta /2\) whenever \(0<\varepsilon \leq \varepsilon _{0}\). For any \(\xi _{1}\leq \xi _{\alpha}-\beta \) and \(\varepsilon \leq \varepsilon _{0}\), we have \(\xi _{1}\leq \xi _{\alpha}^{\varepsilon}-\beta /2\) and

$$ v(\xi _{1})=v_{-}\operatorname{exp} \biggl( \int _{-\infty}^{\xi _{1}}\frac{-w'(s)}{w(s)-s}\,ds \biggr). $$

For any \(s\in (-\infty ,\xi _{1}]\), we have

$$ w(s)-s=w(s)-w\bigl(\xi _{\alpha}^{\varepsilon}\bigr)+\xi _{\alpha}^{\varepsilon}-s=\bigl(w'( \varsigma )-1\bigr) \bigl(s-\xi _{\alpha}^{\varepsilon}\bigr)\geq \beta /2,\quad s< \varsigma < \xi _{\alpha}^{\varepsilon}. $$

So, we arrive at

$$ 1\leq \operatorname{exp} \biggl( \int _{-\infty}^{\xi _{1}}\frac{-w'(s)}{w(s)-s}\,ds \biggr)\leq \operatorname{exp} \biggl(\frac{2}{\beta} \bigl(u_{-}-w( \xi _{1})\bigr) \biggr)\rightarrow 1 \quad \text{as } \varepsilon \rightarrow 0^{+}, $$

where we have used the Lemma 5.1. As a result, we get

$$ \lim_{\varepsilon \rightarrow 0^{+}}v^{\varepsilon}(\xi )=v_{-}, \quad \text{uniformly for } \xi < \xi _{\alpha}-\beta . $$

The other half can be derived analogously. This completes the proof. □

In the following, we analyze the limiting behavior of \(v^{\varepsilon}\) in the neighborhood of \(\xi =\xi _{\alpha}\) as \(\varepsilon \rightarrow 0^{+}\). Setting

$$ \sigma =\xi _{\alpha}=\lim_{\varepsilon \rightarrow{0^{+}}} \xi _{\alpha}^{\varepsilon }=\lim_{\varepsilon \rightarrow{0^{+}}}w^{ \varepsilon} \bigl(\xi _{\alpha}^{\varepsilon}\bigr)=w(\sigma )=w_{\delta}, $$
(5.1)

we have

$$ u_{+}< \sigma < u_{-}. $$
(5.2)

Take \(\psi \in C_{0}^{\infty}[\xi _{1},\xi _{2}]\) (\(\xi _{1}<\sigma < \xi _{2}\)) such that \(\psi (\xi )\equiv \psi (\sigma )\) for ξ in a neighborhood Ω of \(\xi =\sigma \) (ψ is called a sloping test function). When \(0<\varepsilon <\varepsilon _{0}\), \(\xi _{\alpha}^{\varepsilon}\in \Omega \subset (\xi _{1},\xi _{2})\). It follows from (4.2) that

$$ -\int _{\xi _{1}}^{\xi _{2}} \biggl(\frac{(w^{\varepsilon})^{2}}{2}- w^{\varepsilon }\xi \biggr)\psi '\,d\xi + \int _{\xi _{1}}^{\xi _{2}}w^{\varepsilon}\psi \,d\xi = \varepsilon \int _{\xi _{1}}^{\xi _{2}}w^{\varepsilon}\psi '' d \xi $$
(5.3)

and

$$ -\int _{\xi _{1}}^{\xi _{2}}\bigl(v^{\varepsilon }w^{\varepsilon}-v^{ \varepsilon} \xi \bigr)\psi '\,d\xi +\int _{\xi _{1}}^{\xi _{2}}v^{ \varepsilon}\psi \,d\xi =0. $$
(5.4)

Using (5.4) for \(\alpha _{1},\alpha _{2}\in \Omega \) s.t. \(\alpha _{1}<\sigma <\alpha _{2}\), we calculate

$$ \begin{aligned} \int _{\xi _{1}}^{\xi _{2}}\bigl(v^{\varepsilon }w^{\varepsilon}-v^{ \varepsilon} \xi \bigr)\psi '\,d\xi =\int _{\xi _{1}}^{\alpha _{1}}\bigl(v^{ \varepsilon }w^{\varepsilon}-v^{\varepsilon} \xi \bigr)\psi '\,d\xi +\int _{\alpha _{2}}^{\xi _{2}}\bigl(v^{\varepsilon }w^{\varepsilon}-v^{ \varepsilon} \xi \bigr)\psi '\,d\xi . \end{aligned} $$

Using Lemmas 5.1-5.2, we have

$$ \begin{aligned} &\lim_{\varepsilon \rightarrow{0^{+}}}\int _{\xi _{1}}^{ \xi _{2}} \bigl(v^{\varepsilon }w^{\varepsilon}-v^{\varepsilon} \xi \bigr)\psi 'd \xi \\ &\quad =\int _{\xi _{1}}^{\alpha _{1}}(v_{-}u_{-}-v_{-} \xi )\psi '\,d\xi + \int _{\alpha _{2}}^{\xi _{2}}(v_{+} u_{+}-v_{+}\xi )\psi '\,d\xi \\ &\quad =(v_{-}u_{-}-v_{-}\alpha _{1}-v_{+}u_{+}+v_{+}\alpha _{2})\psi ( \sigma ) +\int _{\xi _{1}}^{\alpha _{1}}v_{-}\psi (\xi ) \,d \xi + \int _{\alpha _{2}}^{\xi _{2}}v_{+}\psi (\xi )\,d\xi . \end{aligned} $$

Passing to the limit \(\alpha _{1}\rightarrow \sigma ^{-}\) and \(\alpha _{2}\rightarrow \sigma ^{+}\) in the above formula, we find

$$ \begin{aligned} \lim_{\varepsilon \rightarrow{0^{+}}}\int _{\xi _{1}}^{ \xi _{2}} \bigl(v^{\varepsilon }w^{\varepsilon}-v^{\varepsilon} \xi \bigr)\psi 'd \xi ={}&\bigl(-\sigma (v_{-}-v_{+})+(v_{-}u_{-}-v_{+}u_{+}) \bigr)\psi (\sigma ) \\ &{}+ \int _{\xi _{1}}^{\xi _{2}}H(\xi -\sigma )\psi (\xi )\,d\xi , \end{aligned} $$

where \(H(x)\) is a step function: \(H(x)=v_{-}\) for \(x<0\) and \(H(x)=v_{+}\) for \(x>0\). Using (5.4), we arrive at

$$ \lim_{\varepsilon \rightarrow{0^{+}}}\int _{\xi _{1}}^{ \xi _{2}}\bigl(v^{\varepsilon}-H(\xi - \sigma )\bigr) \psi (\xi ) \,d\xi =\bigl(- \sigma (v_{-}-v_{+})+(v_{-}u_{-}-v_{+}u_{+}) \bigr)\psi (\sigma ) $$
(5.5)

for all sloping test functions \(\psi \in C_{0}^{\infty}[\xi _{1},\xi _{2}]\). By the approximation process, (5.5) holds for all \(\psi \in C_{0}^{\infty}[\xi _{1},\xi _{2}]\).

Analogously, from (5.3), we can conclude that

$$ -\sigma (u_{-}-u_{+})+ \biggl(\frac{u_{-}^{2}}{2}- \frac{u_{+}^{2}}{2} \biggr)=0 $$
(5.6)

in the limit \(\varepsilon \rightarrow{0^{+}}\). It follows from (5.6) that \(\sigma =w_{\delta}=\frac{u_{-}+u_{+}}{2}\). Consequently, \(v^{\varepsilon}\) converges to a sum of a step function and a weighted Dirac delta function with the weight \(\frac{(u_{-}-u_{+})(v_{-}+v_{+})}{2} \) in the weak star topology of \(C_{0}^{\infty}(R)\).

It is important to note that the strength \(\frac{(v_{-}+v_{+})(u_{-}-u_{+})}{2}\) differs from the \(\eta (t)\) in (2.15). The reason is caused by the introduction of the similarity variable \(\xi =\frac{x}{\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds}\).

Case 2

\(u_{-}< u_{+}\).

Lemma 5.3

For any \(\beta >0\),

$$\begin{aligned}& \lim_{\varepsilon \rightarrow 0^{+}}w^{\varepsilon}_{\xi}( \xi )=0,\quad \textit{for } \xi \leq u_{-}-\beta \textit{ or } \xi \geq u_{+}+ \beta , \\& \lim_{\varepsilon \rightarrow 0^{+}}\bigl(w^{\varepsilon},v^{ \varepsilon}\bigr) ( \xi )=\textstyle\begin{cases} (u_{-},v_{-}),& \xi < u_{-}-\beta , \\ ( \xi ,0),& u_{-}-\beta \leq \xi \leq u_{+}+\beta , \\ (u_{+},v_{+}),& \xi >u_{+}+\beta , \end{cases}\displaystyle \end{aligned}$$
(5.7)

uniformly in the above intervals.

Proof

Take \(\xi _{3}=u_{+}+\beta \). Integrating the first equation of (4.2) twice over \([\xi _{3},\xi ]\) gives

$$ \begin{aligned} w(\xi )-w(\xi _{3}) &=w'( \xi _{3})\int _{\xi _{3}}^{\xi} \operatorname{exp} \biggl(\int _{\xi _{3}}^{r}\frac{w(s)-s}{\varepsilon}\,ds \biggr)\,dr \\ &\geq w'(\xi _{3})\int ^{\xi - \xi _{3}}_{0}\operatorname{exp} \frac{1}{2\varepsilon}\bigl(2(u_{-}-\xi _{3})r-r^{2} \bigr)\,dr. \end{aligned} $$

We obtain

$$ \begin{aligned} u_{+}-u_{-} \geq w'(\xi _{3})\int ^{+\infty}_{0}\operatorname{exp} \frac{1}{2\varepsilon}\bigl(2(u_{-}-\xi _{3})r-r^{2} \bigr)\,dr \geq w'(\xi _{3}) \varepsilon C_{2} \end{aligned} $$

in going to the limit \(\xi \rightarrow +\infty \), which provides

$$ \bigl\vert w'(\xi _{3}) \bigr\vert \leq \frac{u_{+}-u_{-}}{\varepsilon C_{2}}, $$

where \(C_{2}\) is a constant independent of ε. So, we have

$$ \bigl\vert w'(\xi ) \bigr\vert \leq \frac{u_{+}-u_{-}}{\varepsilon C_{2}}\operatorname{exp} \biggl(\int _{\xi _{3}}^{\xi} \frac{w(s)-s}{\varepsilon}\,ds \biggr). $$

As \(w(s)-s\leq u_{+}-\xi _{3}<-\beta /2 \), we get

$$ \begin{aligned} \bigl\vert w'(\xi ) \bigr\vert \leq \frac{u_{+}-u_{-}}{\varepsilon C_{2}}\operatorname{exp} \biggl(\frac{-\beta}{2\varepsilon} (\xi -\xi _{3}) \biggr) \end{aligned} $$

giving

$$ \lim_{\varepsilon \rightarrow{0^{+}}}w_{\xi}^{\varepsilon}(\xi )=0, \quad \text{uniformly for } \xi \geq u_{+}+\beta . $$

We now take \(\xi _{4}\) satisfying \(\xi >\xi _{4}\geq u_{+}+\beta \). Then, one has

$$ \begin{aligned} \bigl\vert w(\xi )-w(\xi _{4}) \bigr\vert &= \bigl\vert w'(\xi _{4}) \bigr\vert \int _{\xi _{4}}^{\xi} \operatorname{exp} \biggl(\int _{\xi _{4}}^{r}\frac{w(s)-s}{\varepsilon}\,ds \biggr)\,dr \\ &\leq \bigl\vert w'(\xi _{4}) \bigr\vert \int _{ \xi _{4}}^{\xi}\operatorname{exp} \biggl(\int _{\xi _{4}}^{r}\frac{-\beta}{2\varepsilon}\,ds \biggr)\,dr \\ &=\frac{2\varepsilon}{\beta} \bigl\vert w'(\xi _{4}) \bigr\vert \biggl\{ 1-\operatorname{exp} \biggl( \frac{\beta}{2\varepsilon}(\xi _{4}-\xi ) \biggr) \biggr\} . \end{aligned} $$

If we pass to the limit \(\xi \rightarrow +\infty \), we obtain

$$ \bigl\vert u_{+}-w(\xi _{4}) \bigr\vert \leq \frac{2\varepsilon}{\beta} \bigl\vert w'(\xi _{4}) \bigr\vert , $$

which means that

$$ \lim_{\varepsilon \rightarrow{0^{+}}}w^{\varepsilon}(\xi )=u_{+}, \quad \text{uniformly for } \xi \geq u_{+}+\beta . $$

Furthermore, for \(\xi >\xi _{3}\), we have

$$ v_{+}\geq v(\xi )=v_{+}\operatorname{exp} \biggl( \int ^{+\infty}_{\xi} \frac{w'(s)}{w(s)-s}\,ds \biggr)\geq \frac{v_{+}(u_{+}-\xi )}{w(\xi )-\xi}\rightarrow v_{+},\quad \text{as } \varepsilon \rightarrow 0^{+}, $$

which indicates that

$$ \lim_{\varepsilon \rightarrow{0^{+}}}v^{\varepsilon}(\xi )=v_{+}, \quad \text{uniformly for } \xi \geq u_{+}+\beta . $$

In a similar manner, the conclusion for \(\xi \leq u_{-}-\beta \) can be obtained.

Now, we are in a position to analyze the limit solutions on \([u_{-}-\beta ,u_{+}+\beta ]\). Set \(G(\xi )=w(\xi )-\xi \). Suppose that \(\xi _{1}\) and \(\xi _{2}\) satisfying \(-\infty < \xi _{1}<\xi _{2}< +\infty \) are two consecutive zeros of \(G(\xi )\). Integrating (4.2) over \((\xi _{1},\xi _{2})\) yields \(\varepsilon \ln \frac{w'(\xi _{2})}{w'(\xi _{1})}=\int _{\xi _{1}}^{ \xi _{2}}G(\xi )\,d\xi \). If \(G(\xi )>0\) on \((\xi _{1},\xi _{2})\), then we obtain \(w'(\xi _{2})> w'(\xi _{1})\), which contradicts \(w'(\xi _{2})-1\leq 0\) and \(w'(\xi _{1})-1\geq 0\). Similarly, if \(G(\xi )<0\), one can also deduce a contradiction. Consequently, \(G(\xi )\) possesses a unique zero point. Let \(\xi _{\alpha}^{\varepsilon}\) be the unique point satisfying \(w(\xi _{\alpha}^{\varepsilon})=\xi _{\alpha}^{\varepsilon}\in [u_{-}- \beta ,u_{+}+\beta ]\). Then, from (4.2), we have \(w''(\xi )>0\) for \(\xi \in (-\infty ,\xi _{\alpha}^{\varepsilon})\), while \(w''(\xi )<0\) for \(\xi \in (\xi _{\alpha}^{\varepsilon},+\infty )\). Consequently, we obtain

$$ G'(\xi )=w'(\xi )-1\leq w'\bigl(\xi _{\alpha}^{\varepsilon}\bigr)-1\leq 0 $$

giving

$$ w(u_{+}+\beta )-(u_{+}+\beta )\leq w(\xi )-\xi \leq w(u_{-}-\beta )-(u_{-}- \beta ). $$

If we pass to the limit \(\varepsilon \rightarrow 0^{+}\), we arrive at \(-\beta \leq \lim_{\varepsilon \rightarrow{0^{+}}}(w(\xi )- \xi )\leq \beta \). Since β is arbitrary, one deduces \(\lim_{\varepsilon \rightarrow{0^{+}}}(w(\xi )-\xi )=0\) yielding

$$ \lim_{\varepsilon \rightarrow {0^{+}}}v^{\varepsilon}(\xi )=0, \quad \text{uniformly for } u_{-}-\beta \leq \xi \leq u_{+}+\beta . $$

In consequence, a vacuum solution is obtained.

Consequently, we draw a conclusion. □

Theorem 5.4

Let \((w^{\varepsilon},v^{\varepsilon})(\xi )\) be the solution of (4.2)-(4.3) and \(u_{-}< u_{+}\). Then,

$$ \lim_{\varepsilon \rightarrow {0^{+}}}\bigl(w^{\varepsilon},v^{ \varepsilon}\bigr) ( \xi )= \textstyle\begin{cases} (u_{-},v_{-}),&\xi < u_{-}, \\ (\xi ,0),&u_{-}\leq \xi \leq u_{+}, \\ (u_{+},v_{+}),&\xi >u_{+}. \end{cases} $$

Theorem 5.4 shows that the vacuum Riemann solution to (2.1)-(2.2) is stable under viscous perturbation.

According to the above discussions, we have the following conclusion.

Theorem 5.5

Assume that \((w^{\varepsilon},v^{\varepsilon})(x,t)\) is the solution depending on the variable \(\xi =\frac{x}{\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds}\) of (4.1) with (2.2). Then, the limit, \(\lim_{\varepsilon \rightarrow 0^{+}}(w^{\varepsilon},v^{ \varepsilon})(x,t)=(w,v)(x,t)\) exists in the sense of distributions, and \((w,v)(x,t)\) solves (2.1) with (2.2). The \((w,v)(x,t)\) can be shown explicitly as

$$ (w,v) (x,t)=\textstyle\begin{cases} (u_{-},v_{-}),&x< \frac{u_{-}+u_{+}}{2} \int _{0}^{t} \frac{1}{(1+s)^{\mu}}\,ds, \\ (\frac{u_{-}+u_{+}}{2}, ( \frac{(u_{-}-u_{+})(v_{-}+v_{+})}{2}\int _{0}^{t} \frac{1}{(1+s)^{\mu}}\,ds )\delta (x-x(t)) ),& x= \frac{u_{-}+u_{+}}{2} \int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds, \\ (u_{+},v_{+}),& x>\frac{u_{-}+u_{+}}{2} \int _{0}^{t} \frac{1}{(1+s)^{\mu}}\,ds, \end{cases} $$

when \(u_{-}>u_{+}\), and

$$ (w,v) (x,t)=\textstyle\begin{cases} (u_{-},v_{-}),&x< u_{-} \int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds, \\ (\frac{x}{\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds},0 ),& u_{-} \int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds\leq x\leq u_{+} \int _{0}^{t} \frac{1}{(1+s)^{\mu}}\,ds, \\ (u_{+},v_{+}),& x>u_{+} \int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds \end{cases} $$

when \(u_{-}< u_{+}\).

Remark 5.6

From Theorem 5.5, one can observe that the limits of solutions depending on \(\xi =\frac{x}{\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds}\) of (4.1) and (2.2) are just the Riemann solutions of (2.1) with (2.2) as \(\varepsilon \rightarrow 0^{+}\). Besides, it is not hard to see that if \((w^{\varepsilon},v^{\varepsilon})\) solves the problem (4.1) and (2.2), then \((u^{\varepsilon},v^{\varepsilon})=( \frac{w^{\varepsilon}}{(1+t)^{\mu}},v^{\varepsilon})\) solves the problem (1.3) and (1.2). Note that ε is independent of t, then the limit, \(\lim_{\varepsilon \rightarrow 0^{+}}(u^{\varepsilon},v^{ \varepsilon})(x,t) =(\frac{w}{(1+t)^{\mu}},v)(x,t)=(u,v)(x,t)\), exists in the sense of distributions. \((u,v)(x,t)\) solves (1.1)-(1.2) and can be expressed by

$$ (u,v) (x,t)=\textstyle\begin{cases} (\frac{u_{-}}{(1+t)^{\mu}},v_{-}),&x< \frac{u_{-}+u_{+}}{2} \int _{0}^{t} \frac{1}{(1+s)^{\mu}}\,ds, \\ (\frac{u_{-}+u_{+}}{2(1+t)^{\mu}}, ( \frac{(u_{-}-u_{+})(v_{-}+v_{+})}{2}\int _{0}^{t} \frac{1}{(1+s)^{\mu}}\,ds )\delta (x-x(t)) ),& x= \frac{u_{-}+u_{+}}{2} \int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds, \\ (\frac{u_{+}}{(1+t)^{\mu}},v_{+}),& x>\frac{u_{-}+u_{+}}{2} \int _{0}^{t} \frac{1}{(1+s)^{\mu}}\,ds \end{cases} $$

for \(u_{-}>u_{+}\), and

$$ (u,v) (x,t)=\textstyle\begin{cases} (\frac{u_{-}}{(1+t)^{\mu}},v_{-}),&x< u_{-} \int _{0}^{t} \frac{1}{(1+s)^{\mu}}\,ds, \\ (\frac{x}{\int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds(1+t)^{\mu}},0 ),& u_{-} \int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds\leq x\leq u_{+} \int _{0}^{t}\frac{1}{(1+s)^{\mu}}\,ds, \\ (\frac{u_{+}}{(1+t)^{\mu}},v_{+}),& x>u_{+} \int _{0}^{t} \frac{1}{(1+s)^{\mu}}\,ds \end{cases} $$

for \(u_{-}< u_{+}\). Hence, the solutions of (1.1) with (1.2) to viscous perturbations are stable.

6 Conclusion

This paper solves the Riemann problem for a \(2\times 2\) hyperbolic system of conservation laws with a time-gradually-degenerate damping. Two kinds of solutions involving the delta-shocks and vacuum are constructed explicitly by means of the variable substitution method. Under the influence of the time-dependent damping, the Riemann solutions are non-self-similar. The contact discontinuities and delta-shock curves are monotone increasing (decreasing) and concave (convex). Moreover, with the vanishing viscosity method, the stability of the non-self-similar solutions containing delta-shock and vacuum is proved by introducing a time-dependent viscous system.