Approximation of G-variational inequality problems and fixed-point problems of G-κ-strictly pseudocontractive mappings by an intermixed method endowed with a graph

Abstract

In this paper, we first study G-κ-strictly pseudocontractive mappings and we establish a strong convergence theorem for finding the fixed points of two G-κ-strictly pseudocontractive mappings, two G-nonexpansive mappings, and two G-variational inequality problems in a Hilbert space endowed with a directed graph without the Property G. Moreover, we prove an interesting result involving the set of fixed points of a G-κ-strictly pseudocontractive and G-variational inequality problem and if Λ is a G-κ-strictly pseudocontractive mapping, then $I-\Lambda$ is a $G-\frac{(1-\kappa )}{2}$-inverse strongly monotone mapping, shown in Lemma 3.3. In support of our main result, some examples are also presented.

1 Introduction

Let H be a real Hilbert space. Let ζ be a nonempty, closed, and convex subset of H with inner product $\langle \cdot ,\cdot \rangle$ and norm $\|\cdot \|$, respectively, and let $\mathfrak{T}$ be a self-mapping of ζ. We use $F (\mathfrak{T})$ to denote the set of fixed points of $\mathfrak{T}$ (i.e., $F (\mathfrak{T} ) = \{x \in \zeta : \mathfrak{T}x = x\}$). The iterative schemes to approximate the fixed points of nonlinear mappings have a long history and have been studied intensively by many researchers [10, 11, 17, 23]. A mapping $\mathfrak{T}$ is called κ-strictly pseudocontractive if there exists a constant $\kappa \in [0,1)$ such that

$$\begin{aligned} \Vert \mathfrak{T}x-\mathfrak{T}y \Vert ^{2}\leq \Vert x-y \Vert ^{2}+\kappa \bigl\Vert (I- \mathfrak{T})x-(I- \mathfrak{T})y \bigr\Vert ^{2}, \quad\forall x,y\in \zeta . \end{aligned}$$

(1)

If $\kappa =1$, a mapping $\mathfrak{T}$ is called a pseudocontractive mapping.

Note that the class of κ-strictly pseudocontractive strictly includes the class of nonexpansive mappings that are self-mappings $\mathfrak{T}$ on ζ such that

$$\begin{aligned} \Vert \mathfrak{T}x-\mathfrak{T}y \Vert \leq \Vert x-y \Vert ,\quad\forall x,y\in \zeta . \end{aligned}$$

(2)

Strictly pseudocontractive was first introduced by Browder and Petryshyn [8] in 1967. It is well known that strictly pseudocontractive is more general than nonexpansive mappings and they have a wider range of applications. Therefore, it is important to develop the theory of iterative methods for strictly pseudocontractive. Many authors have proposed iterative algorithms and proved the strong convergence theorems for a nonexpansive mapping and a κ-strictly pseudocontractive mapping in Hilbert space to find their fixed points, see, for example, [1, 4, 10, 13, 15, 18, 29].

To prove the strong convergence of iterations determined by nonexpansive mapping, Moudafi [19] established a theorem for finding the fixed points of nonexpansive mappings. More precisely, he established the following result, known as the viscosity approximation method. To construct an iterative algorithm such that it converges strongly to the fixed points of a finite family of strictly pseudocontractive by using the concept of the viscosity approximation method, Yao et al. [31] proposed the intermixed algorithm for two strictly pseudocontractive mappings as follows:

Algorithm 1

For arbitrarily given $x_{0}\in \zeta$, $y_{0}\in \zeta$, let the sequences $\{x_{n}\}$ and $\{y_{n}\}$ be generated iteratively by

$$ \textstyle\begin{cases} x_{n+1} = (1-\beta _{n})x_{n}+\beta _{n}P_{\zeta}[\alpha _{n}f(y_{n})+(1-k- \alpha _{n})x_{n}+kTx_{n}],&n\geq 0, \\ y_{n+1} = (1-\beta _{n})y_{n}+\beta _{n}P_{\zeta}[\alpha _{n}g(x_{n})+(1-k- \alpha _{n})y_{n}+kSy_{n}],&n\geq 0, \end{cases} $$

(3)

where $\{\alpha _{n}\}$ and $\{\beta _{n}\}$ are two sequences of real numbers in (0,1), $T,S:\zeta \rightarrow \zeta$ are strictly λ-pseudocontractive mappings, $f:\zeta \rightarrow \mathcal{H}$ is a $\rho _{1}$-contraction, $g:\zeta \rightarrow \mathcal{H}$ is a $\rho _{2}$-contraction, and $k\in (0,1-\lambda )$ is a constant.

We now move onto some basics and definitions in graph theory. Let $G=(V(G),E(G))$ be a directed graph, where $V(G)$ is a set of vertices of a graph and E(G) is a set of its edges. We denote by $G^{-1}$ the directed graph obtained from G by reversing the direction of the edges. That is,

$$ E\bigl(G^{-1}\bigr)=\bigl\{ (x,y): (x,y)\in E(G)\bigr\} . $$

The following basic definitions of domination in graphs are needed to prove the main theorem. Let $G=(V(G),E(G))$ be a directed graph. A set $X\subseteq V(G)$ is called a dominating set if every $z\in V(G)\setminus X$ there exists $x\in X$ such that $(x,z)\in E(G)$ and we say that x dominates z or z is dominated by x. Let $z\in V$, a set $X\subseteq V$ is dominated by z if $(z,x)\in E(G)$ for any $x\in X$ and we say that X dominates z if $(x,z)\in E(G)$ for all $x\in X$. In this paper, we always assume that $E(G)$ contains all loops.

In 2008, by combining the notions in fixed-point theory and graph theory, Jachymski [12] generalized the Banach contraction principle in a complete metric space endowed with a directed graph. He also introduced a contractive-type mapping with a directed graph as follows:

Definition 1.1

Let $(X,d)$ be a metric space and $G=(V(G),E(G))$ be a directed graph, where $V(G)=X$ and $E(G)$ contains all loops, that is $\Delta \subseteq E(G)$. We say that a mapping $\mathbf{f}:X\rightarrow X$ is a Banach G-contraction if f preserves the edges of G, i.e.,

$$ \text{for any }x,y\in X\text{ such that }(x,y)\in E(G)\text{ implies }( \mathbf{f}x, \mathbf{f}y)\in E(G) $$

and there exists $k\in (0,1)$ such that

$$ d(\mathbf{f}x,\mathbf{f}y)\leq kd(x,y)\quad\text{for all }x,y\in X\text{with }(x,y) \in E(G). $$

Definition 1.2

Let ζ be a nonempty convex subset of a Banach space, $G=(V(G),E(G))$ be a directed graph such that $V(G)=\zeta$ and $\mathcal{T}:\zeta \rightarrow \zeta$, then $\mathcal{T}$ is said to be a G-nonexpansive mapping if the following conditions hold:

• (i) $\mathcal{T}$ is edge preserving, i.e., for any $x,y\in \zeta$ such that $(x,y)\in E(G)\Rightarrow (\mathcal{T} x,\mathcal{T} y)\in E(G)$;

• (ii) $\|\mathcal{T} x-\mathcal{T}y\|\leq \|x-y\|$, where $(x,y)\in E(G)$ for any $x,y\in \zeta$.

This mapping was introduced by Tiammee et al. [25].

The variational inequality problem (VIP) is to find a point $u\in C$ such that

$$ \langle Au,v-u\rangle \geq 0, $$

for all $v\in C$. The set of all solutions of the variational inequality is denoted by $VI(C,A)$. Historically, the variational inequality was introduced by Stampachhia [22]. Since then, variational inequalities have been used in various topics such as physic, optimization, and applied sciences, see, for example, [3, 20, 30].

Recently, in 2019, Kangtunyakarn [14] introduced G-variational inequality problems and G-α-inverse strongly monotone mappings as follows:

Definition 1.3

Let ζ be a nonempty, convex subset of a real Hilbert space H and let $G=(V(G),E(G))$ be a directed graph with $\zeta =V(G)$. The G-variational inequality problem is to find a point $x^{*}\in \zeta$ such that

$$\begin{aligned} \bigl\langle y-x^{*},Ax^{*}\bigr\rangle \geq 0, \end{aligned}$$

(4)

for all $y\in \zeta$ with $(x^{*},y)\in E(G)$ and $A:\zeta \rightarrow H$ is a mapping. The set of all solutions of (4) is denoted by $G-VI(\zeta ,A)$.

Definition 1.4

Let ζ be a nonempty, convex subset of a real Hilbert space H and let $G=(V(G),E(G))$ be a directed graph with $\zeta =V(G)$. The mapping $A:\zeta \rightarrow H$ is said to be G-α-inverse strongly monotone if there exists $\alpha >0$ such that

$$\begin{aligned} \langle Ax-Ay, x-y\rangle \geq \alpha \Vert Ax-Ay \Vert ^{2}, \end{aligned}$$

for all $x,y\in \zeta$ with $(x,y)\in E(G)$.

Let ζ be a nonempty, convex subset of a Banach space X and let $G=(V(G),E(G))$ be a directed graph such that $V(G)=\zeta$. Then, ζ is said to have Property G [25] if every sequence $\{a_{n}\}$ in ζ converges weakly to $x\in \zeta$, there exists a subsequence $\{a_{n_{k}}\}$ of $\{a_{n}\}$ such that $(a_{n_{k}},x)\in E(G)$ for all $k\in \mathbbm{ }\mathbb{N}$. During the course of this research, when investigating the literature on research methods, it was found that many researchers were using the Property G to prove the strong convergence theorems, see for example [14, 16, 26, 28].

In this paper, we will use some suitable conditions instead of the Property G.

Question 1

Can we prove a strong convergence theorem for finding the fixed points of two $G-\kappa$-strictly pseudocontractive mappings, two G-nonexpansive mappings, and two G-variational inequality problems in a Hilbert space endowed with a directed graph without the Property G?

By using the concept of (3), we introduce a new iterative method as follows:

Algorithm 2

Starting with $x_{1},y_{1}\in \zeta$, let the sequences $\{x_{n}\}$ and $\{y_{n}\}$ be defined by

$$ \textstyle\begin{cases} x_{n+1} = \delta _{n} x_{n}+\eta _{n} P_{\zeta}(I-\lambda _{1} \mathcal{S}_{1})x_{n}+\mu _{n}P_{\zeta}(\alpha _{n} f(y_{n})+(1- \alpha _{n})\mathcal{B}_{1} x_{n}), \\ y_{n+1} = \delta _{n} y_{n}+\eta _{n} P_{\zeta}(I-\lambda _{2} \mathcal{S}_{2})y_{n}+\mu _{n}P_{\zeta}(\alpha _{n} g(x_{n})+(1- \alpha _{n})\mathcal{B}_{2} y_{n}). \end{cases} $$

By putting $\mathcal{S}_{1}=\mathcal{S}_{2} = 0$, we obtain

$$ \textstyle\begin{cases} x_{n+1} = \delta _{n} x_{n}+\eta _{n}x_{n}+\mu _{n}P_{\zeta}(\alpha _{n}f(y_{n})+(1- \alpha _{n})\mathcal{B}_{1} x_{n}), \\ y_{n+1} = \delta _{n} y_{n}+\eta _{n}y_{n}+\mu _{n}P_{\zeta}(\alpha _{n}g(x_{n})+(1- \alpha _{n})\mathcal{B}_{2} y_{n}), \end{cases} $$

which is a modified version of [31].

To answer Question 1, we prove a strong convergence theorem for solving fixed-point problems in a Hilbert space endowed with a directed graph by using Algorithm 2, where $\mathcal{S}_{1},\mathcal{S}_{2}:\zeta \rightarrow H$ are the $G-\alpha$-inverse strongly monotone mappings, $f,g:H\rightarrow H$ are G-contraction mappings, and $\mathcal{B}_{1},\mathcal{B}_{2}:\zeta \rightarrow \zeta$ are G-nonexpansive mappings with some extra conditions in Theorem 3.2.

Inspired by the concept above, we introduce the definition of a $G-\kappa$-strictly pseudocontractive mapping that is different from κ-strictly pseudocontractive, see Example 2.5, and prove a strong convergence theorem for finding the fixed points of two G-κ-strictly pseudocontractive mappings, two G-nonexpansive mappings, and two G-variational inequality problems in a Hilbert space endowed with a directed graph without the Property G. Moreover, we prove an interesting Lemma involving the set of fixed points of a G-κ-strictly pseudocontractive and G-variational inequality problem and if Λ is a G-κ-strictly pseudocontractive mappings, then $I-\Lambda$ is a $G-\frac{(1-\kappa )}{2}$-inverse strongly monotone mapping, shown in Lemma 3.3. Finally, we give some examples for the main theorem.

2 Preliminaries

In this paper, we denote the weak convergence and the strong convergence by $^{\backprime \backprime}\rightharpoonup ^{\prime \prime}$ and $^{\backprime \backprime}\rightarrow ^{\prime \prime}$, respectively. For every $x\in H$, there exists a unique nearest point $P_{\zeta }x$ in ζ such that $\|x-P_{\zeta }x\|\leq \|x-y\|$ for all $y\in \zeta$. $P_{\zeta}$ is called the metric projection of H onto ζ. Furthermore, $P_{\zeta}$ is a firmly nonexpansive mapping of H onto ζ, i.e.,

$$ \Vert P_{\zeta }x-P_{\zeta }y \Vert ^{2}\leq \langle P_{\zeta }x-P_{\zeta }y,x-y \rangle ,\quad \forall x,y\in H. $$

In a real Hilbert space H, it is well known that H satisfies Opial’s condition [21], i.e., for any sequence $\{x_{n}\}\subset H$ with $x_{n}\rightharpoonup x$, the inequality

$$ \liminf_{n \to \infty} \Vert x_{n}-x \Vert < \liminf _{n \to \infty} \Vert x_{n}-y \Vert , $$

holds for every $y\in H$ with $y\neq x$.

The following Definitions and Lemmas are needed to prove the main theorem.

Lemma 2.1

([27])

Let $\{s_{n}\}$ be a sequence of nonnegative real numbers satisfying

$$ s_{n+1}\leq (1-\alpha _{n})s_{n}+\delta _{n}, \quad\forall n\geq 0, $$

where $\alpha _{n}$ is a sequence in $(0,1)$ and $\{\delta _{n}\}$ is a sequence such that

• (i) $\sum_{i=1}^{\infty} \alpha _{n} = \infty$;

• (ii) $\limsup_{n \to \infty}\frac{\delta _{n}}{\alpha _{n}} \leq 0$ or $\sum_{n=1}^{\infty}\lvert \delta _{n} \rvert < \infty$.

Then, $\lim_{n \to \infty}s_{n}=0$.

Lemma 2.2

([24])

For a given $z\in H$ and $u\in \zeta$,

$$ u=P_{\zeta }z\quad \Leftrightarrow\quad \langle u-z,v-u\rangle \geq 0,\quad \forall v \in \zeta . $$

Definition 2.3

([25])

Let $G=(V(G),E(G))$ be a directed graph. A graph G is called transitive if for any $x,y,z\in V(G)$ with $(x,y)$ and $(y,z)$ are in $E(G)$, then $(x,z)\in E(G)$.

Next, we introduce the definition of a $G-\kappa$-strictly pseudocontractive mapping.

Definition 2.4

Let ζ be a nonempty, closed, and convex subset of a real Hilbert space H and let $G=(V(G),E(G))$ be a directed graph with $\zeta =V(G)$ and $\Lambda :\zeta \rightarrow \zeta$. Then, Λ is said to be $G-\kappa$-strictly pseudocontractive if there exists a constant $\kappa \in [0,1)$ and the following conditions hold;

• (i) Λ is edge preserving, i.e., for any $x,y\in \zeta$ such that $(x,y)\in E(G)\Rightarrow (\Lambda x,\Lambda y)\in E(G)$;

• (ii) $\|\Lambda x-\Lambda y\|^{2}\leq \|x-y\|^{2}+\kappa \|(I-\Lambda )x-(I- \Lambda )y\|^{2}$, where $(x,y)\in E(G)$ for any $x,y\in \zeta$.

Example 2.5

Let $\mathbb{R}$ be the set of real numbers, $\zeta =[-10,10]$ with $G=(\zeta , E(G))$ and $E(G)=\{(x,y)\in \mathbb{R}\times \mathbb{R}: x,y\in [0,10]\}$. Define the mapping $\Lambda :\zeta \rightarrow \zeta$ by

$$ \Lambda x = \textstyle\begin{cases} \max(-x,x); &x\in (-10,10], \\ 0;&x=-10, \end{cases} $$

for all $x\in \zeta$ with

$$ \max(a,b) = \frac{1}{2}\bigl(a+b+ \vert a-b \vert \bigr). $$

Then, Λ is a G-$(\frac{1}{2})$-strictly pseudocontractive mapping, but Λ is not a $\frac{1}{2}$-strictly pseudocontractive mapping.

Solution. Let $x,y\in \zeta$ and $(x,y)\in E(G)$. Then, we have $x,y\in [0,10]$.

It follows that $\Lambda x,\Lambda y\in [0,10]$. Thus,

$$\begin{aligned} (\Lambda x,\Lambda y) \in E(G). \end{aligned}$$

From the definition of Λ, we have

$$\begin{aligned} \bigl\langle (I-\Lambda )x-(I-\Lambda )y, x-y\bigr\rangle &=\bigl\langle x- \max(-x,x)-y+\max(-y,y), x-y\bigr\rangle \\ &=\biggl\langle x-y-\frac{1}{2}\bigl(-x+x+ \vert -x-x \vert \bigr) \\ &\quad{}+\frac{1}{2}\bigl(-y+y+ \vert -y-y \vert \bigr), x-y\biggr\rangle \\ &=\bigl\langle x-y+\bigl( \vert y \vert - \vert x \vert \bigr),x-y\bigr\rangle \\ &=\bigl[x-y+\bigl( \vert y \vert - \vert x \vert \bigr)\bigr](x-y) \end{aligned}$$

(5)

and

$$\begin{aligned} \bigl\vert (I-\Lambda )x-(I-\Lambda )y \bigr\vert ^{2}&= \bigl\vert x- \max(-x,x)-y+\max(-y,y) \bigr\vert ^{2} \\ &= \biggl\vert x-\frac{1}{2} \bigl(-x+x+ \vert -x-x \vert \bigr)-y+ \frac{1}{2} \bigl(-y+y+ \vert -y-y \vert \bigr) \biggr\vert ^{2} \\ &= \bigl\vert (x-y)+\bigl( \vert y \vert - \vert x \vert \bigr) \bigr\vert ^{2} \\ &\leq \bigl\vert (x-y)+\bigl( \vert y \vert - \vert x \vert \bigr) \bigr\vert \cdot \bigl\{ \vert x-y \vert + \bigl\vert \bigl( \vert x \vert - \vert y \vert \bigr) \bigr\vert \bigr\} \\ &\leq \bigl\vert (x-y)+\bigl( \vert y \vert - \vert x \vert \bigr) \bigr\vert \cdot \bigl\{ \vert x-y \vert + \vert x-y \vert \bigr\} \\ &=2 \bigl\vert (x-y)+\bigl( \vert y \vert - \vert x \vert \bigr) \bigr\vert \cdot \vert x-y \vert . \end{aligned}$$

(6)

From (5) and (6), we have

$$\begin{aligned} \bigl\langle (I-\Lambda )x-(I-\Lambda )y, x-y\bigr\rangle &\geq \frac{1}{2} \bigl\vert (I- \Lambda )x-(I-\Lambda )y \bigr\vert ^{2} \\ &\geq \frac{1-\frac{1}{2}}{2} \bigl\vert (I-\Lambda )x-(I-\Lambda )y \bigr\vert ^{2}, \end{aligned}$$

(7)

for all $x,y\in \zeta$ with $(x,y)\in E(G)$.

From the definition of Λ and (7), we have

$$\begin{aligned} \vert \Lambda x-\Lambda y \vert ^{2}&= \bigl\vert (I-I+\Lambda )x-(I-I+\Lambda )y \bigr\vert ^{2} \\ &= \bigl\vert x-(I-\Lambda )x-y+(I-\Lambda )y \bigr\vert ^{2} \\ &= \bigl\vert (x-y)-\bigl[(I-\Lambda )x-(I-\Lambda )y\bigr] \bigr\vert ^{2} \\ &= \bigl\vert (x-y) \bigr\vert ^{2}-2\bigl\langle x-y, (I-\Lambda )x-(I-\Lambda )y\bigr\rangle +\bigl[(I- \Lambda )x-(I-\Lambda )y \bigr]^{2} \\ &\leq \bigl\vert (x-y) \bigr\vert ^{2}-2 \biggl[\frac{1-\frac{1}{2}}{2} \bigl\vert (I-\Lambda )x-(I- \Lambda )y \bigr\vert ^{2} \biggr]+ \bigl[(I-\Lambda )x-(I-\Lambda )y\bigr]^{2} \\ &= \bigl\vert (x-y) \bigr\vert ^{2}+\frac{1}{2}\bigl[(I- \Lambda )x-(I-\Lambda )y\bigr]^{2} . \end{aligned}$$

Hence, we obtain that Λ is a G-$(\frac{1}{2})$-strictly pseudocontractive mapping.

Next, we will show that Λ is not a $\frac{1}{2}$-strictly pseudocontractive mapping. Choose $x=-9$ and $y=-10$. Then,

$$ \bigl\lvert \Lambda (-9)-\Lambda (-10) \bigr\rvert ^{2}=\lvert 9-0 \rvert ^{2}=81>33= \lvert -9+10 \rvert ^{2}+\frac{1}{2} \bigl\lvert -9-\Lambda (-9)+10+ \Lambda (-10) \bigr\rvert ^{2}. $$

Hence, Λ is not a $\frac{1}{2}$-strictly pseudocontractive mapping.

Example 2.6

Let $H=\mathbb{R}$, $\zeta =[-10,10]$ with $G=(\zeta , E(G))$ and $E(G)=\{(x,y)|x\cdot y>0\}$. Define the mapping $\bar{\Lambda}:\zeta \rightarrow \zeta$ by $\bar{\Lambda}_{1}:[-10,10]\rightarrow [-10,10]$ defined by

$$ \bar{\Lambda}_{1}x=\operatorname{sgn}\bigl(\operatorname{sgn}(x) \bigr)= \textstyle\begin{cases} 1;&x\in (0,10], \\ 0;&x=0, \\ -1;&x\in [-10,0), \end{cases} $$

for all $x\in [-10,10]$ with

$$ \operatorname{sgn}(x)= \textstyle\begin{cases} -1;&x< 0, \\ 0;&x=0, \\ 1;&x>0. \end{cases} $$

Then, Λ is a G-$(\frac{1}{2})$-strictly pseudocontractive mapping, but Λ is not a $\frac{1}{2}$-strictly pseudocontractive mapping.

Solution. Let $x,y\in \zeta$ and $(x,y)\in E(G)$. Then, we have $x,y>0$.

Case I; $x,y>0$, we have $\bar{\Lambda}x=\bar{\Lambda}y=1$. Then, $\bar{\Lambda}x\cdot \bar{\Lambda}y>0$.

Case II; $x,y<0$, we have $\bar{\Lambda}x=\bar{\Lambda}y=-1$. Then, $\bar{\Lambda}x\cdot \bar{\Lambda}y>0$.

Thus, $(\bar{\Lambda}x,\bar{\Lambda}y)\in E(G)$.

From case I; $x,y>0$, then

$$\begin{aligned} \vert x-y \vert ^{2}+\frac{1}{2} \bigl\vert (I-\bar{ \Lambda})x-(I-\bar{\Lambda})y \bigr\vert ^{2}&= \vert x-y \vert ^{2}+ \frac{1}{2} \vert x-y-\bar{\Lambda}x+\bar{\Lambda}y \vert ^{2} \\ &=\frac{3}{2} \vert x-y \vert ^{2} \\ &\geq 0 \\ &= \vert \bar{\Lambda}x-\bar{\Lambda}y \vert ^{2}. \end{aligned}$$

From case II; $x,y<0$, by using the same technique as in Case I, we obtain that

$$\begin{aligned} \vert x-y \vert ^{2}+\frac{1}{2} \bigl\vert (I-\bar{ \Lambda})x-(I-\bar{\Lambda})y \bigr\vert ^{2}\geq \vert \bar{ \Lambda}x-\bar{\Lambda}y \vert ^{2}. \end{aligned}$$

Thus, Λ̄ is a G-$(\frac{1}{2})$-strictly pseudocontractive mapping.

Next, we will show that Λ̄ is not a $\frac{1}{2}$-strictly pseudocontractive mapping. Choose $x=1$ and $y=\frac{-1}{5}$. Then,

$$\begin{aligned} \vert \bar{\Lambda}x-\bar{\Lambda}y \vert ^{2}=4\geq 1.76&= \biggl\vert 1+\frac{1}{5} \biggr\vert ^{2}+\frac{1}{2} \biggl\vert 1-\bar{\Lambda}(1)+\frac{1}{5}+ \bar{\Lambda} \biggl(- \frac{1}{5} \biggr) \biggr\vert ^{2} . \end{aligned}$$

Hence, Λ̄ is not a $\frac{1}{2}$-strictly pseudocontractive mapping.

Lemma 2.7

([14])

Let ζ be a nonempty, closed, and convex subset of a real Hilbert space H and let $G=(V(G),E(G))$ be a directed graph with $\zeta =V(G)$. Let $E(G)$ be convex and G be transitive with $E(G)=E(G)^{-1}$ and let $A:\zeta \rightarrow H$ be a $G-\alpha$-inverse strongly monotone operator with $A^{-1}(0)\neq 0$. Then, $G-VI(\zeta ,A)=A^{-1}(0)=F(P_{\zeta}(I-\lambda A))$, for all $\lambda >0$.

Lemma 2.8

([14])

Let H be a Hilbert space and ζ be a nonempty, closed, and convex subset of H with ζ having a property G. Let $G=(V(G),E(G))$ be a directed graph where $V(G)=\zeta$ and $E(G)$ is a convex set. Let $A:\zeta \rightarrow H$ be a $G-\alpha$-inverse strongly monotone mapping with $F(P_{\zeta}(I-\lambda A))\times F(P_{\zeta}(I-\lambda A))\subseteq E(G)$, for all $\lambda \in (0,2\alpha )$. Then, $F(P_{\zeta}(I-\lambda A))$ is closed and convex.

Lemma 2.9

([25])

Let $\mathcal{X}$ be a normed space and ζ be a subset of $\mathcal{X}$ having a property G. Let $G=(V(G),E(G))$ be a directed graph such that $V(G)=\zeta$ and $E(G)$ is convex. Suppose $\mathcal{T}:\zeta \rightarrow \zeta$ is a G-nonexpansive mapping and $F(\mathcal{T})\times F(\mathcal{T})\subseteq E(G)$. Then, $F(\mathcal{T})$ is closed and convex.

Lemma 2.10

Let ζ be a nonempty, closed, and convex subset of a real Hilbert space H and let $G=(V(G),E(G))$ be a directed graph with $\zeta =V(G)$. Let $E(G)$ be convex and G be transitive with $E(G)=E(G)^{-1}$. Let $\mathcal{T}:\zeta \rightarrow \zeta$ be a G- nonexpansive mapping and $\Lambda :\zeta \rightarrow \zeta$ be a $G-\kappa$-strictly pseudocontractive mapping. Define a mapping $\mathcal{B}:\zeta \rightarrow \zeta$ by $\mathcal{B}x=\mathcal{T}((1-b)I+b\Lambda )x$ for all $x\in \zeta$ and $b\in (0,1-k)$. Then, $\mathcal{B}$ is a G-nonexpansive mapping, for all $x,y\in \zeta$ with $(x,y)\in E(G)$.

Proof

Let $x,y\in \zeta$ and $(x,y)\in E(G)$.

Since Λ is edge preserving and $(x,y)\in E(G)$, then $(\Lambda x,\Lambda y)\in E(G)$.

As $(x,y),(\Lambda x,\Lambda y) \in E(G)$ and $E(G)$ is convex, we obtain

$$ \bigl((1-b)x+b\Lambda x, (1-b)y+b\Lambda y\bigr)\in E(G). $$

Since $\mathcal{T}$ is edge preserving and $((1-b)x+b\Lambda x, (1-b)y+b\Lambda y)\in E(G)$, then

$$ \bigl(\mathcal{T}\bigl((1-b)x+b\Lambda x\bigr), \mathcal{T}\bigl((1-b)y+b\Lambda y\bigr)\bigr)\in E(G). $$

Thus, $\mathcal{B}$ is edge preserving.

We have

$$\begin{aligned} \Vert \mathcal{B}x-\mathcal{B}y \Vert ^{2}&= \bigl\Vert \mathcal{T}\bigl((1-b)x+b\Lambda x\bigr)- \mathcal{T}\bigl((1-b)y+b\Lambda y\bigr) \bigr\Vert ^{2} \\ &\leq \bigl\Vert (1-b)x+b\Lambda x-(1-b)y-b\Lambda y \bigr\Vert ^{2} \\ &= \bigl\Vert (1-b) (x-y)+b(\Lambda x-\Lambda y) \bigr\Vert ^{2} \\ &=(1-b) \Vert x-y \Vert ^{2}+b \Vert \Lambda x-\Lambda y \Vert ^{2}-b(1-b) \bigl\Vert (x-y)-( \Lambda x-\Lambda y) \bigr\Vert ^{2} \\ &\leq (1-b) \Vert x-y \Vert ^{2}+b \bigl\{ \Vert x-y \Vert ^{2}+\kappa \bigl\Vert (I-\Lambda )x-(I- \Lambda )y \bigr\Vert ^{2} \bigr\} \\ &\quad{}-b(1-b) \bigl\Vert (x-y)-(\Lambda x-\Lambda y) \bigr\Vert ^{2} \\ &\leq (1-b) \Vert x-y \Vert ^{2}+b \Vert x-y \Vert ^{2}+kb \bigl\Vert (x-y)-(\Lambda x-\Lambda y) \bigr\Vert ^{2} \\ &\quad{}-b(1-b) \bigl\Vert (x-y)-(\Lambda x-\Lambda y) \bigr\Vert ^{2} \\ &= \Vert x-y \Vert ^{2}+\bigl(kb-b(1-b)\bigr) \bigl\Vert (x-y)-( \Lambda x-\Lambda y) \bigr\Vert ^{2} \\ &= \Vert x-y \Vert ^{2}+b\bigl(k-(1-b)\bigr) \bigl\Vert (x-y)-( \Lambda x-\Lambda y) \bigr\Vert ^{2} \\ &\leq \Vert x-y \Vert ^{2}, \end{aligned}$$

for all $x,y\in \zeta$ with $(x,y)\in E(G)$.

This implies that $\|\mathcal{B} x-\mathcal{B} y\|\leq \|x-y\|$.

Hence, $\mathcal{B}$ is a G-nonexpansive mapping. □

Lemma 2.11

Let ζ be a nonempty, closed, and convex subset of a real Hilbert space H and let $G=(V(G),E(G))$ be a directed graph with $\zeta =V(G)$. Let $E(G)$ be convex and G be transitive with $E(G)=E(G)^{-1}$. Let $\mathcal{T}:\zeta \rightarrow \zeta$ be a G- nonexpansive mapping and $\Lambda :\zeta \rightarrow \zeta$ be a $G-\kappa$-strictly pseudocontractivea mapping. Define a mapping $\mathcal{B}:\zeta \rightarrow \zeta$ by $\mathcal{B}x=\mathcal{T}((1-b)I+b\Lambda )x$ for all $x\in \zeta$, $b\in (0,1-k)$ and $F(\mathcal{B})\times (F(\mathcal{T})\cap F(\Lambda ))\subseteq E(G)$. Then,

$$ F(\mathcal{B})=F(\mathcal{T})\cap F(\Lambda ). $$

Proof

It is obvious that $F(\mathcal{T})\cap F(\Lambda )\subseteq F(\mathcal{B})$. Next, we claim that $F(\mathcal{B})\subseteq F(\mathcal{T})\cap F(\Lambda )$. To show this, let $x_{0}\in F(\mathcal{B})$, $x^{*}\in F(\mathcal{T})\cap F(\Lambda )$, Then, we have $(x_{0},x^{*})\in E(G)$. Since Λ is edge preserving and $(x_{0},x^{*})\in E(G)$, then $(\Lambda x_{0}, x^{*})\in E(G)$. Since $E(G)=E(G)^{-1}$ and $(\Lambda x_{0}, x^{*})\in E(G)$, then we have $(x^{*},\Lambda x_{0})\in E(G)$. By the transitivity of G and $(x_{0},x^{*})\in E(G)$ and $(x^{*},\Lambda x_{0})\in E(G)$, then we have $(x_{0},\Lambda x_{0})\in E(G)$. As $(x_{0},x^{*})$ and $(\Lambda x_{0},x^{*})$ are in $E(G)$ and $E(G)$ is convex, we obtain

$$ \bigl((1-b)x_{0}+b\Lambda x_{0}, (1-b)x^{*}+bx^{*} \bigr)=\bigl((1-b)x_{0}+b\Lambda x_{0},x^{*}\bigr) \in E(G). $$

Then, we have

$$\begin{aligned} \|x_{0}=x^{*}\|^{2}&= \bigl\Vert \mathcal{T}\bigl((1-b)x_{0}+b\Lambda x_{0}\bigr)- \mathcal{T}x^{*} \bigr\Vert ^{2} \\ &\leq \bigl\Vert (1-b)x_{0}+b\Lambda x_{0}-x^{*} \bigr\Vert ^{2} \\ &= \bigl\Vert (1-b) \bigl(x_{0}-x^{*}\bigr)+b\bigl( \Lambda x_{0}-x^{*}\bigr) \bigr\Vert ^{2} \\ &=(1-b) \bigl\Vert x_{0}-x^{*} \bigr\Vert ^{2}+b \bigl\Vert \Lambda x_{0}-x^{*} \bigr\Vert ^{2} \\ &\quad{}-b(1-b) \bigl\Vert \bigl(x_{0}-x^{*}\bigr)-\bigl( \Lambda x_{0}-x^{*}\bigr) \bigr\Vert ^{2} \\ &\leq (1-b) \bigl\Vert x_{0}-x^{*} \bigr\Vert ^{2}+b \bigl\{ \bigl\Vert x_{0}-x^{*} \bigr\Vert ^{2}+k \bigl\Vert (I- \Lambda )x_{0}-(I-\Lambda )x^{*} \bigr\Vert ^{2} \bigr\} \\ &\quad{}-b(1-b) \Vert x_{0}-\Lambda x_{0} \Vert ^{2} \\ &= \bigl\Vert x_{0}-x^{*} \bigr\Vert ^{2}+bk \bigl\Vert (I-\Lambda )x_{0}-(I-\Lambda )x^{*} \bigr\Vert ^{2}-b(1-b) \Vert x_{0}-\Lambda x_{0} \Vert ^{2} \\ &= \bigl\Vert x_{0}-x^{*} \bigr\Vert ^{2}+bk \Vert x_{0}-\Lambda x_{0} \Vert ^{2}-b(1-b) \Vert x_{0}- \Lambda x_{0} \Vert ^{2} \\ &= \bigl\Vert x_{0}-x^{*} \bigr\Vert ^{2}-b \bigl((1-k)-b\bigr) \Vert x_{0}-\Lambda x_{0} \Vert ^{2}, \end{aligned}$$

(8)

for all $x_{0},x^{*}\in \zeta$ with $(x_{0},x^{*})\in E(G)$.

From (8), this implies that

$$ b\bigl((1-k)-b\bigr) \Vert x_{0}-\Lambda x_{0} \Vert ^{2}\leq \|x_{0}=x^{*} \Vert ^{2}- \|x_{0}=x^{*} \|^{2}=0, $$

for all $x_{0},x^{*}\in \zeta$ with $(x_{0},x^{*})\in E(G)$.

Then, we have $\Lambda x_{0}=x_{0}$.

That is, $x_{0}\in F(\Lambda )$.

Since $x_{0}\in F(\mathcal{B})$ from the definition of $\mathcal{B}$, we have

$$ x_{0}=\mathcal{B}x_{0}=\mathcal{T}\bigl((1-b)x_{0}+b \Lambda x_{0}\bigr)= \mathcal{T}x_{0}. $$

Then, we have $x_{0}\in F(\mathcal{T})$, therefore $x_{0}\in F(\mathcal{T})\cap F(\Lambda )$.

It follows that $F(\mathcal{B})\subseteq F(\mathcal{T})\cap F(\Lambda )$.

Hence, $F(\mathcal{B})=F(\mathcal{T})\cap F(\Lambda )$. □

3 Main results

In this section, we prove a strong convergence theorem for solving the fixed-point problem of two G-nonexpansive mappings, two $G-\kappa _{i}$-strictly pseudocontractive mappings, and two G-variational inequality problems in Hilbert space endowed with a directed graph.

The following Proposition is needed to prove the main theorem.

Proposition 3.1

Let ζ be a nonempty, closed, and convex subset of a real Hilbert space H and let $G=(V(G),E(G))$ be a directed graph with $\zeta =V(G)$. Let $E(G)$ be convex and G be transitive with $E(G)=E(G)^{-1}$. For every $i=1,2$, let $\mathcal{S}_{i}:\zeta \rightarrow H$ be a $G-\alpha _{i}$-inverse strongly monotone mapping with

$$ \textstyle\begin{cases} x_{n+1} = \delta _{n} x_{n}+\eta _{n} P_{\zeta}(I-\lambda _{1} \mathcal{S}_{1})x_{n}+\mu _{n}P_{\zeta}(\alpha _{n} f(y_{n})+(1- \alpha _{n})\mathcal{B}_{1} x_{n}), \\ y_{n+1} = \delta _{n} y_{n}+\eta _{n} P_{\zeta}(I-\lambda _{2} \mathcal{S}_{2})y_{n}+\mu _{n}P_{\zeta}(\alpha _{n} g(x_{n})+(1- \alpha _{n})\mathcal{B}_{2} y_{n}), \end{cases} $$

(9)

where $(x_{0},x_{0})$ and $(y_{0},y_{0})$ are in $E(G)$. If $\zeta =V(G)$ dominates $x_{0}$ and $y_{0}$, then $(x_{n},x_{n+1})$, $(y_{n},y_{n+1})$ are in $E(G)$ for all $n\in \mathbb{N}$.

Proof

Since $\zeta =V(G)$ dominates $x_{0}$ and $P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{0}\in \zeta$, then we have $(P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{0},x_{0})\in E(G)$. From $E(G)=E(G)^{-1}$ and $(P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{0},x_{0})\in E(G)$, we have $(x_{0},P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{0})\in E(G)$. Since $\zeta =V(G)$ dominates $x_{0}$ and $U_{0}=P_{\zeta}(\alpha _{0} f(y_{0})+(1-\alpha _{0})\mathcal{B}_{1} x_{0}) \in \zeta$, then we have $(U_{0},x_{0})\in E(G)$. From $E(G)=E(G)^{-1}$ and $(U_{0},x_{0})\in E(G)$, we have $(x_{0},U_{0})\in E(G)$. Since $(x_{0},x_{0})$, $(x_{0},P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{0})$ and $(x_{0},U_{0})$ are in $E(G)$ and $E(G)$ is convex, we obtain

$$ \bigl(\delta _{0}x_{0}+\eta _{0}x_{0}+ \mu _{0}x_{0}, \delta _{0}x_{0}+ \eta _{0}P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{0}+ \mu _{0}U_{0}\bigr)=(x_{0},x_{1}) \in E(G). $$

Since $\zeta =V(G)$ dominates $x_{0}$ and $P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{1}\in \zeta$, then we have $(P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{1},x_{0})\in E(G)$. From $E(G)=E(G)^{-1}$ and $(P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{1},x_{0})\in E(G)$, we have $(x_{0},P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{1})\in E(G)$. Since $\zeta =V(G)$ dominates $x_{0}$ and $U_{1}=P_{\zeta}(\alpha _{1} f(y_{1})+(1-\alpha _{1})\mathcal{B}_{1} x_{1}) \in \zeta$, then we have $(U_{1},x_{0})\in E(G)$. From $E(G)=E(G)^{-1}$ and $(U_{1},x_{0})\in E(G)$, we have $(x_{0} ,U_{1})\in E(G)$. Since $(x_{0},x_{1})$, $(x_{0},P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{1})$ and $(x_{0},U_{1})$ are in $E(G)$ and $E(G)$ is convex, we obtain

$$ \bigl(\delta _{0}x_{0}+\eta _{0}x_{0}+ \mu _{0}x_{0}, \delta _{0}x_{1}+ \eta _{0}P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{1}+ \mu _{0}U_{1}\bigr)=(x_{0},x_{2}) \in E(G). $$

Since $\zeta =V(G)$ dominates $x_{0}$ and $P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{k}\in \zeta$, then we have $(P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{k},x_{0})\in E(G)$, for $k\in \mathbb{N}$. From $E(G)=E(G)^{-1}$ and $(P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{k},x_{0})\in E(G)$, we have $(x_{0}, P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{k})\in E(G)$, for $k\in \mathbb{N}$. Since $\zeta =V(G)$ dominates $x_{0}$ and $U_{k}=P_{\zeta}(\alpha _{k} f(y_{k})+(1-\alpha _{k})\mathcal{B}_{k} x_{k}) \in \zeta$, then we have $(U_{k},x_{0})\in E(G)$, for $k\in \mathbb{N}$. From $E(G)=E(G)^{-1}$ and $(U_{k},x_{0})\in E(G)$, we have $(x_{0},U_{k})\in E(G)$, for $k\in \mathbb{N}$.

Next, Assume that $(x_{0},x_{k})\in E(G)$, for $k\in \mathbb{N}$. Since $(x_{0},x_{k})$, $(x_{0}, P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{k})$, and $(x_{0},U_{k})$ are in $E(G)$ and $E(G)$ is convex, then we obtain $(\delta _{0}x_{0}+\eta _{0}x_{0}+\mu _{0}x_{0}, \delta _{0}x_{k}+ \eta _{0}P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{k}+\mu _{0}U_{k})=(x_{0},x_{k+1}) \in E(G)$.

Hence, by induction, we have $(x_{0},x_{n})\in E(G)$. From $E(G)=E(G)^{-1}$ and $(x_{0},x_{n})\in E(G)$, we have $(x_{n},x_{0})\in E(G)$, for all $n\in \mathbb{N}$. By the transitivity of G and $(x_{n},x_{0}),(x_{0},x_{n+1})\in E(G)$, we have

$$ (x_{n},x_{n+1})\in E(G), \quad\text{for all }n\in \mathbb{N}. $$

(10)

Applying the same arguments as for deriving (10), we also obtain

$$ (y_{n},y_{n+1})\in E(G),\quad\text{for all }n\in \mathbb{N}. $$

 □

Theorem 3.2

Let ζ be a nonempty, closed, and convex subset of a real Hilbert space H and let $G=(V(G),E(G))$ be a directed graph with $\zeta =V(G)$. Let $E(G)$ be convex and G be transitive with $E(G)=E(G)^{-1}$. For every $i=1,2$, let $\mathcal{S}_{i}:\zeta \rightarrow H$ be a $G-\alpha _{i}$-inverse strongly monotone mapping with $\alpha =\min \{\alpha _{1},\alpha _{2}\}$ and let $g,f:H\rightarrow H$ be an $a_{g}$ and $a_{f}-G$-contraction mapping with $a=\max \{a_{g},a_{f}\}$. For every $i=1,2$, let $\mathcal{T}_{i}:\zeta \rightarrow \zeta$ be a G-nonexpansive mapping and $\Lambda _{i}:\zeta \rightarrow \zeta$ be a $G-\kappa _{i}$-strictly pseudocontractive mapping with $k=\max \{\kappa _{1},\kappa _{2}\}$. Define a mapping $\mathcal{B}:\zeta \rightarrow \zeta$ by $\mathcal{B}_{i}x=\mathcal{T}_{i}((1-b)I+b\Lambda _{i})x$ for all $x\in \zeta$ and $i=1,2$, $b\in (0,1-k)$. Assume that $\mathfrak{F}_{i}=F(\mathcal{B}_{i})\cap G-VI(\zeta ,\mathcal{S}_{i}) \neq \emptyset$ for all $i=1,2$ with $F(\mathcal{B}_{i})\times F(\mathcal{B}_{i})\subseteq E(G)$, $G-VI(\zeta ,\mathcal{S}_{i})\times G-VI(\zeta ,\mathcal{S}_{i}) \subseteq E(G)$ and $F(\mathcal{B})\times (F(\mathcal{T})\cap F(\Lambda ))\subseteq E(G)$ for all $i=1,2$ and there exists $x_{0},y_{0}\in \zeta$ such that $(x_{0},x_{0})$ and $(y_{0},y_{0})$ are in $E(G)$ and $\zeta =V(G)$ dominates $x_{0}$ and $y_{0}$. Let the sequences $\{x_{n}\}$ and $\{y_{n}\}$ be generated by $x_{0},y_{0}\in \zeta$ and

$$ \textstyle\begin{cases} x_{n+1} = \delta _{n} x_{n}+\eta _{n} P_{\zeta}(I-\lambda _{1} \mathcal{S}_{1})x_{n}+\mu _{n}P_{\zeta}(\alpha _{n} f(y_{n})+(1- \alpha _{n})\mathcal{B}_{1} x_{n}), \\ y_{n+1} = \delta _{n} y_{n}+\eta _{n} P_{\zeta}(I-\lambda _{2} \mathcal{S}_{2})y_{n}+\mu _{n}P_{\zeta}(\alpha _{n} g(x_{n})+(1- \alpha _{n})\mathcal{B}_{2} y_{n}), \end{cases} $$

(11)

where $\{\delta _{n} \}, \{\eta _{n} \}, \{ \mu _{n} \}, \{\alpha _{n} \} \subseteq [0,1]$ with $\delta _{n}+\eta _{n}+\mu _{n}=1$ and $\lambda \in (0,2\alpha )$ with $\lambda =\min\{\lambda _{1},\lambda _{2}\}$. Assume the following conditions hold;

• (i) $0 <\xi \leq \delta _{n},\eta _{n}$, $\mu _{n}\leq \bar{\xi}$ for all $n\in N$ and for some $\xi ,\bar{\xi}>0$;

• (ii) $\lim_{n \rightarrow \infty} \alpha _{n}=0$ and $\sum_{n=1}^{\infty} \alpha _{n} = \infty$;

• (iii) $\sum_{n=1}^{\infty} \lvert \delta _{n+1}-\delta _{n} \rvert < \infty$, $\sum_{n=1}^{\infty} \lvert \eta _{n+1}-\eta _{n} \rvert < \infty$, $\sum_{n=1}^{\infty} \lvert \alpha _{n+1}-\alpha _{n} \rvert < \infty$.

If $\{x_{n}\}$ dominates $P_{\mathfrak{F}_{1}} f(y_{0})$ and $\{y_{n}\}$ dominates $P_{\mathfrak{F}_{2}} g(x_{0})$, then $\{x_{n}\}$ converges strongly to $x^{*}=P_{\mathfrak{F}_{1}} f(y_{0})$ and $\{y_{n}\}$ converges strongly to $y^{*}=P_{\mathfrak{F}_{2}} g(x_{0})$, where $P_{\mathfrak{F}_{i}}$ is a metric projection on $\mathfrak{F}_{i}$, for all $i=1,2$.

Proof

The proof of this theorem will be divided into five steps.

Step 1: We will show that $\{x_{n}\}$ is bounded.

First, we will prove that

$$\begin{aligned} \bigl\Vert P_{\zeta}(I-\lambda \mathcal{S})x-P_{\zeta}(I- \lambda \mathcal{S})y \bigr\Vert \leq \Vert x-y \Vert , \end{aligned}$$

(12)

for all $x,y\in \zeta$ and $(x,y)\in E(G)$.

Letting $x,y\in \zeta$ and $(x,y)\in E(G)$, we have

$$\begin{aligned} \bigl\Vert P_{\zeta}(I-\lambda \mathcal{S})x- P_{\zeta}(I- \lambda \mathcal{S})y \bigr\Vert ^{2}&\leq \bigl\Vert x-y-\lambda ( \mathcal{S} x-\mathcal{S} y) \bigr\Vert ^{2} \\ &= \Vert x-y \Vert ^{2}-2\lambda \langle x-y,\mathcal{S} y- \mathcal{S} y \rangle \\ &\quad{}+\lambda ^{2} \Vert \mathcal{S} x-\mathcal{S} y \Vert ^{2} \\ &\leq \Vert x-y \Vert ^{2}-2\alpha \lambda \Vert \mathcal{S} x- \mathcal{S} y \Vert ^{2} \\ &\quad{}+\lambda ^{2} \Vert \mathcal{S} x-\mathcal{S} y \Vert ^{2} \\ &= \Vert x-y \Vert ^{2}-\lambda (2\alpha -\lambda ) \Vert \mathcal{S} x-\mathcal{S} y \Vert ^{2} \\ &\leq \Vert x-y \Vert ^{2}, \end{aligned}$$

for all $x,y\in \zeta$ with $(x,y)\in E(G)$.

From Lemmas 2.7 and 2.8, we have $G-VI(\zeta ,\mathcal{S}_{i})$ is closed and convex, for all $i=1,2$.

From Lemma 2.9, we have $F(\mathcal{B}_{i})$ is closed and convex. Then, $\mathfrak{F}_{i}$ is closed and convex, for all $i=1,2$.

Let $x^{*}=P_{\mathfrak{F}_{1}} f(y_{0})$ be dominated by $\{x_{n}\}$ and $y^{*}=P_{\mathfrak{F}_{2}} g(x_{0})$ be dominated by $\{y_{n}\}$, we have $(x_{n},x^{*})$ and $(y_{n},y^{*})$ are in $E(G)$ for all $n\in \mathbb{N}$. From Lemma 2.7, we have $G-VI(\zeta ,\mathcal{S}_{1})=\mathcal{S}_{1}^{-1}(0)$.

Then, $x^{*}\in \mathcal{S}_{1}^{-1}(0)$. Since $\mathcal{S}_{1}x^{*}=0$, we have

$$\begin{aligned} \bigl\Vert P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{n}-x^{*} \bigr\Vert ^{2}&\leq \bigl\Vert x_{n}-x^{*}- \lambda _{1}\mathcal{S}_{1}x_{n} \bigr\Vert ^{2} \\ &= \bigl\Vert x_{n}-x^{*}-\bigl(\lambda _{1} \mathcal{S}_{1}x_{n}-\lambda _{1} \mathcal{S}_{1}x^{*}\bigr) \bigr\Vert ^{2} \\ &= \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2}-2 \lambda _{1}\bigl\langle x_{n}-x^{*}, \mathcal{S}_{1}x_{n}- \mathcal{S}_{1} x^{*}\bigr\rangle \\ &\quad{}+\lambda _{1}^{2} \bigl\Vert \mathcal{S}_{1}x_{n}- \mathcal{S}_{1} x^{*} \bigr\Vert ^{2} \\ &= \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2}-2 \lambda _{1}\alpha \bigl\Vert \mathcal{S}_{1}x_{n}- \mathcal{S}_{1} x^{*} \bigr\Vert ^{2}+\lambda _{1}^{2} \Vert \mathcal{S}_{1}x_{n} \Vert ^{2} \\ &= \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2}- \lambda _{1}(2\alpha -\lambda _{1}) \Vert \mathcal{S}_{1}x_{n} \Vert ^{2} \\ &\leq \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2} . \end{aligned}$$

From the definition of $\{x_{n}\}$, we have

$$\begin{aligned} \bigl\Vert x_{n+1}-x^{*} \bigr\Vert &= \bigl\Vert \delta _{n} x_{n}+\eta _{n} P_{\zeta}(I-\lambda _{1} \mathcal{S}_{1})x_{n}+ \mu _{n}P_{\zeta}\bigl(\alpha _{n}f(y_{n})+(1- \alpha _{n})\mathcal{B}_{1} x_{n}\bigr) \\ &\quad{}-(\delta _{n}+\eta _{n}+\mu _{n})x^{*} \bigr\Vert \\ &\leq \delta _{n} \bigl\Vert x_{n}-x^{*} \bigr\Vert +\eta _{n} \bigl\Vert P_{\zeta}(I-\lambda _{1} \mathcal{S}_{1})x_{n}-x^{*} \bigr\Vert +\mu _{n} \bigl\Vert P_{\zeta}\bigl(\alpha _{n}f(y_{n}) \\ &\quad{}+(1-\alpha _{n})\mathcal{B}_{1} x_{n} \bigr)-x^{*} \bigr\Vert \\ &\leq (1-\mu _{n}) \bigl\Vert x_{n}-x^{*} \bigr\Vert +\mu _{n} \bigl\Vert \alpha _{n} \bigl(f(y_{n})-x^{*}\bigr)+(1- \alpha _{n}) \bigl( \mathcal{B}_{1} x_{n}-x^{*}\bigr) \bigr\Vert \\ &\leq (1-\mu _{n}) \bigl\Vert x_{n}-x^{*} \bigr\Vert +\mu _{n}\alpha _{n} \bigl\Vert f(y_{n})-x^{*} \bigr\Vert +\mu _{n}(1-\alpha _{n}) \bigl\Vert x_{n}-x^{*} \bigr\Vert \\ &\leq (1-\mu _{n}) \bigl\Vert x_{n}-x^{*} \bigr\Vert +\mu _{n}\alpha _{n} a \bigl\Vert y_{n}-y^{*} \bigr\Vert +\mu _{n}\alpha _{n} \bigl\Vert f\bigl(y^{*}\bigr)-x^{*} \bigr\Vert \\ &\quad{}+\mu _{n}(1-\alpha _{n}) \bigl\Vert x_{n}-x^{*} \bigr\Vert \\ &=(1-\mu _{n}\alpha _{n}) \bigl\Vert x_{n}-x^{*} \bigr\Vert +\mu _{n}\alpha _{n} a \bigl\Vert y_{n}-y^{*} \bigr\Vert +\mu _{n}\alpha _{n} \bigl\Vert f\bigl(y^{*} \bigr)-x^{*} \bigr\Vert . \end{aligned}$$

(13)

Similarly, we obtain

$$\begin{aligned} \bigl\Vert y_{n+1}-y^{*} \bigr\Vert &\leq (1-\mu _{n}\alpha _{n}) \bigl\Vert y_{n}-y^{*} \bigr\Vert +\mu _{n} \alpha _{n} a \bigl\Vert x_{n}-x^{*} \bigr\Vert +\mu _{n}\alpha _{n} \bigl\Vert g\bigl(x^{*}\bigr)-y^{*} \bigr\Vert . \end{aligned}$$

(14)

Combining (13) and (14), we have

$$\begin{aligned} \bigl\Vert x_{n+1}-x^{*} \bigr\Vert + \bigl\Vert y_{n+1}-y^{*} \bigr\Vert &\leq (1-\mu _{n}\alpha _{n}) \bigl\{ \bigl\Vert x_{n}-x^{*} \bigr\Vert + \bigl\Vert y_{n}-y^{*} \bigr\Vert \bigr\} \\ &\quad{}+\mu _{n}\alpha _{n} a \bigl\{ \bigl\Vert x_{n}-x^{*} \bigr\Vert + \bigl\Vert y_{n}-y^{*} \bigr\Vert \bigr\} \\ &\quad{}+\mu _{n}\alpha _{n}\bigl\{ \bigl\Vert g \bigl(x^{*}\bigr)-y^{*} \bigr\Vert + \bigl\Vert f \bigl(y^{*}\bigr)-x^{*} \bigr\Vert \bigr\} \\ &=\bigl(1-\mu _{n}\alpha _{n}(1-a)\bigr) \bigl\{ \bigl\Vert x_{n}-x^{*} \bigr\Vert + \bigl\Vert y_{n}-y^{*} \bigr\Vert \bigr\} \\ &\quad{}+ \frac{\mu _{n}\alpha _{n} \{ \Vert g(x^{*})-y^{*} \Vert + \Vert f(y^{*})-x^{*} \Vert \}}{(1-a)}. \end{aligned}$$

By induction, we can derive that

$$\begin{aligned} \bigl\Vert x_{n}-x^{*} \bigr\Vert + \bigl\Vert y_{n}-y^{*} \bigr\Vert &\leq \max \biggl\{ \bigl\Vert x_{1}-x^{*} \bigr\Vert + \bigl\Vert y_{1}-y^{*} \bigr\Vert ,\frac{ \Vert g(x^{*})-y^{*} \Vert + \Vert f(y^{*})-x^{*} \Vert }{1-a} \biggr\} , \end{aligned}$$

for every $n\in \mathbb{N}$. This implies that $\{x_{n}\}$ and $\{y_{n}\}$ are bounded.

Step 2: We claim that $\lim_{n \to \infty}\|x_{n+1}-x_{n}\|= \lim_{n \to \infty}\|y_{n+1}-y_{n}\|=0$.

From Proposition 3.1, we have $(x_{n},x_{n+1})$ and $(y_{n},y_{n+1})$ are in $E(G)$ for all $n\in \mathbb{N}$. First, we let $U_{n}=P_{\zeta}(\alpha _{n}f(y_{n})+(1-\alpha _{n})\mathcal{B}_{1} x_{n})$ and $V_{n}=P_{\zeta}(\alpha _{n} g(x_{n})+(1-\alpha _{n})\mathcal{B}_{2} y_{n})$. Then, we observe that

$$\begin{aligned} \Vert U_{n}-U_{u-1} \Vert &= \bigl\Vert P_{\zeta}\bigl(\alpha _{n}f(y_{n})+(1-\alpha _{n}) \mathcal{B}_{1} x_{n}\bigr)-P_{\zeta} \bigl(\alpha _{n-1}f(y_{n-1}) \\ &\quad{}+(1-\alpha _{n-1})\mathcal{B}_{1} x_{n-1}\bigr) \bigr\Vert \\ &\leq \alpha _{n} \bigl\Vert f(y_{n})-f(y_{n-1}) \bigr\Vert +\lvert \alpha _{n}-\alpha _{n-1} \rvert \bigl\Vert f(y_{n-1}) \bigr\Vert \\ &\quad{}+(1-\alpha _{n}) \Vert \mathcal{B}_{1} x_{n}-\mathcal{B}_{1} x_{n-1} \Vert \\ &\quad{}+\lvert \alpha _{n}-\alpha _{n-1} \rvert \Vert \mathcal{B}_{1} x_{n-1} \Vert \\ &\leq \alpha _{n} a \Vert y_{n}-y_{n-1} \Vert +\lvert \alpha _{n}-\alpha _{n-1} \rvert \bigl\{ \bigl\Vert f(y_{n-1}) \bigr\Vert + \Vert \mathcal{B}_{1} x_{n-1} \Vert \bigr\} \\ &\quad{}+(1-\alpha _{n}) \Vert x_{n}-x_{n-1} \Vert . \end{aligned}$$

(15)

By the definition of $x_{n}$ and (15) we obtain

$$\begin{aligned} \Vert x_{n+1}-x_{n} \Vert &= \bigl\Vert \delta _{n} x_{n}+\eta _{n} P_{\zeta}(I- \lambda _{1} \mathcal{S}_{1})x_{n}+\mu _{n} U_{n}-\delta _{n-1} x_{n-1} \\ &\quad{}-\eta _{n-1} P_{\zeta}(I-\lambda _{1} \mathcal{S}_{1})x_{n-1}- \mu _{n-1} U_{n-1} \bigr\Vert \\ &\leq \delta _{n} \Vert x_{n}-x_{n-1} \Vert + \lvert \delta _{n}-\delta _{n-1} \rvert \Vert x_{n-1} \Vert +\eta _{n} \bigl\Vert P_{\zeta}(I- \lambda _{1}\mathcal{S}_{1})x_{n} \\ &\quad{}-P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{n-1} \bigr\Vert +\lvert \eta _{n}-\eta _{n-1} \rvert \bigl\Vert P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})x_{n-1} \bigr\Vert \\ &\quad{}+\mu _{n} \Vert U_{n}-U_{n-1} \Vert +\lvert \mu _{n}-\mu _{n-1} \rvert \Vert U_{n-1} \Vert \\ &\leq (1-\mu _{n}) \Vert x_{n}-x_{n-1} \Vert +\lvert \delta _{n}-\delta _{n-1} \rvert \Vert x_{n-1} \Vert \\ &\quad{}+\lvert \eta _{n}-\eta _{n-1} \rvert \bigl\Vert P_{\zeta}(I-\lambda _{1} \mathcal{S}_{1})x_{n-1} \bigr\Vert \\ &\quad{}+\mu _{n}\lvert \alpha _{n}-\alpha _{n-1} \rvert \bigl\{ \bigl\Vert f(y_{n-1}) \bigr\Vert + \Vert G_{1} x_{n-1} \Vert \bigr\} \\ &\quad{}+\mu _{n}(1-\alpha _{n}) \Vert x_{n}-x_{n-1} \Vert +\lvert \mu _{n}- \mu _{n-1} \rvert \Vert U_{n-1} \Vert \\ &\quad{}+\mu _{n}\alpha _{n} a \Vert y_{n}-y_{n-1} \Vert . \end{aligned}$$

(16)

Using the same method as derived in (16), we have

$$\begin{aligned} \Vert y_{n+1}-y_{n} \Vert &\leq (1-\mu _{n}) \Vert y_{n}-y_{n-1} \Vert +\lvert \delta _{n}- \delta _{n-1} \rvert \Vert y_{n-1} \Vert \\ &\quad{}+\lvert \eta _{n}-\eta _{n-1} \rvert \bigl\Vert P_{\zeta}(I-\lambda _{2} \mathcal{S}_{2})y_{n-1} \bigr\Vert \\ &\quad{}+\mu _{n}\lvert \alpha _{n}-\alpha _{n-1} \rvert \bigl\{ \bigl\Vert g(x_{n-1}) \bigr\Vert + \Vert \mathcal{B}_{2} y_{n-1} \Vert \bigr\} \\ &\quad{}+\mu _{n}(1-\alpha _{n}) \Vert y_{n}-y_{n-1} \Vert +\lvert \mu _{n}- \mu _{n-1} \rvert \Vert V_{n-1} \Vert \\ &\quad{}+\mu _{n}\alpha _{n} a \Vert x_{n}-x_{n-1} \Vert . \end{aligned}$$

(17)

From (16) and (17), we obtain

$$\begin{aligned} \Vert x_{n+1}-x_{n} \Vert + \Vert y_{n+1}-y_{n} \Vert &\leq (1-\mu _{n}) \bigl[ \Vert x_{n}-x_{n-1} \Vert + \Vert y_{n}-y_{n-1} \Vert \bigr] \\ &\quad{}+\lvert \delta _{n}-\delta _{n-1} \rvert \bigl[ \Vert x_{n-1} \Vert + \Vert y_{n-1} \Vert \bigr] \\ &\quad{}+\lvert \eta _{n}-\eta _{n-1} \rvert \bigl[ \bigl\Vert P_{\zeta}(I-\lambda _{1} \mathcal{S}_{1})x_{n-1} \bigr\Vert \\ &\quad{}+ \bigl\Vert P_{\zeta}(I-\lambda _{2}\mathcal{S}_{2})y_{n-1} \bigr\Vert \bigr] \\ &\quad{}+\lvert \mu _{n}-\mu _{n-1} \rvert \bigl[ \Vert U_{n-1} \Vert + \Vert V_{n-1} \Vert \bigr] \\ &\quad{}+\mu _{n}\alpha _{n} a \bigl[ \Vert y_{n}-y_{n-1} \Vert + \Vert x_{n}-x_{n-1} \Vert \bigr] \\ &\quad{}+\mu _{n}\lvert \alpha _{n}-\alpha _{n-1} \rvert \bigl[ \bigl\Vert f(y_{n-1}) \bigr\Vert + \Vert \mathcal{B}_{1} x_{n-1} \Vert \\ &\quad{}+ \bigl\Vert g(x_{n-1}) \bigr\Vert + \Vert \mathcal{B}_{2}y_{n-1} \Vert \bigr] \\ &\quad{}+\mu _{n}(1-\alpha _{n}) \bigl[ \Vert x_{n}-x_{n-1} \Vert + \Vert y_{n}-y_{n-1} \Vert \bigr] \\ &\leq \bigl(1-\alpha _{n}\bar{\xi}(1-a)\bigr) \bigl[ \Vert x_{n}-x_{n-1} \Vert + \Vert y_{n}-y_{n-1} \Vert \bigr] \\ &\quad{}+\lvert \delta _{n}-\delta _{n-1} \rvert \bigl[ \Vert x_{n-1} \Vert + \Vert y_{n-1} \Vert \bigr] \\ &\quad{}+\lvert \eta _{n}-\eta _{n-1} \rvert \bigl[ \bigl\Vert P_{\zeta}(I-\lambda _{1} \mathcal{S}_{1})x_{n-1} \bigr\Vert \\ &\quad{}+ \bigl\Vert P_{\zeta}(I-\lambda _{2}\mathcal{S}_{2})y_{n-1} \bigr\Vert \bigr] \\ &\quad{}+\lvert \mu _{n}-\mu _{n-1} \rvert \bigl[ \Vert U_{n-1} \Vert + \Vert V_{n-1} \Vert \bigr] \\ &\quad{}+\xi \lvert \alpha _{n}-\alpha _{n-1} \rvert \bigl[ \bigl\Vert f(y_{n-1}) \bigr\Vert + \Vert \mathcal{B}_{1} x_{n-1} \Vert \\ &\quad{}+ \bigl\Vert g(x_{n-1}) \bigr\Vert + \Vert \mathcal{B}_{2} y_{n-1} \Vert \bigr]. \end{aligned}$$

Applying Lemma 2.1 and the conditions (ii) and (iii) we can conclude that

$$\begin{aligned} \Vert x_{n+1}-x_{n} \Vert \rightarrow 0 \quad\text{and}\quad \Vert y_{n+1}-y_{n} \Vert \rightarrow 0 \quad\text{as } n\rightarrow \infty . \end{aligned}$$

(18)

Step 3: We prove that $\lim_{n \to \infty}\|U_{n}-P_{\zeta }(I-\lambda _{1} \mathcal{S}_{1})U_{n}\|= \lim_{n \to \infty}\|U_{n}- \mathcal{B}_{1} U_{n}\|=0$.

To show this, take $\tilde{u}_{n}=\alpha _{n}f(y_{n})+(1-\alpha _{n})\mathcal{B}_{1} x_{n}$, $\forall n\in \mathbb{N}$.

Since $x^{*}$ is dominated by $\{x_{n}\}$, $y^{*}$ is dominated by $\{y_{n}\}$ and $(x_{n},x_{n+1})$, and $(y_{n},y_{n+1})$ are in $E(G)$ for all $n\in \mathbb{N}$, then we derive that

$$\begin{aligned} \bigl\Vert x_{n+1}-x^{*} \bigr\Vert ^{2}&= \bigl\Vert \delta _{n}\bigl(x_{n}-x^{*}\bigr)+ \eta _{n}\bigl(P_{\zeta }(I- \lambda _{1} \mathcal{S}_{1})x_{n}-x^{*}\bigr)+\mu _{n}\bigl(U_{n}-x^{*}\bigr) \bigr\Vert ^{2} \\ &\leq \delta _{n} \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2}+\eta _{n} \bigl\Vert P_{\zeta }(I- \lambda _{1} \mathcal{S}_{1})x_{n}-x^{*} \bigr\Vert ^{2} \\ &\quad{}-\delta _{n}\eta _{n} \bigl\Vert x_{n}-P_{\zeta }(I- \lambda _{1} \mathcal{S}_{1})x_{n} \bigr\Vert ^{2}+\mu _{n} \bigl\Vert \tilde{u}_{n}-x^{*} \bigr\Vert ^{2} \\ &\leq (1-\mu _{n}) \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2}-\delta _{n}\eta _{n} \bigl\Vert x_{n}-P_{ \zeta }(I-\lambda _{1} \mathcal{S}_{1})x_{n} \bigr\Vert ^{2} \\ &\quad{}+\mu _{n} \bigl\Vert \alpha _{n}\bigl(f(y_{n})- \mathcal{B}_{1} x_{n}\bigr)+\bigl( \mathcal{B}_{1} x_{n}-x^{*}\bigr) \bigr\Vert ^{2} \\ &\leq (1-\mu _{n}) \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2}-\delta _{n}\eta _{n} \bigl\Vert x_{n}-P_{ \zeta }(I-\lambda _{1} A_{1})x_{n} \bigr\Vert ^{2} \\ &\quad{}+\mu _{n} \bigl\{ \bigl\Vert \mathcal{B}_{1} x_{n}-x^{*} \bigr\Vert ^{2}+2 \alpha _{n}\bigl\langle f(y_{n})-\mathcal{B}_{1} x_{n},\tilde{u}_{n}-x^{*} \bigr\rangle \bigr\} \\ &\leq \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2}- \delta _{n}\eta _{n} \bigl\Vert x_{n}-P_{\zeta }(I- \lambda _{1} \mathcal{S}_{1})x_{n} \bigr\Vert ^{2} \\ &\quad{}+2\mu _{n}\alpha _{n} \bigl\Vert f(y_{n})- \mathcal{B}_{1} x_{n} \bigr\Vert \bigl\Vert \tilde{u}_{n}-x^{*} \bigr\Vert , \end{aligned}$$

which implies that

$$\begin{aligned} \delta _{n}\eta _{n} \bigl\Vert x_{n}-P_{\zeta }(I- \lambda _{1} \mathcal{S}_{1})x_{n} \bigr\Vert ^{2}&\leq \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2}- \bigl\Vert x_{n+1}-x^{*} \bigr\Vert ^{2} \\ &\quad{}+2\mu _{n}\alpha _{n} \bigl\Vert f(y_{n})- \mathcal{B}_{1} x_{n} \bigr\Vert \bigl\Vert \tilde{u}_{n}-x^{*} \bigr\Vert \\ &\leq \Vert x_{n}-x_{n+1} \Vert \bigl\{ \bigl\Vert x_{n}-x^{*} \bigr\Vert + \bigl\Vert x_{n+1}-x^{*} \bigr\Vert \bigr\} \\ &\quad{}+2\mu _{n}\alpha _{n} \bigl\Vert f(y_{n})- \mathcal{B}_{1} x_{n} \bigr\Vert \bigl\Vert \tilde{u}_{n}-x^{*} \bigr\Vert . \end{aligned}$$

Then, we have

$$\begin{aligned} \bigl\Vert x_{n}-P_{\zeta }(I-\lambda _{1} \mathcal{S}_{1})x_{n} \bigr\Vert \rightarrow 0 \quad\text{as } n\rightarrow \infty . \end{aligned}$$

(19)

Observe that

$$\begin{aligned} x_{n+1}-x_{n}=\eta _{n}\bigl(P_{\zeta}(I- \lambda _{1} \mathcal{S}_{1})x_{n}-x_{n} \bigr)+ \mu _{n}(U_{n}-x_{n}). \end{aligned}$$

It follows that

$$\begin{aligned} \mu _{n} \Vert U_{n}-x_{n} \Vert \leq \eta _{n} \bigl\Vert P_{\zeta }(I-\lambda _{1} \mathcal{S}_{1})x_{n}-x_{n} \bigr\Vert + \Vert x_{n+1}-x_{n} \Vert . \end{aligned}$$

From (18) and (19), we obtain

$$\begin{aligned} \Vert U_{n}-x_{n} \Vert \rightarrow 0 \quad\text{as } n\rightarrow \infty . \end{aligned}$$

(20)

Since $\zeta =V(G)$ dominates $x_{0}$ and $U_{n},x_{n}\in \zeta$, then we have $(U_{n},x_{0})$ and $(x_{n},x_{0})$ are in $E(G)$, for all $n\in \mathbb{N}$. Since $E(G)=E(G)^{-1}$ and $(x_{n},x_{0})\in E(G)$, then $(x_{0},x_{n})\in E(G)$, for all $n\in \mathbb{N}$. By the transitivity of G and $(U_{n},x_{0}),(x_{0},x_{n})\in E(G)$, for all $n\in \mathbb{N}$, we obtain $(U_{n},x_{n})\in E(G)$, for all $n\in \mathbb{N}$. Since $E(G)=E(G)^{-1}$ and $(U_{n},x_{n})\in E(G)$, then $(x_{n},U_{n})\in E(G)$, for all $n\in \mathbb{N}$.

Observe that

$$\begin{aligned} \bigl\Vert U_{n}-P_{\zeta }(I-\lambda _{1} \mathcal{S}_{1})U_{n} \bigr\Vert &\leq \Vert U_{n}-x_{n} \Vert + \bigl\Vert x_{n}-P_{\zeta }(I- \lambda _{1} \mathcal{S}_{1})x_{n} \bigr\Vert \\ &\quad{}+ \bigl\Vert P_{\zeta }(I-\lambda _{1} \mathcal{S}_{1})x_{n}-P_{\zeta }(I- \lambda _{1} \mathcal{S}_{1})U_{n} \bigr\Vert \\ &\leq \Vert U_{n}-x_{n} \Vert + \bigl\Vert x_{n}-P_{\zeta}(I-\lambda _{1} \mathcal{S}_{1})x_{n} \bigr\Vert + \Vert x_{n}-U_{n} \Vert \\ &=2 \Vert U_{n}-x_{n} \Vert + \bigl\Vert x_{n}-P_{\zeta }(I-\lambda _{1} \mathcal{S}_{1})x_{n} \bigr\Vert , \end{aligned}$$

by (12), (19), and (20), we obtain

$$\begin{aligned} \bigl\Vert U_{n}-P_{\zeta }(I-\lambda _{1} \mathcal{S}_{1})U_{n} \bigr\Vert \rightarrow 0 \quad\text{as } n\rightarrow \infty . \end{aligned}$$

(21)

Applying the same arguments as for deriving (21), we also obtain

$$\begin{aligned} \bigl\Vert V_{n}-P_{\zeta }(I-\lambda _{2} \mathcal{S}_{2})V_{n} \bigr\Vert \rightarrow 0 \quad \text{as } n\rightarrow \infty . \end{aligned}$$

Consider

$$\begin{aligned} \Vert x_{n+1}-U_{n} \Vert &\leq \Vert x_{n+1}-x_{n} \Vert + \Vert x_{n}-U_{n} \Vert . \end{aligned}$$

From (18) and (20), we have

$$\begin{aligned} \Vert x_{n+1}-U_{n} \Vert \rightarrow 0 \quad\text{as } n\rightarrow \infty . \end{aligned}$$

(22)

Since

$$\begin{aligned} \Vert x_{n}-\mathcal{B}_{1}x_{n} \Vert & \leq \Vert x_{n}-x_{n+1} \Vert + \Vert x_{n+1}-U_{n} \Vert + \Vert U_{n}- \mathcal{B}_{1} x_{n} \Vert \\ &\leq \Vert x_{n}-x_{n+1} \Vert + \Vert x_{n+1}-U_{n} \Vert + \Vert \tilde{u}_{n}- \mathcal{B}_{1} x_{n} \Vert \\ &= \Vert x_{n}-x_{n+1} \Vert + \Vert x_{n+1}-U_{n} \Vert \\ &\quad{}+ \bigl\Vert \alpha _{n} f(y_{n})+(1-\alpha _{n})\mathcal{B}_{1} x_{n}- \mathcal{B}_{1} x_{n} \bigr\Vert \\ &= \Vert x_{n}-x_{n+1} \Vert + \Vert x_{n+1}-U_{n} \Vert +\alpha _{n} \bigl\Vert f(y_{n})- \mathcal{B}_{1} x_{n} \bigr\Vert , \end{aligned}$$

from (18), (22), and condition (ii), we obtain

$$\begin{aligned} \Vert x_{n}-\mathcal{B}_{1} x_{n} \Vert \rightarrow 0 \quad\text{as } n\rightarrow \infty . \end{aligned}$$

(23)

Consider

$$\begin{aligned} \Vert U_{n}-\mathcal{B}_{1} U_{n} \Vert & \leq \Vert U_{n}-x_{n} \Vert + \Vert x_{n}- \mathcal{B}_{1} x_{n} \Vert + \Vert \mathcal{B}_{1} x_{n}-\mathcal{B}_{1} U_{n} \Vert \\ &\leq \Vert U_{n}-x_{n} \Vert + \Vert x_{n}-\mathcal{B}_{1} x_{n} \Vert + \Vert x_{n}-U_{n} \Vert \\ &\leq 2 \Vert U_{n}-x_{n} \Vert + \Vert x_{n}-\mathcal{B}_{1} x_{n} \Vert . \end{aligned}$$

From (20) and (23), we have

$$\begin{aligned} \Vert U_{n}-\mathcal{B}_{1} U_{n} \Vert \rightarrow 0 \quad\text{as } n\rightarrow \infty . \end{aligned}$$

(24)

Applying the same arguments as for deriving (24), we also obtain

$$\begin{aligned} \Vert V_{n}-\mathcal{B}_{2} V_{n} \Vert \rightarrow 0 \quad\text{as } n\rightarrow \infty . \end{aligned}$$

Step 4: We claim that $\limsup_{n \to \infty}\langle f(y^{*})-x^{*},U_{n}-x^{*} \rangle \leq 0$, where $x^{*}=P_{\mathfrak{F}_{1}} f(y^{*})$.

First, take a subsequence $\{U_{n_{k}}\}$ of $\{U_{n}\}$ such that

$$\begin{aligned} \limsup_{n \to \infty}\bigl\langle f \bigl(y^{*}\bigr)-x^{*},U_{n}-x^{*} \bigr\rangle = \lim_{k \to \infty}\bigl\langle f\bigl(y^{*} \bigr)-x^{*},U_{n_{k}}-x^{*} \bigr\rangle . \end{aligned}$$

(25)

Since $\{x_{n}\}$ is bounded, there exists a subsequence $\{x_{n_{k}}\}$ of $\{x_{n}\}$ such that $x_{n_{k}}\rightharpoonup \hat{x}\in \zeta$ as $k\rightarrow \infty$. From (20), we obtain $U_{n_{k}}\rightharpoonup \hat{x}$ as $k\rightarrow \infty$. Since ζ dominates $x_{0}$, x̂ and $U_{n_{k}}$ are in ζ, then $(\hat{x},x_{o})$ and $(U_{n_{k}},x_{o})$ are in $E(G)$. From $E(G)=E(G)^{-1}$ and $(U_{n_{k}},x_{o})\in E(G)$, then $(x_{o}, U_{n_{k}})\in E(G)$. By the transitivity of G, $(\hat{x},x_{o})$ and $(x_{o}, U_{n_{k}})$ are in $E(G)$, we have $(\hat{x}, U_{n_{k}})\in E(G)$. From $E(G)=E(G)^{-1}$ and $(\hat{x}, U_{n_{k}})\in E(G)$, then $(U_{n_{k}},\hat{x})\in E(G)$.

Next, we need to show that $\hat{x}\in \mathfrak{F}_{1}=F(\mathcal{B}_{1})\cap VI(\zeta , \mathcal{S}_{1})$. Assume $\hat{x}\notin F(\mathcal{B}_{1})$. Then, we have $\hat{x}\neq \mathcal{B}_{1} \hat{x}$. By Opial’s condition, we obtain

$$\begin{aligned} \liminf_{k \to \infty} \Vert U_{n_{k}}-\hat{x} \Vert &< \liminf_{k \to \infty} \Vert U_{n_{k}}-\mathcal{B}_{1} \hat{x} \Vert \\ &\leq \liminf_{k \to \infty} \Vert U_{n_{k}}- \mathcal{B}_{1}U_{n_{k}} \Vert + \liminf_{k \to \infty} \Vert \mathcal{B}_{1}U_{n_{k}}- \mathcal{B}_{1} \hat{x} \Vert \\ &\leq \liminf_{k \to \infty} \Vert U_{n_{k}}-\hat{x} \Vert . \end{aligned}$$

This is a contradiction.

Therefore,

$$\begin{aligned} \hat{x}\in F(\mathcal{B}_{1}). \end{aligned}$$

(26)

Assume $\hat{x}\notin VI(\zeta ,\mathcal{S}_{1})$, then we obtain $\hat{x}\neq P_{\zeta}(I-\lambda _{1}\mathcal{S}_{1})\hat{x}$.

From Opial’s condition, $(U_{n_{k}},\hat{x})\in E(G)$ and (21), we have

$$\begin{aligned} \liminf_{k \to \infty} \Vert U_{n_{k}}-\hat{x} \Vert &< \liminf_{k \to \infty} \bigl\Vert U_{n_{k}}-P_{\zeta}(I- \gamma _{1} \mathcal{S}_{1})\hat{x} \bigr\Vert \\ &\leq \liminf_{k \to \infty} \bigl\Vert U_{n_{k}}-P_{\zeta}(I- \lambda _{1}\mathcal{S}_{1})U_{n_{k}} \bigr\Vert \\ &\quad{}+ \liminf_{k \to \infty} \bigl\Vert P_{\zeta}(I-\lambda _{1} \mathcal{S}_{1})U_{n_{k}}-P_{C}(I- \lambda _{1}\mathcal{S}_{1})\hat{x} \bigr\Vert \\ &\leq \liminf_{k \to \infty} \Vert U_{n_{k}}-\hat{x} \Vert . \end{aligned}$$

This is a contradiction.

Therefore,

$$\begin{aligned} \hat{x}\in VI(\zeta ,\mathcal{S}_{1}). \end{aligned}$$

(27)

By (26) and (27), this yields that

$$\begin{aligned} \hat{x}\in \mathfrak{F}_{1}= F(\mathcal{B}_{1}) \cap VI(\zeta , \mathcal{S}_{1}). \end{aligned}$$

(28)

Since $U_{n_{k}}\rightharpoonup \hat{x}$ as $k\rightarrow \infty$, (28) and Lemma 2.2, we can derive that

$$\begin{aligned} \limsup_{n \to \infty}\bigl\langle f \bigl(y^{*}\bigr)-x^{*},U_{n}-x^{*} \bigr\rangle &= \lim_{k \to \infty}\bigl\langle f\bigl(y^{*} \bigr)-x^{*},U_{n_{k}}-x^{*} \bigr\rangle \\ &=\bigl\langle f\bigl(y^{*}\bigr)-x^{*}, \hat{x}-x^{*}\bigr\rangle \\ &\leq 0. \end{aligned}$$

(29)

Following the same method as for (29), we obtain that

$$\begin{aligned} \limsup_{n \to \infty}\bigl\langle g \bigl(x^{*}\bigr)-y^{*},V_{n}-y^{*} \bigr\rangle \leq 0. \end{aligned}$$

(30)

Step 5: Finally, we prove that the sequences $\{x_{n}\}$ and $\{y_{n}\}$ converge strongly to $x^{*}=P_{\mathfrak{F}_{1}} f(y^{*})$ and $y^{*}=P_{\mathfrak{F}_{2}} g(x^{*})$, respectively.

By the firm nonexpansiveness of $P_{\zeta}$, $(x_{n},x_{n+1})$ and $(y_{n},y_{n+1})$ being in $E(G)$ we derive that

$$\begin{aligned} \bigl\Vert U_{n}-x^{*} \bigr\Vert ^{2}&= \bigl\Vert P_{\zeta}\tilde{u}_{n}-x^{*} \bigr\Vert ^{2} \\ &\leq \bigl\langle \tilde{u}_{n}-x^{*},U_{n}-x^{*} \bigr\rangle \\ &=\bigl\langle \alpha _{n}\bigl(f(y_{n})-x^{*} \bigr)+(1-\alpha _{n}) \bigl(\mathcal{B}_{1}x_{n}-x^{*} \bigr),U_{n}-x^{*} \bigr\rangle \\ &=\alpha _{n}\bigl\langle f(y_{n})-x^{*},U_{n}-x^{*} \bigr\rangle +(1-\alpha _{n}) \bigl\langle \mathcal{B}_{1}x_{n}-x^{*},U_{n}-x^{*} \bigr\rangle \\ &=\alpha _{n}\bigl\langle f(y_{n})-f\bigl(y^{*} \bigr),U_{n}-x^{*}\bigr\rangle +\alpha _{n} \bigl\langle f\bigl(y^{*}\bigr)-x^{*},U_{n}-x^{*} \bigr\rangle \\ &\quad{}+(1-\alpha _{n}) \bigl\Vert \mathcal{B}_{1}x_{n}-x^{*} \bigr\Vert \bigl\Vert U_{n}-x^{*} \bigr\Vert \\ &\leq \alpha _{n} a \bigl\Vert y_{n}-y^{*} \bigr\Vert \bigl\Vert U_{n}-x^{*} \bigr\Vert +\alpha _{n}\bigl\langle f\bigl(y^{*}\bigr)-x^{*},U_{n}-x^{*} \bigr\rangle \\ &\quad{}+(1-\alpha _{n}) \bigl\Vert x_{n}-x^{*} \bigr\Vert \bigl\Vert U_{n}-x^{*} \bigr\Vert \\ &\leq \frac{\alpha _{n}a}{2} \bigl\{ \bigl\Vert y_{n}-y^{*} \bigr\Vert ^{2}+ \bigl\Vert U_{n}-x^{*} \bigr\Vert ^{2} \bigr\} +\alpha _{n}\bigl\langle f \bigl(y^{*}\bigr)-x^{*},U_{n}-x^{*}\bigr\rangle \\ &\quad{}+\frac{(1-\alpha _{n})}{2} \bigl\{ \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2}+ \bigl\Vert U_{n}-x^{*} \bigr\Vert ^{2} \bigr\} \\ &=\frac{\alpha _{n}a}{2} \bigl\Vert y_{n}-y^{*} \bigr\Vert ^{2}+\frac{(1-\alpha _{n})}{2} \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2} \\ &\quad{}+ \biggl(\frac{\alpha _{n}a}{2}+\frac{(1-\alpha _{n})}{2} \biggr) \bigl\Vert U_{n}-x^{*} \bigr\Vert ^{2} \\ &\quad{}+\alpha _{n}\bigl\langle f\bigl(y^{*} \bigr)-x^{*},U_{n}-x^{*}\bigr\rangle \\ &=\frac{\alpha _{n}a}{2} \bigl\Vert y_{n}-y^{*} \bigr\Vert ^{2}+\frac{(1-\alpha _{n})}{2} \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2} \\ &\quad{}+ \biggl(\frac{1-\alpha _{n}(1-a)}{2} \biggr) \bigl\Vert U_{n}-x^{*} \bigr\Vert ^{2} \\ &\quad{}+\alpha _{n}\bigl\langle f\bigl(y^{*} \bigr)-x^{*},U_{n}-x^{*}\bigr\rangle , \end{aligned}$$

which yields

$$\begin{aligned} \bigl\Vert U_{n}-x^{*} \bigr\Vert ^{2}&\leq \frac{\alpha _{n}a}{1+\alpha _{n}(1-a)} \bigl\Vert y_{n}-y^{*} \bigr\Vert ^{2}+\frac{(1-\alpha _{n})}{1+\alpha _{n}(1-a)} \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2} \\ &\quad{}+\frac{\alpha _{n}}{1+\alpha _{n}(1-a)}\bigl\langle f\bigl(y^{*}\bigr)-x^{*},U_{n}-x^{*} \bigr\rangle . \end{aligned}$$

(31)

From the definition of $x_{n}$ and (31), we obtain

$$\begin{aligned} \bigl\Vert x_{n+1}-x^{*} \bigr\Vert ^{2}&\leq \delta _{n} \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2}+\eta _{n} \bigl\Vert P_{ \zeta}(I- \lambda _{1}\mathcal{S}_{1})x_{n}-x^{*} \bigr\Vert ^{2}+\mu _{n} \bigl\Vert U_{n}-x^{*} \bigr\Vert ^{2} \\ &\leq (1-\mu _{n}) \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2}+ \frac{\mu _{n}\alpha _{n}a}{1+\alpha _{n}(1-a)} \bigl\Vert y_{n}-y^{*} \bigr\Vert ^{2} \\ &\quad{}+\frac{\mu _{n}\alpha _{n}}{1+\alpha _{n}(1-a)}\bigl\langle f\bigl(y^{*}\bigr)-x^{*},U_{n}-x^{*} \bigr\rangle \\ &\quad{}+\frac{\mu _{n}(1-\alpha _{n})}{1+\alpha _{n}(1-a)} \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2} \\ &= \biggl(1-\frac{\mu _{n}\alpha _{n}(2-a)}{1+\alpha _{n}(1-a)} \biggr) \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2}+\frac{\mu _{n}\alpha _{n}a}{1+\alpha _{n}(1-a)} \bigl\Vert y_{n}-y^{*} \bigr\Vert ^{2} \\ &\quad{}+\frac{\mu _{n}\alpha _{n}}{1+\alpha _{n}(1-a)}\bigl\langle f\bigl(y^{*}\bigr)-x^{*},U_{n}-x^{*} \bigr\rangle . \end{aligned}$$

(32)

Similarly, as derived above, we also have

$$\begin{aligned} \bigl\Vert y_{n+1}-y^{*} \bigr\Vert ^{2}&\leq \biggl(1- \frac{\mu _{n}\alpha _{n}(2-a)}{1+\alpha _{n}(1-a)} \biggr) \bigl\Vert y_{n}-y^{*} \bigr\Vert ^{2}+\frac{\mu _{n}\alpha _{n}a}{1+\alpha _{n}(1-a)} \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2} \\ &\quad{}+\frac{\mu _{n}\alpha _{n}}{1+\alpha _{n}(1-a)}\bigl\langle g\bigl(x^{*}\bigr)-y^{*},V_{n}-y^{*} \bigr\rangle . \end{aligned}$$

(33)

From (32) and (33), we deduce that

$$\begin{aligned} & \bigl\Vert x_{n+1}-x^{*} \bigr\Vert ^{2}+ \bigl\Vert y_{n+1}-y^{*} \bigr\Vert ^{2} \\ &\quad\leq \biggl(1-\frac{\mu _{n}\alpha _{n}(2-a)}{1+\alpha _{n}(1-a)} \biggr) \bigl\{ \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2}+ \bigl\Vert y_{n}-y^{*} \bigr\Vert ^{2} \bigr\} \\ &\quad\quad{}+\frac{\mu _{n}\alpha _{n}a}{1+\alpha _{n}(1-a)} \bigl\{ \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2}+ \bigl\Vert y_{n}-y^{*} \bigr\Vert ^{2} \bigr\} \\ &\quad\quad{}+\frac{\mu _{n}\alpha _{n}}{1+\alpha _{n}(1-a)} \bigl( \bigl\langle f\bigl(y^{*} \bigr)-x^{*},U_{n}-x^{*}\bigr\rangle +\bigl\langle g\bigl(x^{*}\bigr)-y^{*},V_{n}-y^{*} \bigr\rangle \bigr) \\ &\quad= \biggl(1-\frac{\mu _{n}\alpha _{n}(2-a)}{1+\alpha _{n}(1-a)}+ \frac{\mu _{n}\alpha _{n}a}{1+\alpha _{n}(1-a)} \biggr) \bigl\{ \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2}+ \bigl\Vert y_{n}-y^{*} \bigr\Vert ^{2} \bigr\} \\ &\quad\quad{}+\frac{\mu _{n}\alpha _{n}}{1+\alpha _{n}(1-a)} \bigl( \bigl\langle f\bigl(y^{*} \bigr)-x^{*},U_{n}-x^{*}\bigr\rangle +\bigl\langle g\bigl(x^{*}\bigr)-y^{*},V_{n}-y^{*} \bigr\rangle \bigr) \\ &\quad= \biggl(1-\frac{2\mu _{n}\alpha _{n}(1-a)}{1+\alpha _{n}(1-a)} \biggr) \bigl\{ \bigl\Vert x_{n}-x^{*} \bigr\Vert ^{2}+ \bigl\Vert y_{n}-y^{*} \bigr\Vert ^{2} \bigr\} \\ &\quad\quad{}+\frac{\mu _{n}\alpha _{n}}{1+\alpha _{n}(1-a)} \bigl( \bigl\langle f\bigl(y^{*} \bigr)-x^{*},U_{n}-x^{*}\bigr\rangle +\bigl\langle g\bigl(x^{*}\bigr)-y^{*},V_{n}-y^{*} \bigr\rangle \bigr). \end{aligned}$$

Applying the condition $(ii)$, (29), (30), and Lemma 2.1, we can conclude that the sequences $\{x_{n}\}$ and $\{y_{n}\}$ converge strongly to $x^{*}=P_{\mathfrak{F}_{1}} f(y^{*})$ and $y^{*}=P_{\mathfrak{F}_{2}} g(x^{*})$, respectively. This completes the proof. □

Lemma 3.3

Let ζ be a nonempty, closed, and convex subset of a real Hilbert space H and let $G=(V(G),E(G))$ be a directed graph with $\zeta =V(G)$. Let $\Lambda :\zeta \rightarrow \zeta$ be edge preserving and Λ be a $G-\kappa$-strictly pseudocontractive. Then, the following conditions hold;

• (i) Λ is $G-\kappa$-strictly pseudocontractive ⇔ $(I-\Lambda )$ is $G- (\frac{1-\kappa}{2} )$-inverse strongly monotone;

• (ii) $G-VI(\zeta ,I-\Lambda )=F(\Lambda )$.

Proof

i) $(\Leftarrow )$ Let $(x,y)\in E(G)$.

Since Λ is edge preserving and $(x,y)\in E(G)$, then $(\Lambda x,\Lambda y)\in E(G)$.

Suppose that $M=I-\Lambda$ is $G- (\frac{1-\kappa}{2} )-$ inverse strongly monotone, i.e.,

$$\begin{aligned} \langle x-y,M x-M y \rangle \geq \biggl(\frac{1-\kappa}{2} \biggr) \Vert M x-M y \Vert ^{2}, \end{aligned}$$

for all $x,y\in \zeta$ with $(x,y)\in E(G)$. Then,

$$\begin{aligned} \Vert \Lambda x-\Lambda y \Vert ^{2}&= \bigl\Vert (I-M)x-(I-M)y \bigr\Vert ^{2} \\ &= \Vert Mx-My \Vert ^{2}+ \Vert x-y \Vert ^{2}-2 \langle x-y, Mx-My\rangle \\ &\leq \Vert Mx-My \Vert ^{2}+ \Vert x-y \Vert ^{2}-2 \biggl(\frac{1-\kappa}{2} \biggr) \Vert Mx-My \Vert ^{2} \\ &= \Vert x-y \Vert ^{2}+\kappa \Vert Mx-My \Vert ^{2}, \end{aligned}$$

for all $x,y\in \zeta$ with $(x,y)\in E(G)$.

Thus, Λ is a $G-\kappa$-strictly pseudocontractive mapping.

$(\Rightarrow )$ Conversely, suppose that $M=I-\Lambda$ and Λ is a $G-\kappa$-strictly pseudocontractive mapping.

Let $x,y\in \zeta$ with $(x,y)\in E(G)$, i.e.,

$$\begin{aligned} \Vert \Lambda x-\Lambda y \Vert ^{2}\leq \Vert x-y \Vert ^{2}+\kappa \Vert Mx-My \Vert ^{2}, \end{aligned}$$

for all $x,y\in \zeta$ with $(x,y)\in E(G)$. Then,

$$\begin{aligned} \Vert \Lambda x-\Lambda y \Vert ^{2}&= \bigl\Vert (I-M)x-(I-M)y \bigr\Vert ^{2} \\ &= \Vert Mx-My \Vert ^{2}+ \Vert x-y \Vert ^{2}-2 \langle x-y, Mx-My\rangle \\ &\leq \Vert x-y \Vert ^{2}+\kappa \Vert Mx-My \Vert ^{2}. \end{aligned}$$

Hence,

$$\begin{aligned} \langle x-y,M x-M y \rangle \geq \biggl(\frac{1-\kappa}{2} \biggr) \Vert M x-M y \Vert ^{2}, \end{aligned}$$

for all $x,y\in \zeta$ with $(x,y)\in E(G)$. This implies that M is a $G-\kappa$-strictly pseudocontractive mapping.

$ii)$ Let $\hat{x}\in G-VI(\zeta ,I-\Lambda )$.

From i) and Lemma 2.7, we have

$$ \hat{x}\in (I-\Lambda )^{-1}(0). $$

This implies that $(I-\Lambda )\hat{x}=0$.

Thus, $\hat{x}\in F(\Lambda )$. Therefore,

$$\begin{aligned} G-VI(\zeta ,I-\Lambda )\subseteq F(\Lambda ). \end{aligned}$$

(34)

Let $\breve{x}\in F(\Lambda )$ and $y\in \zeta$ with $(\breve{x},y)\in E(G)$.

Since $\Lambda \breve{x}=\breve{x}$, we have $(I-\Lambda )\breve{x}=0$.

This implies that $\breve{x}\in (I-\Lambda )^{-1}(0)$.

From Lemma 2.7 and $\breve{x}\in (I-\Lambda )^{-1}(0)$, we have

$$ \bigl\langle y-\breve{x},(I-\Lambda )\breve{x}\bigr\rangle =0, $$

for all $y\in \zeta$ with $(\breve{x},y)\in E(G)$. Then, $\breve{x}\in G-VI(\zeta ,I-\Lambda )$.

Therefore,

$$\begin{aligned} F(\Lambda )\subseteq G-VI(\zeta ,I-\Lambda ). \end{aligned}$$

(35)

From (34) and (35), we have

$$ G-VI(\zeta ,I-\Lambda )=F(\Lambda ). $$

 □

Corollary 3.4

Let ζ be a nonempty, closed, and convex subset of a real Hilbert space H and let $G=(V(G),E(G))$ be a directed graph with $\zeta =V(G)$. Let $E(G)$ be convex and G be transitive with $E(G)=E(G)^{-1}$. For every $i=1,2$, let $\mathcal{A}_{i}:\zeta \rightarrow \zeta$ be a $G-\kappa$-strictly pseudocontractive mapping with $F(\mathcal{A}_{i})\neq \emptyset$ and let $g,f:H\rightarrow H$ be an $a_{g}$ and $a_{f}-G$-contraction mapping with $a=\max \{a_{g},a_{f}\}$. For every $i=1,2$, let $\mathcal{T}_{i}:\zeta \rightarrow \zeta$ be a G-nonexpansive mapping and $\Lambda _{i}:\zeta \rightarrow \zeta$ be a $G-\kappa _{i}$-strictly pseudocontractive mapping with $k=\max \{\kappa _{1},\kappa _{2}\}$. Define a mapping $\mathcal{B}_{i}:\zeta \rightarrow \zeta$ by $\mathcal{B}_{i}x=\mathcal{T}_{i}((1-b)I+b\Lambda _{i})x$ for all $x\in \zeta$ and $i=1,2$, $b\in (0,1-k)$. Assume that $\mathfrak{F}_{i}=F(\mathcal{B}_{i})\cap F(\mathcal{A}_{i})\neq \emptyset$ for all $i=1,2$ with $F(\mathcal{B}_{i})\times F(\mathcal{B}_{i})\subseteq E(G)$ and $G-VI(\zeta ,\mathcal{S}_{i})\times G-VI(\zeta ,\mathcal{S}_{i}) \subseteq E(G)$ for all $i=1,2$ and there exists $x_{0},y_{0}\in \zeta$ such that $(x_{0},x_{0})$ and $(y_{0},y_{0})$ are in $E(G)$ and $\zeta =V(G)$ dominates $x_{0}$ and $y_{0}$. Let the sequences $\{x_{n}\}$ and $\{y_{n}\}$ be generated by $x_{0},y_{0}\in \zeta$ and

$$ \textstyle\begin{cases} x_{n+1} = \delta _{n} x_{n}+\eta _{n} P_{\zeta}(I-\lambda _{1}(I- \mathcal{A}_{1}))x_{n}+\mu _{n}P_{\zeta}(\alpha _{n} f(y_{n})+(1- \alpha _{n})\mathcal{B}_{1} x_{n}), \\ y_{n+1} = \delta _{n} y_{n}+\eta _{n} P_{\zeta}(I-\lambda _{2}(I- \mathcal{A}_{2}))y_{n}+\mu _{n}P_{\zeta}(\alpha _{n} g(x_{n})+(1- \alpha _{n})\mathcal{B}_{2} y_{n}), \end{cases} $$

(36)

where $\{\delta _{n} \}, \{\eta _{n} \}, \{ \mu _{n} \}, \{\alpha _{n} \} \subseteq [0,1]$ with $\delta _{n}+\eta _{n}+\mu _{n}=1$ and $\lambda \in (0,2\alpha )$ with $\alpha =\min\{\frac{1-\kappa _{1}}{2}, \frac{1-\kappa _{2}}{2}\}$. Assume the following conditions hold;

• (i) $0 <\xi \leq \delta _{n},\eta _{n}$, $\mu _{n}\leq \bar{\xi}$ for all $n\in N$ and for some $\xi ,\bar{\xi}>0$;

• (ii) $\lim_{n \rightarrow \infty} \alpha _{n}=0$ and $\sum_{n=1}^{\infty} \alpha _{n} = \infty$;

• (iii) $\sum_{n=1}^{\infty} \lvert \delta _{n+1}-\delta _{n} \rvert < \infty$, $\sum_{n=1}^{\infty} \lvert \eta _{n+1}-\eta _{n} \rvert < \infty$, $\sum_{n=1}^{\infty} \lvert \alpha _{n+1}-\alpha _{n} \rvert < \infty$.

If $\{x_{n}\}$ dominates $P_{\mathfrak{F}_{1}} f(y_{0})$ and $\{y_{n}\}$ dominates $P_{\mathfrak{F}_{2}} g(x_{0})$, then $\{x_{n}\}$ converges strongly to $x^{*}=P_{\mathfrak{F}_{1}} f(y_{0})$ and $\{y_{n}\}$ converges strongly to $y^{*}=P_{\mathfrak{F}_{2}} g(x_{0})$, where $P_{\mathfrak{F}_{i}}$ is a metric projection on $\mathfrak{F}_{i}$, for all $i=1,2$.

Proof

From Theorem 3.2 and Lemma 3.3, we have the desired conclusion. □

4 Example

In this section, we give some examples to support our main theorem.

Example 4.1

Let $H=\mathbb{R}$ and $\zeta =[-10,10]$ and let $G=(V(G),E(G))$ be such that $V(G)=\zeta$, $E(G)=\{ (x,y) : xy>0\}$. Let $\mathcal{T}_{1}:[-10,10]\rightarrow [-10,10]$ be defined by

$$ \mathcal{T}_{1}x=\frac{\lvert x \rvert }{x}, $$

for all $x\in [-10,10]$ and let $\mathcal{T}_{2}:[-10,10]\rightarrow [-10,10]$ be defined by

$$ \mathcal{T}_{2}x = \textstyle\begin{cases} \frac{1-x}{2};&x< 0, \\ 0.5;&x=0, \\ \frac{x+1}{2};&x>0, \end{cases} $$

for all $x\in [-10,10]$.

Let $\Lambda _{1}:[-10,10]\rightarrow [-10,10]$ be defined by

$$ \Lambda _{1}x=\operatorname{sgn}\bigl(\operatorname{sgn}(x)\bigr)= \textstyle\begin{cases} 1;&x\in (0,10], \\ 0;&x=0, \\ -1;&x\in [-10,0), \end{cases} $$

for all $x\in [-10,10]$ and let $\Lambda _{2}:[-10,10]\rightarrow [-10,10]$ be defined by

$$ \Lambda _{2}x= \textstyle\begin{cases} \frac{2x+3}{5};&x\in (0,10], \\ 0;&x=0, \\ \frac{2x-11}{5};&x\in [-10,0), \end{cases} $$

for all $x\in [-10,10]$.

Let $\mathcal{S}_{1}:[-10,10]\rightarrow \mathbb{R}$ be defined by

$$ \mathcal{S}_{1}x= \textstyle\begin{cases} \frac{x+5}{12}-\frac{1}{2};&x\in (0,10], \\ 0;&x=0, \\ \frac{x+5}{12}+\frac{1}{2};&x\in [-10,0), \end{cases} $$

for all $x\in [-10,10]$, and let $\mathcal{S}_{2}:[-10,10]\rightarrow \mathbb{R}$ be defined by

$$ \mathcal{S}_{2}x= \textstyle\begin{cases} \frac{2(x-1)}{\sqrt{19}};&x\in (0,10], \\ 0;&x=0, \\ \frac{2(x+1)}{\sqrt{19}};&x\in [-10,0). \end{cases} $$

For every $i=1,2$, let $\mathcal{B}_{i}:\zeta \rightarrow \zeta$ be defined by $\mathcal{B}_{i}x=\mathcal{T}_{i}(0.5I+0.5\Lambda _{i})x$ for all $x\in [-10,10]$. Let $f,g:\mathbb{R}\rightarrow \mathbb{R}$ be defined by

$$ f(x)=\frac{\operatorname{sgn}(x)}{2}= \textstyle\begin{cases} \frac{1}{2};&x>0, \\ 0;&x=0, \\ -\frac{1}{2};&x< 0 \end{cases} $$

and

$$ g(x)=\frac{\operatorname{sgn}(x)}{3}+\frac{1}{2}= \textstyle\begin{cases} \frac{5}{6};&x>0, \\ 0;&x=0, \\ \frac{1}{6};&x< 0. \end{cases} $$

Let the sequences $\{x_{n}\}$ and $\{y_{n}\}$ be generated by $x_{0},y_{0}\in \zeta$ and

$$ \textstyle\begin{cases} x_{n+1} = (\frac{n}{3n+2}) x_{n}+(\frac{n+\frac{1}{2}}{3n+2}) P_{ \zeta}(I-0.7\mathcal{S}_{1})x_{n}+ (\frac{n+\frac{3}{2}}{3n+2})P_{ \zeta}(\frac{1}{8n} f(y_{n})+(1-\frac{1}{8n})\mathcal{B}_{1} x_{n}), \\ y_{n+1} = (\frac{n}{3n+2}) y_{n}+(\frac{n+\frac{1}{2}}{3n+2}) P_{ \zeta}(I-0.5\mathcal{S}_{2})y_{n}+(\frac{n+\frac{3}{2}}{3n+2}) P_{ \zeta}(\frac{1}{8n} g(x_{n})+(1-\frac{1}{8n})\mathcal{B}_{2} y_{n}). \end{cases} $$

Then, the sequences $\{x_{n}\}$ and $\{y_{n}\}$ converge strongly to 1.

Solution. For every $i=1,2$, it is clear that $\mathcal{S}_{i}^{-1}(0)\neq \emptyset$, since $1\in \mathcal{S}_{i}^{-1}(0)$, $E(G)=E(G)^{-1}$ and $E(G)$ is convex. By the definitions of $\mathcal{T}_{1}$, $\mathcal{T}_{2}$, f, g, $\mathcal{S}_{1}$, $\mathcal{S}_{2}$, $\Lambda _{1}$, and $\Lambda _{2}$, we have $\mathcal{T}_{1}$ and $\mathcal{T}_{2}$ are G-nonexpansive mappings, f and g are G-contraction mappings, $\mathcal{S}_{1}$ and $\mathcal{S}_{2}$ are G-12, $\frac{\sqrt{19}}{2}$-inverse strongly monotone mappings, respectively, $\Lambda _{1}$ and $\Lambda _{2}$ are G-$\frac{1}{2}$, $\frac{1}{3}$-strictly pseudocontractive mappings, respectively. However, $\mathcal{T}_{1}$ and $\mathcal{T}_{2}$ are not nonexpansive mappings, as if we choose $x_{1}=1$, $y_{1}=-\frac{1}{5}$, $x_{2}=0$ and $y_{2}=1$, then we see that $\lvert \mathcal{T}_{1}x_{1}-\mathcal{T}_{1}y_{1} \rvert =2> \frac{6}{5}=\lvert x_{1}-y_{1} \rvert$ and $\lvert \mathcal{T}_{2}x_{2}-\mathcal{T}_{2}y_{2} \rvert =1.5>1= \lvert x_{2}-y_{2} \rvert$.

f and g are not contraction mappings, as if we choose $x=\frac{1}{4}$ and $y=-\frac{1}{5}$, then we see that $\lvert f(x)-f(y) \rvert =1>\frac{7}{10}=\lvert x-y \rvert$ and $\lvert g(x)-g(y) \rvert =\lvert \frac{5}{6}-\frac{1}{6} \rvert = \frac{4}{6}>\frac{9}{20}=\lvert \frac{1}{4}+\frac{1}{5} \rvert = \lvert x-y \rvert$.

$\mathcal{S}_{1}$ and $\mathcal{S}_{2}$ are not 12, $\frac{\sqrt{19}}{2}$-inverse strongly monotone mappings, respectively. If $x=2$ and $y=10$ with $(2,10)\in E(G)$, then

$$\begin{aligned} \bigl\langle \mathcal{S}_{1}(2)-\mathcal{S}_{1}(10), 2-10 \bigr\rangle =5.33< 5.38=12 \bigl\lvert \mathcal{S}_{1}(2)- \mathcal{S}_{1}(10) \bigr\rvert ^{2}. \end{aligned}$$

If $x=10$ and $y=8$ with $(10,8)\in E(G)$, then

$$\begin{aligned} \bigl\langle \mathcal{S}_{2}(10)-\mathcal{S}_{2}(8), 10-8 \bigr\rangle =\biggl\langle \frac{18}{\sqrt{19}}, 2\biggr\rangle =8.26< 37.16= \frac{\sqrt{19}}{2}\bigl\lvert \mathcal{S}_{2}(10)-\mathcal{S}_{2}(8) \bigr\rvert ^{2}. \end{aligned}$$

$\Lambda _{1}$ and $\Lambda _{2}$ are not $\frac{1}{2}$, $\frac{1}{3}$-strictly pseudocontractive mappings, respectively, as if we choose $x=1$ and $y=-\frac{1}{5}$, then we have

$$\begin{aligned} \vert \Lambda _{1} x-\Lambda _{1} y \vert ^{2}=4> 1.76&= \biggl\vert 1+\frac{1}{5} \biggr\vert ^{2}+\frac{1}{2} \biggl\vert 1-\Lambda _{1}(1)+ \frac{1}{5}+\Lambda _{1} \biggl(-\frac{1}{5} \biggr) \biggr\vert ^{2} \end{aligned}$$

and

$$\begin{aligned} \vert \Lambda _{2}x-\Lambda _{2}y \vert ^{2}=10.76>2.88= \biggl\vert 1+\frac{1}{5} \biggr\vert ^{2}+\frac{1}{3} \biggl\vert (I-\Lambda _{2}) (1)-(I-\Lambda _{2}) \biggl(- \frac{1}{5}\biggr) \biggr\vert ^{2}. \end{aligned}$$

By the definitions of $\mathcal{S}_{i}$, $\mathcal{T}_{i}$, and $\Lambda _{i}$ for every $i=1,2$, we have $1=F(\mathcal{B}_{i})\cap G-VI(\zeta ,\mathcal{S}_{i})$. We observed that the parameters satisfy all the conditions of Theorem 3.2. Hence, we can conclude that the sequences $\{x_{n}\}$ and $\{y_{n}\}$ converge strongly to 1.

Newton’s Method is a mathematical tool often used in numerical analysis, which serves to approximate numerical solutions (i.e., x-intercepts, zeros, or roots). Given a function $g(x)$ defined over the domain of real numbers x, and the derivative of said function $g'(x)$. If $x_{n}$ is an approximation of a solution of $g(x)=0$ and if $\acute{g}(x_{n})\neq 0$, the next approximation defined for each $n=0,1,2,\ldots$ by:

$$ x_{n+1}=x_{n}-\frac{g(x_{n})}{g'(x_{n})}, $$

(37)

where $x_{0}$ is an initial point, is the most popular, studied, and used method for generating a sequence $\{x_{n}\}$ approximating the solution.

Newton’s Method (37) is also an example of Picard iteration, for the equation

$$ x_{n+1}=Tx_{n}, $$

where $T=I-\frac{g}{g'}$. Several authors have used the Picard iteration for approximation of fixed points (see [5, 6, 9]).

Π is an important mathematic constant. The search for the accurate value of Π led not only to more accuracy, but also to the development of new concepts and techniques. Many researches have been trying to approximate the value of Π (see [2, 7]). By using our main result, we introduce a new method to approximate the value of Π as shown in the following example.

Example 4.2

Let $H=\mathbb{R}$ and $\zeta =[3,4]$ and let $G=(V(G),E(G))$ be a directed graph with $V(G)=\zeta$, $E(G)=\{ (x,y) : x,y\in [3,\frac{11}{3}]\}$. For an approximate value of Π, for every $i=1,2$, define $F_{i}:C\rightarrow H$ by $F_{i}(x)=\frac{x}{5i}-\frac{\Pi}{5i}$ and $F_{i}$ is a subdifferentiable. It is easy to show that $I- \frac{F_{i}}{F'_{i}}$ is a G-nonexpansive mapping.

For every $i=1,2$, define $\mathcal{T}_{i}:[3,4]\rightarrow [3,4]$ by

$$ \mathcal{T}_{i}x = \textstyle\begin{cases} x-\frac{F(x)}{\acute{F}(x)};&\text{if }x\in [3,4), \\ \frac{11}{3};&\text{if }x=4, \end{cases} $$

for all $x\in [3,4]$.

For every $i=1,2$, let $\Lambda _{i}:[3,4]\rightarrow [3,4]$ be defined by

$$ \Lambda _{i}x= \textstyle\begin{cases} \frac{2ix+\Pi}{2i+1};&\text{if }x\in [3,4), \\ \frac{11}{3};&\text{if }x=4, \end{cases} $$

for all $x\in [3,4]$.

For every $i=1,2$, let $\mathcal{S}_{i}:[3,4]\rightarrow \mathbb{R}$ be defined by

$$ \mathcal{S}_{i}x= \textstyle\begin{cases} 2i(\frac{x}{3\Pi}-\frac{1}{3});&\text{if }x\in [3,4), \\ \frac{11}{3};&\text{if }x=4, \end{cases} $$

for all $x\in [3,4]$.

For every $i=1,2$, let $\mathcal{B}_{i}:\zeta \rightarrow \zeta$ be defined by $\mathcal{B}_{i}x=\mathcal{T}_{i}(0.5I+0.5\Lambda _{i})x$ for all $x\in [3,4]$. Let $f,g:\mathbb{R}\rightarrow \mathbb{R}$ be defined by

$$ f(x)= \textstyle\begin{cases} \frac{2x}{3}+1;&\text{if }x\in [3,4], \\ \frac{11}{3};&\text{if }x\notin [3,4] \end{cases} $$

and

$$ g(x)= \textstyle\begin{cases} \frac{x+7}{3};&\text{if }x\in [3,4], \\ \frac{11}{3};&\text{if }x\notin [3,4]. \end{cases} $$

Let the sequences $\{x_{n}\}$ and $\{y_{n}\}$ be generated by $x_{0},y_{0}\in \zeta$ and

$$ \textstyle\begin{cases} x_{n+1} = (\frac{n}{3n+2}) x_{n}+(\frac{n+\frac{1}{2}}{3n+2}) P_{ \zeta}(I-0.7\mathcal{S}_{1})x_{n}+ (\frac{n+\frac{3}{2}}{3n+2})P_{ \zeta}(\frac{1}{8n} f(y_{n})+(1-\frac{1}{8n})\mathcal{B}_{1} x_{n}), \\ y_{n+1} = (\frac{n}{3n+2}) y_{n}+(\frac{n+\frac{1}{2}}{3n+2}) P_{ \zeta}(I-0.5\mathcal{S}_{2})y_{n}+(\frac{n+\frac{3}{2}}{3n+2}) P_{ \zeta}(\frac{1}{8n} g(x_{n})+(1-\frac{1}{8n})\mathcal{B}_{2} y_{n}). \end{cases} $$

(38)

Then, the sequences $\{x_{n}\}$ and $\{y_{n}\}$ converge strongly to Π.

Solution. For every $i=1,2$, it is clear that $\mathcal{S}_{i}^{-1}(0)\neq \emptyset$, since $\Pi \in \mathcal{S}_{i}^{-1}(0)$, $E(G)=E(G)^{-1}$ and $E(G)$ is convex. By the definitions of $\mathcal{T}_{1}$, $\mathcal{T}_{2}$, f, g, $\mathcal{S}_{1}$, $\mathcal{S}_{2}$, $\Lambda _{1}$, and $\Lambda _{2}$, we have $\mathcal{T}_{1}$ and $\mathcal{T}_{2}$ are G-nonexpansive mappings, f and g are G-contraction mappings, $\mathcal{S}_{1}$ and $\mathcal{S}_{2}$ are G-$\frac{3\Pi}{2}$, $\frac{3\Pi}{4}$-inverse strongly monotone mappings, respectively, $\Lambda _{1}$ and $\Lambda _{2}$ are G-$\frac{1}{15}$-strictly pseudocontractive mappings.

By the definitions of $\mathcal{S}_{i}$, $\mathcal{T}_{i}$, and $\Lambda _{i}$ for every $i=1,2$, we have $\Pi =F(\mathcal{B}_{i})\cap G-VI(\zeta ,\mathcal{S}_{i})$. We observed that the parameters satisfy all the conditions of Theorem 3.2. Hence, we can conclude that the sequences $\{x_{n}\}$ and $\{y_{n}\}$ converge strongly to Π.

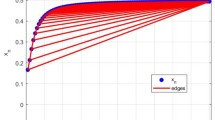
Using the algorithm (38), we have the numerical result to approximate the value of Π as shown in Table 1 and Fig. 1, where $x_{1}=y_{1}=4.00000$ and $n=N=150$.

Figure 1

The convergence of $\{x_{n}\}$ and $\{y_{n}\}$ with initial values $x_{1}=y_{1}=4.00000$ and $n=N=150$

Table 1 The values of $\{x_{n}\}$ and $\{y_{n}\}$ with initial values $x_{1}=y_{1}=4.00000$ and $n=N=150$

Remark 1

We show that $\mathcal{T}_{1}$ and $\mathcal{T}_{2}$ are not nonexpansive mappings, as if we choose $x_{1}=x_{2}=4$, $y_{1}=\frac{11}{3}$ and $y_{2}=3.5$, then we see that $\lvert \mathcal{T}_{1}x_{1}-\mathcal{T}_{1}y_{1} \rvert =0.526>0.3= \lvert x_{1}-y_{1} \rvert$ and $\lvert \mathcal{T}_{2}x_{2}-\mathcal{T}_{2}y_{2} \rvert =0.526>0.5= \lvert x_{2}-y_{2} \rvert$.

f and g are not contraction mappings, as if we choose $x_{1}=\frac{25}{10}$, $x_{2}=\frac{29}{10}$ and $y_{1}=y_{2}=3$, then we see that $\lvert f(x_{1})-f(y_{1}) \rvert =0.67>0.5=\lvert x_{1}-y_{1} \rvert$ and $\lvert g(x_{2})-g(y_{2}) \rvert =0.3>0.1=\lvert x_{2}-y_{2} \rvert$.

$\mathcal{S}_{1}$ and $\mathcal{S}_{2}$ are not $\frac{3\Pi}{2}$, $\frac{3\Pi}{4}$-inverse strongly monotone mappings, respectively. If $x_{1}=x_{2}=3.5$ and $y_{1}=y_{2}=3$ with $(3.5,3)\in E(G)$, then

$$\begin{aligned} \bigl\langle \mathcal{S}_{1}(x_{1})-\mathcal{S}_{1}(y_{1}), x_{1}-y_{1} \bigr\rangle =0.025< 0.047=\frac{3\Pi}{2} \bigl\lvert \mathcal{S}_{1}(x_{1})- \mathcal{S}_{1}(y_{1}) \bigr\rvert ^{2} \end{aligned}$$

and

$$\begin{aligned} \bigl\langle \mathcal{S}_{2}(x_{2})-\mathcal{S}_{2}(y_{2}), x_{2}-y_{2} \bigr\rangle =0.025< 9.42=\frac{3\Pi}{4}\bigl\lvert \mathcal{S}_{2}(x_{2})- \mathcal{S}_{2}(y_{2}) \bigr\rvert ^{2}. \end{aligned}$$

$\Lambda _{1}$ and $\Lambda _{2}$ are not $\frac{1}{15}$-strictly pseudocontractive mappings, as if we choose $x_{1}=x_{2}=4$ and $y_{1}=y_{2}=\frac{11}{3}$, then we have $\lvert \Lambda _{1}x_{1}-\Lambda _{1}y_{1} \rvert ^{2}=0.24>0.22= \lvert x_{1}-y_{1} \rvert ^{2}+\frac{1}{15}\lvert (I-\Lambda _{1})x_{1}-(I- \Lambda _{1})y_{1} \rvert ^{2}$, and $\lvert \Lambda _{2}x_{2}-\Lambda _{2}y_{2} \rvert ^{2}=0.32>0.22= \lvert x_{2}-y_{2} \rvert ^{2}+\frac{1}{15}\lvert (I-\Lambda _{1})x_{2}-(I- \Lambda _{1})y_{2} \rvert ^{2}$.

5 Conclusion

In this work, we introduce the definition of a $G-\kappa$-strictly pseudocontractive mapping that is different from a κ-strictly pseudocontractive mapping and prove a strong convergence theorem for finding the fixed points of two G-κ-strictly pseudocontractive mappings, two G-nonexpansive mappings, and two G-variational inequality problems in a Hilbert space endowed with a directed graph. However, we should like to note the following:

(1) Our result is proved without the Property G.

(2) We give some examples to support our main theorem and we have the numerical result to approximate the value of Π as shown in Table 1 and Fig. 1.