Charged AdS black holes in 4D Einstein–Gauss–Bonnet massive gravity

Abstract

We investigate Einstein–Gauss–Bonnet–Maxwell massive gravity in 4D AdS background and find an exact black hole solution. The horizon structure of the black holes studied. Treating the cosmological constant as pressure and Gauss–Bonnet coupling parameters, and massive gravity parameters as variables we drive the first law of black hole thermodynamics. To study the global stability of the black holes we compute the Gibbs free energy. The local stability of the black hole is also studied through specific heat. We analyze the effects of graviton mass and Gauss–Bonnet coupling parameters on the phase transition of the black holes. Finally, the effects of graviton mass and massive gravity parameters on the Joule–Thomson expansion of the black hole are studied.

Appendix

The Ricci and Kretschmann scalar for the metric function (2.11) are given by

$$\begin{aligned} R= & {} -\frac{f_{{rr}}(r) r^{2}+4 f_{r}(r) r +2 f(r) -2}{r^{2}},\nonumber \\ K= & {} f_{{rr}}^{2}(r)+\frac{4 f_{r}^{2}(r)}{r^{2}}+\frac{4 (-1+f(r) )^{2}}{r^{4}}, \end{aligned}$$

(.1)

where we use $f(r)= e^{2A(r)}$, $f_{rr}(r)$ stands for $d^2f(r)/dr^2$ and $f_r(r)$ stands for df(r)/dr.

The differentiation of f(r) with respect to r is given by

$$\begin{aligned} f_{r}= & {} \frac{r}{\alpha }\left[ 1-\sqrt{ 1+4 \alpha \left\{ \frac{2 M}{r^{3}}-\frac{Q^{2}}{r^{4}}-\frac{1}{l^{2}}-\frac{m^{2} (2 c^{2} c_{2} +c c_{1} r )}{2 r^{2}} \right\} } \right] \nonumber \\{} & {} -\frac{r^{2} \Bigr [ -\frac{6 M}{r^{4}}+\frac{4 Q^{2}}{r^{5}}-\frac{m^{2} c c_{1}}{2 r^{2}}+\frac{m^{2} (2 c^{2} c_{2} +c c_{1} r )}{r^{3}}\Bigr ]}{\sqrt{1+4 \alpha \biggl \{ \frac{2 M}{r^{3}}-\frac{Q^{2}}{r^{4}}-\frac{1}{l^{2}}-\frac{m^{2} (2 c^{2} c_{2} +c c_{1} r )}{2 r^{2}} \biggl \}}}, \end{aligned}$$

(.2)

$$\begin{aligned} f_{rr}= & {} \frac{1}{\alpha }\Biggr [ 1-\sqrt{1+4 \alpha \biggl \{ \frac{2 M}{r^{3}}-\frac{Q^{2}}{r^{4}}-\frac{1}{l^{2}}-\frac{m^{2} (2 c^{2} c_{2} +c c_{1} r )}{2 r^{2}} \biggl \} }\Biggr ]\nonumber \\{} & {} +\frac{2 \alpha r^{2} \Bigr [ -\frac{6 M}{r^{4}}+\frac{4 Q^{2}}{r^{5}}-\frac{m^{2} c c_{1}}{2 r^{2}}+\frac{m^{2} (2 c^{2} c_{2} +c c_{1} r )}{r^{3}}\Bigr ]^{2}}{\Bigr [ 1+4 \alpha \Bigl \{ \frac{2 M}{r^{3}}-\frac{Q^{2}}{r^{4}}-\frac{1}{l^{2}}-\frac{m^{2} (2 c^{2} c_{2} +c c_{1} r )}{2 r^{2}}\Bigl \}\Bigr ]^{\frac{3}{2}}}\nonumber \\{} & {} +\frac{l (-2 c^{2} c_{2} m^{2} r^{2}-m^{2} c c_{1} r^{3}+4 Q^{2})}{r^{2} \sqrt{\Bigl \{ (-4 c^{2} c_{2} m^{2} r^{2}-2 m^{2} c c_{1} r^{3}+8 M r -4 Q^{2}) l^{2}-4 r^{4}\Bigl \} \alpha +r^{4} l^{2}}}. \end{aligned}$$

(.3)

Therefore, using (.2) and (.3), Ricci and Kretschmann scalar are given by

$$\begin{aligned} R= & {} \frac{6}{\alpha } \Biggr [ -1+{ \sqrt{1+\frac{8 \alpha M}{r^{3}}-\frac{4 \alpha Q^{2}}{r^{4}}-\frac{4 \alpha }{l^{2}}-\frac{4 \alpha m^{2} c^{2} c_{2}}{r^{2}}-\frac{2 \alpha m^{2} c c_{1}}{r}}}\Biggr ]\nonumber \\{} & {} - \frac{ \bigl ( -4 c^{2} c_{2} m^{2} r^{2}-m^{2} c c_{1} r^{3}+12 M r -8 Q^{2}\bigl )^{2} \alpha l^{3}}{2r^{2} \Bigr [ \bigl \{ (-4 c^{2} c_{2} m^{2} r^{2}-2 m^{2} c c_{1} r^{3}+8 M r -4 Q^{2}) l^{2}-4 r^{4}\bigl \} \alpha +r^{4} l^{2}\Bigr ]^{\frac{3}{2}} }\nonumber \\{} & {} -\frac{l \bigl ( -10 c^{2} c_{2} m^{2} r^{2}-3 m^{2} c c_{1} r^{3}+24 M r -12 Q^{2} \bigl )}{r^{2} \sqrt{ \bigl \{ (-4 c^{2} c_{2} m^{2} r^{2}-2 m^{2} c c_{1} r^{3}+8 M r -4 Q^{2}) l^{2}-4 r^{4}\bigl \} \alpha +r^{4} l^{2}}}, \end{aligned}$$

(.4)

$$\begin{aligned} K= & {} \Biggr [ \frac{1}{\alpha } \left[ {1-\sqrt{1+4 \alpha \left\{ \frac{2 M}{r^{3}}-\frac{Q^{2}}{r^{4}}-\frac{1}{l^{2}}-\frac{m^{2} (2 c^{2} c_{2} +c c_{1} r )}{2 r^{2}} \right\} }} \right] \nonumber \\{} & {} -\frac{4 r \Bigr [ -\frac{6 M}{r^{4}}+\frac{4 Q^{2}}{r^{5}}-\frac{m^{2} c c_{1}}{2 r^{2}}+\frac{m^{2} (2 c^{2} c_{2} +c c_{1} r )}{r^{3}}\Bigr ]}{\sqrt{1+4 \alpha \biggl \{ \frac{2 M}{r^{3}}-\frac{Q^{2}}{r^{4}}-\frac{1}{l^{2}}-\frac{m^{2} (2 c^{2} c_{2} +c c_{1} r )}{2 r^{2}}\biggl \}}} \nonumber \\{} & {} +\frac{2\alpha r^{2} \left[ -\frac{6 M}{r^{4}}+\frac{4 Q^{2}}{r^{5}}-\frac{m^{2} c c_{1}}{2 r^{2}}+\frac{m^{2} (2 c^{2} c_{2} +c c_{1} r )}{r^{3}}\right] ^{2} }{ \Bigr [ 1+4 \alpha \Bigl \{ \frac{2 M}{r^{3}}-\frac{Q^{2}}{r^{4}}-\frac{1}{l^{2}}-\frac{m^{2} (2 c^{2} c_{2} +c c_{1} r )}{2 r^{2}}\Bigl \}\Bigr ]^{\frac{3}{2}}}\nonumber \\{} & {} -\frac{r^{2} \Bigr [ \frac{24 M}{r^{5}}-\frac{20 Q^{2}}{r^{6}}+\frac{2 m^{2} c c_{1}}{r^{3}}-\frac{3 m^{2} (2 c^{2} c_{2} +c c_{1} r )}{r^{4}}\Bigr ]}{\sqrt{1+4 \alpha \Bigl \{ \frac{2 M}{r^{3}}-\frac{Q^{2}}{r^{4}}-\frac{1}{l^{2}}-\frac{m^{2} (2 c^{2} c_{2} +c c_{1} r )}{2 r^{2}}\Bigl \}}} \Biggr ]^{2} \nonumber \\{} & {} +\frac{4}{{r^{2}}} \Biggr [ \frac{r}{{\alpha }} \left[ 1-\sqrt{1+4 \alpha \left\{ \frac{2 M}{r^{3}}-\frac{Q^{2}}{r^{4}}-\frac{1}{l^{2}}-\frac{m^{2} (2 c^{2} c_{2} +c c_{1} r )}{2 r^{2}}\right\} }\right] \nonumber \\{} & {} -\frac{r^{2} \Bigr [ -\frac{6 M}{r^{4}}+\frac{4 Q^{2}}{r^{5}}-\frac{m^{2} c c_{1}}{2 r^{2}}+\frac{m^{2} (2 c^{2} c_{2} +c c_{1} r )}{r^{3}}\Bigr ]}{\sqrt{1+4 \alpha \Bigl \{ \frac{2 M}{r^{3}}-\frac{Q^{2}}{r^{4}}-\frac{1}{l^{2}}-\frac{m^{2} (2 c^{2} c_{2} +c c_{1} r )}{2 r^{2}}\Bigl \}}}\Biggr ]^{2} \nonumber \\{} & {} +\frac{1}{{\alpha ^{2}}}\Biggr [ 1-\sqrt{1+4 \alpha \Bigl \{ \frac{2 M}{r^{3}}-\frac{Q^{2}}{r^{4}}-\frac{1}{l^{2}}-\frac{m^{2} (2 c^{2} c_{2} +c c_{1} r )}{2 r^{2}}\Bigl \}}\Biggr ]^{2} \end{aligned}$$

(.5)

Fig. 20

$M=5$, $Q=1$, $c=1$, $c_1=-1$ and $c_2=1$. $m=0$ denoted by solid black line, $m=1$ denoted by dash red line, $m=2$ denoted by blue dash line, $m=3$ denoted by gold dash line

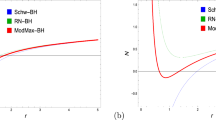
In Fig. 20, Kretschmann scalar is plotted. From the figure, it is clear that 4D Einstein–Gauss–Bonnet massive gravity black hole has a true singularity at $r=0$.