Skip to main content
SpringerLink
Log in

Generalization of the Kuramoto model to the Winfree model by a symmetry breaking coupling

  • Regular Article
  • Published:
The European Physical Journal Plus Aims and scope Submit manuscript

Abstract

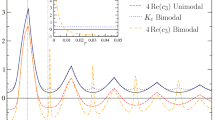
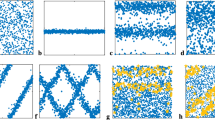
We construct a nontrivial generalization of the paradigmatic Kuramoto model by using an additional coupling term that explicitly breaks its rotational symmetry resulting in a variant of the Winfree model. Consequently, we observe the characteristic features of the phase diagrams of both the Kuramoto model and the Winfree model depending on the degree of the symmetry breaking coupling strength for unimodal frequency distribution. The phase diagrams of both the Kuramoto and the Winfree models resemble each other for symmetric bimodal frequency distribution for a range of the symmetry breaking coupling strength except for region shift and difference in the degree of spread of the macroscopic dynamical states and bistable regions. The dynamical transitions in the bistable states are characterized by an abrupt (first-order) transition in both the forward and reverse traces. For asymmetric bimodal frequency distribution, the onset of bistable regions depends on the degree of the asymmetry. Large degree of the symmetry breaking coupling strength promotes the synchronized stationary state, while a large degree of heterogeneity, proportional to the separation between the two central frequencies, facilitates the spread of the incoherent and standing wave states in the phase diagram for a low strength of the symmetry breaking coupling. We deduce the low-dimensional equations of motion for the complex order parameters using the Ott-Antonsen ansatz for both unimodal and bimodal frequency distributions. We also deduce the Hopf, pitchfork, and saddle-node bifurcation curves from the evolution equations for the complex order parameters mediating the dynamical transitions. Simulation results of the original discrete set of equations of the generalized Kuramoto model agree well with the analytical bifurcation curves.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

Data Availability Statement

The data sets on the current study are available from the corresponding author on reasonable request. [Authors’ comment: The homoclinic bifurcation curve is obtained by using XPPAUT software [43].]

References

  1. T. Stankovski, T. Pereira, P.V.E. McClintock, A. Stefanovska, Coupling functions: universal insights into dynamical interactionmechanisms. Rev. Mod. Phys. 89, 045001 (2017)

    Article  ADS  Google Scholar 

  2. A. Koseska, E. Volkov, J. Kurths, Transition from amplitude to oscillation death via turing bifurcation. Phys. Rev. Lett. 111, 024103 (2013)

    Article  ADS  Google Scholar 

  3. A. Zakharova, M. Kapeller, E. Schöll, Chimera death: symmetry breaking in dynamical networks. Phys. Rev. Lett. 112, 154101 (2014)

    Article  ADS  Google Scholar 

  4. T. Banerjee, Mean-field-diffusion?induced chimera death state. EuroPhys. Lett. 110, 69993 (2015)

    Article  Google Scholar 

  5. K. Premalatha, V.K. Chandrasekar, M. Senthilvelan, M. Lakshmanan, Impact of symmetry breaking in networks of globally coupled oscillators. Phys. Rev. E 91, 052915 (2015)

    Article  ADS  MathSciNet  Google Scholar 

  6. I. Schneider, M. Kapeller, S. Loss, A. Zakharova, B. Fiedler, E. Schöll, Stable and transient multicluster oscillation death in nonlocally coupled networks. Phys. Rev. E 92, 052915 (2015)

    Article  ADS  Google Scholar 

  7. K. Premalatha, V.K. Chandrasekar, M. Senthilvelan, M. Lakshmanan, Different kinds of chimera death states in nonlocally coupled oscillators. Phys. Rev. E 93, 052213 (2016)

    Article  ADS  Google Scholar 

  8. W. Zou, M. Zhan, J. Kurths, The impact of propagation and processing delays on amplitude and oscillation deaths in the presence of symmetry-breaking coupling, Chaos 27, 114303 (2017). https://doi.org/10.1063/1.5006750

    Article  ADS  MathSciNet  MATH  Google Scholar 

  9. R. Karnatak, R. Ramaswamy, A. Prasad, Amplitude death in the absence of time delays in identical coupled oscillators. Phys. Rev. E 76, 035201(R) (2007)

    Article  ADS  Google Scholar 

  10. A. Sharma, M.D. Shrimali, Amplitude death with mean-field diffusion. Phys. Rev. E 85, 057204 (2012)

    Article  ADS  Google Scholar 

  11. W. Zou, S. He, C. Yao, Stability of amplitude death in conjugate-coupled nonlinear oscillator networks. Appl. Math. Lett. 131, 108052 (2022)

    Article  MathSciNet  MATH  Google Scholar 

  12. A.T. Winfree, Biological rhythms and the behaviour of populations of coupled oscillators. J. Theor. Biol. 16, 15 (1967)

    Article  ADS  Google Scholar 

  13. A.T. Winfree, The Geomentry of Biological Time (Springer, New York, 1980)

    Book  Google Scholar 

  14. J. Buck, Synchronous rhythmic flashing of fireflies II. Q. Rev. Biol. 63, 265–289 (1988)

    Article  Google Scholar 

  15. Z. Néda, E. Ravasz, T. Vicsek, Y. Brechet, A.L. Barabasi, Physics of the rhythmic applause. Phys. Rev. E 61, 6987 (2000)

    Article  ADS  Google Scholar 

  16. Y. Kuramoto, Chemical Oscillations, Waves and Turbulence (Springer, Berlin, 1984)

    Book  MATH  Google Scholar 

  17. J.A. Acebron, L.L. Bonilla, C.J.P. Vicente, F. Ritort, R. Spigler, The Kuramoto model: a simple paradigm for synchronization phenomena. Rev. Mod. Phys. 77, 137 (2005)

    Article  ADS  Google Scholar 

  18. A. Pikovsky, M. Rosenblum, J. Kurths, Synchronization: A Universal Concept in Nonlinear Science (Cambridge University Press, Cambridge, 2001)

    Book  MATH  Google Scholar 

  19. C.S. Peskin, Mathematical Aspects of Heart Physiology (Courant Institute of Mathematical Sciences, New York, 1975)

    MATH  Google Scholar 

  20. S.P. Benz, C.J. Burroughs, Coherent emission from two-dimensional Josephson junction arrays. Appl. Phys. Lett. 58, 2162 (1991)

    Article  ADS  Google Scholar 

  21. M. Rohden, A. Sorge, M. Timme, D. Witthaut, Self organized synchronization in decentralized power grids. Phys. Rev. Lett. 109, 064101 (2012)

    Article  ADS  Google Scholar 

  22. V.K. Chandrasekar, M. Manoranjani, and Shamik Gupta Kuramoto model in the presence of additional interactions that break rotational symmetry. Phys. Rev. E 102, 012206 (2020)

    Article  ADS  MathSciNet  Google Scholar 

  23. R. Gallego, E. Montbrio, D. Pazo, Synchronization scenarios in the Winfree model of coupled oscillators. Phys. Rev. E 96, 042208 (2017)

    Article  ADS  Google Scholar 

  24. J.T. Ariaratnam, S.H. Stroatz, Phase diagram for the Winfree model of coupled nonlinear oscillators. Phys. Rev. Lett. 86, 19 (2001)

    Article  Google Scholar 

  25. F. Giannuzzi, D. Marinazzo, G. Nardulli, M. Pellicoro, S. Stramalia, Phase diagram of a generalized Winfree model. Phys. Rev. E 75, 051104 (2007)

    Article  ADS  Google Scholar 

  26. D. Pazo, E. Montbrio, Low-dimensional dynamics of populations of pulse-coupled oscillators. Phys. Rev. X 4, 011009 (2014)

    Google Scholar 

  27. S. Takagi, T. Ueda, Emergence and transitions of dynamic patterns of thickness oscillation of the plasmodium of the true slime mold Physarum polycephalum. Physica D 237, 420 (2008)

    Article  ADS  Google Scholar 

  28. Y. Murakami, H. Fukuta, Stability of a pair of planar counter-rotating vortices in a rectangular box. Fluid Dyn. Res. 31, 1 (2002)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  29. H. Fukuta, Y. Murakami, Vortex merging, oscillation, and quasiperiodic structure in a linear array of elongated vortices. Phys. Rev. E 57, 449 (1998)

    Article  ADS  Google Scholar 

  30. P. Meunier, T. Leweke, Elliptic instability of a co-rotating vortex pair. J. Fluid. Mech. 533, 125 (2005)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  31. G.N. Throumoulopoulos, H. Tasso, Magnetohydrodynamic counter-rotating vortices and synergetic stabilizing effects of magnetic field and plasma flow. Phys. Plasmas 17, 032508 (2010)

    Article  ADS  Google Scholar 

  32. K.T. Kapale, J.P. Dowling, Vortex phase qubit: generating arbitrary, counterrotating, coherent superpositions in Bose-Einstein condensates via optical angular momentum beams. Phys. Rev. Lett. 95, 173601 (2005)

    Article  ADS  Google Scholar 

  33. S. Thanvanthri, K.T. Kapale, J.P. Dowling, Arbitrary coherent superpositions of quantized vortices in Bose-Einstein condensates via orbital angular momentum of light. Phys. Rev. A 77, 053825 (2008)

    Article  ADS  Google Scholar 

  34. K. Czolczynski, P. Perlikowski, A. Stefanski, T. Kapitaniak, Synchronization of pendula rotating in different directions. Commun. Nonlin. Sci. Num. Simul. 17, 3658 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  35. M. Kapitaniak, K. Czolczynsk, P. Perlikowski, A. Stefanski, T. Kapitaniak, Synchronous states of slowly rotating pendula. Phys. Rep. 541, 1 (2014)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  36. K. Czolczynski, P. Perlikowski, A. Stefanski, T. Kapitaniak, Synchronization of slowly rotating pendulums. Int. J. Bifurc. Chaos 22, 1250128 (2012)

    Article  MATH  Google Scholar 

  37. K. Sathiyadevi, V.K. Chandrasekar, M. Lakshmanan, Emerging chimera states under nonidentical counter-rotating oscillators. Phys. Rev. E 105, 034211 (2022)

    Article  ADS  MathSciNet  Google Scholar 

  38. M. Tabor, Chaos and Integrability in Nonlinear Dynamics: An Introduction (John Wiley and Sons, New York, 1988)

    MATH  Google Scholar 

  39. E. Ott, T.M. Antonsen, Low dimensional behavior of large systems of globally coupled oscillators. Chaos 18, 037113 (2008)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  40. E. Ott, T.M. Antonsen, Long time evolution of phase oscillator systems. Chaos 19, 023117 (2009)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  41. R. Gallego, E. Montbrio, D. Pazo, Phys. Rev. E 96, 042208 (2017)

    Article  ADS  Google Scholar 

  42. S. Shinomoto, Y. Kuramoto, Prog. Theor. Phys. 75, 1105 (1986)

    Article  ADS  Google Scholar 

  43. B. Ermentrout, Simulating, Analyzing, and Animating Dynamical Systems: A Guide to XPPAUT for Researchers and Students (Society for Industrial & Applied Math, Philadelphia, 2002)

    Book  MATH  Google Scholar 

Download references

Acknowledgements

The work of V.K.C. is supported by the DST-CRG Project under Grant No. CRG/2020/004353 and DST, New Delhi for computational facilities under the DST-FIST program (SR/FST/PS- 1/2020/135)to the Department of Physics. M.M. thanks the Department of Science and Technology, Government of India, for providing financial support through an INSPIRE Fellowship No. DST/INSPIRE Fellowship/2019/IF190871. S.G. acknowledges support from the Science and Engineering Research Board (SERB), India under SERB-TARE scheme Grant No. TAR/2018/000023 and SERB-MATRICS scheme Grant No. MTR/2019/000560. He also thanks ICTP—The Abdus Salam International Centre for Theoretical Physics, Trieste, Italy for support under its Regular Associateship scheme. DVS is supported by the DST-SERB-CRG Project under Grant No. CRG/2021/000816.

Author information

Authors and Affiliations

  1. Department of Physics, Center for Nonlinear Science and Engineering, School of Electrical and Electronics Engineering, SASTRA Deemed University, Thanjavur, 613 401, India

    M. Manoranjani & V. K. Chandrasekar

  2. Department of Theoretical Physics, Tata Institute of Fundamental Research, Mumbai, 400005, India

    Shamik Gupta

  3. Department of Physics, Indian Institute of Science Education and Research, Thiruvananthapuram, 695016, India

    D. V. Senthilkumar

Authors
  1. M. Manoranjani
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Shamik Gupta
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. D. V. Senthilkumar
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. V. K. Chandrasekar
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to V. K. Chandrasekar.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Manoranjani, M., Gupta, S., Senthilkumar, D.V. et al. Generalization of the Kuramoto model to the Winfree model by a symmetry breaking coupling. Eur. Phys. J. Plus 138, 144 (2023). https://doi.org/10.1140/epjp/s13360-023-03760-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1140/epjp/s13360-023-03760-5

Search

Navigation