Long timescale behavior of large self-modulation of electromagnetic wave caused by vacuum nonlinearity

Abstract

The vacuum is expected to exhibit electromagnetic nonlinearity via virtual electron–positron pairs. An electromagnetic wave is modulated. The self-modulation has been believed to be vanishingly small. However, a large self-modulation can appear in a long timescale, even though the intensity of electromagnetic wave is not extremely strong. To demonstrate this assertion, we theoretically consider standing electromagnetic waves with modest intensity confined in a two-dimensional rectangular cavity. We first calculate in a short timescale by applying a perturbative linear approximation. We show a secular term. Then, beyond the linear approximation, we derive differential equations for slowly varying amplitude and phase in a long timescale. A large self-modulation of polarization, phase, and energy ratio of each mode are obtained by solving the equations. In particular, the energy ratio is classified into three types. Namely, it keeps oscillating, eventually converges, or stays constant.

Graphical Abstract

Appendices

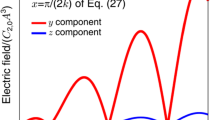
Appendix A: The function ${\mathcal {S}}$ appearing in Eq.(33)

In this “Appendix A,” any symbols are not related to physical meanings referred to in the main text.

Let y be an analytic function of x, we solve the following equation

$$\begin{aligned} y'^2=\left( 1-y^2\right) \left( 1-\lambda _1y-\lambda _2y^2\right) , \end{aligned}$$

(A.1)

with the initial values of $y(0)=0$ and $y'(0)=1$. The constants $\lambda _1$ and $\lambda _2$ are determined such that the second parentheses in the right-hand side does not have a root in $[-1,1]$. Such a region V is given by

$$\begin{aligned} V= {\left\{ \begin{array}{ll} \lambda _2<-\frac{\lambda _1^2}{4} &{} (\mid \lambda _1\mid \ge 2)\\ \lambda _2<\lambda _1+1 &{} (-2<\lambda _1\le 0) \\ \lambda _2<-\lambda _1+1 &{} (0<\lambda _1<2) \\ \end{array}\right. }. \end{aligned}$$

(A.2)

By defining a unique $\varphi$ for each x by

$$\begin{aligned} x=\int _0^{\varphi }\frac{\text {d}\theta }{\sqrt{1-\lambda _1\sin \theta -\lambda _2\sin ^2\theta }}, \end{aligned}$$

(A.3)

the unique solution $y(x)={\mathcal {S}}(x; \lambda _1, \lambda _2)$ is given by

$$\begin{aligned} {\mathcal {S}}(x; \lambda _1, \lambda _2)=\sin \varphi . \end{aligned}$$

(A.4)

The function ${\mathcal {S}}(x; \lambda _1, \lambda _2)$ can be expressed by Jacobi’s elliptic function $\text {sn}$ as follows. For given $(\lambda _1, \lambda _2)\in V$, we define a series of parameters. Let

$$\begin{aligned} \Delta= & {} \frac{\lambda _1}{1-\lambda _2+\sqrt{(1-\lambda _2)^2-\lambda _1^2}}, \nonumber \\ k= & {} \frac{\sqrt{\lambda _1^2+4\lambda _2}}{1+\lambda _2+\sqrt{(1-\lambda _2)^2-\lambda _1^2}}, \end{aligned}$$

(A.5)

$\Delta$ lies in $-1<\Delta <1$ and k is a pure imaginary number or lies in [0, 1). There is unique $x_0$ on the real axis and in the fundamental parallelogram such that

$$\begin{aligned} \text {sn}(x_0,k)=-\Delta , \ \ \text {cn}(x_0,k)=\sqrt{1-\Delta ^2}. \end{aligned}$$

(A.6)

Let

$$\begin{aligned} A=\sqrt{\frac{1-\Delta ^2}{1-k^2\Delta ^2}}, \end{aligned}$$

(A.7)

$A>0$ holds. Using these parameters, we obtain

$$\begin{aligned} {\mathcal {S}}(x;\lambda _1,\lambda _2)=\frac{\text {sn}(Ax+x_0,k)+\Delta }{\Delta \text {sn}(Ax+x_0,k)+1}. \end{aligned}$$

(A.8)

The function ${\mathcal {S}}(x; \lambda _1, \lambda _2)$ can be regarded as a generalization of Jacobi’s elliptic function $\text {sn}$.

Appendix B: Solution of oscillating $\alpha$

In the case that $\alpha$ oscillates, its value lies between the maximum value $\alpha _M$ and minimum value $\alpha _m$. Note that $0<\alpha _m<\alpha _M$. We perform a transformation of variable by

$$\begin{aligned} \alpha (T)=\frac{\alpha _M+\alpha _m}{2} +\frac{\alpha _M-\alpha _m}{2}h(T). \end{aligned}$$

(B.1)

The range of h is $h\in [-1,1]$ and Eq. (21) is transformed into

$$\begin{aligned} h'^2=({\mathscr {A}}+{\mathscr {B}}h+{\mathscr {C}}h^2) (1-h^2), \end{aligned}$$

(B.2)

where ${\mathscr {A}}, {\mathscr {B}},$ and ${\mathscr {C}}$ are constants, especially, ${\mathscr {A}}>0$.

We define $\varphi _0\in [0,2\pi )$ to satisfy $\sin \varphi _0=h(0)$. If $h(0)\ne \pm 1$, then $h'(0)\ne 0$ and we choose $\varphi _0$ to accord the signs of $\cos \varphi _0$ and $h'(0)$. Hence, $\varphi _0$ is uniquely determined. Let $\lambda _1=-{\mathscr {B}}/{\mathscr {A}}$ and $\lambda _2=-{\mathscr {C}}/{\mathscr {A}}$. By defining $T_0$ by

$$\begin{aligned} T_0=\int _0^{\varphi _0}\frac{\text {d}\theta }{\sqrt{1-\lambda _1\sin \theta -\lambda _2\sin ^2\theta }}, \end{aligned}$$

(B.3)

we obtain ${\mathcal {S}}(T_0; \lambda _1, \lambda _2)=h(0)$ and ${\mathcal {S}}'(T_0; \lambda _1, \lambda _2)=h'(0)/\sqrt{{\mathscr {A}}}$. Therefore,

$$\begin{aligned} h(T)={\mathcal {S}}(\sqrt{{\mathscr {A}}}T+T_0; \lambda _1, \lambda _2). \end{aligned}$$

(B.4)

The remaining task is to give the values of ${\mathscr {A}}, {\mathscr {B}},$ and ${\mathscr {C}}$ for each oscillating subtype. For subtype $O_1$,

$$\begin{aligned} {\mathscr {A}}&=(c_1+c_2)c_2 (\alpha _{1-}+\alpha _{2-}-2\alpha _{1+})\nonumber \\&\quad (\alpha _{1-}+\alpha _{2-}-2\alpha _{2+}),\nonumber \\ {\mathscr {B}}&=2(c_1+c_2)c_2 (\alpha _{1-}-\alpha _{2-})\nonumber \\&\quad (\alpha _{1-}+\alpha _{2-} -\alpha _{1+}-\alpha _{2+}),\nonumber \\ {\mathscr {C}}&=(c_1+c_2)c_2 (\alpha _{1-}-\alpha _{2-})^2. \end{aligned}$$

(B.5)

For subtype $O_2$,

$$\begin{aligned} \begin{aligned} {\mathscr {A}}&=(c_1+c_2)c_2 (\alpha _{2+}+\alpha _{1+}-2\alpha _{1-})\\&\quad (\alpha _{2+}+\alpha _{1+}-2\alpha _{2-}),\\ {\mathscr {B}}&=2(c_1+c_2)c_2 (\alpha _{2+}-\alpha _{1+})\\&\quad (\alpha _{2+}+\alpha _{1+} -\alpha _{1-}-\alpha _{2-}),\\ {\mathscr {C}}&=(c_1+c_2)c_2 (\alpha _{2+}-\alpha _{1+})^2. \end{aligned}\nonumber \\ \end{aligned}$$

(B.6)

For subtype $O_3$,

$$\begin{aligned} \begin{aligned} {\mathscr {A}}&=(c_1+c_2)c_2 (\alpha _{2+}+\alpha _{2-}-2\alpha _{1+})\\&\quad (\alpha _{2+}+\alpha _{2-}-2\alpha _{1-}),\\ {\mathscr {B}}&=2(c_1+c_2)c_2 (\alpha _{2+}-\alpha _{2-})\\&\quad \left( \alpha _{2+}+\alpha _{2-} -\alpha _{1+}-\alpha _{1-}\right) ,\\ {\mathscr {C}}&=(c_1+c_2)c_2 (\alpha _{2+}-\alpha _{2-})^2. \end{aligned}\nonumber \\ \end{aligned}$$

(B.7)