Two-particle superposition effects in two-slit interference

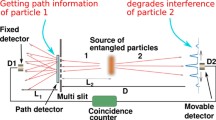
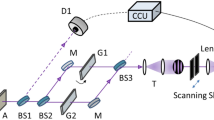
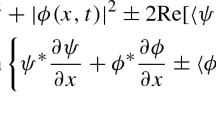Abstract

Entanglement can modify the interference patterns of multi-particle systems. We analyse, using the path integral formalism, a novel example of multi-particle interference and some unexplored aspects of this phenomenon by considering the two-slit arrangement with two distinguishable particles in a superposition state. The two components of entanglement, multiplicity of multi-particle terms and their coherence, can be studied separately by comparing the patterns of product, mixed and superposition states. This suggests a scheme to detect multi-particle superposition in some favourable cases. Moreover, the dependence of the effect on the entanglement degree, measured by the Schmidt number, is not a monotone.

Graphical abstract

Appendices

Appendix 1: Wave functions

We adapt the wave functions derived in [8] to the case of distinguishable particles with masses $m_1$ and $m_2$. The two slits are denoted A and B. After the slits the not normalized one-particle wave functions are $\psi _A+\psi _B$, ... Their explicit form is

$$\begin{aligned}&\psi _A(x,t)= {{\mathcal {C}}}_1(\sigma )e^{im_1x^2/2\hbar (t-t_s)}e^{-(\alpha _1(\sigma ) -i\beta _1(\sigma ) )x^2} \nonumber \\&\quad e^{-(\delta _1(\sigma )+i\gamma _1(\sigma ))x} \end{aligned}$$

(17)

and

$$\begin{aligned}&\psi _B(x,t)= {{\mathcal {C}}}_1(\sigma )e^{im_1x^2/2\hbar (t-t_s)}e^{-(\alpha _1(\sigma ) -i\beta _1(\sigma ) )x^2} \nonumber \\&\quad e^{(\delta _1(\sigma )+i\gamma _1(\sigma ))x} \end{aligned}$$

(18)

with $t_s$ the time at which the particles reach the slit,

$$\begin{aligned}&{{\mathcal {C}}}_1(\sigma )=\pi ^{-1/4} \left( \frac{1}{\sigma } + \frac{i\hbar \sigma t_s}{m_1} \right) ^{-1/2} \nonumber \\&\qquad \qquad \left( \frac{m_1}{2i\hbar (t-t_s)(D_1+iF_1)} \right) ^{1/2} \nonumber \\&\qquad \qquad \times e^{-x_0^2/2b_s^2}e^{G^2(D_1(\sigma )-iF_1(\sigma ))/4(D_1^2(\sigma )+F_1^2(\sigma ))} \nonumber \\ \end{aligned}$$

(19)

$$\begin{aligned}&\alpha _1(\sigma )=\frac{D_1(\sigma )H_1^2}{4(D_1^2(\sigma )+F_1^2(\sigma ))};\beta _1(\sigma )=\frac{F_1(\sigma )H_1^2}{4(D_1^2(\sigma )+F_1^2(\sigma ))} \nonumber \\ \end{aligned}$$

(20)

$$\begin{aligned}&\gamma _1(\sigma )=\frac{D_1(\sigma )GH_1}{2(D_1^2(\sigma )+F_1^2(\sigma ))};\delta _1(\sigma )=\frac{GH_1F_1(\sigma )}{2(D_1^2(\sigma )+F_1^2(\sigma ))} \nonumber \\ \end{aligned}$$

(21)

$$\begin{aligned}&D_1(\sigma )=\frac{1}{2b_s^2}+\frac{\sigma ^2}{\mu _1(\sigma )}; F_1(\sigma )=-\frac{\hbar \sigma ^4t_s}{m_1\mu _1(\sigma )}-\frac{m_1}{2\hbar (t-t_s)}\nonumber \\ \end{aligned}$$

(22)

and

$$\begin{aligned} G=\frac{x_0}{b_s^2}; H_1=\frac{m_1}{\hbar (t-t_s)}; \mu _1(\sigma )=2\left( 1+\frac{\hbar ^2 \sigma ^4 t^2}{m_1^2} \right) \nonumber \\ \end{aligned}$$

(23)

The rest of the one-particle wave functions can be obtained in the same way, introducing in the equations $m_1$ or $m_2$ and $\sigma$, ${\bar{\sigma }}$, $\chi$ or ${\bar{\chi }}$ according to the case considered.

Appendix 2: Dependence on the fixed detector

We analyse in this Appendix the dependence of the joint patterns on the choice of the fixed detection position. We vary it from $y=0$ to all the range of possible values. In Fig. 5 we include three of these curves.

The shape of the curves is very similar but the intensity of the maxima strongly depends on the value of the fixed point. For $y=0$ we have the larger values. When y increases the values of the peaks sharply decrease ($y=0.7$), reaching a minimum around $y=0.9$. For higher values of y the behaviour changes from a decrease to an increase of the values of the maxima. Around $y=1.7$ we reach a relative maximum of the peak values. We observe an oscillation of the maxima values of the patterns when the fixed point changes. The same oscillatory behaviour persists for larger values of y, being the peaks values progressively smaller. The same comportment is observed for other values of the degree of non-orthogonality (without modifying the coefficients of the superposition).

Fig. 5

Representation of P versus x as in Fig. 2 left, for the fixed points $y=0,0.7,1.7$ (red, black, blue)

We must discuss the connection of these oscillations with the concept of conditional interference, introduced in [1] and experimentally studied for light in [7]. A pattern shows conditionality when the location of the fringes depends in an oscillatory way on the relative positions of the detectors. As discussed in detail in [7] the interference patterns are in general the sum of conditional and non-conditional contributions, and only for some input light states the conditionality becomes manifest. In our case we do not observe conditional behaviour. The non-conditional terms are dominant. The prevalence of the last contributions can be easily understood. The relation between both types of contribution reflects a sort of complementarity between one- and two-particle interference [1]. As shown in Fig. 2 left, the superposition pattern is very close to that of the product state, indicating that the multi-particle contributions, and consequently the conditional ones, are small when compared to the non-conditional ones. The impossibility of observing conditionality does not imply the absence of conditional terms and, most important, the possibility of determining other multi-particle effects (as for example the modifications with respect to product state patterns) . It would be interesting, following the approach in [7], to find input states where the conditionality could be observed.