1 Introduction

Einstein’s gravity theory, is the most outstanding theory of twentieth century which passed a large amount of classical tests successfully. It explains dynamics of our solar system with acceptable accuracy. Existence of BH and gravitational waves, as the original predictions of this theory, have been detected by collaborations of LIGO and Virgo [1,2,3]. Based on the recent observational data it is confronted with some challenges [4,5,6]. A natural way for addressing the related failures is to extending this theory to the well-known alternative theories of gravity such as the quadratic curvature theories [7,8,9,10], the Levelock gravity [11,12,13,14], the braneworld scenario [15,16,17], the f(R) and f(T) theories of gravity [18,19,20,21,22] and, the scalar–tensor (ST) theory [23,24,25,26]. Briefly, we need to have an alternative theory of gravity to work as good as general relativity in the Solar System and, to address its shortcomings such as accelerated expansion of the Universe, inflation, formation of the large scale structures, dark matter and dark energy [27]. The ST modified gravity, in which the gravity is coupled to a scalar field, has appeared successful in the context of relativistic cosmology [28, 29]. It is well-known that dark energy and inflation are two outstanding subjects in modern cosmology and, gravitational ST theories are often utilized for describing them [30, 31]. Study of ST theories is motivated based on the facts that in one hand they can be viewed as the simplest extension of Einstein’s theory of gravity and, on the other hand they are advantages of quantum gravity theory in the low energy limit [32]. The ST gravity appeared successful under the Solar System tests and, its post-Newtonian limit is consistence with the general relativity [33, 34].

On the other hand, diverging the electric field and self energy at the position of the point-like charged particles are the famous challenges of Maxwell’s classical electrodynamics. Various models of nonlinear electromagnetic theories, as the natural extensions of Maxwell’s electrodynamics, are proposed with the aim of solving the aforementioned problems. The Born–Infeld, logarithmic, exponential, power-law, EH and other models of nonlinear electromagnetic theory have been used frequently for study of charged BHs in various spacetime dimensions [35,36,37,38,39,40]. Theories of nonlinear electrodynamics, in addition to the first-order Maxwell invariant \(\mathcal{{F}}=F^{\alpha \beta }F_{\alpha \beta }\), include its higher powers. It is believed that the additional therms are important when the electromagnetic fields are highly strength and, it is the case for the BHs with a large amount of electric charge. Therefore, by utilizing them, it is expected to get a more realistic description for the physical and thermodynamical properties of the BHs. Evidently, in the case of weak fields the higher order therms are negligible and the Maxwell’s theory is recovered [41, 42]. The EH electromagnetic theory, which includes firs and second powers of Maxwell invariant, is just the quantum corrected effective Lagrangian of electrodynamics. This theory is the advantage of quantum electrodynamics (QED) by taking weak field and slowly varying limit of the complete one-loop approximation [43,44,45]. Properties of the BTZ and BTZ-like BHs, four-dimensional modified RN, Einstein–Gauss–Bonnet AdS BHs and Gauss–Bonnet magnetic branes have been investigated in the presence of EH electrodynamics [43,44,45,46,47]. Through application of EH nonlinear electrodynamics it has been shown that finite self-energy can produced from a point like charged particle [48].

In the present work, I intend to pursue obtaining the new nonlinearly charged exact solutions in three-dimensional ST theory, studying thermodynamics and thermal stability of the new BHs under the influence of EH non-linear electrodynamics. This is motivated by the fact that lower dimensional models are easier for understanding the BHs’ quantum problems [49, 50]. Also, from the viewpoint of A(dS)/CFT duality, in comparison with the two and higher-dimensional spacetimes, the three-dimensional BHs are even more interesting systems [51].

We outlined this paper as follows: in the next section, by introducing the action of three-dimensional ST and EH nonlinear electrodynamics, it has been shown that the field equations of this theory cannot be solved directly because they are coupled strongly. Then by using the conformal transformations, we showed that the action of ST gravity can be translated to that of Einstein-dilaton gravity where, under some simple assumptions, the field equations are decoupled and can be solved easily. In Sect. 3, through obtaining the Einstein frame exact solutions and studying thermodynamic properties, we noticed that, based on some physical arguments, there are a set of relations which are mathematically inconsistent. In Sect. 4, with the aim of resolving the inconsistency problem encountered in Sect. 3, we supposed that through conformal transformation the nonlinearity parameter of EH electrodynamics transforms as \(a\rightarrow ae^{4\alpha \phi }\). By adding this transformation relation, we obtained the exact solutions which are free of any mathematical problem. Then, we studied thermodynamic properties and explored thermal stability of dilatonic EH BHs in Sect. 5. Section 6 is devoted to obtaining the Jordan frame exact solutions by applying the inverse transformations on the corresponding quantities in the Einstein frame. Then thermodynamics and thermal stability of three-dimensional ST BHs have been studied in the framework of canonical ensemble method. In Sect. 7, we summarized and discussed the results.

2 The general formalism

It is well-known that the ST gravity theory may be formulated in the Jordan frame or in its conformally related frame, known as the Einstein frame. Here, we start with the general form of Jordan frame action in which the Ricci scalar is multiplied by an arbitrary function of the scalar field. For a three-dimensional theory, it can be written as [52,53,54]

$$\begin{aligned} I^{(ST)}= & {} -\frac{1}{16\pi }\int \sqrt{-{\tilde{g}}}d^3x\left[ X(\psi )\;\tilde{\mathcal{{{R}}}}\right. \nonumber \\{} & {} \left. -Y(\psi ){\tilde{g}}^{\mu \nu }{\tilde{\nabla }}_\mu \psi {\tilde{\nabla }}^\nu \psi -Z(\psi )+L(\tilde{\mathcal{{F}}})\right] . \end{aligned}$$
(II.1)

Here, \({\tilde{g}}_{\mu \nu }\) and \(\tilde{\mathcal{{{R}}}}={\tilde{g}}^{\mu \nu }\tilde{\mathcal{{{R}}}}_{\mu \nu }\) are the metric and the Ricci scalar, \(X(\psi )\), \(Y(\psi )\) and \(Z(\psi )\) are multiplicative coefficients which are considered as functions of the scalar field \(\psi \). The covariant derivative compatible with \({\tilde{g}}_{\mu \nu }\) is denoted by \({\tilde{\nabla }}\) and, the EH nonlinear electromagnetic lagrangian is [55,56,57]

$$\begin{aligned} L(\tilde{\mathcal{{F}}})=-\tilde{\mathcal{{F}}}+a \tilde{\mathcal{{F}}}^2+\mathcal{{O}}\left( a^2\right) . \end{aligned}$$
(II.2)

It is considered as a function of \(\tilde{\mathcal{{F}}}={\tilde{F}}^{\alpha \beta }{\tilde{F}}_{\alpha \beta }\) with \({\tilde{F}}_{\alpha \beta }=\partial _\alpha A_\beta -\partial _\beta A_\alpha \), and \({\tilde{F}}^{\rho \lambda }={\tilde{g}}^{\rho \alpha }{\tilde{g}}^{\lambda \beta }{\tilde{F}}_{\alpha \beta }\). The coefficient a, known as the nonlinearity parameter, has the dimension of \(Length^2\).

Through variation of the action (II.1), the various equations of motion achieved as

$$\begin{aligned}{} & {} {\tilde{\nabla }}_\alpha \left( L'(\tilde{\mathcal{{F}}})\;\tilde{\mathcal{{F}}}^{\alpha \beta }\right) =0, \end{aligned}$$
(II.3)
$$\begin{aligned}{} & {} X(\psi ) \left( \tilde{\mathcal{{{R}}}}_{\alpha \beta }-\frac{1}{2}\tilde{\mathcal{{{R}}}}{\tilde{g}}_{\alpha \beta }\right) -\left( {\tilde{\nabla }}_\alpha {\tilde{\nabla }}_\beta -{\tilde{g}}_{\alpha \beta }{\tilde{\square }}\right) X(\psi )\nonumber \\{} & {} \quad =T^{(s)}_{\alpha \beta }+T^{(em)}_{\alpha \beta }, \end{aligned}$$
(II.4)

where, \( {\tilde{\square }}={\tilde{\nabla }}_\mu {\tilde{\nabla }}^\mu \), \(T^{(s)}_{\alpha \beta }\) and \(T^{(em)}_{\alpha \beta }\) are the scalar and electromagnetic energy-momentum tensors, and

$$\begin{aligned}{} & {} T^{(s)}_{\alpha \beta }=Y(\psi ){\tilde{\nabla }}_\alpha \psi {\tilde{\nabla }}_\beta \psi -\frac{1}{2}\left[ Z(\psi )+Y(\psi )\;({\tilde{\nabla }}\psi )^2\right] {\tilde{g}}_{\alpha \beta }, \end{aligned}$$
(II.5)
$$\begin{aligned}{} & {} T^{(em)}_{\alpha \beta }=\frac{1}{2}L(\tilde{\mathcal{{F}}})\;{\tilde{g}}_{\alpha \beta } -2L'(\tilde{\mathcal{{F}}})\;\tilde{\mathcal{{F}}}_{\alpha \nu }\tilde{\mathcal{{F}}}_\beta ^{\;\;\nu }, \end{aligned}$$
(II.6)
$$\begin{aligned}{} & {} 2Y(\psi ){\tilde{\square }}\psi =Z'(\psi )-Y'(\psi )({\tilde{\nabla }}\psi )^2\nonumber \\{} & {} \quad -\frac{X'(\psi )}{X(\psi )} \left[ 4{\tilde{\square }} X(\psi )+Y(\psi )({\tilde{\nabla }}\psi )^2 +3Z(\psi )\right. \nonumber \\{} & {} \quad \left. -3L(\tilde{\mathcal{{F}}})+4\tilde{\mathcal{{F}}}L'(\tilde{\mathcal{{F}}})\right] . \end{aligned}$$
(II.7)

The Jordan frame field equations are strongly coupled, such that finding the exact solutions is not easy. We try to decouple them by translating the action (II.1) to the well-known Einstein frame where the theory is known as the Einstein-dilaton gravity with the metric tensor \(g_{\mu \nu }\) [58]. It can be done by using the conformal transformations [59,60,61,62]. One can introduce the conformal transformations through [63]

$$\begin{aligned} {\tilde{g}}_{\mu \nu }=\Omega ^2g_{\mu \nu }, \end{aligned}$$
(II.8)

together with \({\tilde{F}}_{\mu \nu }={F}_{\mu \nu }\) and, \(\Omega \) is assumed to be a well-defined function of coordinates. Using the conformal transformations (II.8) in the ST action (II.1) we obtain [64]

$$\begin{aligned} \tilde{\mathcal{{{R}}}}=\Omega ^{-2}\mathcal{{{R}}}-4\Omega ^{-3}\square \Omega +2\Omega ^{-4}(\nabla \Omega )^2, \end{aligned}$$
(II.9)

with the Einstein frame’s scalar field \(\phi \), by assuming \(\psi =\psi (\phi )\), satisfying the following differential equation

$$\begin{aligned} \left( \frac{d \ln \Omega (\psi )}{d \psi }\right) ^2+\frac{Y(\psi )}{2X(\psi )}=2\left( \frac{d \phi }{d \psi } \right) ^2, \end{aligned}$$
(II.10)

and letting

$$\begin{aligned} V(\phi )= & {} Z(\psi )X^{-3}(\psi ), \quad \text {and}\quad \Omega (\psi )X(\psi )=1, \end{aligned}$$
(II.11)
$$\begin{aligned} L(\mathcal{{F}}, \phi )= & {} X^{-3}(\phi )L\left( X^{4}(\phi )\mathcal{{F}}\right) , \end{aligned}$$
(II.12)

we obtain

$$\begin{aligned} I= & {} -\frac{1}{16\pi }\int \sqrt{-g}d^3x \nonumber \\{} & {} \left[ \mathcal{{R}}-2g^{\mu \nu }\partial _\mu \phi \partial _\nu \phi -V(\phi )+L(\mathcal{{F}}, \phi )\right] . \end{aligned}$$
(II.13)

Note that, the Ricci scalar \(\mathcal{{R}}\) is defined as \(g^{\mu \nu }\mathcal{{R}}_{\mu \nu }\). The scalar potential \(V(\phi )\) is an unknown function of the scalar field \(\phi \). The electromagnetic Lagrangian \(L(\mathcal{{F}}, \phi )\) is considered as a function of \(\mathcal{{F}}=F^{\mu \nu }F_{\mu \nu }\) and \(\phi \). Also, \(F_{\mu \nu }=\partial _\mu A_\nu -\partial _\nu A_\mu \) and \(A_\mu \) is the electromagnetic potential. The action (II.13) is just the three-dimensional action of Einstein-dilaton theory and the scalar-coupled EH electrodynamics \(L(\mathcal{{F}}, \phi )\) must be chosen as [65,66,67]

$$\begin{aligned} L(\mathcal{{F}}, \phi )=-\mathcal{{F}}e^{-2\alpha \phi }+a\mathcal{{F}}^2e^{-10\alpha \phi }+\mathcal{{O}}\left( a^2\right) . \end{aligned}$$
(II.14)

Here, \(\alpha \) is the coupling constant between scalar and electromagnetic fields. Therefore, we have [68, 69]

$$\begin{aligned} \Omega (\phi )=e^{2\alpha \phi }. \end{aligned}$$
(II.15)

Note that (II.14) reduces to the scalar-coupled Maxwell Lagrangian if one take \(a=0\) [70].

The unknown functions \(X(\phi )\), \(Y(\phi )\) and \(Z(\phi )\) will be identified after calculating \(g_{\mu \nu }\), \(\phi \) and \(V(\phi )\). These will be done in the next sections.

3 Exact solutions and thermodynamics in the Einstein frame

Variation of Eq. (II.13) with respect to gravitational, electromagnetic and scalar fields, leads to the related field equations as

$$\begin{aligned}{} & {} \mathcal{{R}}_{\mu \nu }=V(\phi )g_{\mu \nu }+2\nabla _\mu \phi \nabla _\nu \phi \nonumber \\{} & {} \qquad \quad \quad -\mathcal{{F}}e^{-2\alpha \phi }\left( 1-3a\mathcal{{F}}e^{-8\alpha \phi }\right) g_{\mu \nu }\nonumber \\{} & {} \quad -2e^{-2\alpha \phi }\left( 2a\mathcal{{F}}e^{-8\alpha \phi }-1\right) F_{\mu \alpha }F_{\nu }^{\;\alpha }, \end{aligned}$$
(III.1)
$$\begin{aligned}{} & {} \nabla _\mu \left[ \left( 2a \mathcal{{F}} e^{-8\alpha \phi }-1\right) e^{-2\alpha \phi }F^{\mu \nu }\right] =0, \end{aligned}$$
(III.2)
$$\begin{aligned}{} & {} 4\Box \phi =\frac{d V(\phi )}{d\phi }-2\alpha \mathcal{{F}}e^{-2\alpha \phi }\left( 1-5a \mathcal{{F}} e^{-8\alpha \phi }\right) , \nonumber \\{} & {} \phi =\phi (r). \end{aligned}$$
(III.3)

We consider the following \((2+1)\)-dimensional circularly symmetric geometry for solving the field equations of this theory

$$\begin{aligned} ds^2= & {} g_{\mu \nu }dx^{\mu }dx^{\nu }=-f(r)dt^2\nonumber \\{} & {} +\frac{1}{f(r)}dr^2+r^2R^2(r)d\theta ^2, \end{aligned}$$
(III.4)

where, f(r) and R(r) are two unknown functions to be determined. It leads to the following differential equations

$$\begin{aligned} e_{tt}\equiv & {} f''(r)+\left( \frac{1}{r}+\frac{R'(r)}{R(r)}\right) f'(r)\nonumber \\{} & {} +2\left[ V(\phi )+a\mathcal{{F}}^2e^{-10\alpha \phi }\right] =0, \end{aligned}$$
(III.5)
$$\begin{aligned} e_{rr}\equiv & {} e_{tt}+2f(r)\left( \frac{R''(r)}{R(r)}\right. \nonumber \\{} & {} \left. +\frac{2R'(r)}{r R(r)}+2\phi '^2(r)\right) =0, \end{aligned}$$
(III.6)
$$\begin{aligned} e_{\theta \theta }\equiv & {} \frac{f'(r)}{r}+\frac{R''(r)}{R(r)}f(r)+\frac{R'(r)}{R(r)}\left( f'(r)+\frac{2}{r}f(r)\right) \nonumber \\{} & {} +V(\phi )-\mathcal{{F}}e^{-2\alpha \phi }+3a\mathcal{{F}}^2e^{-10\alpha \phi }=0. \end{aligned}$$
(III.7)

Here, prime means derivative with respect to the argument. Noting Eqs. (III.5) and (III.6) we obtain

$$\begin{aligned} \frac{R''(r)}{R(r)}+\frac{2}{r}\frac{R'(r)}{R(r)}+2\phi '^2(r)=0. \end{aligned}$$
(III.8)

Now, we consider a power-law solution as \(R(r)=\left( \frac{ r}{r_0}\right) ^{\sigma }\). Replacing into Eq.(III.8), we have

$$\begin{aligned} \phi (r)=\lambda \ln \left( \frac{b}{r}\right) , \quad \text {with} \quad \lambda =\sqrt{-\frac{\sigma (\sigma +1)}{2}}. \end{aligned}$$
(III.9)

Evidently, b must be positive and, \(\sigma \) must be restricted to the interval \(-1< \sigma \le 0\). Similar ansatz function has been used frequently for solving the problem of mathematical indeterminacy [71,72,73]. The only non-vanishing component of electromagnetic tensor \(F_{\mu \nu }\) is \(F_{tr}=-A'_t(r)\) and, one can easily show that \( \mathcal{{F}}=-2F_{tr}^2=-2(A'_t(r))^2\).

We return to the electromagnetic field equation (III.2). Noting Eq. (III.4), we have

$$\begin{aligned} 4a e^{-8\alpha \phi }F_{tr}^3+F_{tr}-\frac{q}{rR}e^{2\alpha \phi }=0, \end{aligned}$$
(III.10)

which, in terms of an integration constant q, can be solved to obtain \(F_{tr}\) in the following form

$$\begin{aligned} F_{tr}=\frac{q}{rR}e^{2\alpha \phi }-4a\frac{q^3}{r^3R^3}e^{-2\alpha \phi }+\mathcal{{O}}\left( a^2\right) , \end{aligned}$$
(III.11)

which recovers the corresponding quantity in the Einstein-EH theory, as the dilaton field is turned off [57]. The temporal component of electromagnetic four-potential can be obtained easily as

$$\begin{aligned} A_{t}= & {} -\int F_{tr} dr=\frac{q}{\sigma +2\alpha \lambda }\left( \frac{r_0}{r}\right) ^{\sigma }\left( \frac{b}{r}\right) ^{2\alpha \lambda }\nonumber \\{} & {} +\frac{4aq^3}{b^2\left( 2\alpha \lambda -3\sigma -2\right) }\left( \frac{r_0}{r}\right) ^{3\sigma }\left( \frac{b}{r}\right) ^{2-2\alpha \lambda }, \end{aligned}$$
(III.12)

An important point to be noted is that in order to \(A_t(r)\) be physically reasonable (i.e. zero at infinity) the following conditions are required to be satisfied

$$\begin{aligned}{} & {} 2\alpha \lambda +\sigma >0, \end{aligned}$$
(III.13)
$$\begin{aligned}{} & {} 3\sigma +2-2\alpha \lambda >0. \end{aligned}$$
(III.14)

By using (III.3) and (III.7) it is shown that f(r) and \(V(\phi )\) are governed by the following differential equations

$$\begin{aligned}{} & {} f'(r)+\frac{\sigma }{r}f(r)\nonumber \\{} & {} \quad +\frac{r}{\sigma +1}\left[ V(\phi )+\frac{2q^2}{b^2}\left( \frac{r_0}{b}\right) ^{2\sigma }\left( \frac{b}{r}\right) ^{2\left( \alpha \lambda +\sigma +1\right) }\right. \nonumber \\{} & {} \quad \left. -\frac{4aq^2}{b^4}\left( \frac{r_0}{b}\right) ^{4\sigma }\left( \frac{b}{r}\right) ^{2\left( -\alpha \lambda +2\sigma +2\right) }\right] =0, \end{aligned}$$
(III.15)
$$\begin{aligned}{} & {} \frac{dV(\phi )}{d\phi }-\frac{4\lambda }{\sigma +1}V(\phi )\nonumber \\{} & {} \quad +\frac{4q^2}{b^2}\left( \alpha -\frac{2\lambda }{\sigma +1}\right) \left( \frac{r_0}{b}\right) ^{2\sigma }Exp\left[ \left( 2\alpha +\frac{2\lambda }{\sigma +1} \right) \phi \right] \nonumber \\{} & {} \quad +\frac{8aq^4}{b^4}\left( \frac{2\lambda }{\sigma +1}+\alpha \right) \left( \frac{r_0}{b}\right) ^{4\sigma }\nonumber \\{} & {} \quad \times Exp\left[ \left( \frac{4\lambda }{\sigma +1}-2\alpha \right) \phi \right] =0. \end{aligned}$$
(III.16)

Since, in the absence of the dilaton field (e.i. \( \phi =0=\sigma \)) the action (II.13) reduces to that of Einstein-\(\Lambda \)-EH theory, we can fix the integration constant by use of \( V(\phi =0)=2\Lambda \). Therefore

$$\begin{aligned} V(\phi )= & {} 2\Lambda Exp\left( \frac{4\lambda \phi }{\sigma +1} \right) \nonumber \\{} & {} +2\Lambda _1Exp\left[ \left( 2\alpha +\frac{2(\sigma +1)}{\lambda } \right) \phi \right] \nonumber \\{} & {} +2\Lambda _2Exp\left[ \left( \frac{4(\sigma +1)}{\lambda }-2\alpha \right) \phi \right] , \end{aligned}$$
(III.17)

where

$$\begin{aligned} \Lambda _1= & {} -\frac{q^2(\alpha \lambda +\sigma )}{b^2(\alpha \lambda +2\sigma +1)}\left( \frac{r_0}{b}\right) ^{2\sigma },\quad \text {and}\quad \nonumber \\ \Lambda _2= & {} \frac{2aq^4(\alpha \lambda -\sigma )}{b^4(\alpha \lambda -3\sigma -2)}\left( \frac{r_0}{b}\right) ^{4\sigma }. \end{aligned}$$
(III.18)

By substituting Eq. (III.17) into Eq. (III.15), the metric function f(r) can be calculated as

$$\begin{aligned} f(r)= \left\{ \begin{array}{ll} -\frac{m}{r^\sigma }-\frac{2\Lambda b^2}{\Gamma }\left( \frac{r}{b}\right) ^{2\sigma +2} +\frac{2q^{2}}{\Gamma _1}\left( \frac{r_0}{b}\right) ^{2\sigma }\left( \frac{b}{r}\right) ^{2(\alpha \lambda +\sigma )}\\ \quad -\frac{8aq^{4}}{b^2\Gamma _2}\left( \frac{r_0}{b}\right) ^{4\sigma }\left( \frac{b}{r}\right) ^{2(-\alpha \lambda +2\sigma +1)},\quad \sigma \ne -\frac{2}{3},\\ -m r^{\frac{2}{3}}-6\Lambda (rb^2)^{\frac{2}{3}}\ln \left( \frac{r}{L}\right) \\ \quad +\frac{9q^{2}}{(\alpha -1)^2}\left( \frac{b}{r_0}\right) ^{\frac{4}{3}} \left( \frac{r}{b}\right) ^{\frac{2}{3}(2-\alpha )}\\ \quad -\frac{36aq^{4}}{b^2\alpha ^2}\left( \frac{b}{r_0}\right) ^{\frac{8}{3}}\left( \frac{r}{b}\right) ^{\frac{2}{3}(1+\alpha )}, \quad \sigma = -\frac{2}{3}, \end{array} \right. \end{aligned}$$
(III.19)

where, L is a dimensional constant, m is the constant of integration and,

$$\begin{aligned}{} & {} \Gamma =(\sigma +1)(3\sigma +2),\quad \text {and}\nonumber \\{} & {} \Gamma _1 =(2\alpha \lambda +\sigma )(\alpha \lambda +2\sigma +1),\;\;\text {and}\;\;\;\nonumber \\{} & {} \Gamma _2 = (\alpha \lambda -3\sigma -2)(2\alpha \lambda -3\sigma -2). \end{aligned}$$
(III.20)

Now, with the aim of checking validity of the TFL, we must calculate the related quantities through appropriate approaches.

Entropy of the BHs, as an important thermodynamic quantity, can be calculated by use of the Hawking-Bekenstein entropy-area law [74,75,76]. In our case, the entropy can be written as

$$\begin{aligned} S=\frac{\pi }{2r_0^{\sigma }}r_+^{\sigma +1}, \end{aligned}$$
(III.21)

where, \(r_+\) is the BH horizon radius which is the real root(s) of the relation \(f(r_+)=0\). Note that Eq. (III.21) recovers its standard form (i.e. \(S=\frac{\pi r_+}{2}\)) when the dilaton field is turned off by letting \(\sigma =0\) [35, 36].

Now, we calculate the BH total charge Q, regarding the electric flux at infinite distance (i.e. \(r\rightarrow \infty \)) from the BH [77,78,79,80,81]. By using the Gauss’s law one can write

$$\begin{aligned} Q=\frac{1}{4\pi }\int \left[ 1+4aF_{tr}^2\left( \frac{r}{b} \right) ^{4\alpha \lambda }\right] r^{^{2\alpha \lambda +\sigma +1}}F_{tr} d\Omega . \end{aligned}$$
(III.22)

Then, making use of Eq. (III.11) after some simple calculations we arrived at

$$\begin{aligned} Q=\frac{q}{2}, \end{aligned}$$
(III.23)

which is compatible with the charge of BTZ BHs obtained in the previous works [82, 83].

The BH total mass M, which is related to the mass parameter m, is an important quantity. In the case of three-dimensional BHs one can write [84,85,86]

$$\begin{aligned} M=\frac{\sigma +1}{8r_0^{\sigma }}m. \end{aligned}$$
(III.24)

The horizon electric potential \(\Phi \), measured with respect to a reference point, is determined by using the following relation [87,88,89]

$$\begin{aligned} \Phi =A_\mu \chi ^\mu |_{\text {reference}}-A_\mu \chi ^\mu |_{r=r_+}. \end{aligned}$$
(III.25)

Note that \(A_t\) is the temporal part of electromagnetic potential \(A_\mu \) and, \(\chi ^\mu =C\delta ^\mu _t\) is the null generator of the horizon and C is a constant coefficient to be fixed later. Noting Eqs. (III.12) and (III.25), we have

$$\begin{aligned} \Phi (r_+)= & {} \frac{Cq}{\sigma +2\alpha \lambda }\left( \frac{r_0}{r_+}\right) ^{\sigma }\left( \frac{b}{r_+}\right) ^{2\alpha \lambda }\nonumber \\{} & {} +\frac{4Caq^3}{b^2\left( 2\alpha \lambda -3\sigma -2\right) } \left( \frac{r_0}{r_+}\right) ^{3\sigma }\left( \frac{b}{r_+}\right) ^{2-2\alpha \lambda }.\nonumber \\ \end{aligned}$$
(III.26)

The BH temperature on the horizon, \(r=r_+\), is given noting the definition of surface gravity \(\kappa \) [90]. That is

$$\begin{aligned} T= & {} \frac{\kappa }{2\pi }=\frac{f'(r_+)}{4\pi }=\frac{-1}{2\pi }\left[ \frac{\Lambda r_+}{\sigma +1}\left( \frac{r_+}{b}\right) ^{2\sigma }\right. \nonumber \\{} & {} \left. +\frac{q^2r_+}{b^2(\alpha \lambda +2\sigma +1)}\left( \frac{r_0}{b}\right) ^{2\sigma }\left( \frac{b}{r_+}\right) ^{2(\alpha \lambda +\sigma +1)}\right. \nonumber \\{} & {} \left. +\frac{4aq^4r_+}{b^4(\alpha \lambda -3\sigma -2)}\left( \frac{r_0}{b}\right) ^{4\sigma }\left( \frac{b}{r_+}\right) ^{2(-\alpha \lambda +2\sigma +2)}\right] ,\nonumber \\{} & {} -1< \sigma \le 0. \end{aligned}$$
(III.27)

For checking validity of the TFL, we need to calculate Smarr mass relation. Thus, by using the requirement \(f(r_+)=0\) and, replacing m into Eq. (III.24), one obtains

$$\begin{aligned} M(r_+,q)= \left\{ \begin{array}{ll} -\frac{\sigma +1}{4}\left( \frac{b}{r_0}\right) ^{\sigma }\left[ \frac{\Lambda b^2}{\Gamma }\left( \frac{r_+}{b}\right) ^{2\sigma +2} -\frac{q^{2}}{\Gamma _1}\left( \frac{r_0}{b}\right) ^{2\sigma }\left( \frac{b}{r_+}\right) ^{2(\alpha \lambda +\sigma )}+\frac{4aq^{4}}{b^2\Gamma _2}\left( \frac{r_0}{b}\right) ^{4\sigma }\left( \frac{b}{r_+}\right) ^{2(2\sigma +1-\alpha \lambda )}\right] , \quad \sigma \ne -\frac{2}{3},\\ -6\Lambda (b)^{\frac{4}{3}}\ln \left( \frac{r}{L}\right) +\frac{9q^{2}r_+^{-\frac{2}{3}}}{(\alpha -1)^2}\left( \frac{b}{r_0}\right) ^{\frac{4}{3}} \left( \frac{r_+}{b}\right) ^{\frac{2}{3}(2-\alpha )}-\frac{36aq^{4}r_+^{-\frac{2}{3}}}{b^2\alpha ^2}\left( \frac{b}{r_0}\right) ^{\frac{8}{3}}\left( \frac{r_+}{b}\right) ^{\frac{2}{3}(1+\alpha )}, \quad \sigma = -\frac{2}{3}, \end{array} \right. \end{aligned}$$
(III.28)

Now, we calculate the intensive parameters T and \(\Phi \), conjugate to the BH entropy and charge, respectively. It is a matter of calculation to show that

$$\begin{aligned} \left( \frac{\partial M}{\partial S}\right) _Q=T,\;\; \text {for}\;\sigma = -\frac{2}{3},\;\;\text {and}\;\;\sigma \ne -\frac{2}{3}, \end{aligned}$$
(III.29)
$$\begin{aligned} \left( \frac{\partial M}{\partial Q}\right) _S=\frac{q(\sigma +1)(\alpha \lambda +2\sigma +1)^{-1}}{2\alpha \lambda +\sigma }\left( \frac{r_0}{r_+}\right) ^{\sigma }\left( \frac{b}{r_+}\right) ^{2\alpha \lambda } \end{aligned}$$
$$\begin{aligned}{} & {} \quad -\frac{8aq^3(\sigma +1)\left( \alpha \lambda -3\sigma -2\right) ^{-1}}{r_+^{2}\left( 2\alpha \lambda -3\sigma -2\right) }\left( \frac{r_0}{r_+}\right) ^{3\sigma }\left( \frac{r_+}{b}\right) ^{2\alpha \lambda },\;\;\nonumber \\{} & {} \text {for}\;\sigma = -\frac{2}{3},\;\;\text {and}\;\;\sigma \ne -\frac{2}{3}. \end{aligned}$$
(III.30)

Therefore, noting (III.26)

$$\begin{aligned} \left( \frac{\partial M}{\partial Q}\right) _S=\Phi (r_+), \end{aligned}$$
(III.31)

provided that

$$\begin{aligned} C=\frac{\sigma +1}{\alpha \lambda +2\sigma +1}=-\frac{2(\sigma +1)}{\alpha \lambda -3\sigma -2}, \end{aligned}$$
(III.32)

is chosen. It means that the TFL is valid in the form of

$$\begin{aligned} d M(S,Q)= T dS+\Phi dQ, \end{aligned}$$
(III.33)

if C is fixed to \(C=\frac{3(\sigma +1)}{5\sigma +3}\), and the following relation is fulfilled

$$\begin{aligned} 3\alpha \lambda +\sigma =0. \end{aligned}$$
(III.34)

Note that combination of Eqs. (III.13) and (III.34) gives \(\sigma >0\) which noting the allowed range of \(\sigma \) (i.e. \(-1< \sigma \le 0\)) is not acceptable. Also, the inequality (III.14) can be rewritten as \(2\alpha \lambda +\sigma <2(2\sigma +1)\), in which, noting (III.13), the left-hand side is positive while the right-hand side can be negative. Therefore the set of three requirements (III.13), (III.14) and (III.34) are mathematically inconsistent. In the next section, we solve this problem by use of a proposal.

4 The corrected exact solutions and thermodynamic quantities

In order to avoid mathematical inconsistency mentioned in the last section, we assume that under conformal transformations, the nonlinearity parameter a transforms as \(a\rightarrow ae^{4\alpha \phi }\). It leads to some corrections in the quantities have been calculated in the previous sections. Hereafter, we label the corrected quantities by adding the superscript (c) for identifying them from uncorrected ones. The corrected scalar-coupled Lagrangian of EH electrodynamics is

$$\begin{aligned} L^{(c)}(\mathcal{{F}}, \phi )=-\mathcal{{F}}e^{-2\alpha \phi }+a\mathcal{{F}}^2e^{-6\alpha \phi }+\mathcal{{O}}\left( a^2\right) , \end{aligned}$$
(IV.1)

and the solution of electromagnetic field equation becomes

$$\begin{aligned} F^{(c)}_{tr}=\frac{q}{rR}e^{2\alpha \phi }-4a\frac{q^3}{r^3R^3}e^{2\alpha \phi }+\mathcal{{O}}\left( a^2\right) . \end{aligned}$$
(IV.2)

Then, the temporal part of electromagnetic four-potential takes the form of

$$\begin{aligned} A^{(c)}_{t}= & {} -\int F_{tr} dr=\frac{q}{\sigma +2\alpha \lambda }\left( \frac{r_0}{r}\right) ^{\sigma }\left( \frac{b}{r}\right) ^{2\alpha \lambda }\nonumber \\{} & {} -\frac{4aq^3}{b^2\left( 3\sigma +2\alpha \lambda +2\right) }\left( \frac{r_0}{r}\right) ^{3\sigma }\left( \frac{b}{r}\right) ^{2+2\alpha \lambda }, \end{aligned}$$
(IV.3)

and, in order to the electric potential vanish at infinity, we must have

$$\begin{aligned}{} & {} 2\alpha \lambda +\sigma >0, \end{aligned}$$
(IV.4)
$$\begin{aligned}{} & {} 2\alpha \lambda +3\sigma +2>0, \end{aligned}$$
(IV.5)

which are clearly consistent for both \(\alpha \) and \(\lambda \) positive.

Also, \(V(\phi )\) and f(r) get some modifications and the corrected forms are as follows

$$\begin{aligned} V^{(c)}(\phi )= & {} 2\Lambda Exp\left( \frac{4\lambda \phi }{\sigma +1} \right) +2\Lambda _1Exp\left[ \left( 2\alpha +\frac{2(\sigma +1)}{\lambda } \right) \phi \right] \nonumber \\{} & {} +2\Lambda ^{(c)}_2Exp\left[ \left( 2\alpha +\frac{4(\sigma +1)}{\lambda }\right) \phi \right] , \end{aligned}$$
(IV.6)
$$\begin{aligned} f^{(c)}(r)= \left\{ \begin{array}{ll} -\frac{m}{r^\sigma }-\frac{2\Lambda b^2}{\Gamma }\left( \frac{r}{b}\right) ^{2\sigma +2} +\frac{2q^{2}}{\Gamma _1}\left( \frac{r_0}{b}\right) ^{2\sigma }\left( \frac{b}{r}\right) ^{2(\alpha \lambda +\sigma )}-\frac{8aq^{4}}{b^{2}\Gamma ^{(c)}_2}\left( \frac{r_0}{b}\right) ^{4\sigma }\left( \frac{b}{r}\right) ^{2(\alpha \lambda +2\sigma +1)}, \;\;\;\;\;\;\; \sigma \ne -\frac{2}{3},\\ -m r^{\frac{2}{3}}-6\Lambda (rb^2)^{\frac{2}{3}}\ln \left( \frac{r}{L}\right) +\frac{9q^{2}}{(\alpha -1)^2}\left( \frac{b}{r_0}\right) ^{\frac{4}{3}} \left( \frac{r}{b}\right) ^{\frac{2}{3}(2-\alpha )}-\frac{36aq^{4}}{b^2\alpha ^2}\left( \frac{b}{r_0}\right) ^{\frac{8}{3}}\left( \frac{r}{b}\right) ^{\frac{2}{3}(1-\alpha )}, \;\;\;\;\; \sigma = -\frac{2}{3}, \end{array} \right. \end{aligned}$$
(IV.7)

where,

$$\begin{aligned} \Lambda ^{(c)}_2= & {} \frac{2aq^4(\alpha \lambda +\sigma )}{b^4(\alpha \lambda +3\sigma +2)}\left( \frac{r_0}{b}\right) ^{4\sigma },\quad \text {and}\;\;\;\nonumber \\ \Gamma ^{(c)}_2= & {} (\alpha \lambda +3\sigma +2)(2\alpha \lambda +3\sigma +2). \end{aligned}$$
(IV.8)

Note that \(V^{(c)}(\phi )\) and \(f^{(c)}(r)\) recover their corresponds in the Einstein-Maxwell-dilaton theory if we set \(a=0\) [70]. Also, when the scalar field is absent the metric function (VI.7) reduces to that of ref. [57]. Then, the corrected values of electric potential and Hawking temperature are written as

$$\begin{aligned}{} & {} \Phi ^{(c)}(r_+)= \frac{Cq}{\sigma +2\alpha \lambda }\left( \frac{r_0}{r_+}\right) ^{\sigma }\left( \frac{b}{r_+}\right) ^{2\alpha \lambda }\nonumber \\{} & {} \quad -\frac{4Caq^3}{b^2\left( 2\alpha \lambda +3\sigma +2\right) }\left( \frac{r_0}{r_+}\right) ^{3\sigma }\left( \frac{b}{r_+}\right) ^{2\alpha \lambda +2},\;\; -1< \sigma \le 0,\nonumber \\ \end{aligned}$$
(IV.9)
$$\begin{aligned} T^{(c)}= & {} \frac{\kappa }{2\pi }=\frac{f^{(c)'}(r_+)}{4\pi }=\frac{-1}{2\pi }\left[ \frac{\Lambda r_+}{\sigma +1}\left( \frac{r_+}{b}\right) ^{2\sigma }\right. \\{} & {} \left. +\frac{q^2r_+}{b^2(\alpha \lambda +2\sigma +1)}\left( \frac{r_0}{b}\right) ^{2\sigma }\left( \frac{b}{r}\right) ^{2(\alpha \lambda +\sigma +1)}\right. \end{aligned}$$
$$\begin{aligned}{} & {} \qquad \qquad \left. -\frac{4aq^4r_+}{b^4(\alpha \lambda +3\sigma +2)}\left( \frac{r_0}{b}\right) ^{4\sigma }\left( \frac{b}{r_+}\right) ^{2(\alpha \lambda +2\sigma +2)}\right] ,\nonumber \\{} & {} \quad -1< \sigma \le 0, \end{aligned}$$
(IV.10)

which recover the electric potential and horizon temperature of dilatonic Maxwell-BTZ BHs for the case \(a=0\) [70]. In addition, for the case \(\sigma =0\) the BH temperature reduces to that of Einstein-EH theory presented in Ref. [57].

Noting the fact that \(f^{(c)}(r_+)=0\) and replacing \(m(r_+)\) into Eq. (III.24), we have

$$\begin{aligned}{} & {} M^{(c)}(r_+,q)\nonumber \\{} & {} \quad = \left\{ \begin{array}{ll} -\frac{\sigma +1}{4}\left( \frac{r_+}{r_0}\right) ^{\sigma }\left[ \frac{\Lambda b^2}{\Gamma }\left( \frac{r_+}{b}\right) ^{2\sigma +2} -\frac{q^{2}}{\Gamma _1}\left( \frac{r_0}{b}\right) ^{2\sigma }\left( \frac{b}{r_+}\right) ^{2(\alpha \lambda +\sigma )}\right. \\ \\ \left. \quad +\frac{4aq^{4}}{b^2\Gamma ^{(c)}_2}\left( \frac{r_0}{b}\right) ^{4\sigma }\left( \frac{b}{r_+}\right) ^{2(\alpha \lambda +2\sigma +1)}\right] , \;\;\;\;\; \sigma \ne -\frac{2}{3},\\ \\ -\frac{1}{8}\left( \frac{r_0}{r_+}\right) ^{\frac{2}{3}}\left[ 2\Lambda (r_+b^2)^{\frac{2}{3}}\ln \left( \frac{r_+}{L}\right) -\frac{3q^{2}}{(\alpha -1)^2}\left( \frac{b}{r_0}\right) ^{\frac{4}{3}} \left( \frac{r_+}{b}\right) ^{\frac{2}{3}(2-\alpha )}\right. \\ \left. \quad +\frac{12aq^{4}}{b^2\alpha ^2}\left( \frac{b}{r_0}\right) ^{\frac{8}{3}}\left( \frac{r_+}{b}\right) ^{\frac{2}{3}(1-\alpha )}\right] , \;\;\;\;\; \sigma = -\frac{2}{3}, \end{array} \right. \end{aligned}$$
(IV.11)
Fig. 1
figure 1

\(f^{(c)}(r)\) versus r for Middle: \(a{=}0.05, \sigma {=}{-}0.33, m{=}10.8 \text {(black)}, 11.49 \text {(blue)}, 12.3 \text {(red)}, 13 \text {(blue-dashed),} 13.6 \text {(brown)}.\) Right: \(m{=}13, a{=}0.05, \sigma {=}{-}0.195 \text {(black)}, {-}0.205 \text {(blue)}, {-}0.22 \text {(red)}, {-}0.245 \text {(blue-dashed),} {-}0.285 \text {(brown)}\)

Full size image
Fig. 2
figure 2

\(f^{(c)}(r)\) versus r for : Left: Right:

Full size image

It is nothing but the Smarr mass relation, which regarding Eqs. (III.21) and (III.23), presents \(M^{(c)}\) as a function of S and Q. After some algebraic calculations, we showed that

$$\begin{aligned} \left( \frac{\partial M^{(c)}}{\partial S}\right) _Q=T^{(c)},\;\; \text {for}\;\sigma = -\frac{2}{3},\;\;\text {and}\;\;\sigma \ne -\frac{2}{3},\nonumber \\ \end{aligned}$$
(IV.12)
$$\begin{aligned} \left( \frac{\partial M^{(c)}}{\partial Q}\right) _S=\frac{q(\sigma +1)(\alpha \lambda +2\sigma +1)^{-1}}{2\alpha \lambda +\sigma }\left( \frac{r_0}{r_+}\right) ^{\sigma }\left( \frac{b}{r_+}\right) ^{2\alpha \lambda } \end{aligned}$$
$$\begin{aligned}{} & {} -\frac{8aq^3(\sigma +1)\left( \alpha \lambda +3\sigma +2\right) ^{-1}}{b^{2}\left( 2\alpha \lambda +3\sigma +2\right) }\left( \frac{r_0}{r_+}\right) ^{3\sigma }\left( \frac{b}{r_+}\right) ^{2\alpha \lambda +2},\nonumber \\{} & {} \quad \;\; \text {for}\;\sigma = -\frac{2}{3},\;\;\text {and}\;\;\sigma \ne -\frac{2}{3}. \end{aligned}$$
(IV.13)

Therefore, noting (IV.9)

$$\begin{aligned} \left( \frac{\partial M^{(c)}}{\partial Q}\right) _S=\Phi ^{(c)}(r_+), \end{aligned}$$
(IV.14)

provided that

$$\begin{aligned} C=\frac{\sigma +1}{\alpha \lambda +2\sigma +1}=\frac{2(\sigma +1)}{\alpha \lambda +3\sigma +2}, \end{aligned}$$
(IV.15)

is chosen. It mens that the following relation must be fulfilled

$$\begin{aligned} \alpha \lambda +\sigma =0. \end{aligned}$$
(IV.16)

The coefficient C is fixed to \(C=1\) and, Eq. (VI.16) gives \(\alpha =2\) for the case \(\sigma = -\frac{2}{3}\). Finally the TFL remains valid as it has been presented in Eq. (III.33). Now the required conditions presented in Eqs. (VI.4), (VI.5) and (VI.16) are compatible and the mentioned problem of mathematical inconsistency has been resolved.

The plots of \(f^{(c)}(r)\) have been depicted in Figs. 1 and 2. They show that our dilatonic solutions exhibit BHs with one, two and three horizons. Also, they show that extreme BHs with zero temperature can exist.

5 Stability properties

Here, we analyze thermal stability of our novel BHs, by use of the canonical ensemble method. Thus, we have to calculate the heat capacity by treating the BH charge as a constant. The heat capacity is defined as [91, 92]

$$\begin{aligned} C_Q =T^{(c)}\left( \frac{\partial S}{\partial T^{(c)}}\right) _Q= T^{(c)}\left( \frac{\partial ^2M^{(c)}}{\partial S^2}\right) ^{-1}_Q. \end{aligned}$$
(V.1)

Note that, Eq. (IV.12) has been used in the last step of Eq. (V.1). In this method, a physically reasonable BH (i.e. \(T^{(c)}>0\)) with positive \(C_Q\) is locally stable. In other words, simultaneous positivity of \(T^{(c)}\) and \(\left( \partial ^2\,M^{(c)}/\partial S^2\right) _Q\) means thermal stability. The unstable BHs will be stable through experiencing phase transition. Real root(s) of \(T^{(c)}=0\) are the first-order phase transition points. Also, the real root(s) of \(M^{(c)}_{SS}=\left( \partial ^2\,M^{(c)}/\partial S^2\right) _Q=0\), where the heat capacity diverges, are the second-order phase transition points [77, 87, 93,94,95]. Now, with these issues in mind, we analyze thermal stability or phase transition of both of the new BH solutions we just obtained here. At first, we calculate the denominator of the BH heat capacity. The result is in the following form

$$\begin{aligned} M^{(c)}_{SS}= & {} \frac{-1}{\pi ^2(\sigma +1)}\left( \frac{r_0}{r_+}\right) ^{\sigma }\left[ \frac{\Lambda (2\sigma +1)}{\sigma +1}\left( \frac{r_+}{b}\right) ^{2\sigma }\right. \nonumber \\{} & {} \left. -\frac{q^2(2\alpha \lambda +2\sigma +1)}{b^2(\alpha \lambda +2\sigma +1)}\left( \frac{r_0}{b}\right) ^{2\sigma }\left( \frac{b}{r_+}\right) ^{2(\alpha \lambda +\sigma +1)}\right. \nonumber \\{} & {} \left. +\frac{4aq^4(-2\alpha \lambda +4\sigma +3)}{b^4(\alpha \lambda +3\sigma +2)}\left( \frac{r_0}{b}\right) ^{4\sigma }\left( \frac{b}{r_+}\right) ^{2(\alpha \lambda +2\sigma +2)}\right] ,\;\;\;\; \nonumber \\{} & {} -1< \sigma \le 0. \end{aligned}$$
(V.2)

By use of the relation (VI.16), it can be reduced to

Fig. 3
figure 3

\(T^{(c)}(r_+)\) (dashed) and \(M^{(c)}_{SS}(r_+)\) (continues) for \(a=0.8,\;\Lambda =-1,\;q=1,\;b=2.2,\;r_0=\;3.4\): Left: \(\sigma =-0.16.\)   Right: \(\sigma =-0.36,\;10\;M^{(c)}_{SS}\)

Full size image
Fig. 4
figure 4

\(T^{(c)}(r_+)\) (dashed) and \(M^{(c)}_{SS}(r_+)\) (continues) for \(a=0.8,\;\Lambda =-1,\;q=1\): Left: \(b=2.5,\;r_0=\;3.4,\;\sigma =-0.25.\)   Right: \( b=1,\;r_0=\;3,\; \sigma =-\frac{2}{3},\;4\;M^{(c)}_{SS}\)

Full size image
$$\begin{aligned}{} & {} M^{(c)}_{SS}=\frac{-1}{\pi ^2(\sigma +1)^2}\left( \frac{r_0}{r_+}\right) ^{\sigma }\left[ \Lambda (2\sigma +1)\left( \frac{r_+}{b}\right) ^{2\sigma } -\frac{q^2}{r_+^2}\left( \frac{r_0}{b}\right) ^{2\sigma }\right. \nonumber \\{} & {} \quad \left. +\frac{6aq^4(2\sigma +1)}{b^4}\left( \frac{r_0}{b}\right) ^{4\sigma }\left( \frac{b}{r_+}\right) ^{2(\sigma +2)}\right] ,\;\;\;\; -1{<} \sigma \le 0.\nonumber \\ \end{aligned}$$
(V.3)

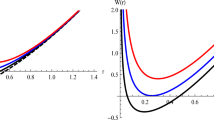
The plots of \(T^{(c)}(r_+)\) and \(M^{(c)}_{SS}(r_+)\) are shown in Figs. 3 and 4. Noting Fig. 3-left, it is understood that the BH temperature does not vanish and \(r_{ext}\) does not exist. Thus there is no first-order phase transition point. There is a real root for \(M^{(c)}_{SS}(r_+)=0\) which we label by \(r_1\), where the second-order phase transition occurs. The BHs with horizon radii greater than \(r_1\) are locally stable. The plots show that it is possible that \(r_{ext}\) exist but \(r_{1}\) does not. In that case, \(M^{(c)}_{SS}(r_+)\) is positive and no second-order phase transition occurs (Fig. 3-Right). A first-order phase transition occurs at \(r_+=r_{ext}\), where the BH temperature vanishes. Therefore, the BHs with the horizon radius greater than \(r_{ext}\) are locally stable.

The plots of Fig. 4-left show that there are two points of firs-order phase transition which we label by \(r_{1ext}\) and \(r_{2ext}\) and, one point of second-order phase transition exist which we label by \(r_1\). The BHs with horizon radius greater than \(r_{2ext}\) are locally stable. Also, noting Fig. 4-Right, there is only one point of first-order phase transition (i.e. \(r_+=r_{ext}\)) and, there are two points of second-order phase transition (\(r_1\) \(r_2\), \(r_1<r_2\)). The physically reasonable BHs with horizon radii in the intervals \(r_{ext}<r_+<r_1\) and \(r_+>r_{2}\) are locally stable.

6 Properties of the scalar–tensor black holes

With the BH solutions obtained in the Einstein frame, we tend to pursue exact ST solutions and, thermal properties in the Jordan frame. We consider the line element of ST gravity in the following form [96, 97]

$$\begin{aligned} d{\tilde{s}}^2={\tilde{g}}_{\mu \nu }dx^\mu dx^\nu =-A(r)dt^2+\frac{1}{B(r)}dr^2+r^2H^2(r)d\theta ^2.\nonumber \\ \end{aligned}$$
(VI.1)

Here, the metric coefficients A(r), B(r), and H(r) will be fixed as functions of r. That is possible by imposing the inverse transformations on the Einstein frame solutions with the metric function \(f^{(c)}(r)\) given by Eq. (IV.7). Regarding Eqs. (II.5), (II.15), (III.9) and (IV.16), we have \({\tilde{g}}_{\mu \nu }=g_{\mu \nu }\left( \frac{r}{b}\right) ^{4\sigma }\), which results in

$$\begin{aligned} A(r)= \left\{ \begin{array}{ll} \left( \frac{r}{b}\right) ^{4\sigma } F_1(r), \quad \sigma \ne -\frac{2}{3},\\ \\ \left( \frac{b}{r}\right) ^{\frac{8}{3}}F_2(r), \quad \sigma = -\frac{2}{3}, \end{array} \right. \end{aligned}$$
(VI.2)
$$\begin{aligned} B(r)= \left\{ \begin{array}{ll} \left( \frac{b}{r}\right) ^{4\sigma }F_1(r), \quad \sigma \ne -\frac{2}{3},\\ \\ \left( \frac{r}{b}\right) ^{\frac{8}{3}}F_2(r), \quad \sigma = -\frac{2}{3}, \end{array} \right. \end{aligned}$$
(VI.3)

where,

$$\begin{aligned} F_1(r)= & {} -\frac{m}{r^\sigma }-\frac{2\Lambda b^2}{\gamma }\left( \frac{r}{b}\right) ^{2\sigma +2} +\frac{2q^{2}}{\gamma _1}\left( \frac{r_0}{b}\right) ^{2\sigma }\nonumber \\{} & {} -\frac{8aq^{4}}{b^{2}\gamma _2}\left( \frac{r_0}{r}\right) ^{4\sigma }\left( \frac{b}{r}\right) ^{2(\sigma +1)}, \end{aligned}$$
(VI.4)
$$\begin{aligned} F_2(r)= & {} -m r^{\frac{2}{3}}-6\Lambda (rb^2)^{\frac{2}{3}}\ln \left( \frac{r}{L}\right) +9q^{2}\left( \frac{b}{r_0}\right) ^{\frac{4}{3}}\nonumber \\{} & {} -\frac{9aq^{4}}{b^2}\left( \frac{r}{r_0}\right) ^{\frac{8}{3}}\left( \frac{b}{r}\right) ^{\frac{2}{3}}, \end{aligned}$$
(VI.5)

and \(\gamma =(\sigma +1)(3\sigma +2)\), \(\gamma _1=-\sigma (\sigma +1)\), \(\gamma _2=2(\sigma +1)(\sigma +2)\). Also,

$$\begin{aligned} H(r)=\left( \frac{r}{b}\right) ^{2\sigma }\left( \frac{r}{r_0}\right) ^{\sigma },\;\;\;-1< \sigma \le 0. \end{aligned}$$
(VI.6)

Note that in obtaining metric functions A(r), B(r) and H(r), Eq. (IV.16) and the condition \(a\rightarrow ae^{-4\alpha \phi }\) have been used.

We have depicted B(r) in Figs. 4 and 5 by choosing different values for the parameters. It is understood that the scalar tensor BHs can occur with two horizons, without horizons and with one horizon having zero temperature named as extreme BH.

Fig. 5
figure 5

B(r) versus r for\(\Lambda =-1,\;q=1,\;r_0=3.4,\;b=2.2\): Left: \(m=11.1,\;\sigma =-0.36,\;a=0.1\;\text {(black)},\;0.48\;\text {(blue)},\;0.85\;\text {(red)}.\) Middle: \(a=0.5,\;\sigma =-0.36,\;m=10.9\;\text {(black)},\;11.092\;\text {(blue)},\;11.25\;\text {(red)}.\) Right: \( a=0.5,\;m=11.2,\;\sigma =-0.358\;\text {(black)},\;-0.366\;\text {(blue)},\;-0.375\;\text {(red)}\)

Full size image
Fig. 6
figure 6

50B(r) versus r for \(\sigma =-\frac{2}{3},\;\Lambda =-1,\;q=1,\;r_0=2,\;L=0.8,\;a=0.5\): Left: \(m=6.5,\;b=2.35\;\text {(black)},\;2.412\;\text {(blue)},\;2.5\;\text {(red)}.\) Right: \( b=2.5,\;m=6.4\;\text {(black)},\;6.82\;\text {(blue)},\;7.15\;\text {(red)}\)

Full size image

Now, regarding Eqs. (II.10), (II.11) and (II.15), one can show that

$$\begin{aligned} \psi (\phi )= & {} \frac{2\sqrt{2\alpha ^2-1}}{\alpha }e^{\alpha \phi },\quad \alpha >\frac{1}{\sqrt{2}}, \end{aligned}$$
(VI.7)
$$\begin{aligned} Y(\phi )= & {} -e^{-4\alpha \phi }, \end{aligned}$$
(VI.8)
$$\begin{aligned} Z(\phi )= & {} V^{(c)}(\phi )e^{-6\alpha \phi }, \end{aligned}$$
(VI.9)

where, \(V^{(c)}(\phi )\) is given by Eq. (VI.6) with \(\Lambda _1=0=\Lambda ^{(c)}_2\) by applying the condition (VI.16). Thus the physical scalar potential \(Z(\phi )\), can be written in the following explicit form

$$\begin{aligned} Z(\phi )=2\Lambda Exp\left[ \left( \frac{4\lambda }{\sigma +1}-6\alpha \right) \phi \right] . \end{aligned}$$
(VI.10)

Also, noting (III.9) and (VI.7), it is understood that the physical scalar field \(\psi \) vanishes when r is chosen very large.

The radius of event horizon(s) are obtained via \( B(r_+)=0\) or equivalently \(F_i(r_+)=0\) with \(i=1,\;2\). It leads

$$\begin{aligned} m(r_+)=\left\{ \begin{array}{ll} -2r_+^{\sigma }\left[ \frac{\Lambda b^2}{\gamma }\left( \frac{r_+}{b}\right) ^{2\sigma +2} -\frac{q^{2}}{\gamma _1}\left( \frac{r_0}{b}\right) ^{2\sigma }+\frac{4aq^{4}}{b^{2}\gamma _2}\left( \frac{r_0}{r_+}\right) ^{4\sigma }\left( \frac{b}{r_+}\right) ^{2(\sigma +1)}\right] , \quad \sigma \ne -\frac{2}{3}, \\ \\ -3r_+^{-\frac{2}{3}}\left[ 2\Lambda (r_+b^2)^{\frac{2}{3}}\ln \left( \frac{r_+}{L}\right) -3q^{2}\left( \frac{b}{r_0}\right) ^{\frac{4}{3}} +\frac{3aq^{4}}{b^2}\left( \frac{r_+}{r_0}\right) ^{\frac{8}{3}}\left( \frac{b}{r_+}\right) ^{\frac{2}{3}} \right] , \quad \sigma = -\frac{2}{3}, \end{array} \right. \end{aligned}$$
(VI.11)

which can be substituted in Eq. (III.24) to give the Smarr mass formula. That is

$$\begin{aligned} {\tilde{M}}=\left\{ \begin{array}{ll} -\frac{\sigma +1}{4}\left( \frac{r_+}{r_0}\right) ^{\sigma }\left[ \frac{\Lambda b^2}{\gamma }\left( \frac{r_+}{b}\right) ^{2\sigma +2} -\frac{q^{2}}{\gamma _1}\left( \frac{r_0}{b}\right) ^{2\sigma }+\frac{4aq^{4}}{b^{2}\gamma _2}\left( \frac{r_0}{r_+}\right) ^{4\sigma }\left( \frac{b}{r_+}\right) ^{2(\sigma +1)}\right] , \;\;\;\;\;\;\; \sigma \ne -\frac{2}{3},\\ \\ -\frac{1}{8}\left( \frac{r_+}{r_0}\right) ^{-\frac{2}{3}}\left[ 2\Lambda (r_+b^2)^{\frac{2}{3}}\ln \left( \frac{r}{L}\right) -3q^{2}\left( \frac{b}{r_0}\right) ^{\frac{4}{3}} +\frac{3aq^{4}}{b^2}\left( \frac{r_+}{r_0}\right) ^{\frac{8}{3}}\left( \frac{b}{r_+}\right) ^{\frac{2}{3}} \right] , \;\;\;\;\;\;\;\;\; \sigma = -\frac{2}{3}. \end{array} \right. \end{aligned}$$
(VI.12)

It is well-known that the BH entropy is a thermodynamic quantity which remains invariant under conformal transformation. Therefor, it is the same as that presented in Eq. (III.21). That is

$$\begin{aligned} {\tilde{S}}=\frac{\pi r_+}{2}\left( \frac{ r_+}{r_0}\right) ^{\sigma }, \end{aligned}$$
(VI.13)

with the \(r_+\) as the outer horizon radius of ST BHs. Also, the electric charge as the other conformal-invariant quantity is just the same as given in Eq. (III.23). That is \({\tilde{Q}}=q/2\).

Now, the horizon temperature for the ST BHs can be calculated by use of the surface gravity’s definition. That gives

$$\begin{aligned} {\tilde{T}}= & {} \frac{1}{4\pi }\left( \sqrt{\frac{B(r)}{A(r)}}\frac{d A(r)}{dr}\right) _{_{r=r_+}}\nonumber \\= & {} \frac{1}{4\pi }\left( \frac{b}{r_+}\right) ^{4\sigma }\frac{d }{dr}\left[ \left( \frac{b}{r}\right) ^{-4\sigma }F_i(r)\right] _{_{r=r_+}}=\frac{F'_i(r_+)}{4\pi }, \;\;\;\;\;i=1,\;2.\nonumber \\ \end{aligned}$$
(VI.14)

Note that \(r_+\) is the radius of outer event horizon and, in obtaining (VI.14) we have used the condition \(F_i(r_+)=0\). Thus, the BH horizon temperature can be written explicitly as

$$\begin{aligned} {\tilde{T}}= & {} \frac{-1}{2\pi (\sigma +1)r_+}\left[ \Lambda b^2\left( \frac{r_+}{b}\right) ^{2\sigma +2}+ q^{2}\left( \frac{r_0}{b}\right) ^{2\sigma }\right. \nonumber \\{} & {} \left. -\frac{2aq^{4}(5\sigma +2)}{b^{2}(\sigma +2)}\left( \frac{r_0}{r_+}\right) ^{4\sigma }\left( \frac{b}{r_+}\right) ^{2(\sigma +1)}\right] , \;\;\;\;\; -1< \sigma \le 0.\nonumber \\ \end{aligned}$$
(VI.15)
Fig. 7
figure 7

\({\tilde{T}}(r_+)\) (dashed) and \({\tilde{M}}_{{\tilde{S}} {\tilde{S}}}(r_+)\) (continues) for \(a=0.8,\;\Lambda =-1,\;q=1,\;b=2.2,\;r_0=\;3.4\): Left: \(\sigma =-0.16.\)   Right: \(\sigma =-0.36,\;10{\tilde{M}}_{{\tilde{S}} {\tilde{S}}}\)

Full size image
Fig. 8
figure 8

\({\tilde{T}}(r_+)\) (dashed) and \({\tilde{M}}_{{\tilde{S}} {\tilde{S}}}(r_+)\) (continues) for \(a=0.8,\;\Lambda =-1,\;q=1\): Left: \(b=1.2,\;r_0=\;3.4,\;\sigma =-0.25,\;2{\tilde{M}}_{{\tilde{S}} {\tilde{S}}}.\)   Right: \( b=1,\;r_0=\;2.8,\; \sigma =-\frac{2}{3},\;10{\tilde{M}}_{{\tilde{S}} {\tilde{S}}}\)

Full size image

At this stage by applying the transformation \(a\rightarrow ae^{-4\alpha \phi }\) on the electric potential (IV.9), we have

$$\begin{aligned} {\tilde{\Phi }}(r_+)= & {} -\frac{q}{\sigma }\left( \frac{r_0}{r_+}\right) ^{\sigma }\left( \frac{r_+}{b}\right) ^{2\sigma }\nonumber \\{} & {} -\frac{4aq^3}{b^2\left( \sigma +2\right) } \left( \frac{r_0}{r_+}\right) ^{3\sigma }\left( \frac{b}{r_+}\right) ^{2+2\sigma },\;\;\;\;\; -1< \sigma \le 0\nonumber \\ \end{aligned}$$
(VI.16)

where, the condition (IV.16) and \(C=1\) have been used. Therefore, one can show that

$$\begin{aligned} {\tilde{\Phi }}=\frac{\partial {\tilde{M}}}{\partial {\tilde{Q}}}, \;\;\;\;\;\;\;\;\text {and}\;\;\;\;\;\;\;\;{\tilde{T}}=\frac{\partial {\tilde{M}}}{\partial {\tilde{S}}}. \end{aligned}$$
(VI.17)

It is understood from (VI.17) that the TFL is valid for our novel ST BHs, which can be written as

$$\begin{aligned} d{\tilde{M}}={\tilde{T}}d{\tilde{S}}+{\tilde{\Phi }} d{\tilde{Q}}. \end{aligned}$$
(VI.18)

Here, we calculate the heat capacity of the ST BHs by use of the relation

$$\begin{aligned} {\tilde{C}}_Q ={\tilde{T}}\left( \frac{\partial {\tilde{S}}}{\partial {\tilde{T}}}\right) _{{\tilde{Q}}}= {\tilde{T}}\left( {\tilde{M}}_{{\tilde{S}} {\tilde{S}}}\right) ^{-1}. \end{aligned}$$
(VI.19)

Note that in the last step Eq. (VI.17) has been used. Now, by doing some algebraic calculations, we have

$$\begin{aligned} {\tilde{M}}_{{\tilde{S}} {\tilde{S}}}= & {} \frac{-1}{\pi ^2(\sigma +1)^2}\left( \frac{r_0}{r_+}\right) ^{\sigma }\left[ \Lambda (2\sigma +1)\left( \frac{r_+}{b}\right) ^{2\sigma } -\frac{q^2}{r_+^2}\left( \frac{r_0}{b}\right) ^{2\sigma }\right. \nonumber \\{} & {} \left. +\frac{6aq^4(2\sigma +1)}{b^4(\sigma +2)}\left( \frac{r_0}{r_+}\right) ^{4\sigma }\left( \frac{b}{r_+}\right) ^{2(\sigma +2)}\right] , \end{aligned}$$
(VI.20)

which is valid for \(-1< \sigma \le 0.\) The plots of \({\tilde{T}}(r_+)\) and \({\tilde{M}}_{{\tilde{S}} {\tilde{S}}}(r_+)\) are shown simultaneously in Figs. 7 and 8, for analyzing the BH temperature and characterizing the points of first and second order phase transitions. The left panel of Fig. 7 shows that the BH temperature can be positive for all BH radii and, no first-order phase transition can occur. The equation \({\tilde{M}}_{{\tilde{S}} {\tilde{S}}}(r_+)=0\) has only one real root which is the position of second-order phase transition. The BHs with horizon radii greater than \(r_1\) are locally stable. As it can be seen in right panel of Fig. 7, \({\tilde{M}}_{{\tilde{S}} {\tilde{S}}}(r_+)\) can be positive and, no second-order phase transition takes place. The BH temperature vanishes at \(r_+=r_{ext}\) and it is a first-order phase transition point. The BHs with horizon radii greater than \(r_{ext}\) which have positive temperature are physically reasonable. They have also positive heat capacity and are locally stable. The left panel of Fig. 8 shows that it is possible for the BH temperature to have two real roots which we have labeled by \(r_{1ext}\) and \(r_{2ext}\). They are known as the points of first-order phase transition. Also, \({\tilde{M}}_{{\tilde{S}} {\tilde{S}}}(r_+)\) has only one vanishing point (i.e. \(r_+=r_{1}\)) at which the BH heat capacity diverges. Therefore, the BH with horizon radius equal to \(r_{1}\) experiences second-order phase transition and, the BHs with horizon radii greater than \(r_{2ext}\) are locally stable. The right panel of Fig. 8 shows that there are two points of first-order phase transition which have been shown by \(r_{1ext}\) and \(r_{2ext}\). Also, there are two points of second-order phase transition labeled by \(r_{1}\) and \(r_{2}\). The BHs with horizon radii in the intervals \(r_{1ext}<r_+<r_1\) and \(r_2<r_+<r_{2ext}\) are locally stable. It must be noted that stability properties of ST BHs are slightly different from those of Einstein-dilaton ones. This is obvious through comparison of right panels of Figs. 4 and 8. It is due to transformation relation of the nonlinearity parameter a from one frame to the other one.

7 Conclusion

We explored charged ST BHs in the presence of EH nonlinear electrodynamics. The starting point was obtaining the ST field equations by varying the related three-dimensional action. They are strongly coupled such that one cannot solve them directly. We showed that this problem can be resolved by using a mathematical tool named as the conformal transformation, which transforms the ST action to that of Einstein-dilaton gravity theory which is written in the Einstein frame. In this frame the field equations are decoupled and, the solutions can be obtained easily. The thermodynamics and thermal stability of the solutions have been studied in the Einstein frame. We noticed that in order to (a) the electric potential vanish at infinity and (b) the TFL remain valid, three requirements must be fulfilled which are not consistent, mathematically (see Eqs. (III.13), (III.14) and (III.34)). With the aim of resolving the confronted problem, we assumed through conformal transformations the nonlinearity parameter of the EH theory must obey the transformation relation \(a\rightarrow ae^{4\alpha \phi }\). Then we obtained the corrected exact solutions and studied thermodynamics and thermal stability properties which are free of any theoretical and mathematical problems. In addition they fulfill TFL in its standard form. As shown in Figs. 1 and 2, the corrected Einstein-dilaton solutions can produce multi-horizon BHs which is related to the quantum anti-evaporation phenomena. In addition they show BHs with one-horizon and extreme BHs too. The extreme BHs with two horizon radii exist which is due to consideration of EH electrodynamics. Thermal stability of the BHs have been studied by use of the canonical ensemble method. The results show that these BHs can appear with positive temperature, extreme BHs with one or two horizon radii. Also, the BHs with negative temperature can occur which are not physically reasonable. These BHs are stable in a wide range of horizon radii (see Figs. 3 and 4).

We obtained three-dimensional ST-EH BHs by use of inverse conformal transformation together with \(a\rightarrow ae^{-4\alpha \phi }\). Through drawing the plots, as shown in Figs. 5 and 6, we found that in addition to the one-horizon and extreme BHs, the two-horizon ST BHs can exist too. We calculated charge, temperature, entropy, electric potential and mass of the ST BHs by using the appropriate approaches. Existence of the extreme BHs with two horizon radii is due to consideration of EH electrodynamics. Then through a Smarr mass relation, which gives the BH mass as a function of charge and entropy, we found that the standard form of the TFL is valid for the ST BHs too. Thermal stability of the ST BHs has been investigated by use of the canonical ensemble method. The horizon radius of those BHs which experience first-order or second-order phase transitions have been explored noting the signature of the heat capacities. For a better interpretation, we have depicted the plots of heat capacities versus \(r_+\) in Figs. 7 and 8. Comparison of them with those of Figs. 3 and 4 show that stability properties of ST BHs is slightly different from those of Einstein-dilaton ones. This is due to the transformation property of the nonlinearity parameter a.