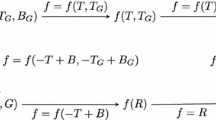1 Introduction

In modified theories of gravity there is a large portion of the literature on modified teleparallel equivalent theories of gravity [1,2,3,4], which has attracted a lot of attention because of many important cosmological applications [5,6,7,8,9,10,11,12,13,14]. The modified teleparallel equivalent theories of gravity is a series of torsion-based modified theories of gravity. A more representative generalization of it is \(f({\mathcal {T}},{\mathcal {B}})\) gravity [15,16,17,18,19,20,21,22,23,24], which has been very successful in understanding large scale structure and cosmology [25,26,27,28,29,30,31]. However, the description of local spacetime is difficult to be obtained as there are high-dimensional terms in the field equation of modified gravity theories. In this regard, the understanding of local structure has important implications for the theoretical developments of gravity theories. The spherical symmetric solution in theories of gravity establishes a fundamental class of solutions to describe compact objects like black holes and stars [32,33,34,35,36,37,38,39,40,41]. Primordial black holes and black hole shadow are now also the focus of research in astrophysics [35, 42, 43]. In addition, they can serve as a starting point for finding more realistic axisymmetric solutions that can describe rotating compact objects [15, 44, 45]. Therefore, it is extremely important to study the properties of these solutions. In the study of the properties of spherically symmetric spacetime solutions, the Birkhoff’s theorem is extremely important.

The Birkhoff’s theorem [46, 47], which states that if the matter distribution is spherically symmetric, then the spacetime is static in a region in which t remains timelike and \((r,\theta ,\phi )\) stay spacelike [46, 48,49,50], is vitally important in studying local systems in modified theories of gravity [48, 51,52,53,54]. The Birkhoff’s theorem admits the fact that a spherically symmetric matter distribution will create a static gravitational field. Thus, we can use it to describe the gravitational field outside the celestial body, which is of great importance in astrophysics. The Birkhoff’s theorem also provides an important reason for the stability of black hole spacetime, and has interesting implications for the question of how a universe composed of locally spherically symmetric objects embedded in a region of vacuum can expand. Because of the vital role that Birkhoff’s theorem plays in theories of gravity, the stability studies are also needed for spherically symmetric solutions in modified theories of gravity to determine whether these theories are physically feasible.

In this article, we investigate the validity of the Birkhoff’s theorem in \(f({\mathcal {T}},{\mathcal {B}})\) gravity. We will give a brief introduction at the beginning of each section. The structure of the paper is as follows. We give a brief introduction to the \(f({\mathcal {T}},{\mathcal {B}})\) gravity in Sect. 2. In Sect. 3 we discuss the feature of spherically symmetric solutions in frame of modified teleparallel equivalent theories of gravity, and give the proof of the Birkhoff’s theorem in \(f({\mathcal {T}},{\mathcal {B}})\) gravity. Section 4 is devoted to the discussion and conclusions. We use over-circles to denote quantities determined using the Levi–Civita connection \(\mathring{\varGamma }^{\lambda }_{\mu \nu }\) throughout this paper, i.e. covariant derivative \(\mathring{\nabla }\), Ricci scalar \(\mathring{R}\), etc. In this article, we will not discuss the special form of \(f(-{\mathcal {T}}+{\mathcal {B}})\), in which \(f_{{\mathcal {T}}}+f_{{\mathcal {B}}}=\)constant, because this form can be considered the teleparallel equivalent of \(f(\mathring{R})\) gravity, and we have a relatively clear understanding of the validity of Birkhoff’s theorem in this theory.

2 Modified teleparallel equivalent theories of gravity

Mathematically speaking, the spacetime, i.e., the collection of all events, is a 4D connected Hausdorff \(C^{\infty }\) manifold \({\mathcal {M}}\) with Lorentz metric g [49]. To set up a partial derivative depending only on the direction of the point we consider, we define an extra structure called connection on \({\mathcal {M}}\), so that we can build field equations on the manifold. In the frame of general relativity the connection is torsion-less Levi–Civita connection \(\mathring{\varGamma }^{\lambda }_{\ \mu \nu }\). In teleparallel gravity this is replaced by the curvature-less teleparallel connection \(\varGamma ^{\lambda }_{\ \mu \nu }\). Another difference is we use vierbein instead of metric as the element, which is defined as:

$$\begin{aligned} g_{\mu \nu }=h^{a}_{\ \mu }h^{b}_{\ \nu }\eta _{ab}, \end{aligned}$$
(1)

where \(h^{a}_{\ \mu }\) are the vierbein components and the Minkowski metric is given by \(\eta _{ab} = \text {diag}(+1, -1, -1, -1)\). We use Greek indices to indicate spacetime coordinates, while Latin indices are used for tangent-space coordinates throughout this paper. The Weitzenböck connection is defined as:

$$\begin{aligned} \varGamma ^{\lambda }_{\ \mu \nu }=h^{\ \lambda }_{a}\partial _{\nu }h^{a}_{\ \mu }+h^{\ \lambda }_{a}\omega ^{a}_{\ b\nu }h^{b}_{\ \mu }, \end{aligned}$$
(2)

where the \(\omega ^{a}_{\ b\nu }\) are the elements of spin connection, which describe inertial effects. \(\omega ^{a}_{\ b\nu }\) are defined as

$$\begin{aligned} \omega ^{a}_{\ b\nu }=h^{a}_{\ \lambda }\partial _{\nu }h^{\ \lambda }_{b}+h^{a}_{\ \lambda }\varGamma ^{\lambda }_{\ \mu \nu }h^{\ \mu }_{b}=h^{a}_{\ \lambda }\nabla _{\nu }h^{\ \lambda }_{b}, \end{aligned}$$
(3)

where \(\nabla _{\nu }\) is the covariant derivative associated with teleparallel connection. The vierbein and spin connection come together with a Lorentz gauge symmetry. Under Lorentz transformation \(\varLambda \), the vierbein transforms as

$$\begin{aligned} h^{a}_{\ \mu }\rightarrow \tilde{h}^{a}_{\ \mu }=\varLambda ^{a}_{\ b}h^{b}_{\ \mu }, \end{aligned}$$
(4)

while the spin connection transforms as

$$\begin{aligned} \omega ^{a}_{\ b\mu }\rightarrow \tilde{\omega }^{a}_{\ b\mu }=\varLambda ^{a}_{\ c}(\varLambda ^{-1})^{d}_{\ b}\omega ^{c}_{\ d\mu }+\varLambda ^{a}_{\ c}\partial _{\mu }(\varLambda ^{-1})^{c}_{\ b}. \end{aligned}$$
(5)

In this case it is always possible to choose a gauge locally such that the spin connection vanishes, namely \(\omega ^{a}_{\ b\mu }\equiv 0\). This gauge is called Weitzenböck gauge. It further follows that in any other gauge the spin connection takes the simple form

$$\begin{aligned} \omega ^{a}_{\ b\nu }=\varLambda ^{a}_{\ c}\partial _{\mu }(\varLambda ^{-1})^{c}_{\ b}, \end{aligned}$$
(6)

which shows that the spin connection is a pure gauge parameter. The torsion tensor is defined as

$$\begin{aligned} T^{\lambda }_{\ \mu \nu }=\varGamma ^{\lambda }_{\ \nu \mu }-\varGamma ^{\lambda }_{\ \mu \nu }. \end{aligned}$$
(7)

In order to get the torsion scalar in the teleparallel gravity, it is convenient to define two other tensors: contortion tensor \(K^{\ \mu \nu }_{\lambda }\) and superpotential \(S^{\ \mu \nu }_{\lambda }\) as

$$\begin{aligned} K^{\mu \nu }_{\ \ \lambda }=\frac{1}{2}(T^{\nu \mu }_{\ \ \lambda }+T^{\ \mu \nu }_{\lambda }-T^{\mu \nu }_{\ \ \lambda }) , \end{aligned}$$
(8)

and

$$\begin{aligned} S^{\ \mu \nu }_{\lambda }=\frac{1}{2}(K^{\mu \nu }_{\ \ \lambda }-\delta ^{\mu }_{\lambda }T^{\ \sigma \nu }_{\sigma }+\delta ^{\nu }_{\lambda }T^{\ \sigma \mu }_{\sigma }). \end{aligned}$$
(9)

Then the torsion scalar \({\mathcal {T}}\) is defined as

$$\begin{aligned} {\mathcal {T}}=T^{\lambda }_{\ \mu \nu }S^{\ \mu \nu }_{\lambda }. \end{aligned}$$
(10)

If we calculate the Ricci scalar \(\mathring{R}\) in terms of vierbein, we will find it is [17, 55]

$$\begin{aligned} \mathring{R}=-{\mathcal {T}}+\frac{2}{h}\partial _{\mu }(hT^{\mu }), \end{aligned}$$
(11)

where \(h=\det (h^{a}_{\ \mu })=\sqrt{|g|}\) is the determinant of vierbein \(h^{a}_{\ \mu }\), and \(T^{\mu }=T_{\sigma }^{\ \sigma \mu }\). We define the boundary term as

$$\begin{aligned} {\mathcal {B}}=\frac{2}{h}\partial _{\mu }(hT^{\mu }). \end{aligned}$$
(12)

So we have a teleparallel equivalent theory of gravity to General Relativity. Since the torsion scalar \({\mathcal {T}}\) and Ricci scalar \(\mathring{R}\) are different only by a boundary term \({\mathcal {B}}\), they actually describe the same physics at the lowest order level, and we could just throw boundary term in the action and write Lagrangian as \({\mathcal {L}}=\mathring{R}\rightarrow {\mathcal {T}}\). But if we were to modify this theory of gravity, in principle we could not throw away the boundary term. In the spirit of modified theory of gravity we write the general Lagrangian as a function of \({\mathcal {T}}\) and \({\mathcal {B}}\), namely \(f({\mathcal {T}},{\mathcal {B}})\). Then the action is

$$\begin{aligned} S=\int d^{4}x\frac{1}{16\pi G}h\cdot f({\mathcal {T}},{\mathcal {B}})+{\mathcal {L}}_{m}, \end{aligned}$$
(13)

where \({\mathcal {L}}_{m}\) is the matter Lagrangian. The Lagrangian of gravity is the function of vierbein and spin connection \(f=f(h,\omega )\), while the matter part is the function of vierbein and matter field \(\varPhi \): \({\mathcal {L}}_{m}={\mathcal {L}}_{m}(h,\varPhi )\) [56,57,58]. Thus, the equation of spin connection is identically zero, and we will ignore it for simplicity. We can derive the field equation [15, 17, 56]:

$$\begin{aligned} {\mathcal {W}}^{\ \nu }_{\mu }&=2\delta ^{\nu }_{\mu }\mathring{\square } f_{{\mathcal {B}}}-2\mathring{\nabla }^{\nu }\mathring{\nabla }_{\mu } f_{{\mathcal {B}}}+\delta ^{\nu }_{\mu }{\mathcal {B}}f_{{\mathcal {B}}} -\delta ^{\nu }_{\mu }f\nonumber \\&\quad -2S^{\ \nu \rho }_{\mu }(\partial _{\rho }f_{{\mathcal {B}}} +\partial _{\sigma }f_{{\mathcal {T}}})+2\omega ^{a}_{\ \mu \lambda } S^{\ \nu \lambda }_{a}f_{{\mathcal {T}}}\nonumber \\&\quad +\frac{2}{h}h^{a}_{\ \mu }\partial _{\lambda } (hS^{\ \lambda \nu }_{a})f_{{\mathcal {T}}}-2T^{\sigma }_{\ \lambda \mu } S^{\ \nu \lambda }_{\sigma }f_{{\mathcal {T}}}=16\pi G\varTheta ^{\ \nu }_{\mu }, \end{aligned}$$
(14)

where \(\varTheta ^{\ \nu }_{\mu }\) represents the stress-energy tensor, it comes from the Euler–Lagrangian equation of \({\mathcal {L}}_{m}\) part in full Lagrangian, and implies that matter couples to the vierbein in the standard form. If we set \({\mathcal {B}}=0\), the equation will reduce to the form of which in the \(f({\mathcal {T}})\) gravity. This form of field equation is not symmetric while the energy-momentum tensor is. Thus, the symmetric part and anti-symmetric part of the field equation must satisfy

$$\begin{aligned} {\mathcal {W}}_{(\mu \nu )}=8\pi G\varTheta _{\mu \nu },\quad {\mathcal {W}}_{[\mu \nu ]}=0 \end{aligned}$$
(15)

because of the Lorentz invariance. The corresponding field equation for spin connection is zero identically. The anti-symmetric part of the field equation vanishes because it coincides with the equation for spin connection [56]. In this case, the superpotential \(S_{\mu \nu }^{\ \ \ \rho }\) only left with totally symmetric part with respect to \(\mu \) and \(\nu \) in field equation and its anti-symmetric part should vanish, which will give us some useful restrictions. We will use these functions in last sections.

3 The spherically symmetric solution and Birkhoff’s theorem

Birkhoff’s theorem: if the matter distribution is spherically symmetric (\(T_{\mu \nu }=0\) for \(\mu \ne \nu \)), then the spacetime is static in a region in which t remains timelike and \((r,\theta ,\phi )\) stay spacelike.

Obviously, this version of Birkhoff’s theorem includes the original version of Birkhoff’s theorem, which states that the spherically symmetric vacuum spacetime is necessarily static. It also includes a less trivial version of Birkhoff’s theorem, which states that if a solution of the field equation is spherically symmetric and the matter distribution is static \((\dot{T}_{\mu \nu }=0)\), then the spacetime is static in a region in which t remains timelike and \((r,\theta ,\phi )\) stay spacelike. This version is always used in other modified theories of gravity [48, 51].

In this section we give the definition of spherical symmetry, discuss the general characteristic of spherically symmetric field equation and give the proof of Birkhoff’s theorem. This analysis is to lay the groundwork for proving the Birkhoff’s theorem. In the whole process of proof, we first write the form of solution \((h^{a}_{\ \mu },\omega ^{a}_{\ b\mu })\) through SO(3) symmetry, and then return to the corresponding field equation, and restrict the form of the solution through the conditions that the energy-momentum tensor and field equation need to meet. In this analysis, we do not make any demands on the form of Lagrangian f. In other words, we restrict the properties of the symmetric solution through the physical properties of energy-momentum tensor and the field equation, and in principle, a general spherically symmetric solution should hold true for all forms of f. Based on this principle, we divide this section into three summaries, giving the most general forms of vierbein and spin connection \((h^{a}_{\ \mu },\omega ^{a}_{\ b\mu })\) under spherical symmetry; discussing the form of the spherical symmetric energy-momentum tensor, and discussing the properties of the solution of the spherical symmetric field equation. In the case of a spherically symmetric distribution of matter, we naturally require off-diagonal components of energy-momentum tensor vanish. We will find that using only this one requirement, Birkhoff’s theorem will be satisfied automatically.

We require the solution are invariant under the action of the SO(3) group. The most general form of vierbein \(h^{a}=h^{a}_{\ \mu }dx^{\mu }\) in Weitzenböck gauge is given by [44, 56]

$$\begin{aligned} h^{0}&=C_{1}dt+C_{2}dr\nonumber \\ h^{1}&=\sin {\theta }\cos {\phi }(C_{3}dt+C_{4}dr)\nonumber \\&\quad +(C_{5}\cos {\theta }\cos {\phi }-C_{6}\sin {\phi })d\theta \nonumber \\&\quad -\sin {\theta }(C_{5}\sin {\phi }+C_{6} \cos {\theta }\cos {\phi })d\phi \nonumber \\ h^{2}&=\sin {\theta }\sin {\phi }(C_{3}dt+C_{4}dr)\nonumber \\&\quad +(C_{5}\cos {\theta }\sin {\phi }+C_{6}\cos {\phi })d\theta \nonumber \\&\quad +\sin {\theta }(C_{5}\cos {\phi }-C_{6} \cos {\theta }\sin {\phi })d\phi \nonumber \\ h^{3}&=\cos {\theta }(C_{3}dt+C_{4}dr)\nonumber \\&\quad -C_{5}\sin {\theta }d\theta +C_{6}\sin ^{2}\theta d\phi \end{aligned}$$
(16)

with vanishing spin connection. Here \(C_{1}\sim C_{6}\) are all functions of t and r. It seems that the SO(3) leaves us 6 undetermined functions, however, we will find only 3 of them are dynamical and the other 3 are gauge. To see this fact clearer we do the following transformation to vierbein:

$$\begin{aligned}&C_{1}=A(t,r)\cosh \alpha (t,r),\quad C_{2}=B(t,r)\sinh \beta (t,r),\nonumber \\&C_{3}=A(t,r)\sinh \alpha (t,r),\quad C_{4}=B(t,r)\cosh \beta (t,r),\nonumber \\&C_{5}=Y(t,r)\cos \varphi (t,r),\quad C_{6}=Y(t,r)\sin \varphi (t,r) \end{aligned}$$
(17)

and apply Lorentz transformations introduced in [44]. After this we end up with a general spherical symmetric solution \((h^{a}_{\ \mu },\omega ^{a}_{\ b\mu })\):

$$\begin{aligned} h^{a}_{\ \mu }=\begin{pmatrix} A\cosh {(\alpha -\beta )}&{}0&{}\ 0&{}0\\ A\sinh {(\alpha -\beta )}&{}B&{}\ 0&{}0\\ 0&{}0&{}\ Y&{}0\\ 0&{}0&{}\ 0&{}Y\sin {\theta } \end{pmatrix} \end{aligned}$$
(18)

with non-vanishing spin connection components

$$\begin{aligned} \omega ^{0}_{\ 1t}&=\omega ^{1}_{\ 0t}=\partial _{t}\alpha ,\quad -\omega ^{2}_{\ 3t}=\omega ^{3}_{\ 2t}=\partial _{t}\varphi ,\nonumber \\ \omega ^{0}_{\ 1r}&=\omega ^{1}_{\ 0r}=\partial _{r}\alpha ,\quad -\omega ^{2}_{\ 3r}=\omega ^{3}_{\ 2r}=\partial _{r}\varphi ,\nonumber \\ \omega ^{0}_{\ 2\theta }&=\omega ^{2}_{\ 0\theta }=\sinh {\alpha }\cos {\varphi },\nonumber \\ -\omega ^{1}_{\ 2\theta }&=\omega ^{2}_{\ 1\theta }=\cosh {\alpha }\cos {\varphi },\nonumber \\ \omega ^{0}_{\ 3\theta }&=\omega ^{3}_{\ 0\theta }=-\sinh {\alpha }\sin {\varphi },\nonumber \\ -\omega ^{1}_{\ 3\theta }&=\omega ^{3}_{\ 1\theta }=-\cosh {\alpha }\sin {\varphi },\nonumber \\ \omega ^{0}_{\ 2\phi }&=\omega ^{2}_{\ 0\phi }=\sinh {\alpha }\sin {\varphi }\sin {\theta },\nonumber \\ -\omega ^{1}_{\ 2\phi }&=\omega ^{2}_{\ 1\phi }=\cosh {\alpha }\sin {\varphi }\sin {\theta },\nonumber \\ \omega ^{0}_{\ 3\phi }&=\omega ^{3}_{\ 0\phi }=\sinh {\alpha }\cos {\varphi }\sin {\theta },\nonumber \\ -\omega ^{1}_{\ 3\phi }&=\omega ^{3}_{\ 1\phi }=\cosh {\alpha }\cos {\varphi }\sin {\theta },\nonumber \\ -\omega ^{2}_{\ 3\phi }&=\omega ^{3}_{\ 2\phi }=\cos {\theta }. \end{aligned}$$
(19)

In this form of vierbein and spin connection, generally the Lorentz parameters \(\varphi \), \(\alpha \) and \(\beta \) are all functions of t and r. However, intrinsically they are redundant parameters of Lorentz gauge. These parameters should be understood as the undetermined functions which can get different corresponding values by taking different functional forms with the same pair values of (t, r). In this case, we can see them as the independent Lorentz parameters having no relation to vierbein and metric, this conclusion will not affect our generality of discussion in the following. In this way, we find it’s true that only 3 components in Weitzenböck gauge vierbein are dynamical, and the other 3 are gauge. This makes us be able to treat them differently. The corresponding metric with respect to such vierbein is given by

$$\begin{aligned} g_{\mu \nu }=\begin{pmatrix} A^{2}&{}-F&{}0&{}0\\ -F&{}-B^{2}&{}0&{}0\\ 0&{}0&{}-Y^{2}&{}0\\ 0&{}0&{}0&{}Y^{2}\sin ^{2}{\theta } \end{pmatrix}, \end{aligned}$$
(20)

where \(F=AB\sinh ^{2}{(\alpha -\beta )}\). There appears two off-diagonal components in metric, it indeed satisfies the spherically symmetric Lie derivative condition. The metric and vierbein [56] introduced here is the most general form which contains the vierbein we used before [15, 45, 59,60,61,62,63], which has only diagonal components. Under these metric and vierbein we find scalars \({\mathcal {T}}={\mathcal {T}}(t,r)\) and \({\mathcal {B}}={\mathcal {B}}(t,r)\), so that the Lagrangian f, its derivative over \({\mathcal {T}}\) and \({\mathcal {B}}\), and the field equation are all functions of t and r only [44]. This can also be understood as the result of spherical symmetry.

3.1 The spherically symmetric energy–momentum tensor

We will see in this sub-section that the requirement of spherical symmetry on energy–momentum tensor will bring us some restrictions on vierbein. It’s convenient to write the energy-momentum tensor \(\varTheta _{\mu \nu }\) as

$$\begin{aligned}&\mathring{G}_{\mu \nu }f_{{\mathcal {T}}}+\frac{1}{2}g_{\mu \nu } (f-{\mathcal {T}}f_{{\mathcal {T}}}-{\mathcal {B}}f_{{\mathcal {B}}}) +(\mathring{\nabla }_{\mu }\mathring{\nabla }_{\nu }-g_{\mu \nu } \mathring{\square })f_{{\mathcal {B}}}\nonumber \\&\qquad +S_{(\mu \nu )}^{\ \ \ \ \ \rho }\partial _{\rho } (f_{{\mathcal {T}}}+f_{{\mathcal {B}}})={\mathcal {W}}_{(\mu \nu )} \equiv 8\pi G\varTheta _{\mu \nu }. \end{aligned}$$
(21)

As we explained in Sect. 2, the anti-symmetric part of the field equation agrees with the equation of spin connection, thus, it vanishes identically:

$$\begin{aligned} S_{[\mu \nu ]}^{\ \ \ \ \rho }\partial _{\rho }(f_{{\mathcal {T}}} +f_{{\mathcal {B}}})={\mathcal {W}}_{[\mu \nu ]}\equiv 0. \end{aligned}$$
(22)

Due to the spherical symmetry the dynamical variables can only be functions of time t and radial coordinate r only, thus, the derivative \(\partial _{\theta }\) and \(\partial _{\phi }\) will naturally produce zero. The dynamical equations can only give restriction to \(\mu =t,r\), and in the field equation we can only assume \(\rho =t,r\) for \(S_{(\mu \nu )}^{\ \ \ \ \ \rho }\partial _{\rho }\) term.

The spherical symmetry implies only diagonal components of energy density \(\varTheta _{\mu \nu }\) is non-zero in non-vacuum condition [45, 46, 48, 50, 52, 54, 64]. This requires the metric must be diagonal. To see this we notice that we are free to add a constant \(\kappa \) in modified teleparallel theories of gravity. The constant appearing in Lagrangian can be seen as a consequence of Taylor expansion of Lagrangian [12, 65], or the appearance of cosmological constant [2, 48, 66, 67]. Adding a constant into Lagrangian is equivalent to adding an extra energy-momentum tensor \(\kappa g_{\mu \nu }=8\pi GT_{\mu \nu }\). For 01 components of the energy-momentum tensor we find the corresponding energy flux q, which should be zero identically under spherical symmetry [48, 49], becomes non-zero, namely \(q=g_{01}\kappa =-AB\kappa \sinh (\alpha -\beta )\ne 0\). This can be understood as a consequence of Lorentz boost. For the integrity of the theory, we require the 01 component of energy-momentum tensor to be zero in spherical symmetry with a cosmological constant. Thus, we have to require \(\alpha =\beta \). This reduction of Lorentz parameter \(\alpha =\beta \) can be understood as the result of a moderate Lorentz boost which cuts off the energy flux in the view of the observer. This can also be understood as the result of spherical symmetry, in which condition we only need 1 boost acting on coordinates (t, r) and 1 rotation acting on sphere \(\varOmega _{2}\) because of spherical symmetry the \(\phi \) and \(\theta \) make no differences. In this case, this reduction will not affect the generality of our discussion.

We also notice that for general spherically symmetric solution \((h^{a}_{\ \mu },\omega ^{a}_{\ b\mu })\) the field equation must be valid for Einstein equation. Under the general spherically symmetric solution \((h^{a}_{\ \mu },\omega ^{a}_{\ b\mu })\), for any Lagrangian f that satisfies the spherically symmetric field equation \({\mathcal {W}}_{(\mu \nu )}[f]=8\pi G\varTheta _{\mu \nu }=0\) for \(\mu \ne \nu \), we can always define a new Lagrangian \(\tilde{f}=f+{\mathcal {T}}\) that must satisfy the field equation \({\mathcal {W}}_{(\mu \nu )}[\tilde{f}]=0\) for \(\mu \ne \nu \) as well. In this case, we find the difference between \({\mathcal {W}}_{(\mu \nu )}[f]\) and \({\mathcal {W}}_{(\mu \nu )}[\tilde{f}]\) is \({\mathcal {W}}_{(\mu \nu )}[\tilde{f}]-{\mathcal {W}}_{(\mu \nu )}[f]=\mathring{G}_{\mu \nu }\), thus, we have to require \(\mathring{G}_{\mu \nu }=0\) for \(\mu \ne \nu \). This result can be understood as the condition of spherical symmetry must be valid for all Lagrangian including \(f=-{\mathcal {T}}+{\mathcal {B}}\), which is just the teleparallel equivalent of General Relativity satisfying Einstein equation \(\mathring{G}_{\mu \nu }=8\pi GT_{\mu \nu }\) with vanishing off-diagonal components to the general spherical symmetric solution \((h^{a}_{\ \mu },\omega ^{a}_{\ b\mu })\). In this case, we get equation

$$\begin{aligned} \mathring{G}_{01}=0\longrightarrow Y'\frac{\dot{B}}{B} +\dot{Y}\frac{A'}{A}-\dot{Y}'=0. \end{aligned}$$
(23)

Other off-diagonal components of \(\mathring{G}_{\mu \nu }\) vanish under the diagonal metric.

3.2 The solutions to the field equation

The local solution of the field equation under spherical symmetry condition depends on hypersurface Y=constant [49]. Thus, we can take \(Y=r\), where r is the area parameter for 2 dimensional hypersurface \(\varOmega _{2}\) which has area=\(4\pi r^{2}\). In this case \(Y'=1\) and \(\dot{Y}=0\), the 01 component of Einstein equation tells us

$$\begin{aligned} \dot{B}=0. \end{aligned}$$
(24)

Now we come to discuss field equations. There are only 2 non-zero components of anti-symmetric field equation, 01 and 23 components. The 01 component of anti-symmetric field equation is

$$\begin{aligned} {\mathcal {W}}_{[01]}&=\frac{B\cos {\varphi }\cosh {\alpha }-1}{2r} \partial _{t}(f_{{\mathcal {T}}}+f_{{\mathcal {B}}})\nonumber \\&\quad -\frac{A\cos {\varphi }\sinh {\alpha }}{2r}\partial _{r} (f_{{\mathcal {T}}}+f_{{\mathcal {B}}})=0. \end{aligned}$$
(25)

The 23 component of anti-symmetric field equation is

$$\begin{aligned} {\mathcal {W}}_{[23]}&=\frac{r\sin \theta \sin \varphi \sinh \alpha }{2A} \partial _{t}(f_{{\mathcal {T}}}+f_{{\mathcal {B}}})\nonumber \\&\quad -\frac{r\sin \theta \sin \varphi \cosh \alpha }{2B} \partial _{r}(f_{{\mathcal {T}}}+f_{{\mathcal {B}}})=0. \end{aligned}$$
(26)

The other components of anti-symmetric field equation are all zero. We combine \({\mathcal {W}}_{[23]}\) with \({\mathcal {W}}_{[01]}\), then we have

$$\begin{aligned} \left( \frac{B\cos \varphi }{\cosh \alpha }-1\right) \partial _{t} (f_{{\mathcal {T}}}+f_{{\mathcal {B}}})&=0\nonumber \\ \left( \frac{B\cos \varphi }{\cosh \alpha }-1\right) \partial _{r}(f_{{\mathcal {T}}}+f_{{\mathcal {B}}})&=0 \end{aligned}$$
(27)

if we assume \(\sin \varphi \ne 0\) in (26). From this, we can only require

$$\begin{aligned} B(r)=\frac{\cosh \alpha (t,r)}{\cos \varphi (t,r)} \end{aligned}$$
(28)

because \(f_{{\mathcal {T}}}+f_{{\mathcal {B}}}\) is function of t and r, it cannot be zero with derivative of both t and r. However, this does not make sense. This relation implies that the dynamical function B is a pure gauge parameter, which comes from the Lorentz transformation. If so, then there is only A is a dynamical component in metric, which is obviously incorrect. Then to make the function reasonable and to make \({\mathcal {W}}_{[23]}=0\), we can only require

$$\begin{aligned} \sin \varphi =0 \end{aligned}$$
(29)

for (26). In this case, from the 01 and 23 components of anti-symmetric field equation we fix the gauge of \(\varphi =0\). For symmetric field equations, they have only one non-vanishing off-diagonal component, which is given by

$$\begin{aligned} {\mathcal {W}}_{(01)}&=\mathring{\nabla }_{r}\mathring{\nabla }_{t} f_{{\mathcal {B}}}+\frac{B\cosh {\alpha }-1}{2r}\partial _{t} (f_{{\mathcal {T}}}+f_{{\mathcal {B}}})\nonumber \\&\quad +\frac{A\sinh {\alpha }}{2r}\partial _{r}(f_{{\mathcal {T}}} +f_{{\mathcal {B}}})=0, \end{aligned}$$
(30)

while other off-diagonal components of symmetric field equation are all zero. We note that for Lagrangian f that satisfies symmetric field equation \({\mathcal {W}}_{(\mu \nu )}[f]=0\) we can always define a new Lagrangian \(\tilde{f}=f+H({\mathcal {T}})\) that satisfies \({\mathcal {W}}_{(\mu \nu )}[\tilde{f}]=0\) as well. From (21) we learn that the \({\mathcal {W}}_{(\mu \nu )}[\tilde{f}]\) is given by

$$\begin{aligned}&{\mathcal {W}}_{(\mu \nu )}[\tilde{f}]\nonumber \\&\quad = {\mathcal {W}}_{(\mu \nu )}[f] +\left( \mathring{G}_{\mu \nu }H_{{\mathcal {T}}} +\frac{1}{2}g_{\mu \nu }(H-{\mathcal {T}}H_{{\mathcal {T}}}) +S_{(\mu \nu )}^{\ \ \rho }\partial _{\rho }H_{{\mathcal {T}}}\right) \nonumber \\&\quad ={\mathcal {W}}_{(\mu \nu )}[f]+8\pi GK_{(\mu \nu )}[H], \end{aligned}$$
(31)

where \(K_{\mu \nu }\) is the corresponding energy-momentum tensor with respect to added Lagrangian \(H({\mathcal {T}})\), and the anti-symmetric part

$$\begin{aligned} {\mathcal {W}}_{[\mu \nu ]}[\tilde{f}]={\mathcal {W}}_{[\mu \nu ]}[f] +S_{[\mu \nu ]}^{\ \ \ \ \rho }\partial _{\rho }H_{{\mathcal {T}}}\equiv 0 \end{aligned}$$
(32)

is supposed to be zero identically. In this case, only 01 component in off-diagonal components of symmetric field equation (30) with additional \(H({\mathcal {T}})\) is non-zero, which is given by

$$\begin{aligned} \frac{B\cosh \alpha -1}{2r}\partial _{t}H_{{\mathcal {T}}}=0. \end{aligned}$$
(33)

The solution \(B=1/\cosh \alpha \) is equivalent to say dynamical function B is a pure gauge thus it is redundant, which is incorrect. The correct solution is

$$\begin{aligned} \partial _{t}H_{{\mathcal {T}}}=0. \end{aligned}$$
(34)

Only 01 component in off-diagonal components of anti-symmetric field equation (25) is non-zero, which then becomes

$$\begin{aligned} \frac{A\sinh \alpha }{2r}\partial _{r}H_{{\mathcal {T}}}=0, \end{aligned}$$
(35)

which tells us \(\sinh \alpha =0\) because \(H_{{\mathcal {T}}}\) is a function of t and r, it cannot generally satisfies \(\partial _{t}H_{{\mathcal {T}}}=0\) and \(\partial _{r}H_{{\mathcal {T}}}=0\) at the same time. The \(H({\mathcal {T}})\) is a function of tensors \({\mathcal {T}}\), which is given by

$$\begin{aligned} {\mathcal {T}}=\frac{2(1-B)}{r^{2}B^{2}} \left[ 2r\left( \frac{A'}{A}\right) +1-B\right] . \end{aligned}$$
(36)

In this case, the equation (34) can be written as

$$\begin{aligned} \partial _{t}H_{\mathcal {T}}=\partial _{t}\left( \frac{A'}{A}\right) \partial _{A'/A}H_{\mathcal {T}}=0. \end{aligned}$$
(37)

We find \(A'/A\) is independent of t. Thus, we have \(A(t,r)=A_{t}(t)A_{r}(r)\). We can define a new time parameter by setting \(d\tilde{t}=A_{t}(t)dt\). Together with \(B=B(r)\), the line element

$$\begin{aligned} ds^{2}=A_{t}^{2}(r)d\tilde{t}^{2}-B^{2}(r)dr^{2}+r^{2} (d\theta ^{2}+\sin ^{2}\theta d\phi ^{2}) \end{aligned}$$
(38)

describes a static spacetime. With these solutions, we find the off-diagonal components of symmetric field equation (30) and anti-symmetric part of field equation (25) vanish identically. Thus, the solution we find is general and complete for modified telerapallel theories of gravity.

3.3 Weitzenböck gauge

In some previous discussions of the Birkhoff’s theorem in \(f({\mathcal {T}},{\mathcal {B}})\) gravity, the authors have always started with the solution (16) under Weitzenböck gauge [56]. However, this form of vierbein is not so good for proving the Birkhoff’s theorem, because treating all 6 functions as dynamical functions would mask the redundant variable relationships that exist inside them. The existence of these internally gauge relationships requires additional equations to determine. Therefore, to fix the redundant parameters we can act the solution with a moderate transformation. In this way we separate 6 functions into 3 dynamical functions and 3 gauge parameters. In principle we cannot establish an equivalent relation between dynamical functions and redundant parameters. In this way we are able to solve the field equations correctly. The corresponding line element is given by

$$\begin{aligned} ds^{2}&=(C_{1}^{2}-C_{3}^{2})dt^{2}+(C_{2}^{2}-C_{4}^{2})dr^{2}\nonumber \\&\quad +(C_{3}C_{4}-C_{1}C_{2})dtdr-(C_{5}^{2}+C_{6}^{2})d\varOmega _{2}^{2}, \end{aligned}$$
(39)

where the differential form of 2-sphere is defined as \(d\varOmega _{2}^{2}=d\theta ^{2}+\sin ^{2}\theta d\phi ^{2}\). To make the metric diagonal we usually require \(C_{3}C_{4}-C_{1}C_{2}=0\) [15, 56]. In our previous discussion we identified redundant parameters \(\alpha =\beta =\varphi =0\), plug them into \(C_{1}\sim C_{6}\), and we can fix 3 functions of

$$\begin{aligned} C_{2}=C_{3}=C_{6}=0. \end{aligned}$$
(40)

This is the same as the result in [56]. However, we note that \(\partial _{t}(f_{{\mathcal {T}}} +f_{{\mathcal {B}}})=0\) from (33). It should also correct under Weitzenböck gauge condition, otherwise we have to require \(B=1/\cosh \alpha \) with \(\alpha =0\) to let 01 component of anti-symmetric field equation zero, which will lead us to a wrong place. In this case, the corresponding line element becomes

$$\begin{aligned} ds^{2}=C_{1}^{2}(t,r)dt^{2}-C_{4}^{2}(t,r)dr^{2}-r^{2}d\varOmega _{2}^{2} \end{aligned}$$
(41)

on \(C_{5}\)=constant hypersurface. We can repeat what we did in Sect. 3.2 for the symmetric part of field equations and use the condition \(\partial _{t}(f_{{\mathcal {T}}} +f_{{\mathcal {B}}})=0\), and we will get the same result

$$\begin{aligned} \dot{C}_{4}=0,\quad \partial _{t}\left( \frac{C_{1}'}{C_{1}}\right) =0. \end{aligned}$$
(42)

The corresponding solution are \(C_{4}=C_{4}(r)\) and \(C_{1}=C_{1,t}(t)C_{1,r}(r)\). The line element now becomes

$$\begin{aligned} ds^{2}=C_{1,r}^{2}(r)d\tilde{t}^{2}-C_{4}^{2}(r)dr^{2} +r^{2}d\varOmega _{2}^{2}, \end{aligned}$$
(43)

in which \(d\tilde{t}=C_{1,t}(t)dt\). The validity of Birkhoff’s theorem is proved under Weitzenböck gauge spherically symmetric solution (16).

3.4 Discussion

In our process of proof, we first get the most general form of solution \((h^{a}_{\ \mu },\omega ^{a}_{\ b\mu })\) by spherical symmetry. Such solution has 6 undetermined functions \(C_{1}\sim C_{6}\) [44, 56]. However, we find actually only 3 of them are dynamical and the other 3 are gauge, we can make a strict distinction between them by this essential difference. Without this distinction, it will be hard to find that Eq. (28) is actually an incorrect solution. Thus, this distinction is actually the most critical step in our proof process. Our proof reveals that the only solution derived from the consistency of the SO(3) spherical symmetric solution, the energy-momentum tensor, and the symmetric and anti-symmetric field equations is static. To be precise, when we require the off-diagonal components of energy-momentum tensor vanish, The corresponding field equation should be equal to zero identically for all forms of Lagrangian f. The fact that not restricting the form of f is an important requirement for a theorem to hold in \(f({\mathcal {T}},{\mathcal {B}})\) gravity has helped us to obtain some important results. We stress that the local solution of the field equation under spherical symmetry condition depends on hypersurface Y=constant, that makes us take \(Y=r\). For correctness, we examine all off-diagonal components of symmetric field equation and each component of anti-symmetric field equation. Eventually, we find that the only solution that makes these equations work is that, for gauge parameters \(\alpha =\beta =\varphi =0\), and for dynamical functions \(A=A(r)\) and \(B=B(r)\), which make the Birkhoff’s theorem be valid in \(f({\mathcal {T}},{\mathcal {B}})\) gravity.

The proof method we use can be directly applied to \(f({\mathcal {T}})\) gravity, where our conclusion is still universal, which is consistent with the result of [68, 69] that the same statement of Birkhoff’s theorem also holes in \(f({\mathcal {T}})\) gravity. Compared with the previous discussion, our proof method does not depend on the selection of gauge, and our proof is more general and universal.

4 Conclusion

In this article, we investigate the Birkhoff’s theorem within modified teleparallel equivalent theories of gravity. We allow \(f({\mathcal {T}},{\mathcal {B}})\) to contain a cosmological constant \(\kappa \) in Lagrangian and the Birkhoff’s theorem should still be valid. Our result is very general and does not depend on specific boundary conditions used for solving the field equation. The validity of the Birkhoff’s theorem reveals an important reason for the stability of the spherically symmetric spacetimes. The validity of Birkhoff’s theorem rises the problem of how a universe consists of locally static objects while it can expand. This casts doubt on our understanding of the spherically symmetric composition on the vary large scale of the universe, because an interesting thing worth pointing out is that we do not strictly require spacetime to be vacuum to make the Birkhoff’s theorem valid. In our progress we require the off-diagonal components of energy-momentum tensor vanish as long as the matter distribution in spacetime is spherically symmetric. This does not contradict the fact that the Birkhoff’s theorem holds in teleparallel equivalent \(f(-{\mathcal {T}}+{\mathcal {B}})=f(\mathring{R})\) gravity while it is valid in \(f(\mathring{R})\) gravity only when spacetime is an approximate vacuum or approximately spherically symmetric [48, 51,52,53,54]. To this extent, there is a good agreement between the Birkhoff’s theorem in these two theories of gravity.

On the other hand, in point of view of classical physics, it is not surprising that a vacuum spacetime is static. More specifically, in vacuum spacetime \(\partial _{t}\) is a killing vector, which implies the energy of the field is conserved. But we know from black hole physics that the particle creation on the horizon of a black hole contribute to the black hole evaporation, which makes the energy locally non-conserved. These processes cannot be described in classical theories of gravity. The Birkhoff’s theorem is a foregone conclusion in the theory of gravity, which is a classically physical view to the vacuum, so it is not surprising that the vacuum spacetime solution is static.

The teleparallel equivalent theories of gravity is very different from General Relativity. The vacuum spacetime in a modified theory of gravity and the spacetime background should have a more diversified structure than in General Relativity. Accordingly, the physical forms of black holes in the \(f({\mathcal {T}},{\mathcal {B}})\) gravity could be much abundant. Finding black hole solutions in gravitational theory has always been an important problem, and in teleparallel theories of gravity, we can only find numerical solutions and cannot get analytical solutions other than Schwarzschild solution, because we take vierbein as the base element in it, which gives our field equation more degrees of freedom than General Relativity. The establishment of Birkhoff’s theorem will deepen our understanding of spacetime structure especially black holes in modified teleparallel equivalent theories of gravity.