Corrections to local-density approximation for superfluid trapped fermionic atoms from the Wigner-Kirkwood $\hbar$ expansion

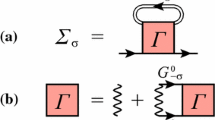
Abstract

A semiclassical second-order differential equation for the inhomogeneous local gap $\Delta (\varvec{r})$ is derived from a strict second-order $\hbar$ expansion of the anomalous pairing tensor and compared with a similar equation given in Simonucci and Strinati (in Phys Rev B 89:054511, 2014). The second-order normal density matrix is given as well. Several extra gradient terms are revealed. Second-order expressions at finite temperature are given for the first time. The corresponding Ginzburg–Landau equation is presented and it is shown that, compared to the equation of Baranov and Petrov (in Phys Rev A 58:R801, 1998), an extra second-order gradient term is present. Applications to the pairing gap in cold atoms in a harmonic trap are discussed.

Appendices

A Explicit expressions for the $Y^\kappa _i$ and $Y^\rho _i$ coefficients at $T=0$

As we have mentioned in the main text, the $\varvec{p}$ integrals of the pair and normal density matrices (6) and (9) can be performed analytically at zero temperature. However, it will turn out that there are certain terms which are divergent in the limit $\Delta \rightarrow 0$. We report here the corresponding values $Y^\kappa _i$ as they are obtained from Pei (including all divergent terms) [9].

The functions $Y^\kappa _i$ and $Y^\rho _i$ can be expressed in terms of two functions $I_5(x_0)$ and $I_6(x_0)$, where $x_0=\mu (\varvec{r})/\Delta (\varvec{r})$, which in turn can be written in terms of two complete elliptic integrals as follows [1, 16]:

$$\begin{aligned} I_5(x_0)= & {} (1 + x_0^2)^{1/4} E\big (\tfrac{\pi }{2},\kappa \big ) -\frac{F\big (\tfrac{\pi }{2},\kappa \big )}{4 x_1^2(1+x_0^2)^{1/4}}, \end{aligned}$$

(32)

$$\begin{aligned} I_6(x_0)= & {} \frac{1}{2(1+x_0^2)^{1/4}} F\big (\tfrac{\pi }{2},\kappa \big ), \end{aligned}$$

(33)

where $x_1^2 = (\sqrt{1 + x_0^2} + x_0)/2$ and $\kappa ^2=x_1^2/\sqrt{1+x_0^2}$, while $F(\pi /2,\kappa )$ and $E(\pi /2,\kappa )$ are the complete elliptic integrals of first and second kind defined by [17, 18]

$$\begin{aligned} F(\alpha ,\kappa )= & {} \int _0^{\alpha } d\phi \frac{1}{\sqrt{1 - \kappa ^2 \sin ^2 \phi }}, \end{aligned}$$

(34)

$$\begin{aligned} E(\alpha ,\kappa )= & {} \int _0^{\alpha } d\phi \sqrt{1 - \kappa ^2 \sin ^2 \phi }, \end{aligned}$$

(35)

with $\kappa ^2 < 1$. The main properties of these elliptic integrals are given in appendix A of [16].

If we write for brevity $I_5$ and $I_6$ for $I_5(x_0)$ and $I_6(x_0)$, respectively, the analytical expressions for the $Y^\kappa _i$ functions read

$$\begin{aligned} Y^\kappa _1(\varvec{r})= & {} \frac{1}{144\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big )^{1/2} \frac{1}{\sqrt{\Delta }}\nonumber \\{} & {} \times \frac{(10x_0^2+7)I_6+(4x_0^2+1)x_0I_5}{1+x_0^2}, \end{aligned}$$

(36)

$$\begin{aligned} Y^\kappa _2(\varvec{r})= & {} -\frac{1}{576\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big )^{1/2} \frac{1}{\Delta \sqrt{\Delta }}\nonumber \\{} & {} \times \frac{(4x_0^4+23x_0^2+7)I_6+(16x_0^4+38x_0^2+10)x_0I_5}{(1+x_0^2)^2},\quad \end{aligned}$$

(37)

$$\begin{aligned} Y^\kappa _3(\varvec{r})= & {} -\frac{1}{48\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big )^{1/2} \frac{1}{\sqrt{\Delta }}\frac{I_5-x_0I_6}{1+x_0^2}, \end{aligned}$$

(38)

$$\begin{aligned} Y^\kappa _4(\varvec{r})= & {} \frac{1}{192\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big )^{1/2} \frac{1}{\Delta \sqrt{\Delta }}\frac{(3x_0^2-1)I_6-4x_0I_5}{(1+x_0^2)^2}, \end{aligned}$$

(39)

$$\begin{aligned} Y^\kappa _5(\varvec{r})= & {} - \frac{1}{24\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big )^{1/2} \sqrt{\Delta }I_6, \end{aligned}$$

(40)

$$\begin{aligned} Y^\kappa _6(\varvec{r})= & {} \frac{7}{192\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big )^{1/2} \sqrt{\Delta }I_6, \end{aligned}$$

(41)

$$\begin{aligned} Y^\kappa _7(\varvec{r})= & {} - \frac{1}{96\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big )^{1/2} \frac{1}{\Delta \sqrt{\Delta }}\nonumber \\{} & {} \times \frac{(4x_0^4+11x_0^2+3)I_5-(2x_0^2-2)x_0I_6}{(1+x_0^2)^2}, \end{aligned}$$

(42)

$$\begin{aligned} Y^\kappa _8(\varvec{r})= & {} \frac{1}{96\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big )^{1/2} \frac{1}{\sqrt{\Delta }}\frac{I_5-x_0I_6}{1+x_0^2}, \end{aligned}$$

(43)

$$\begin{aligned} Y^\kappa _9(\varvec{r})= & {} - \frac{1}{96\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big )^{1/2} \frac{1}{\sqrt{\Delta }} \nonumber \\{} & {} \times \frac{(10x_0^2+7)I_6-(4x_0^2+1)x_0I_5}{1+x_0^2}, \end{aligned}$$

(44)

In our study of the pairing in finite systems it is actually relevant to know the $\Delta \rightarrow 0$ limit because the gap goes to zero at the surface. In this case the auxiliary functions $I_5(x_0)$ and $I_6(x_0)$ behave as $I_5(x_0) \simeq \sqrt{x_0} \simeq 1/\sqrt{\Delta }$ and $I_6(x_0)\simeq \ln (8x_0)/(2 \sqrt{x_0}) \simeq \sqrt{\Delta }\ln \Delta$, respectively [16]. This implies that, as already mentioned, in the limit $\Delta \rightarrow 0$ some of the $Y^\kappa _i$ functions defined previously are divergent. For example, in the function $Y^\kappa _1$ (36) the leading term in this limit behaves as $\nabla ^2 \Delta /\Delta ^2$ and therefore diverges.

It is easy to show that the $\hbar ^0$ (Thomas-Fermi) contribution to the normal density in the presence of the pairing field is given by [1, 16]

$$\begin{aligned} \rho _0(\varvec{r}){} & {} = \int \!\! \frac{d^3p}{(2\pi \hbar )^3} \frac{1}{2}\bigg [ 1 - \frac{h(\varvec{r},\varvec{p})}{E(\varvec{r},\varvec{p})}\bigg ] \nonumber \\{} & {} = \frac{1}{6\pi ^2} \bigg (\frac{2m^*\Delta }{\hbar ^2}\bigg )^{3/2}[I_6 + x_0 I_5]. \end{aligned}$$

(45)

The contributions to the $\hbar ^2$ corrections of the normal density $\rho _2$ as given in Pei [9] read

$$\begin{aligned} Y^\rho _1(\varvec{r})= & {} \frac{1}{48\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big )^{1/2} \frac{1}{\sqrt{\Delta }}\frac{(x_0^2+3)I_5-3x_0I_6}{1+x_0^2}, \end{aligned}$$

(46)

$$\begin{aligned} Y^\rho _2(\varvec{r})= & {} - \frac{1}{192\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big ) ^{1/2}\frac{1}{\Delta \sqrt{\Delta }}\nonumber \\{} & {} \times \frac{(4x_0^4+5x_0^2+5)I_5+(2x_0^2-2)x_0I_6}{(1+x_0^2)^2}, \end{aligned}$$

(47)

$$\begin{aligned} Y^\rho _3(\varvec{r})= & {} - \frac{1}{48\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big )^{1/2} \frac{1}{\sqrt{\Delta }}\frac{I_6+x_0I_5}{1+x_0^2}, \end{aligned}$$

(48)

$$\begin{aligned} Y^\rho _4(\varvec{r})= & {} - \frac{1}{192\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big )^{1/2} \frac{1}{\Delta \sqrt{\Delta }}\nonumber \\{} & {} \times \frac{(x_0^2-3)I_5+4x_0I_6}{(1+x_0^2)^2}, \end{aligned}$$

(49)

$$\begin{aligned} Y^\rho _5(\varvec{r})= & {} - \frac{1}{24\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big )^{1/2} \sqrt{\Delta }I_5, \end{aligned}$$

(50)

$$\begin{aligned} Y^\rho _6(\varvec{r})= & {} \frac{7}{192\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big )^{1/2} \sqrt{\Delta }I_5, \end{aligned}$$

(51)

$$\begin{aligned} Y^\rho _7(\varvec{r})= & {} - \frac{1}{96\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big )^{1/2} \frac{1}{\Delta \sqrt{\Delta }}\nonumber \\{} & {} \times \frac{(3x_0^2-1)I_6-4x_0I_5}{(1+x_0^2)^2}, \end{aligned}$$

(52)

$$\begin{aligned} Y^\rho _8(\varvec{r})= & {} \frac{1}{96\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big )^{1/2} \frac{1}{\sqrt{\Delta }}\frac{I_6+x_0I_5}{1+x_0^2}, \end{aligned}$$

(53)

$$\begin{aligned} Y^\rho _9(\varvec{r})= & {} - \frac{1}{96\pi ^2}\Big (\frac{2m^*}{\hbar ^2}\Big )^{1/2} \frac{1}{\sqrt{\Delta }}\frac{I_5-3x_0I_6}{1+x_0^2}. \end{aligned}$$

(54)

In the $\Delta \rightarrow 0$ limit, only the $Y^\rho _3$, $Y^\rho _4$, $Y^\rho _5$, $Y^\rho _6$, and $Y^\rho _8$ terms in $\rho _2(\varvec{r})$ survive, because the others are multiplied by gradients of $\Delta$. If we furthermore consider $m^* = m$, only $Y^\rho _3$ and $Y^\rho _4$ are relevant. Taking into account the asymptotic behaviour of $I_5(x_0)$ and $I_6(x_0)$ in the limit $\Delta \rightarrow 0$, the normal density in this case agrees with the well-known normal density given by Eq. (13.44) of Ref. [3].

B $2\times 2$ generalized density matrix from Ref. [6]

The finite temperature expressions of section 2 can be straightforwardly derived from Eqs. (5.5) and (5.7) of [6]. The zeroth order of the $2\times 2$ generalized density matrix is given by

$$\begin{aligned} {\mathcal {R}}_0 = \frac{1}{2}\bigg [ {\textsf{I}}+ \frac{{\mathcal {H}}}{E}(1-2f(E)) \bigg ]. \end{aligned}$$

(55)

Proceeding with the expression (5.7) in [6] in the same way, we obtain

$$\begin{aligned} {\mathcal {R}}_2(E)= & {} \bigg [ g_1\frac{{\mathcal {H}}}{E} - g_2\frac{{\mathcal {F}}}{E}\bigg ](1-2f(E))\nonumber \\{} & {} +\bigg [ g_7\frac{{\mathcal {H}}}{E} + g_8\frac{{\mathcal {F}}}{E}\bigg ] 2\frac{\partial ^2f(E)}{\partial E^2} + g_{10}\frac{{\mathcal {H}}}{E} 2\frac{\partial ^3 f(E)}{\partial E^3},\nonumber \\ \end{aligned}$$

(56)

where

$$\begin{aligned} {\mathcal {H}}= \begin{pmatrix} h&{}\Delta \\ \Delta &{}-h\end{pmatrix} ,\qquad {\mathcal {F}}= \begin{pmatrix} -\Delta &{}h \\ h&{}\Delta \end{pmatrix}. \end{aligned}$$

(57)

Finally, this leads to Eqs. (6) and (9) of the main text with the $g_i$ expressed in terms of the $f_i$ given in [6]. Please notice that in [6] there is a sign misprint and $g_2$ should be replaced by $-g_2$ there.