Accessing weak neutral-current coupling $g_{AA}^{eq}$ using positron and electron beams at Jefferson Lab

Abstract

Low-energy neutral-current couplings arising in the Standard Model of electroweak interactions can be constrained in lepton scattering off hydrogen or a nuclear fixed target. Recent polarized electron scattering experiments at Jefferson Lab (JLab) have improved the precision in the parity-violating types of effective couplings. On the other hand, the only known way to access the parity-conserving counterparts is to compare scattering cross sections between a lepton and an anti-lepton beam. We review the current knowledge of both types of couplings and how to constrain them. We also present exploratory calculations for a possible measurement of $g_{AA}^{eq}$ using the planned SoLID spectrometer combined with a possible positron beam at JLab.

Appendix A: Neutral-current DIS asymmetries

In this appendix, we derive asymmetries of lepton deep inelastic scattering off a nuclear target arising from the interference between electromagnetic and weak neutral current (NC) interactions. We are interested in the case of relatively small momentum transfer.

1.1 Neutral-current weak interaction Lagrangian

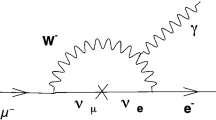
The kinematics of lepton-quark scattering is illustrated in Fig. 6, where the photon-fermion vertex is given by $-ie\gamma ^\mu Q_f$ with $Q_f$ the fermion electric charge in units of $e=\sqrt{4\pi \alpha }$. Likewise, the Z-fermion vertex reads,

$$\begin{aligned} -i\frac{g}{2\cos \theta _W}\gamma ^\mu \left( g_V^f - g_A^f\gamma ^5\right) \ . \end{aligned}$$

(A.1)

The matrix element for NC eq scattering can now be written,

$$\begin{aligned} \frac{1}{i}\mathcal {M}_{NC} =&{\bar{l}}_f \left[ -i\frac{g}{2\cos \theta _W} \gamma ^\mu \left( g_V^l - g_A^l\gamma ^5\right) \right] l_i \nonumber \\&\times \left( -i\frac{g_{\mu \nu } - \frac{q_\mu q_\nu }{M_Z^2}}{q^2 - M_Z^2} \right) {\bar{q}}_f \nonumber \\&\times \left[ -i\frac{g}{2\cos \theta _W} \gamma ^\nu \left( g_V^q - g_A^q\gamma ^5\right) \right] q_i\ , \end{aligned}$$

(A.2)

where $M_Z$ is the Z boson mass, and $l_{i,f}$ and $q_{i,f}$ are the Dirac spinors for the initial and final state electrons and quarks, respectively. At the SM tree level, the gauge coupling g is related to $G_F$,

$$\begin{aligned} \frac{G_F}{\sqrt{2}} = \frac{g^2}{8M_Z^2\cos ^2\theta _W}\ , \end{aligned}$$

(A.3)

so that for small momentum transfer, $\vert q^2\vert \ll M_Z^2$, and dropping the vector-vector interactions, the amplitude in Eq. (A.2) derives from the neutral-current weak interaction Lagrangian

$$\begin{aligned} \mathcal {L}_{int}^{NC}= & {} \frac{G_F}{\sqrt{2}} \left[ g_{VV}^{eq}\ {\bar{l}}_f \gamma ^\mu l_i {\bar{q}}_f \gamma _\mu q_i\ \right. \nonumber \\&+ \left. g_{VA}^{eq}\ {\bar{l}}_f \gamma ^\mu l_i {\bar{q}}_f \gamma _\mu \gamma ^5 q_i\ + g_{AV}^{eq}\ {\bar{l}}_f \gamma ^\mu \gamma ^5 l_i\ {\bar{q}}_f \gamma _\mu q_i \right. \nonumber \\&+ \left. g_{AA}^{eq}\ {\bar{l}}_f \gamma ^\mu \gamma ^5 l_i\ {\bar{q}}_f \gamma _\mu \gamma ^5 q_i \right] , \end{aligned}$$

(A.4)

where we used the same symbols for Dirac spinor fields and coefficient functions.

Fig. 6

One-photon exchange in electron-quark DIS. For the weak neutral current interaction, the photon is replaced by a Z boson

1.2 One-photon exchange amplitude and Z–$\gamma ^*$ interference

For incoming leptons with helicity h (the case of anti-leptons will be treated separately), we have the one-photon exchange amplitude,

$$\begin{aligned} \mathcal {M}_{\gamma }^h = - Q_l Q_q \frac{4\pi \alpha }{q^2} ({\bar{l}}_f\gamma ^\mu P_h l_i)({\bar{q}}_f\gamma _\mu q_i)\ , \end{aligned}$$

(A.5)

where,

$$\begin{aligned} P_h \equiv \frac{1+h\gamma ^5}{2}\ , \end{aligned}$$

(A.6)

is the projection operator for right-handed ($h = +1$) and left-handed ($h = -1$) leptons. The cross section of the electromagnetic process is,

$$\begin{aligned} \sum _{\mathrm {spins}}\vert \mathcal {M}_h^\gamma \vert ^2= & {} 16 Q_l^2 Q_q^2\left( \frac{4\pi \alpha }{q^2}\right) ^2 \nonumber \\&\times \left[ (Pk)(P'k') + (Pk')(P'k) \right] . \end{aligned}$$

(A.7)

For the weak neutral current interaction we have,

$$\begin{aligned} \mathcal {M}_{NC}^h= & {} \sqrt{2}G_F \left[ {\bar{l}}_f \gamma ^\mu \left( g_V^l - g_A^l\gamma ^5\right) P_h l_i\right] \nonumber \\&\times \left[ {\bar{q}}_f\gamma _\mu \left( g_V^q-g_A^q\gamma ^5\right) q_i \right] , \end{aligned}$$

(A.8)

so that the interference term is given by,

$$\begin{aligned}&\left( \mathcal {M}_\gamma ^h\right) ^*\mathcal {M}_{NC}^h \nonumber \\&\quad = - \frac{4\sqrt{2}\pi G_F\alpha }{q^2}Q_l Q_q ({\bar{l}}_i P_{-h} \gamma ^\mu l_f) \nonumber \\&\qquad \times \left[ {\bar{l}}_f\gamma ^\nu (g_V^l - g_A^l \gamma ^5) P_h l_i \right] ({\bar{q}}_i\gamma _\mu q_f)\left[ {\bar{q}}_f\gamma _\nu (g_V^q - g_A^q \gamma ^5) q_i \right] .\nonumber \\ \end{aligned}$$

(A.9)

Averaging over initial and summing over the final spin states,

$$\begin{aligned}&\sum _{\mathrm {spins}} \left( \mathcal {M}_{\gamma }^{h}\right) ^*\mathcal {M}_{NC}^h\nonumber \\&\quad = - \frac{64\sqrt{2}\pi G_F\alpha }{q^2}Q_l Q_q \nonumber \\&\qquad \times \left\{ (kP)(k'P') \left[ g_V^l g_V^q - h g_A^l g_V^q - h g_V^l g_A^q + g_A^l g_A^q \right] \right. \nonumber \\&\qquad +\left. (kP')(k'P) \left[ g_V^l g_V^q - h g_A^l g_V^q + h g_V^l g_A^q - g_A^l g_A^q \right] \right\} \, .\nonumber \\ \end{aligned}$$

(A.10)

Likewise, denoting the anti-lepton coefficient functions by $v_{i,f}$, for incoming anti-leptons with helicity h the leptonic currents in Eqs. (A.5) and (A.8) are now $(\bar{v}_iP_h\gamma ^\mu v_f)$ and $\left[ {\bar{v}}_iP_h \gamma ^\mu \left( g_V^l-g_A^l\gamma ^5\right) v_f\right]$, respectively. Eq. (A.10) then also applies for anti-leptons as long as one substitutes $h\rightarrow -h$ and $g_A^q\rightarrow -g_A^q$.

For anti-quarks at the $\gamma$ or Z vertex one substitutes analogously the quark-coefficient functions by those of anti-quarks, with the result that Eq. (A.10) applies when one replaces $g_A^q\rightarrow -g_A^q$ in the case of lepton scattering. Finally, for anti-lepton scattering off anti-quarks one needs to substitute $h\rightarrow -h$ in Eq. (A.10).

1.3 Electroweak neutral current cross section asymmetries

In DIS one has to combine the scattering cross sections for all quarks in the target with weights according to the PDFs. From the definition in Eq. (9) we have,

$$\begin{aligned} A_{RL}^{e^\pm }= & {} \frac{\vert \mathcal {M}_Z+\mathcal {M}_\gamma \vert _{h=+\vert \lambda \vert }^2 - \vert \mathcal {M}_Z + \mathcal {M}_\gamma \vert _{h=-\vert \lambda \vert }^2}{\vert \mathcal {M}_Z + \mathcal {M}_\gamma \vert _{h=+\vert \lambda \vert }^2 + \vert \mathcal {M}_Z + \mathcal {M}_\gamma \vert _{h=-\vert \lambda \vert }^2} \nonumber \\\approx & {} \frac{(\mathcal {M}_\gamma ^*\mathcal {M}_Z)_{h=+\vert \lambda \vert } - (\mathcal {M}_\gamma ^*\mathcal {M}_Z)_{h=-\vert \lambda \vert }}{\vert \mathcal {M}_\gamma \vert ^2}\ . \end{aligned}$$

(A.11)

If we now use the previous results, inserting the Mandelstam variables neglecting lepton and quark masses, i.e., $s \equiv (k+P)^2 = 2 kP = 2 k'P'$ with $P_\mathrm{quark} = x P_\mathrm{nucleon}$, and likewise $u \equiv (k-P')^2 = -2 kP' = -2 k'P= -(1-y) s$,

$$\begin{aligned} A^{e^-}_{RL}= & {} \vert \lambda \vert \frac{G_F Q^2}{2\sqrt{2}\pi \alpha } \left\{ \frac{\sum q(x,Q^2)Q_q g_{AV}^{eq}[1+(1-y)^2]}{\sum q(x,Q^2) Q_q^2[1+(1-y)^2]} \right. \nonumber \\&+ \left. \frac{\sum q(x,Q^2)Q_q g_{VA}^{eq}[1-(1-y)^2]}{\sum q(x,Q^2) Q_q^2[1+(1-y)^2]} \right\} \ , \end{aligned}$$

(A.12)

where $\lambda$ is the beam polarization and $q(x,Q^2)$ are PDFs. The value $Q_l=-1$ for electron scattering, $Q^2 \equiv -q^2$, and the definitions of $g_{AV}^{eq}$ and $g_{VA}^{eq}$ were also used. We note that for the antiquark contributions the couplings $g_A^q$ (and therefore all $g_{VA}^{eq}$) appear with an extra minus sign. For a proton target we obtain,

$$\begin{aligned} A^{e^-}_{RL,p}= & {} \vert \lambda \vert \frac{3 G_F Q^2}{2\sqrt{2}\pi \alpha (4U^++D^+)} \left[ (2U^+ g_{AV}^{eu} - D^+ g_{AV}^{ed}) \right. \nonumber \\&+ \left. Y(2 u_V g_{VA}^{eu}-d_V g_{VA}^{ed}) \right] \ , \end{aligned}$$

(A.13)

where we have further abbreviated $U^+ \equiv u^+ + c^+$ and $D^+ \equiv d^+ + s^+$, and have assumed $c={\bar{c}}$ and $s={\bar{s}}$ (and thus $c_V=s_V=0$). The function Y is defined in Eq. (14) and we will omit its dependence on y hereafter. For deuterium or other isoscalar targets (ignoring nuclear effects), we substitute $u\rightarrow u+d$ and $d\rightarrow u+d$ in the expression for $A^{e^-}_{RL,p}$ above, and assume that c and s are the same in the proton and the neutron:

$$\begin{aligned} A^{e^-}_{RL,d}= & {} \vert \lambda \vert \frac{3 G_F Q^2}{2\sqrt{2}\pi \alpha (5 + 4 R_C + R_S)} \left\{ \left[ 2 (1 + R_C) g_{AV}^{eu} \right. \right. \nonumber \\&-\left. \left. (1 + R_S) g_{AV}^{ed} \right] +Y (2 g_{VA}^{eu} - g_{VA}^{ed}) R_V \right\} \ . \end{aligned}$$

(A.14)

Ignoring the heavier s and c quarks, these expressions simplify further,

$$\begin{aligned} A^{e^-}_{RL,p}\approx & {} \vert \lambda \vert \frac{3 G_F Q^2}{2 \sqrt{2}\pi \alpha (4u^+ + d^+)} \left[ (2 u^+ g_{AV}^{eu} - d^+ g_{AV}^{ed}) \right. \nonumber \\&+ \left. Y (2 u_V g_{VA}^{eu} - d_V g_{VA}^{ed}) \right] , \end{aligned}$$

(A.15)

$$\begin{aligned} A^{e^-}_{RL,d}\approx & {} \vert \lambda \vert \frac{3 G_F Q^2}{10 \sqrt{2}\pi \alpha } \left[ (2 g_{AV}^{eu} - g_{AV}^{ed}) \right. \nonumber \\&+ \left. R_V Y (2g_{VA}^{eu} - g_{VA}^{ed}) \right] . \end{aligned}$$

(A.16)

We now turn to the calculation of $A^{e^+e^-}_{RL}$,

$$\begin{aligned} A^{e^+e^-}_{RL}= & {} \frac{\vert \mathcal {M}_Z^{e^+}+\mathcal {M}_\gamma ^{e^+}\vert ^2_{h=+\vert \lambda \vert } -\vert \mathcal {M}_Z^{e^-}+\mathcal {M}_\gamma ^{e^-}\vert ^2_{h=-\vert \lambda \vert }}{\vert \mathcal {M}_Z^{e^+}+\mathcal {M}_\gamma ^{e^+}\vert ^2_{h=+\vert \lambda \vert } +\vert \mathcal {M}_Z^{e^-}+\mathcal {M}_\gamma ^{e^-}\vert ^2_{h=-\vert \lambda \vert }} \nonumber \\\approx & {} \frac{(\mathcal {M}_\gamma ^{e^+}\mathcal {M}_Z^{e^+})_{+\vert \lambda \vert } - (\mathcal {M}_\gamma ^{e^-}\mathcal {M}_Z^{e^-})_{-\vert \lambda \vert }}{\vert \mathcal {M}_\gamma \vert ^2}\ , \end{aligned}$$

(A.17)

where the approximation is valid for $Q^2 \ll M_Z^2$. For a nuclear target,

$$\begin{aligned} A^{e^+e^-}_{RL} = \frac{G_F Q^2Y\sum _q q_V Q_q (\vert \lambda \vert g_{VA}^{eq} - g_{AA}^{eq})}{2\sqrt{2}\pi \alpha \sum _q q^+ Q_q^2}\ , \end{aligned}$$

(A.18)

and likewise,

$$\begin{aligned} A^{e^+e^-}_{RR} = \frac{G_F Q^2\sum _q Q_q [{{-}}\vert \lambda \vert q^+ g_{AV}^{eq} -q_V Y g_{AA}^{eq}]}{2\sqrt{2}\pi \alpha \sum _q q^+ Q_q^2}\ . \end{aligned}$$

(A.19)

By substituting $\vert \lambda \vert \rightarrow -\vert \lambda \vert$ one can obtain $A^{e^+e^-}_{LR}$ from Eq. (A.18), and $A^{e^+e^-}_{LL}$ from Eq. (A.19). For $A^{e^+e^-}$ one can use (A.18) or (A.19) and set $\vert \lambda \vert =0$. For the proton,

$$\begin{aligned} A^{e^+e^-}_{RL,p}= & {} \frac{3 G_F Q^2 Y}{2\sqrt{2}\pi \alpha (4 U^+ \!+\! D^+)} \left[ \vert \lambda \vert (2 u_V g_{VA}^{eu} - d_V g_{VA}^{ed}) \right. \nonumber \\&- \left. ({2} u_V g_{AA}^{eu} - d_V g_{AA}^{ed}) \right] \ , \end{aligned}$$

(A.20)

$$\begin{aligned} A^{e^+e^-}_{RR,p}= & {} \frac{3 G_F Q^2}{2\sqrt{2}\pi \alpha ({4}U^+ + D^+)} \left[ {{-}}\vert \lambda \vert (2 U^+ g_{AV}^{eu} - D^+ g_{AV}^{ed}) \right. \nonumber \\&- \left. Y ({2}u_Vg_{AA}^{eu}-d_Vg_{AA}^{ed}) \right] \ , \end{aligned}$$

(A.21)

and for the deuteron,

$$\begin{aligned} A^{e^+e^-}_{RL,d}= & {} \frac{3 G_F Q^2 Y R_V}{2\sqrt{2}\pi \alpha (5 + 4 R_C + R_S)} \left[ \vert \lambda \vert (2 g_{VA}^{eu} - g_{VA}^{ed}) \right. \nonumber \\&- \left. (2 g_{AA}^{eu} - g_{AA}^{ed}) \right] \ , \end{aligned}$$

(A.22)

$$\begin{aligned} A^{e^+e^-}_{RR,d}= & {} \frac{3 G_F Q^2}{2\sqrt{2}\pi \alpha (5 + 4 R_C + R_S)} \left\{ {{-}}\vert \lambda \vert \left[ 2 (1 + R_C) g_{AV}^{eu} \right. \right. \nonumber \\&- \left. \left. (1 + R_S) g_{AV}^{ed} \right] - Y R_V (2 g_{AA}^{eu} - g_{AA}^{ed}) \right\} \ . \end{aligned}$$

(A.23)

Finally, if only u and d quarks are included,

$$\begin{aligned} A^{e^+e^-}_{RL,p}\approx & {} \frac{3 G_F Q^2 Y}{2\sqrt{2}\pi \alpha (4 u^+ + d^+)} \left[ \vert \lambda \vert (2 u_V g_{VA}^{eu} - d_V g_{VA}^{ed}) \right. \nonumber \\&- \left. (2 u_V g_{AA}^{eu} - d_V g_{AA}^{ed}) \right] \ , \end{aligned}$$

(A.24)

$$\begin{aligned} A^{e^+e^-}_{RR,p}\approx & {} \frac{3 G_F Q^2}{2\sqrt{2}\pi \alpha (4 u^+ + d^+)} \left[ {{-}}\vert \lambda \vert (2 u^+ g_{AV}^{eu} - d^+ g_{AV}^{ed}) \right. \nonumber \\&- \left. (2 u_V g_{AA}^{eu} - d_V g_{AA}^{ed}) Y \right] \ , \end{aligned}$$

(A.25)

and

$$\begin{aligned} A^{e^+e^-}_{RL,d}\approx & {} \frac{3 G_F Q^2 Y R_V}{10\sqrt{2}\pi \alpha } \left[ \vert \lambda \vert (2 g_{VA}^{eu} - g_{VA}^{ed}) \right. \nonumber \\&- \left. (2 g_{AA}^{eu} - g_{AA}^{ed}) \right] \ . \end{aligned}$$

(A.26)

$$\begin{aligned} A^{e^+e^-}_{RR,d}\approx & {} \frac{3 G_F Q^2}{10\sqrt{2}\pi \alpha } \left[ {{-}}\vert \lambda \vert (2 g_{AV}^{eu} - g_{AV}^{ed}) \right. \nonumber \\&- \left. Y R_V (2 g_{AA}^{eu} - g_{AA}^{ed}) \right] \ . \end{aligned}$$

(A.27)

The asymmetry measured at CERN on $^{12}$C was,

$$\begin{aligned} A^{\mu ^+\mu ^-}_{LR,C}= & {} - \frac{3 G_F Q^2 Y R_V}{2\sqrt{2}\pi \alpha (5 + 4 R_C + R_S)} \left[ (2 g_{AA}^{\mu u} - g_{AA}^{\mu d}) \right. \nonumber \\&+ \left. \vert \lambda \vert (2 g_{VA}^{\mu u} - g_{VA}^{\mu d}) \right] \ , \end{aligned}$$

(A.28)

while for SoLID we can use unpolarized beams to allow for higher intensities and measure on the deuteron,

$$\begin{aligned} A^{e^+e^-}_d = - \frac{3 G_F Q^2 Y}{2\sqrt{2}\pi \alpha } \frac{R_V (2 g_{AA}^{eu} - g_{AA}^{ed})}{5 + 4 R_C + R_S}\ . \end{aligned}$$

(A.29)