Nonlinear Dynamics of a Linear Dust Particle Chain

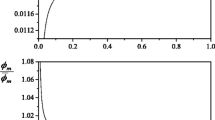
Abstract

The nonlinear stage of development of the dust particle chain instabilities is studied. It is assumed that dust particles interact with the plasma consisting of Maxwellian electrons and a directed flow of cold ions. The truncated equations are derived describing the nonlinear dynamics of the standing wave in the range of parameters close to the threshold for the development of the instability of coupled waves. It is shown that, in a narrow range of the ion flow Mach numbers, the instability of coupled waves becomes saturated in the weakly nonlinear stage of its development. In the remaining cases, the instability development becomes explosive. The nonlinear dynamics is also considered of the aperiodic period-doubling instability, which always becomes saturated in the weakly nonlinear stage of its development.

Appendices

DERIVATIVES OF INTERACTION POTENTIAL

Since the interaction potential (1) is continuous at $x > 0$ and $z = 0$, the coefficients (2) can be calculated in the limit of $z \to + 0$. At $z > 0$, the potential can be reduced to the following form:

$$U(x,z) = \int\limits_0^\infty \,dk\frac{{kq(k)}}{{\beta (k)}}{{e}^{{ - q(k)z}}}{{J}_{0}}(kx),$$

(A.1)

where $\beta (k)$ = $\sqrt {{{{({{k}^{2}} + {{M}^{2}} - 1)}}^{2}} + 4{{k}^{2}}}$ and $q{{(k)}^{2}}$ = $({{k}^{2}} + {{M}^{2}} - 1 + \beta (k)){\text{/}}2$. The integral in expression (A.1) converges at $z = 0$, but its derivatives turn out to be divergent. To regularize these divergences, it is convenient to do the following. The derivatives of potential (A.1) have the following form:

$${{U}^{{(i,j)}}}(x,z) = \int\limits_0^\infty \,dk{{F}^{{(i,j)}}}(k){{e}^{{ - q(k)z}}}J_{0}^{{(i)}}(kx),$$

(A.2)

where $J_{0}^{{(i)}}(kx)$ is the derivative of the Bessel function of order i, and

$${{F}^{{(i,j)}}}(k) = {{( - 1)}^{j}}\frac{{{{k}^{{i + 1}}}q{{{(k)}}^{{j + 1}}}}}{{\beta (k)}}$$

is the algebraic function of the variable k. At $k \to \infty$, the expansion of the ${{F}^{{(i,j)}}}(k)$ function has the form of ${{F}^{{(i,j)}}}(k)$ = ${{P}^{{(i,j)}}}(k) + \Delta {{F}^{{(i,j)}}}(k)$, where ${{P}^{{(i,j)}}}(k)$ is the polynomial of finite degree in k, and $\Delta {{F}^{{(i,j)}}}(k) \to 0$ at $k \to \infty$. Now, expression (A.2) can be identically rewritten in the form of ${{U}^{{(i,j)}}}(x,z)$ = ${{V}^{{(i,j)}}}(x,z) +$$\Delta {{U}^{{(i,j)}}}(x,z)$, where

$$\begin{gathered} {{V}^{{(i,j)}}}(x,z) = \int\limits_0^\infty \,dk{{P}^{{(i,j)}}}(k){{e}^{{ - kz}}}J_{0}^{{(i)}}(kx), \\ \Delta {{U}^{{(i,j)}}}(x,z) \\ \, = \int\limits_0^\infty \,dk\left[ {{{F}^{{(i,j)}}}(k){{e}^{{ - q(k)z}}} - {{P}^{{(i,j)}}}(k){{e}^{{ - kz}}}} \right]J_{0}^{{(i)}}(kx). \\ \end{gathered} $$

(A.3)

The first integral can be calculated analytically, and then we set $z \to 0$, and the second integral converges at $z = 0$. Thus, coefficients (2) can be written as ${{u}_{{i,j}}} = {{v}_{{i,j}}} + \Delta {{u}_{{i,j}}}$, where the values $\Delta {{u}_{{i,j}}} = \Delta {{U}^{{(i,j)}}}(a,0)$ can be calculated using the standard numerical methods, and ${{v}_{{0,1}}} = {{v}_{{0,3}}} = 0$, ${{v}_{{i,0}}} = {{( - 1)}^{i}}i!{\text{/}}{{a}^{{i + 1}}}$, ${{v}_{{1,1}}} = - 1{\text{/}}a$, ${{v}_{{2,1}}} = 1{\text{/}}{{a}^{2}}$, ${{v}_{{1,3}}} = 1{\text{/}}a$,

$$\begin{gathered} {{v}_{{0,2}}} = \frac{{{{a}^{2}}\left( {{{M}^{2}} - 2} \right) - 2}}{{2{{a}^{3}}}}, \\ {{v}_{{0,4}}} = \frac{{{{a}^{4}}\left( {3{{M}^{4}} - 8{{M}^{2}} + 8} \right) + {{a}^{2}}\left( {8 - 12{{M}^{2}}} \right) + 72}}{{8{{a}^{5}}}}, \\ \end{gathered} $$

$${{v}_{{3,1}}} = - \frac{{2{{a}^{2}}{{M}^{2}} + {{a}^{2}} + 4}}{{2{{a}^{3}}}},$$

(A.4)

$$\begin{gathered} {{v}_{{1,2}}} = \frac{{6 - {{a}^{2}}\left( {{{M}^{2}} - 2} \right)}}{{2{{a}^{4}}}}, \\ {{v}_{{2,2}}} = \frac{{{{a}^{2}}\left( {{{M}^{2}} - 2} \right) - 12}}{{{{a}^{5}}}}. \\ \end{gathered} $$

EXPANSION OF FORCE

In the expansion of the force in the equation of motion (4), the quadratic terms have the following form:

$$\begin{gathered} F_{{x,n}}^{{(2)}} = - {{u}_{{2,1}}}\left[ {\delta {{x}_{n}}\delta {{z}_{n}} + \delta {{x}_{{n + 1}}}\delta {{z}_{{n + 1}}}} \right] \\ \, + \frac{1}{2}{{u}_{{3,0}}}\Delta {{x}_{n}}{{\Delta }^{2}}{{x}_{n}} + \frac{1}{2}{{u}_{{1,2}}}\Delta {{z}_{n}}{{\Delta }^{2}}{{z}_{n}}, \\ F_{{z,n}}^{{(2)}} = {{u}_{{1,2}}}\left[ {\delta {{x}_{{n + 1}}}\delta {{z}_{{n + 1}}} - \delta {{x}_{n}}\delta {{z}_{n}}} \right] \\ \, - \;\frac{1}{2}{{u}_{{2,1}}}\left[ {{{{(\delta {{x}_{n}})}}^{2}} + {{{(\delta {{x}_{{n + 1}}})}}^{2}}} \right] \\ - \;\frac{1}{2}{{u}_{{0,3}}}\left[ {{{{(\delta {{z}_{n}})}}^{2}} + {{{(\delta {{z}_{{n + 1}}})}}^{2}}} \right], \\ \end{gathered} $$

(B.1)

where we used notations (5) and $\delta {{f}_{n}} = {{f}_{n}} - {{f}_{{n - 1}}}$. The cubic terms are as follows:

$$\begin{gathered} F_{{x,n}}^{{(3)}} = - \frac{1}{6}{{u}_{{1,3}}}\left[ {{{{(\delta {{z}_{n}})}}^{3}} + {{{(\delta {{z}_{{n + 1}}})}}^{3}}} \right] \\ \, + \frac{1}{2}{{u}_{{2,2}}}\left[ {\delta {{x}_{{n + 1}}}{{{(\delta {{z}_{{n + 1}}})}}^{2}} - \delta {{x}_{n}}{{{(\delta {{z}_{n}})}}^{2}}} \right] \\ \, - \frac{1}{2}{{u}_{{3,1}}}\left[ {\delta {{z}_{n}}{{{(\delta {{x}_{n}})}}^{2}} + \delta {{z}_{{n + 1}}}{{{(\delta {{x}_{{n + 1}}})}}^{2}}} \right] \\ \end{gathered} $$

$$\begin{gathered} \, + \frac{1}{6}{{u}_{{4,0}}}{{\Delta }^{2}}{{x}_{n}}\left[ {{{{(\delta {{x}_{n}})}}^{2}} + \delta {{x}_{n}}\delta {{x}_{{n + 1}}} + {{{(\delta {{x}_{{n + 1}}})}}^{2}}} \right], \\ F_{{z,n}}^{{(3)}} = - \frac{1}{6}{{u}_{{3,1}}}\left[ {{{{(\delta {{x}_{n}})}}^{3}} + {{{(\delta {{x}_{{n + 1}}})}}^{3}}} \right] \\ \end{gathered} $$

(B.2)

$$\begin{gathered} \, - \frac{1}{2}{{u}_{{1,3}}}\left[ {\delta {{x}_{n}}{{{(\delta {{z}_{n}})}}^{2}} + \delta {{x}_{{n + 1}}}{{{(\delta {{z}_{{n + 1}}})}}^{2}}} \right] \\ \, + \frac{1}{6}{{u}_{{0,4}}}{{\Delta }^{2}}{{z}_{n}}\left[ {{{{(\delta {{z}_{n}})}}^{2}} + \delta {{z}_{n}}\delta {{z}_{{n + 1}}} + {{{(\delta {{z}_{{n + 1}}})}}^{2}}} \right] \\ \, + \frac{1}{2}{{u}_{{2,2}}}\left[ {{{{(\delta {{x}_{{n + 1}}})}}^{2}}\delta {{z}_{{n + 1}}} - {{{(\delta {{x}_{n}})}}^{2}}\delta {{z}_{n}}} \right]. \\ \end{gathered} $$

NONLINEARITY COEFFICIENTS

The coefficients in Eq. (10) are as follows:

$$\begin{gathered} {{\gamma }_{1}} = \frac{{\Omega _{{cr}}^{2}\left( {\cos\left( {{{k}_{0}}} \right) + 1} \right)}}{{8\omega _{0}^{2}}}, \\ {{\gamma }_{3}} = - \frac{{3\Omega _{{cr}}^{2}}}{{32\omega _{0}^{2}}}{{\sin}^{2}}({{k}_{0}})\left[ {\left( {{{u}_{{4,0}}} - {{u}_{{0,4}}}} \right){{\sin}^{2}}({{k}_{0}}{\text{/}}2)} \right. \\ \end{gathered} $$

$$\, + \left. {({{u}_{{1,3}}} + {{u}_{{3,1}}})\sin\left( {{{k}_{0}}} \right)} \right],$$

(C.1)

$$\begin{gathered} {{\beta }_{x}} = \frac{{i\left( {{{u}_{{1,2}}} + {{u}_{{3,0}}}} \right)\Omega _{{{\text{cr}}}}^{2}{{\sin}^{3}}\left( {{{k}_{0}}} \right)}}{{2\omega _{0}^{2}}}, \\ {{\beta }_{z}} = \frac{{\left( {{{u}_{{0,3}}} + {{u}_{{2,1}}}} \right)\Omega _{{{\text{cr}}}}^{2}{{\sin}^{4}}\left( {{{k}_{0}}} \right)}}{{2\omega _{0}^{2}\left( {\cos\left( {{{k}_{0}}} \right) - 1} \right)}}. \\ \end{gathered} $$

The coefficients $\alpha$ associated with the perturbations corresponding to the second spatial harmonic can be obtained from the solutions of the following sets of linear equations:

$$\begin{gathered} {\mathbf{M}} \cdot \left( {\begin{array}{*{20}{c}} {{{\alpha }_{{x,0}}}} \\ {{{\alpha }_{{z,0}}}} \end{array}} \right) = {\mathbf{T}}, \\ ({\mathbf{M}} - 4\omega _{0}^{2}{\mathbf{I}}) \cdot \left( {\begin{array}{*{20}{c}} {{{\alpha }_{{x,2}}}} \\ {{{\alpha }_{{z,2}}}} \end{array}} \right) = \frac{1}{2}{\mathbf{T}}, \\ \end{gathered} $$

(C.2)

where ${\mathbf{I}}$ is the unity matrix,

$${\mathbf{M}} = \left( {\begin{array}{*{20}{c}} {4si{{n}^{2}}\left( {{{k}_{0}}} \right){{u}_{{2,0}}},}&{2i\sin\left( {2{{k}_{0}}} \right){{u}_{{1,1}}}} \\ {2i\sin\left( {2{{k}_{0}}} \right){{u}_{{1,1}}},}&{4{{u}_{{0,2}}}si{{n}^{2}}\left( {{{k}_{0}}} \right) + \Omega _{{{\text{cr}}}}^{2}} \end{array}} \right)$$

and

$$\begin{gathered} {\mathbf{T}} = \frac{1}{2}si{{n}^{2}}({{k}_{0}}{\text{/}}2) \\ \, \times \left( {\begin{array}{*{20}{c}} {i\left( {\left( {{{u}_{{1,2}}} - {{u}_{{3,0}}}} \right)\sin\left( {{{k}_{0}}} \right) - 2\sigma {{u}_{{2,1}}}\cos({{k}_{0}})} \right)} \\ { - 2\sigma {{u}_{{1,2}}}\sin\left( {{{k}_{0}}} \right) - \left( {{{u}_{{0,3}}} - {{u}_{{2,1}}}} \right)\cos({{k}_{0}})} \end{array}} \right). \\ \end{gathered} $$