An Approach to Finding the Asymptotics of Polynomials Given by Recurrence Relations

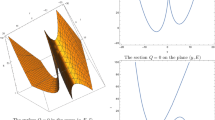
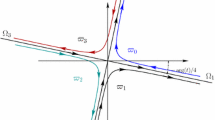
Abstract

Many orthogonal polynomials $u(n,z)$ ($n$ is the number of the polynomial, $z$ is its argument), for example, the Chebyshev, Hermite, Laguerre, Legendre, and other polynomials, are determined by recurrence relations (or finite-difference equations) of the second order. For large numbers $n$, they are approximated by exponential, trigonometric, or special functions of a compound argument. For example, Hermite polynomials are approximated by the Plancherel–Rotach formulas, in which the special function is ${\rm Ai}$, the Airy function, the Legendre polynomials are approximated by the zero-order Bessel function, etc. In the paper, an approach is developed for finding asymptotics of this type, which are uniform in this case (and unified) with respect to the variable $z$. The approach is based on the passage from discrete equations to continuous pseudodifferential equations and the subsequent application of the semiclassical approximation to these equations with complex phases. This is a further development of the considerations proposed in the papers of A.I. Aptekarev, S.Yu. Dobrokhotov, D.N. Tulyakov, and A.V. Tsvetkova devoted to asymptotics of the Plancherel–Rotach type for Hermite polynomials and a subclass of Hermite type orthogonal polynomials with multiple indices.