Skip to main content
SpringerLink
Log in

Semi-Implicit and Semidiscrete Difference Schemes for Solving a Nonstationary Kinetic Equation of Thermal Radiative Transfer and Energy Equation

  • MATHEMATICAL PHYSICS
  • Published:
Computational Mathematics and Mathematical Physics Aims and scope Submit manuscript

An Erratum to this article was published on 01 May 2022

This article has been updated

Abstract

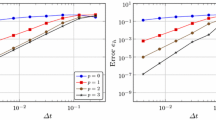
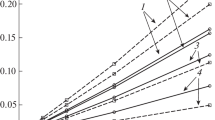
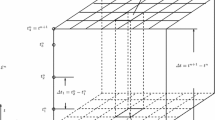
Semi-implicit and semidiscrete difference schemes of higher order accuracy are proposed for solving kinetic equations of thermal radiative transfer and the energy equation by applying a modified splitting method. A feature of the schemes is that thermal radiative transfer is computed using explicit or implicit difference schemes approximating a usual transport equation. The radiation–matter interaction is computed using implicit difference schemes in the semi-implicit case and using analytical solutions of ordinary differential equations in the semidiscrete case. The difference schemes of higher order accuracy are constructed relying on the second-order Runge–Kutta method. Solutions are found without using outer iterations with respect to the collision integral or matrix inversion. The solution algorithms for difference equations are well suited for parallelization. The method can naturally be generalized to multidimensional problems.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1.
Fig. 2.
Fig. 3.

Similar content being viewed by others

Change history

REFERENCES

  1. B. G. Carlson and G. I. Bell, “Solution of the transport equation by the Sn method,” in Proceeding of the Second Unified Nations International Conference on the Peaceful Uses of Atomic Energy, P/2386 (Unified Nations, 1958), Vol. 16, pp. 535–549.

  2. Shih-I Pai, Radiation Gas Dynamics (Springer-Verlag, Wien, 1966).

    Book  Google Scholar 

  3. B. N. Chetverushkin, Mathematical Simulation of Radiating Gas Dynamics (Nauka, Moscow, 1985) [in Russian].

    Google Scholar 

  4. L. P. Bass, A. M. Voloshchenko, and T. A. Germogenova, Discrete Ordinate Methods for Radiative Transfer Problems (Inst. Prikl. Mat. im. M.V. Keldysha Ross. Akad. Nauk, Moscow, 1986) [in Russian].

  5. P. C. R. Feautrier, “Sur la résolution numérique de l'équation de transfert,” Acad. Sci. Paris 258, 3189–3191 (1964).

    Google Scholar 

  6. G. Rybicki, “A modified Feautrier method,” J. Quant. Spectrosc. Radiat. Transfer 11, 589–596 (1971).

    Article  Google Scholar 

  7. V. S. Vladimirov, “Numerical solution of the equation for the sphere,” in Numerical Mathematics (Vychisl. Tsentr Akad. Nauk SSSR, Moscow, 1958), pp. 3–33 [in Russian].

    Google Scholar 

  8. A. V. Nikiforova, V. A. Tarasov, and V. E. Troshchiev, “Solution of the kinetic equations by the divergent method of characteristics,” USSR Comput. Math. Math. Phys. 12 (4), 251–260 (1972).

    Article  Google Scholar 

  9. V. Ya. Gol’din, “A quasi-diffusion method of solving the kinetic equation,” USSR Comput. Math. Math. Phys. 4 (6), 136–149 (1964).

    Article  MathSciNet  Google Scholar 

  10. N. G. Karlykhanov, “Application of the quasi-diffusion method for solving radiative transfer problems,” Vopr. At. Nauki Tekh. Ser. Mat. Model. Fiz. Protsessov 1, 32–38 (2010).

    Google Scholar 

  11. V. Yu. Gusev, M. Yu. Kozmanov, and E. B. Rachilov, “A method of solving implicit difference equations approximating systems of radiation transport and diffusion equations,” USSR Comput. Math. Math. Phys. 24 (12), 156–161 (1984).

    Article  Google Scholar 

  12. G. V. Dolgoleva, “Numerical solution of a system of equations describing radiative transfer and the radiation–matter interaction,” Vopr. At. Nauki Tekh., Ser. Mat. Model. Fiz. Processov, No. 1, 58–60 (1991).

  13. L. P. Fedotova and R. M. Shagaliev, “Difference KM-schemes for two-dimensional nonstationary transfer process in the multigroup kinetic approximation,” Mat. Mod. 3 (6), 29–41 (1991).

    Google Scholar 

  14. D. Yu. Anistratov, E. N. Aristova, and V. Ya. Gol’din, “A nonlinear method for solving problems of radiation transfer in medium,” Mat. Mod. 8 (12), 3–28 (1996).

    MathSciNet  MATH  Google Scholar 

  15. N. G. Karlykhanov, “Construction of optimal multidiagonal methods for solving radiation-transfer problems,” Comput. Math. Math. Phys. 37 (4), 482–486 (1997).

    MathSciNet  MATH  Google Scholar 

  16. G. I. Marchuk and N. N. Yanenko, “Solution of a multidimensional kinetic equation by the splitting method,” Dokl. Akad. Nauk SSSR 157 (6), 1291–1292 (1964).

    MathSciNet  MATH  Google Scholar 

  17. N. N. Yanenko, The Method of Fractional Steps: The Solution of Problems of Mathematical Physics in Several Variables (Nauka, Novosibirsk, 1967; Springer-Verlag, Berlin, 1971).

  18. G. I. Marchuk, Splitting Methods (Nauka, Moscow, 1988) [in Russian].

    Google Scholar 

  19. A. A. Samarskii and P. N. Vabishchevich, Additive Schemes for Problems of Mathematical Physics (Nauka, Moscow, 2001) [in Russian].

    MATH  Google Scholar 

  20. N. Ya. Moiseev, “Modified method of splitting with respect to physical processes for solving radiation gas dynamics equations,” Comput. Math. Math. Phys. 57 (2), 306–317 (2017).

    Article  MathSciNet  Google Scholar 

  21. D. Mihalas, Stellar Atmospheres (Freeman, San Francisco, 1978).

    Google Scholar 

  22. R. E. Alcouff, “A stable diffusion synthetic acceleration method for neutron transport iterations,” Trans. Am. Nucl. Soc. 23, 203 (1976).

    Google Scholar 

  23. R. E. Alcouff, D. R. McCoy, and E. W. Larsen, “Finite difference effects in the synthetic acceleration method,” Trans. Am. Nucl. Soc. 39, 462 (1981).

    Google Scholar 

  24. A. D. Gadzhiev, V. N. Seleznev, and A. A. Shestakov, “DSn-method with artificial dissipation and DMT method of iteration acceleration for the numerical solution of two-dimensional heat transfer equations in kinetic approximation,” Vopr. At. Nauki Tekh., Ser. Mat. Model. Fiz. Processov, No. 4, 33–46 (2003).

  25. V. V. Zav’yalov and A. A. Shestakov, “Simplified solutions of the Fleck problems,” Mat. Model. 22 (2), 93–1042 (2010).

    Google Scholar 

  26. A. Klar, “An asymptotic-induced scheme for nonstationary transport equations in the diffusive limit,” SIAM J. Numer. Anal. 35 (3), 1073–1078 (1998).

    Article  MathSciNet  Google Scholar 

  27. A. Klar and A. Unterreiter, “Uniform stability of a finite difference scheme for transport equations in diffusive regimes,” SIAM J. Numer. Anal. 40 (3), 891–913 (2002).

    Article  MathSciNet  Google Scholar 

  28. R. G. McClarren, T. M. Evans, R. B. Lowrie, and J. D. Densmore, “Semi-implicit time integration for P N thermal radiative transfer,” J. Comput. Phys. 227 (16), 7561–7586 (2008).

    Article  MathSciNet  Google Scholar 

  29. N. Ya. Moiseev and I. Yu. Silant’eva, “Arbitrary-order difference schemes for solving linear advection equations with constant coefficients by the Godunov method with antidiffusion,” Comput. Math. Math. Phys. 48 (7), 1210–1220 (2008).

    Article  MathSciNet  Google Scholar 

  30. N. Ya. Moiseev, “High-order accurate implicit running schemes,” Comput. Math. Math. Phys. 51 (5), 862–875 (2011).

    Article  MathSciNet  Google Scholar 

  31. S. K. Godunov, A. V. Zabrodin, G. P. Prokopov, and M. Ya. Ivanov, Numerical Solution of Multidimensional Problems in Gas Dynamics (Nauka, Moscow, 1976) [in Russian].

    Google Scholar 

  32. Yu. I. Shokin, Method of Differential Approximation (Nauka, Novosibirsk, 1979) [in Russian].

    MATH  Google Scholar 

  33. D. Kahaner, C. Moler, and S. Nash, Numerical Methods and Software (Prentice Hall, Englewood Cliffs, NJ, 1989).

    MATH  Google Scholar 

  34. R. P. Fedorenko, “The application of difference schemes of high accuracy to the numerical solution of hyperbolic equations,” USSR Comput. Math. Math. Phys. 2 (6), 1355–1365 (1962).

    Article  Google Scholar 

  35. J. F. Fleck and J. D. Cummings, Jr., “An implicit Monte Carlo scheme for calculating time and frequency dependent radiation transport,” J. Comput. Phys. 8 (3), 313–342 (1971).

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Russian Federal Nuclear Center—Zababakhin All-Russia Research Institute of Technical Physics, 456770, Snezhinsk, Chelyabinsk oblast, Russia

    N. Ya. Moiseev & V. M. Shmakov

Authors
  1. N. Ya. Moiseev
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. V. M. Shmakov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to N. Ya. Moiseev or V. M. Shmakov.

Ethics declarations

The authors declare that they have no conflicts of interest.

Additional information

Translated by I. Ruzanova

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Moiseev, N.Y., Shmakov, V.M. Semi-Implicit and Semidiscrete Difference Schemes for Solving a Nonstationary Kinetic Equation of Thermal Radiative Transfer and Energy Equation. Comput. Math. and Math. Phys. 62, 476–486 (2022). https://doi.org/10.1134/S0965542522030113

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S0965542522030113

Keywords:

Search

Navigation