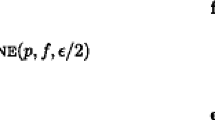Skip to main content
SpringerLink
Log in

Lattice flows in networks

  • Communication Network Theory
  • Published:
Problems of Information Transmission Aims and scope Submit manuscript

Abstract

We consider flows in networks analogous to numerical flows but such that values of arc capacities are elements of a lattice. We present an analog of the max-flow min-cut theorem. However, finding the value of the maximum flow for lattice flows is based on not this theorem but computations in the algebra of matrices over the lattice; in particular, the maximum flow value is found with the help of transitive closure of flow capacity functions. We show that there exists a correspondence between flows and solutions of special-form systems of linear equations over distributive lattices.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Ford, L. and Fulkerson, D., Flows in Networks, Princeton: Princeton Univ. Press, 1962. Translated under the title Potoki v setyakh, Moscow: Mir, 1966.

    MATH  Google Scholar 

  2. Ahuja, R.K., Magnanti, T.L., and Orlin, J.B., Network Flows: Theory, Algorithms, and Applications, Englewoods Cliffs, NJ: Prentice Hall, 1993.

    MATH  Google Scholar 

  3. McCormick, S.T., Submodular Function Minimization, Discrete Optimization, Aardal, K., Nemhauser, G., and Weismantel, R., Eds., Handbooks Oper. Res. Management Sci., vol. 12, Amsterdam: North- Holland, 2005, pp. 321–391.

  4. Granot, F., McCormick, S.T., Queyranne, M.N., and Tardella, F., Structural and Algorithmic Properties for Parametric Minimum Cuts, Math. Program. Ser. A, 2012, vol. 135, no. 1–2, pp. 337–367.

    Article  MathSciNet  MATH  Google Scholar 

  5. Topkis, D.M., Minimizing a Submodular Function on a Lattice, Oper. Res., 1978, vol. 26, no. 2, pp. 305–321.

    Article  MathSciNet  MATH  Google Scholar 

  6. Aigner, M., Combinatorial Theory, New York: Springer, 1979. Translated under the title Kombinatornaya teoriya, Moscow: Mir, 1982.

    MATH  Google Scholar 

  7. Seymour, P.D., The Matroids with the Max-Flow Min-Cut Property, J. Combin. Theory Ser. B, 1977, vol. 23, no. 2–3, pp. 188–222.

    MathSciNet  MATH  Google Scholar 

  8. Leighton, T. and Rao, S., Multicommodity Max-Flow Min-Cut Theoremes and Their Use in Designing Approximation Algorithms, J. ACM, 1999, vol. 46, no. 6, pp. 787–832.

    Article  MathSciNet  MATH  Google Scholar 

  9. Seymour, P.D., Matroids and Multicommodity Flows, European J. Combin., 1981, vol. 2, no. 3, pp. 257–290.

    Article  MathSciNet  MATH  Google Scholar 

  10. Khuller, S., Naor, J., and Klein, P., The Lattice Structure of Flow in Planar Graphs, SIAM J. Discrete Math., 1993, vol. 6, no. 3, pp. 477–490.

    Article  MathSciNet  MATH  Google Scholar 

  11. Ghrist, R. and Krishnan, S., A Topological Max-Flow Min-Cut Theorem, in Proc. 2013 Global Conf. on Signal and Information Processing (GlobalSIP’2013), Austin, TX, USA, Dec. 3–5, 2013, pp. 815–818.

    Chapter  Google Scholar 

  12. Christofides, N., Graph Theory: An Algorithmic Approach, New York: Academic, 1975. Translated under the title Teoriya grafov. Algoritmicheskii podkhod, Moscow: Mir, 1978.

    MATH  Google Scholar 

  13. Grätzer, G.A., General Lattice Theory, New York: Academic, 1978. Translated under the title Obshchaya teoriya reshetok, Moscow: Mir, 1982.

  14. Shmatkov, V.D., Incidence Algebras over Lattices, Uspekhi Mat. Nauk, 1992, vol. 47, no. 4 (286), pp. 217–218 [Russian Math. Surveys (Engl. Transl.), 1992, vol. 47, no. 4, pp. 230–231].

    MathSciNet  MATH  Google Scholar 

  15. Birkhoff, G., Lattice Theory, Providence: Amer. Math. Soc., 1967, 3rd ed. Translated under the title Teoriya reshetok, Moscow: Nauka, 1984.

    MATH  Google Scholar 

  16. Cechlárová, K., Powers of Matrices over Distributive Lattices—A Review, Fuzzy Sets and Systems, 2003, vol. 138, no. 3, pp. 627–641.

    Article  MathSciNet  MATH  Google Scholar 

  17. Warshall, S., A Theorem on Boolean Matrices, J. ACM, 1962, vol. 9, no. 1, pp. 11–12.

    Article  MathSciNet  MATH  Google Scholar 

  18. Kruglov, V.V., Dli, M.I., and Golunov, R.Yu., Nechetkaya logika i iskusstvennye neironnye seti (Fuzzy Logic and Artificial Neural Networks), Moscow: Fizmatlit, 2001.

    Google Scholar 

  19. Gavalec, M. and Zimmermann, K., Solving Systems of Two-Sided (max, min)-Linear Equations, Kybernetika, 2010, vol. 46, no. 3, pp. 405–414.

    MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Ryazan State Radio Engineering University, Ryazan, Russia

    V. D. Shmatkov

Authors
  1. V. D. Shmatkov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to V. D. Shmatkov.

Additional information

Original Russian Text © V.D. Shmatkov, 2016, published in Problemy Peredachi Informatsii, 2016, Vol. 52, No. 1, pp. 27–42.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Shmatkov, V.D. Lattice flows in networks. Probl Inf Transm 52, 24–38 (2016). https://doi.org/10.1134/S003294601601004X

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S003294601601004X

Keywords

Search

Navigation