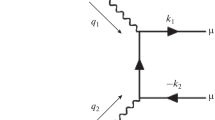
Dilepton production in proton–proton collision through γγ-fusion with one proton scattered elastically while the second produces a hadron jet is considered. Semi-analytical formulas describing the cross section for a muon pair production are presented.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
Recent measurement of the muon magnetic moment at Fermilab has confirmed the deviation from the Standard Model prediction [1]. When averaged with the previous BNL result [2], it leads to a discrepancy of more than four standard deviations [1]. If it is a manifestation of New Physics, one should expect that at higher energies the deviations in the interactions of muons from the Standard Model predictions should be larger. Since ultraperipheral collisions are a source of very clean events, they can help to constrain parameters of new particles that can be responsible for this difference, e.g., see [3, 4].
At the Large Hadron Collider (LHC), muon pairs are produced with high invariant masses, and this gives a chance for New Physics to be detected. It follows that the theoretical description of these reactions in the Standard Model is highly desirable. Recently, the ATLAS collaboration has measured the fiducial cross section for the process when a muon pair is accompanied by the detection of one of the colliding protons in the forward detector [5]. Here, we provide expressions for the cross section for this reaction. Using the derived formulas, the cross section values can be evaluated by the standard numerical integration routines (e.g., provided by the GSL [6]) rather than Monte Carlo simulations. Let us note that numerical results for this reaction were recently presented in [7] (see also [8]).
The master formula describing the reaction under consideration can be easily obtained from the expressions provided in the review of two-photon particle production [9]:
where α is the fine structure constant, \({{Q}_{q}}\) is the electric charge of the quark q, \(\rho _{{\mu \nu }}^{{(1)}}\) and \(\rho _{{\alpha \beta }}^{{(2)}}\) are the density matrices of the photons, \({{M}_{{\mu \alpha }}}\) is the amplitude of the process, \({{p}_{1}}\), \(p_{1}^{'}\), \({{p}_{2}}\), \(p_{2}^{'}\) are the proton and quark momenta before and after the collision (see Fig. 1), \({{k}_{1}}\) and \({{k}_{2}}\) are the momenta of muons, \({{q}_{1}}\) and \({{q}_{2}}\) are the momenta of photons, \(E_{1}^{'}\) and \(E_{2}^{'}\) are the proton and quark energies in the final state, \(d\Gamma \) is the phase volume of the muon pair, and \({{m}_{p}}\) is the proton mass. For the density matrices, we get
and a similar expression for \(\rho _{{\alpha \beta }}^{{(2)}}\).
In order for the proton to remain intact, the square of the momentum transfer \( - q_{1}^{2}\) should be bounded from above: \( - q_{1}^{2} \lesssim {{\hat {q}}^{2}}\), where \(\hat {q} \sim {{\Lambda }_{{QCD}}}\). Following [10], we take \(\hat {q} = 200\) MeV in our calculations below. In this case, if the energy carried by the photon is in the interval \(0.015{{E}_{p}} < {{\omega }_{1}} < 0.15{{E}_{p}}\) then the proton will be detected by the forward detector with near 100% efficiency [11, 12]. As for the quark, its value of the transferred momentum \( - q_{2}^{2}\) is approximately bounded by the invariant mass of the muon pair W, because the cross section for the reaction \(\gamma \gamma \text{*} \to {{\mu }^{ + }}{{\mu }^{ - }}\) at \( - q_{2}^{2} > {{W}^{2}}\) decreases rapidly as \({{W}^{2}}{\text{/}}( - q_{2}^{2})\). Thus the photon with the momentum \({{q}_{1}}\) is emitted quasi-elastically and is polarized transversally, while the photon with the momentum \({{q}_{2}}\) can also be longitudinally polarized.Footnote 1
The most appropriate way to deal with the density matrices \(\rho _{{\mu \nu }}^{{(1)}}\) and \(\rho _{{\alpha \beta }}^{{(2)}}\) is to introduce the basis of virtual photons helicity states. Let us suppose that in the center-of-mass system (c.m.s.) of the colliding photons we have \({{q}_{1}} = ({{\widetilde \omega }_{1}},0,0,{{\widetilde q}_{1}})\) and \({{q}_{2}} = ({{\widetilde \omega }_{2}},0,0, - {{\widetilde q}_{1}})\). The standard set of orthonormal four-vectors orthogonal to the momenta \({{q}_{1}}\), \({{q}_{2}}\) is
These four-vectors correspond to the ±1 and 0 helicity states of virtual photons in their c.m.s. They form a complete orthonormal basis for subspaces orthogonal to \({{q}_{{1\mu }}}\) and \({{q}_{{2\mu }}}\), respectively. Taking into account, that, due to the conservation of the vector currents, \(\rho _{{\mu \nu }}^{{(1)}}{{q}_{{1\mu }}} = \rho _{{\mu \nu }}^{{(2)}}{{q}_{{2\mu }}}\) = 0, we obtain
Here, \(\rho _{{ab}}^{{(i)}}\) are the density matrices in the helicity representation, and, according to [14], in the c.m.s. system of colliding protons in the case \(E \gg {{\omega }_{1}}\), \(xE \gg {{\omega }_{2}}\) we haveFootnote 2
where \(E \equiv \sqrt s {\text{/}}2\) = 6.5 TeV is the colliding proton energy while \(xE\) is the quark energy, \(0 < x < 1\).
Finally, we obtain
where \(({{p}_{1}}{{p}_{2}}) = 2{{E}^{2}}x\), \(({{q}_{1}}{{q}_{2}}) = ({{W}^{2}} - q_{2}^{2}){\text{/}}2\),
\({{M}_{{ \pm \pm }}}\) and \({{M}_{{ \pm 0}}}\) are the amplitudes of the process \(\gamma \gamma \text{*} \to {{\mu }^{ + }}{{\mu }^{ - }}\) with the corresponding photons polarizations. According to Eq. (E.3) from [9],
where m is the muon mass. Thus, \({{\sigma }_{{\gamma \gamma \text{*} \to {{\mu }^{ + }}{{\mu }^{ - }}}}} = \) \({{\sigma }_{{TT}}} + {{\sigma }_{{TS}}}\) should be substituted into Eq. (6).
Integration over \(q_{{1 \bot }}^{2}\) in Eq. (6) is easily performed: \(\int dq_{{1 \bot }}^{2}{\text{/}}q_{1}^{2} = 2\ln \hat {q}\gamma {\text{/}}{{\omega }_{1}}\), where \(\gamma = E{\text{/}}{{m}_{p}}\) is the Lorentz factor of the proton. In the c.m.s. of the protons, the following equations hold:
where \({{E}_{q}}\) and \({{p}_{q}}\) are the quark energy and spatial momentum: \({{p}_{2}} = ({{E}_{q}},{{p}_{q}})\).
It is convenient to change the integration variables from the photon energies \({{\omega }_{1}}\) and \({{\omega }_{2}}\) to the square of the invariant mass of the produced pair \({{W}^{2}}\) and the ratio of photon energies \(y = {{\omega }_{1}}{\text{/}}{{\omega }_{2}}\): \(d{{\omega }_{1}}d{{\omega }_{2}}dq_{{2 \bot }}^{2}\) = \((1{\text{/}}8y)d{{W}^{2}}dydQ_{2}^{2}\), where \(Q_{2}^{2} = - q_{2}^{2}\). The following upper bounds on photon energies should be taken into account: \({{\omega }_{1}} \leqslant \hat {q}\gamma \), \({{\omega }_{2}} \leqslant xE\). Thus, we obtain
where \({{\omega }_{1}} = \sqrt {y({{W}^{2}} + Q_{2}^{2})} {\text{/}}2\), \({{\omega }_{2}} = \sqrt {{{W}^{2}} + Q_{2}^{2}} {\text{/}}(2\sqrt y )\) and \({{f}_{q}}(x,Q_{2}^{2})\) is the q-quark density function. Here, the sum is taken over all quarks and antiquarks, both valent (u, d) and sea. The factor of 2 takes into account the symmetrical process, when the proton from the second vertex remains intact. The differential cross section \(d{{\sigma }_{{pp \to p{{\mu }^{ + }}{{\mu }^{ - }}X}}}{\text{/}}dW\) for \(W > 12\) GeV is shown in Fig. 2. Quark density functions were taken from the set MMHT2014nnlo68cl [15] of the LHAPDF library [16]. The integrated cross section in this region is
It is instructive to compare this result with the cross section for quasielastic \({{\mu }^{ + }}{{\mu }^{ - }}\) pair production [10]:
Let us mention [17, 18] in which the case of lepton pair production in photon fusion with both protons scattered inelastically is studied.
CONCLUSIONS
The cross section for \({{\mu }^{ + }}{{\mu }^{ - }}\) pair production in semi-inclusive pp-scattering at the LHC is calculated (see Eqs. (9) and (10)). The spectrum of the produced pairs is presented in Fig. 2. Taking into account the dependence of differential cross section on photon virtuality explicitly, we have achieved better accuracy in comparison to the equivalent photon approximation.
Change history
27 November 2022
An Erratum to this paper has been published: https://doi.org/10.1134/S0021364022340021
Notes
In order to suppress the background, only the events with the invariant mass of the muon pair W above a few GeV (e.g., 12 GeV [13]) are selected. Therefore, neglecting corrections of the order of \({{\hat {q}}^{2}}{\text{/}}{{\hat {W}}^{2}}\) ~ 3 × 10–4, where \(\hat {W} = 12\) GeV is a lower bound on the invariant mass of the muon pair W, we can consider the photon with the momentum \({{q}_{1}}\) as real and polarized transversally.
In what follows, we consider the high energy limit and neglect the masses of colliding particles.
REFERENCES
B. Abi, T. Albahri, S. Al-Kilani, et al. (Muon g-2 Collab.), Phys. Rev. Lett. 126, 141801 (2021); arXiv: 2104.03281 [hep-ex].
G. Bennett, B. Bousquet, H. Brown, et al. (Muon g-2 Collab.), Phys. Rev. D 73, 072003 (2006); arXiv: hep-ex/0602035 [hep-ex].
S. I. Godunov, V. A. Novikov, M. I. Vysotsky, and E. V. Zhemchugov, JETP Lett. 109, 358 (2019); arXiv: 1808.02431 [hep-ph].
S. I. Godunov, E. K. Karkaryan, V. A. Novikov, A. N. Rozanov, M. I. Vysotsky, and E. V. Zhemchugov, Phys. Rev. D 103, 035016 (2021); arXiv: 2012.01599[hep-ph].
G. Aad, B. Abbott, D. Abbott, et al. (ATLAS Collab.), Phys. Rev. Lett. 125, 261801 (2020); arXiv: 2009.14537 [hep-ex].
M. Galassi, J. Davies, J. Theiler, et al., GNU Scientific Library Reference Manual, 3rd ed. (Network Theory, 2009). http://www.gnu.org/software/gsl.
A. Szczurek, B. Linek, and M. Luszczak, Phys. Rev. D 104, 074009 (2021); arXiv: 2107.02535 [hep-ph].
A. Szczurek, B. Linek, and M. Luszczak, in Proceedings of the European Physical Society Conference on High Energy Physics, 2021, arXiv: 2111.06627 [hep-ph].
V. M. Budnev, I. F. Ginzburg, G. V. Meledin, and V. G. Serbo, Phys. Rep. 15, 181 (1975).
M. I. Vysotsky and E. V. Zhemchugov, Phys. Usp. 62, 910 (2019); arXiv: 1806.07238 [hep-ph].
L. Adamczyk, E. Banaś, A. Brandt, et al. (ATLAS Collab.), Technical Design Report CERN-LHCC-2015-009, ATLAS-TDR-024-2015.
M. Albrow, M. Arneodo, V. Avati, et al. (CMS and TOTEM Collabs.), Technical Design Report CE-RN-LHCC-2014-021, TOTEM-TDR-003.
M. Aaboud, G. Aad, B. Abbott, et al. (ATLAS Collab.), Phys. Lett. B 777, 303 (2018); arXiv: 1708.04053 [hep-ex].
A. Dobrovolskaya and V. Novikov, Z. Phys. C 52, 427 (1991).
L. A. Harland-Lang, A. D. Martin, P. Motylinski, and R. S. Thorne, Eur. Phys. J. C 75, 204 (2015); arXiv: 1412.3989 [hep-ph].
A. Buckley, J. Ferrando, S. Lloyd, K. Nordström, B. Page, M. Rüfenacht, M. Schönherr, and G. Watt, Eur. Phys. J. C 75, 132 (2015); arXiv: 1412.7420 [hep-ph].
L. A. Harland-Lang, M. Tasevsky, V. A. Khoze, and M. G. Ryskin, Eur. Phys. J. C 80, 925 (2020); arXiv: 2007.12704 [hep-ph].
L. A. Harland-Lang, Phys. Rev. D 104, 073002 (2021); arXiv: 2101.04127 [hep-ph].
Funding
This work was supported by the Russian Science Foundation, project no. 19-12-00123.
Author information
Authors and Affiliations
Corresponding authors
Ethics declarations
The authors declare that they have no conflicts of interest.
Additional information
The original online version of this article was revised: Due to a retrospective Open Access order.
Rights and permissions
Open Access. This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Godunov, S.I., Karkaryan, E.K., Novikov, V.A. et al. Forward Proton Scattering in Association with Muon Pair Production via the Photon Fusion Mechanism at the LHC. Jetp Lett. 115, 59–62 (2022). https://doi.org/10.1134/S0021364022020011
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1134/S0021364022020011