Introduction

The square lattice and the equilateral triangular lattice, thus also the honeycomb lattice, are high-symmetry two-dimensional (2D) lattices. They play important roles in mathematics, physics, architectonics, arts and many other fields. For 2D lattices, the uniform scaling of the space, i.e., simultaneous dilation or contraction at two orthogonal directions with the same scale factors, will evidently preserve the character, or symmetry, of their patterns. A natural and perhaps also meaningful question may be raised: Is there any directional scaling symmetry for the high-symmetry 2D lattices that preserves the character of these lattices? Or in other words, is there any scaling transformation along a particular direction that brings a square (equilateral triangular) lattice into a square (equilateral triangular) lattice?

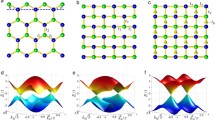
For the equilateral triangular lattice, if directional scaling is performed along any side of the unit triangle, i.e., along the <10>-directions, in the crystallographic nomenclature, then the contraction at any rate will never result in a triangular lattice. However, stretching along that direction with a scale factor γ = 3 results in a perfect equilateral triangular lattice and the side length of the unit triangle in the resulting lattice is times larger (Fig. 1). A particular feature should be noticed that the neighborhood relation of the lattice points has been changed by this transformation. For example, in Fig. 1a the lattice points (0, 1, 2, 3) form two unit triangles, Δ013 and Δ023, but in the resulting lattice in Fig. 1b the two unit triangles formed by the corresponding lattice points are Δ012 and Δ123. The scale factor γ = 3 is the sole possibility of directional scaling symmetry for stretching along the side of the unit triangle in the case of equilateral triangular lattice. For scaling along the bisector line of a unit triangle, the solely possible directional scaling symmetry is the contraction with a scale factor γ = 1/3, which is in fact the inversed transformation of the one described above. This provides a trivial example of directional scaling symmetry. In the case of square lattice, directions along the side or the diagonal of the unit square don't exhibit any scaling symmetry.

Figure 1
figure 1

A trivial example of directional scaling symmetry for equilateral triangular lattice, which is achieved along any side of a unit triangle with a scale factor γ = 3.

(a) The original lattice; (b) the transformation result of (a) along the connection line between points 0 and 3.

Full size image

The lack of scaling symmetry along the most notable <10>-directions in the square and equilateral triangular lattices does not compulsively exclude the possibility of directional scaling symmetry along other directions. Rather, we may even wish that such a directional scaling symmetry, if there is any, can be achievable in principle with more scale factors. We see that if such a directional scaling symmetry can be proven to exist and the corresponding transformation can be formulated, this will evidently promote our understanding of the structural properties of lattices and provide helpful insight into problems involving lattices such as in statistical physics, condensed matter physics, quantum theory and even number theory, etc.

In the effort of investigating the 1D incommensurate systems such as specified by the function cos(2πqn)1,2,3,4, where n is integer and the parameter q is an irrational number such as the golden ratio and 2D quasiperiodic structures5,6,7,8, we came across to the question whether there is any directional scaling symmetry for the square lattice and the equilateral triangular lattice (hence also the honeycomb lattice). We found that the square lattice exhibits directional scaling symmetry along a direction at 22.5° with respect to the side of a unit square, with the drag center of scaling transformation falling on the lattice point and the scale factor is , k = 1,2,3…. In the case of equilateral triangular lattice, the directional scaling symmetry appears at the direction at 15° with respect to any side of the unit triangle, with the drag center of scaling transformation falling on the lattice point and the scaling factor is , k = 1, 2, 3…. A proof based on the function y = arcsin(sin(2πxn)), where the parameter x is the silver number9,10,11 or the platinum number11,12,13, which are respectively related to the 8-fold and 12-fold quasiperiodic structures, is presented.

Results

Directional scaling symmetry in equilateral triangular lattice

The plot of the function y = sinn, where the argument n is non-negative integer (the discussion below is also valid for negative integer, but it is not of concern here), is essentially different from that for y = sinx, where x is real. This fact has been noticed and extensively studied by Strang14,15 and Richert16. In studying the 1D incommensurate structures, we found that the function y = sin(2πμn), where is the platinum number which is related to the dodecagonal quasiperiodic structure8,11,12,13, reveals an interesting picture as illustrated in Fig. 2a. In the boundary regions defined by y = ±1, the graph seems folding together, reminding us of Escher's paintings based on the concept of Poincaré disc. In the central region, however, the graph seems to display locally 12-fold rotational symmetry. This is quite reasonable since is the platinum number. If instead of y = sin(2πμn) we draw the function y = arcsin(sin(2πμn)), we see that the whole domain bounded by y = ±π/2 is globally isometric and the plot displays locally 12-fold rotational symmetry (in a not very strict sense), see Fig. 2b.

Figure 2
figure 2

Plots of the sinusoidal function y = sin(2πμn) (a) and the arcsine function y = arcsin(sin(2πμn)) (b), where and the argument n is non-negative integer.

Full size image

Interestingly, the plot of the function y = arcsin(sin(2πμn)) in Fig. 2b can be taken as a Moiré pattern17,18, i.e., as superposition of two identical simpler lattices (see Fig. S1). In fact, the function y = arcsin(sin(2πμn))itself can be divided into two branches

where both m, n are non-negative integer and if nμ − [nμ] ∈ [0, 3/4], m = [nμ]; if nμ − [nμ] − 1 ∈ [−1/4, 0], then m = [nμ] + 1. Here [x] denotes the truncation of the positive real number x. In the following the first branch in eq.(1) is referred to as the ascending branch, as points generated by this branch fall on the ascending part of the graph for y = arcsin(sinx)) (see Fig. S2) and the second branch is accordingly referred to as the descending branch. The plot of only the ascending branch results in Fig. 3a (for comparison of the two branches, see Fig. S1). From Fig. 3a we can readily find that the plot of the ascending branch constitutes an oblique 2D lattice. So does the plot of the descending branch. In fact, with a proper ratio of the longitudinal scale to the transverse scale the unit triangle in Fig. 3a can be made to have roughly three equal sides, thus the lattice is approximately an equilateral triangular lattice (to be further discussed below).

Figure 3
figure 3

(a) Plot of the ascending branch of the function y = arcsin(sin(2πμn)), where and n is non-negative integer; (b) The result of scaling along the horizontal axis with a scale factor of . Points are indexed with the corresponding argument n.

Full size image

If we compress Fig. 3a along the horizontal axis in a continuous way, the approximate equilateral triangle lattice will at first be distorted and then, when the scale factor comes to a proper value (), the shape of the lattice will again recover, as illustrated in Fig. 3b. This scenario can be repeated infinitely. More importantly, after each contraction, the unit triangle in the lattice can be brought closer to a rigorously equilateral triangle, in the sense that the side lengths suffer from a less relative deviation. And it can be proven that in the extreme case when the ratio of longitudinal scale to transverse scale approaches vanishingly small, the unit triangle turns into a rigorously equilateral triangle (see detailed proof in supplementary information). Notice that the transformation changes the neighborhood relation that, for instance, in Fig. 3a the two unit triangles anchored to the point n = 0 are Δ0-4-15 and Δ0-11-15, whereas after the transformation, the two unit triangles anchored to the point n = 0 are Δ0-15-56 and Δ0-41-56 (Fig. 3b).

Thus this manipulation leads us to the discovery that there exists directional scaling symmetry for the equilateral triangular lattice, which is a scaling transformation, setting the drag point on an arbitrary lattice point, along the direction at 15° with respect to the side of the unit triangle and the scale factor is . The ratio of side lengths involved in this transformation is . Such a scaling transformation can be performed repeatedly. This directional scaling symmetry for equilateral triangular lattice specified above can be easily checked (see detailed proof in supplementary information).

By the way, the equilateral triangular lattice is the superposition of a honeycomb lattice and a times larger equilateral triangular lattice. Taking the lattice in Fig. 3a as an equilateral triangular lattice, the index in the plot helps to specify the points to be removed so as to obtain a honeycomb lattice from the parent triangular lattice (The rules of doing this are clarified in the supplementary information). Obviously, the honeycomb lattice has also directional scaling symmetry and the scale factor and the ratio of side lengths for hexagons before and after the transformation are and , respectively. The drag point is set on the center of an arbitrary unit hexagon and the direction is at 15° with respect to the side of the hexagon. More interestingly, when a honeycomb lattice is obtained after scaling along that particular direction, the center of the unit hexagon remains the center of the unit hexagon in the resulting lattice. The honeycomb lattice and the equilateral triangular lattice share the same directional scaling symmetry may arise from the fact that honeycomb lattice is dual (reciprocal) to the equilateral triangular lattice.

Directional scaling symmetry in square lattice

With the silver number , which is related to the octagonal quasiperiodical structure7,9,10,11, we obtain an interesting plot of the function y = sin(2πλn) (Fig. 4a) in analog to Fig. 2a. Going one step further, we draw the plot of the function y = arcsin(sin(2πλn)), which is globally isometric and displays locally 8-fold rotational symmetry (in a not very strict sense), see Fig. 4b.

Figure 4
figure 4

Plots of the sinusoidal function y = sin(2πλn) (a) and the arcsine function y = arcsin(sin(2πλn)) (b), where and the argument n is non-negative integer.

Full size image

Again, the plot in Fig. 4b can be taken as a Moiré pattern formed by the superposition of two identical simpler lattices (see Fig. S3). Accordingly, the function y = arcsin(sin(2πλn)) can be separated into two branches

Where m, n are non-negative integers and if nλ − [nλ] ∈ [0, 3/4], then m = [nλ]; if nλ − [nλ] − 1 ∈ [−1/4, 0], then m = [nλ] + 1. As above, the first branch is referred to as the ascending branch of the function and the second branch is referred as the descending branch. Thus the plot of y = arcsin(sin(2πλn)) can be taken as the Moiré pattern formed by the overlapping plots for its ascending branch and descending branch (Fig. S3).

The ascending branch of the function y = arcsin(sin(2πλn))is plotted in Fig. 5a (For comparison of the two branches, see Fig. S3). One can easily check that the points in Fig. 5a form a square lattice, in an approximate sense, when a proper ratio of longitudinal scale to transverse scale is chosen (see detailed proof in supplementary information).

Figure 5
figure 5

(a) Plot of the ascending branch of the function y = arcsin(sin(2πλn)), where and n is non-negative integer; (b) The result of scaling along the horizontal axis with scale factor . Points are indexed with the corresponding argument n.

Full size image

If Fig. 5a is compressed along the horizontal axis, the shape of the approximate square unit will at first be distorted, then, when the scale factor comes to a value , the shape of the lattice will be recovered, as illustrated in Fig. 5b. This operation can be performed repeatedly. After each contraction, the approximate unit square gets closer to a rigorous square (see detailed proof in supplementary information). It can be proven that the approximate unit square turns into a rigorous square when the ratio of longitudinal scale to transverse scale becomes vanishing small (see detailed proof in supplementary information). Notice that the neighborhood relation of points in the lattice has been changed by contraction. For example, the unit square, anchored to the original point 0, is () in Fig. 5a, but after the contraction it is the square , see Fig. 5b. Moreover, the unit square is also rotated by 45° by the transformation.

Thus this manipulation leads us to the discovery that directional scaling symmetry exists for the square lattice, which is along the direction at 22.5° with respect to any side of a unit square and the scaling factor is . The ratio of the side lengths of the unit squares before and after transformation is (see detailed proof in supplementary information).

Thus by using the arcsine functions y = arcsin(sin(2πxn)), where the parameter x is either the platinum number or the silver number , we found and proved the existence of directional scaling symmetry for the equilateral triangular lattice (thus also the honeycomb lattice) and the square lattice. With the drag center set on a lattice point, in the case of equilateral triangular lattice, the direction of scaling symmetry is at 15° with regard to the side of the unit triangle and the scale factor is , while in the case of square lattice, the direction of scaling symmetry is at 22.5° with regard to the side of the unit square triangle and the scale factor is . In both cases the directional scaling transformation can be performed repeatedly.

Discussion

With the existence proof of directional scaling symmetry for the square lattice and equilateral triangular lattice, an immediate question will be raised: Are there more possibilities of scaling symmetry for these high-symmetry 2D lattices? Also it reminds us of the possible existence of directional scaling symmetry for 3D cubic and rhombic lattices. To both questions we will bet on a positive answer.

The method of proof involves applying trigonometric functions with the silver ratio and the platinum ratio in argument and approaching a property of the rigorously symmetrical lattices from approximate ones, is new and inspiring. To the least, such a function can be used to generate quasiperiodic lattices with enumerable lattice points, which is very helpful for the calculation of the diffraction pattern and energy bands for quasicrystals. It is of particular importance when the enumeration of the eigenfunctions for the Hamiltonian operator is of concern as in the study of topological insulator and the current work may help the search of topological insulators in quasicrystals19.

With the current work we want to call attention to the directional scaling symmetry for the equilateral triangular lattice and square lattice and the related silver ratio and platinum ratio, which are expected to have some impact on the various physics problems, particularly in statistical physics, condensed matter physics, quantum field theory, etc., modeled on high-symmetry 2D lattices. The scaling symmetry of a lattice will be incorporated into the Hamiltonian for a quantum model defined on it, which in turn will determine the feature of ground energy degeneracy-a pivotal concept for the discussion of quantum critical phenomenon. Remarkably, the golden ratio , the peer of the silver ratio and the platinum ratio here concerned, has been found lying beneath many fundamental physical problems and usually in unexpected places. For instance, the lowest two masses of the bound states, m1 and m2, in the 1D Ising model realized in CoNb2O6 crystal, have the ratio m1/m2 = φ, as predicted by E8 Lie group20. The critical fugacity for the hard-hexagon model is found to be zc = φ521, while the maximum of Hardy's probability, a quantity referring to the Hardy's test of Bell's inequality, for quantum system of arbitrary finite dimension is pHardy = 1/φ522. Such observations have not yet been well understood. It is anticipated by analogy that the silver ratio and the platinum ratio may also be found relevant in the physical problems defined on such lattices, e.g., J1-J2 XY model, triangular Ising antiferromagnet, etc. As in the case of the golden ratio, the discovery may demand years of meticulous research and will be made only in a serendipitous fashion.