Introduction

The prevailing view of the high-temperature (high-Tc) cuprate superconductors is that they are governed by intertwined orders1. In this scenario, different coupled unidirectional spin and charge orders (i.e., stripes) and their fluctuations compete/cooperate with unconventional superconductivity giving rise to a complex phase diagram1,2,3. This behavior is observed not only experimentally3,4,5,6,7,8 but also in single- and multi-band Hubbard models9,10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25,26,27,28. For example, non-perturbative numerical methods that access zero-temperature properties frequently identify several nearly degenerate stripe and d-wave superconducting states for model parameters that are relevant to the cuprates15,17,18,22,23,24,29. The state that ultimately wins out as the ground state, however, is sensitive to subtle factors like the value of the next-nearest-neighbor hopping \({t}^{{\prime} }\)22,23.

At finite temperature, quantum Monte Carlo (QMC) methods20,21 find fluctuating spin stripes at T ~ 0.22t, where t is the nearest neighbor hopping integral. More recently, weaker charge stripe fluctuations have been reported in DCA simulations on large extended clusters26, where the charge correlations were observed to develop after the spin correlations as the temperature is lowered in the hole-doped system. This hierarchy of the spin and charge correlations appears to be a general property of the singleband Hubbard model at finite temperature; it has been observed in subsequent finite-temperature constrained-path auxiliary-field QMC27 and DQMC25 simulations. Curiously, the behavior in real cuprates is reversed, where charge modulations tend to develop before spin modulations3.

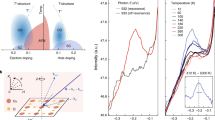
The above mentioned results cast doubt on the idea that the Hubbard model is the correct low-energy effective model for the cuprates 16,22,30. If this is true, then it is essential it identify the missing ingredient. While it is clear that strong electron correlations dominate cuprate physics, there is also a growing body of evidence that electron-phonon (e-ph) interactions are also relevant31,32,33,34,35,36,37,38,39,40,41,42,43. For example, several resonant inelastic x-ray scattering (RIXS) experiments have found evidence for short-range CDW order44,45, which appears to couple to the lattice as evidenced by anomalous softening of several oxygen phonon branches in the vicinity of the ordering wave-vector39,40,41,42. This behavior calls for studies of the influence of the e-ph interaction on stripe order, particularly in light of the apparent sensitivity of stripes to perturbing interactions like \({t}^{{\prime} }\).

The single-band Hubbard-Holstein model is the minimal model describing correlated electrons coupled to the lattice. This model has been widely studied at half-filling, where competition between antiferromagnetic Mott and Q = (π/a, π/a) charge-density-wave (CDW) insulating phases is commonly observed46,47,48,49,50,51,52,53. Away from half-filling, there are suggestions that the e-ph interaction can enhance d-wave pairing correlations for some parameter regimes49,54,55. However, we know comparatively little about how the e-ph interaction might influence stripe correlations and their competition with superconductivity.

Here we present a combined variational Monte Carlo (VMC) and determinant quantum Monte Carlo (DQMC) study of the two-dimensional doped Hubbard-Holstein model to determine how the Holstein coupling alters static and fluctuating stripe correlations. We find that the coupling to the lattice can enhance the charge component of the stripes while also suppressing their spin component, depending on the value of the specific model parameters. Our results show that the e-ph coupling can alter the balance between the stripe and superconducting correlations and suggest a potential solution to how charge-stripes might appear before spin-stripes in a real material.

Results

Stripe order at zero temperature

We first examine the static stripe order in the Hubbard-Holstein model at T = 0 using VMC, focusing on the 〈n〉 = 0.875, \({t}^{{\prime} }=-0.25t\), and U = 8t case (see Methods and Supplementary Notes 1 and 2). Additional results for \({t}^{{\prime} }=0\) are also shown here and in Supplementary Note 3.

For these parameters, we find that a long-range static stripe order produces a lower estimate for the ground state energy than a uniform state. For example, Fig. 1a, b plot the expectation values of the local staggered spin \({S}_{i}^{{{{{{{{\rm{stag}}}}}}}}}={(-1)}^{{i}_{x}+{i}_{y}}\langle {\hat{S}}_{i}^{z}\rangle\) and local excess hole density \({\rho }_{i}=1-\langle {\sum }_{\sigma }{\hat{n}}_{i,\sigma }\rangle\) operators, respectively, obtained from our optimized variational state when the e-ph coupling λ = 0. The results reveal the typical intertwined unidirectional spin and charge stripe observed in the cuprates, where antiphased regions of antiferromagnetically (AFM) ordered spins are separated by vertical hole-rich regions. For this value of \({t}^{{\prime} }\), the spin and charge modulations have periods \(\frac{1}{2}{\lambda }_{{{{{{{{\rm{spin}}}}}}}}}={\lambda }_{{{{{{{{\rm{charge}}}}}}}}}\approx 4a\). We also find that the stripe solution is lower in energy for \({t}^{{\prime} }=0\), but with a different period \(\frac{1}{2}{\lambda }_{{{{{{{{\rm{spin}}}}}}}}}={\lambda }_{{{{{{{{\rm{charge}}}}}}}}}\approx 8a\), see Supplementary Note 3. These results are in agreement with a prior VMC study56 of the Hubbard model that utilized square clusters, suggesting that our choice of cluster geometry does not introduce a significant bias towards the stripe solution.

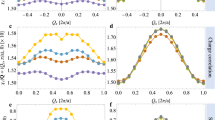
Fig. 1: Variational Monte Carlo (VMC) results for static stripe order in the two-dimensional Hubbard-Holstein model.
figure 1

a The expectation value of the staggered local spin operator in real space \({S}_{i}^{{{{{{{{\rm{stag}}}}}}}}}={(-1)}^{{i}_{x}+{i}_{y}}\langle {\hat{S}}_{i}^{z}\rangle\), where \({S}_{i}^{z}=\frac{1}{2}({\hat{n}}_{i,\uparrow }-{\hat{n}}_{i,\downarrow })\) is the z-component of the local spin operator. Results are shown here for U = 8t, \({t}^{{\prime} }=-0.25t\), 〈n〉 = 0.875, and λ = 0. b The expectation value of the local excess hole density \({\rho }_{i}=1-\langle {\sum }_{\sigma }{\hat{n}}_{i,\sigma }\rangle\) for the same case. c, d shows \({S}_{i}^{{{{{{{{\rm{stag}}}}}}}}}\) and ρi, respectively, along the line (ix, 0) for representative values of λ = 0, 0.5 and Ω = 5t. The data points are the VMC values of the local quantity and the solid lines are sinusoidal fits to the VMC data. e, f shows the fitted amplitudes of the spin and charge modulations, respectively, as a function of λ for \({t}^{{\prime} }=-0.25t\) and various Ω. The insets of these panels show corresponding data for \({t}^{{\prime} }=0\). The error bars in this figure (calculated from the sample standard deviation of our measurements) are smaller than the data points.

Full size image

In the limit of Ω → ∞, the Holstein model can be mapped onto an effective, attractive Hubbard model with U = − λW, reflecting the effective, attractive e-e interaction mediated by the lattice. Therefore, for large Ω, we can crudely estimate the effects of the e-ph coupling by replacing U → Ueff = U − λW. For smaller Ω, this picture still provides a valuable guide for qualitatively understanding the physics of the model; however, additional retardation effects can play a role47,48,52. Based on these considerations, we naively expect λ ≠ 0 will suppress any correlation-driven phenomena for large Ω. Indeed, prior theoretical work at half-filling has shown that the line λW ≈ U defines an approximate boundary between AFM and CDW phases46,47,48,50,52,53.

With this picture in mind, we now examine the influence of the e-ph coupling on the static stripes. Figure 1c, d plot \({S}_{i}^{{{{{{{{\rm{stag}}}}}}}}}\) and ρi along the Ri = a(ix, 0) direction for representative values of λ = 0, 0.5 and Ω = 5t. (The data for the other values of Ω are similar and provided in Supplementary Note 3). A non-zero coupling λ ≠ 0 reduces the magnitude of both modulations as is evident in the raw VMC data, plotted here as the data points. To quantify this observation across our entire set of simulations, we fit the VMC data with sinusoidal functions, as exemplified by the solid lines. The fitted amplitudes for \({S}_{i}^{{{{{{{{\rm{stag}}}}}}}}}\) and ρi are plotted in Fig. 1e, f, respectively, where results for \({t}^{{\prime} }=-0.25t\) are shown in the main panel and results for \({t}^{{\prime} }=0\) are shown in the insets.

For both values of \({t}^{{\prime} }\), we find that the Holstein interaction does not change the underlying period of the stripes. It can, however, shift the stripes from being site-centered to bond-centered for some model parameters. For example, when \({t}^{{\prime} }=-0.25t\) we obtain a site-centered stripe when λ = 0, which shifts to being bond-centered when λ ≠ 0, as shown in Fig. 1c, d. This shift occurs for almost all of the non-zero values of the e-ph coupling that we have checked (see Supplementary Note 4). Conversely, when \({t}^{{\prime} }=0\), we mostly obtain a bond-centered stripe, independent of the values of λ and Ω.

The e-ph coupling also affects the amplitudes of the spin and charge modulations. Increasing λ for \({t}^{{\prime} }=-0.25t\) suppresses both the spin and charge modulations, and the rate of suppression increases as the phonon frequency Ω increases. We find qualitatively similar behavior for large Ω when \({t}^{{\prime} }=0\), as shown in the insets. However, for lower energy phonons (Ω ≲ 2t), the spin modulations are weakly suppressed when λ ≤ 0.75 while the amplitude of the density modulations increases. These results indicate that a Holstein coupling to high-energy phonons suppresses static stripe order while coupling to lower-energy optical modes can stabilize them by enhancing their charge modulations, depending on the value of \({t}^{{\prime} }\).

Having assessed the effects of the e-ph coupling on stripe correlations, we now turn to the relative energies of the stripe and superconducting states. Figures 2 and 3 compare the variational energies of the stripe and uniform solutions for \({t}^{{\prime} }=0\) and − 0.25t, respectively. Focusing first on the \({t}^{{\prime} }=0\) case, Fig. 2a plots the expectation value of the system’s total energy as a function of λ for the optimized variational stripe states, like those shown in Fig. 1. The stripe state’s energy decreases approximately linearly with λ for all Ω, but the rate of decrease is higher for larger phonon energies. The inset of Fig. 2a shows the corresponding data for a uniform state, where we observe similar behavior.

Fig. 2: Variational Monte Carlo estimates for the energies of the Hubbard-Holstein model with \({{{{{{{\bf{{t}}}}}}}^{{\prime} }=\; 0}}\).
figure 2

Results are shown for various phonon energies Ω as a function of λ. a shows the expectation value of the Hamiltonian Estripe for a state with static spin and stripe correlations. The inset shows the expectation value of the Hamiltonian Euniform for a state with uniform d-wave pairing and antiferromagnetic correlations. b plots the difference ΔE = Estripe − Euniform as a function of λ. All energies are reported in units of t. The error bars in this figure (calculated from the sample standard deviation of our measurements) are smaller than the data points.

Full size image

The dominant contribution to the change in energy can be attributed to the shift in the lattice’s equilibrium position when λ ≠ 048, which lowers the energies of the uniform and stripe states by a comparable amount. More subtle differences are observed when we examine the energy differences between the two states, as shown in Fig. 2b. When λ = 0, we find that the stripe state is 0.01437t/site lower in energy than the uniform state, such that the stripe solution is a better approximation for the ground state at this doping, consistent with refs. 18 and 24. For Ω > 2t, increasing λ reduces the energy difference between these states, signaling that the stripe state is suppressed relative to the uniform superconducting state. Conversely, for Ω = t, increasing λ stabilizes the stripe order. For intermediate Ω = 2t, we observe nonmonotonic behavior, where the stripe correlations are enhanced at small λ < 0.5 but suppressed for larger values.

The results for \({t}^{{\prime} }=-0.25t\), shown in Fig. 3, show that the e-ph coupling lowers the energy difference between the stripe and uniform solutions at all Ω. However, the effect is reduced as the phonon energy decreases.

Fig. 3: Variational Monte Carlo estimates for the energies of the Hubbard-Holstein model with \({{{{{{{\bf{{t}}}}}}}^{{\prime} }=\; -0.25t}}\).
figure 3

Results are shown for various phonon energies Ω as a function of λ. a shows the expectation value of the Hamiltonian Estripe for a state with static spin and stripe correlations. The inset shows the expectation value of the Hamiltonian Euniform for a state with uniform d-wave pairing correlations and antiferromagnetic order. b plots the difference ΔE = Estripe − Euniform as a function of λ. All energies are reported in units of t. The error bars in this figure (calculated from the sample standard deviation of our measurements) are smaller than the data points.

Full size image

The results in Figs. 1–3 suggest that anti-adiabatic phonons destabilize static stripe order relative to the uniform state by suppressing both the spin and charge modulations. However, lower-energy phonons can have the opposite effect, increasing the strength of charge modulations while leaving the spin modulations relatively unchanged when \({t}^{{\prime} }=0\). While we can understand the large Ω behavior in terms of a reduced Ueff, the small Ω behavior, which is more relevant for the high-Tc cuprates, highlights the critical role of retardation. For example, studies examining the competition between AFM and CDW in the half-filled Hubbard-Holstein model generally find that the AFM correlations can persist beyond the line defined by U = λW when Ω ≤ t. In this regime, the lattice cannot respond quickly enough to the double occupations created in the AFM exchange process and is less effective in suppressing the AFM correlations. A similar effect appears to be at play here, as the AFM spin stripe correlations are less affected by the e-ph interaction as Ω decreases. At the same time, smaller values of Ω correspond to softer springs, which means that the hole-rich regions can lower their energy more readily by forming large local lattice distortions for a fixed value of λ. These combined effects provide a plausible explanation for our VMC results.

Phase separation

If the system is in a stable phase, then the ground state energy as a function of filling is convex, i.e., ∂2E(n)/∂n2 > 0. One can determine when this condition is violated by calculating the energy per hole57

$$\varepsilon (\delta )=\frac{E(\delta )-E(0)}{\delta },$$
(1)

where \(\delta =1-\langle \hat{n}\rangle\) is the excess hole density. If ϵ(δ) has a local minimum at some hole doping δc, then the system will phase separate for any δ < δc. Using this approach, several VMC studies have observed a tendency towards phase separation in the lightly hole doped Hubbard model56,57,58. For example, Ido et al. (ref. 56) concluded that phase separation occurs for doping levels δ < 0.125 in the singleband model when U = 10t and \({t}^{{\prime} }=0\). But VMC tends to over predict the value of δc; this occurs because the division by δ in Eq. (1) magnifies small errors in the variational energies E(δ) at low doping levels. Indeed, in ref. 58 systematic improvements of the variational energies via Greens function Monte Carlo with the Fixed Node approximation drove the critical doping level δc very close to zero. The nature of the correlations in the ground state did not change as the wave function was improved.

With these caveats in mind, Fig. 4 examines ε(δ) for our model parameters for completeness. For λ = 0 [Fig. 4a], we find that ε(δ) reaches a local minimum at δc = 0.125 for both \({t}^{{\prime} }=0\) and − 0.25t, in agreement with ref. 56. Once the e-ph interaction is included, the local minima develop at δc = 0.125 and 0.167 for \({t}^{{\prime} }=-0.25t\) and all values of Ω, with the latter being the global minimum. The predicted tendency towards phase separation is more severe for the case of \({t}^{{\prime} }=0\) with the region extending to δc = 0.229, as shown in the inset of Fig. 4b.

Fig. 4: Phase separation tendencies predicted by variational Monte Carlo.
figure 4

a, b shows the energy per hole ε(δ) for λ = 0 and λ = 0.25, respectively. Results are shown for \({t}^{{\prime} }=-0.25t\) and \({t}^{{\prime} }=0\) (insets) at different phonon energies. The statistical error bars in this figure (calculated from the sample standard deviation of our measurements) are smaller than the data points. The red arrows indicate the doping level of the variational Monte Carlo calculations shown in Figs. 1–3. The black arrows indicate the global minimum of ε(δ).

Full size image

These results suggest that the Holstein coupling increases the tendency toward phase separation commonly found in the Hubbard and t-J models56,57,58. The doping level of our simulations, indicated by the red arrows, fall below δc when λ ≠ 0. However, the local minima are largely determined by the rapid growth in ε(δ) at low doping, which can be dramatically impacted by small errors in E(δ). Indeed, ref. 58 found that this rapid rise all but disappears in the λ = 0 case as the variational wave functions are improved. We expect a similar change to occur here for λ ≠ 0; however, more detailed simulations will be needed to verify this conjecture. Nevertheless, we believe that we are overestimating δc, and the actual value is smaller. This conjecture is also supported by the fact that we find no indications for phase separation in our finite temperature DQMC results discussed in the next section.

Stripe correlations at finite temperature

We now turn to the influence of the Holstein coupling on the fluctuating stripe correlations observed by DQMC at finite temperatures (see Methods and Supplementary Note 5). Fluctuating stripes are challenging to resolve in the Hubbard model at high-temperatures. For this reason, we focused on N = 16 × 4 clusters with \(\langle \hat{n}\rangle =0.8\) with \({t}^{{\prime} }=-0.25t\), U = 6t, and β = 4/t, where the fluctuating spin stripe correlations are particularly strong21. Figure 5a plots the real-space staggered static spin–spin correlation function

$${C}_{{{{{{{{\rm{spin}}}}}}}}}^{{{{{{{{\rm{stag}}}}}}}}}({{{{{{{{\bf{r}}}}}}}}}_{i})=\frac{1}{N}\int\nolimits_{0}^{\beta }{(-1)}^{{i}_{x}+{i}_{y}}\mathop{\sum}\limits_{j}\left\langle {\hat{S}}_{i+j}^{z}(\tau ){\hat{S}}_{j}^{z}(0)\right\rangle d\tau ,$$

measured in DQMC simulations for several values of λ and Ω = t/2. (Results for Ω = t and Ω = 2t are provided in Supplementary Note 6). Figure 5b shows the corresponding static density-density correlation function

$${C}_{{{{{{{{\rm{den}}}}}}}}}({{{{{{{{\bf{r}}}}}}}}}_{i})=\frac{1}{N}\int\nolimits_{0}^{\beta }\mathop{\sum}\limits_{j}\left[\langle {\hat{n}}_{i+j}(\tau ){\hat{n}}_{j}(0)\rangle -\langle {\hat{n}}_{i+j}(\tau )\rangle \langle {\hat{n}}_{j}(0)\rangle \right]d\tau .$$
Fig. 5: Determinant quantum Monte Carlo results for the fluctuating stripe correlations in real space.
figure 5

Results are shown for the (a) spin and (b) charge stripes at a filling of \(\langle \hat{n}\rangle =0.8\) and an inverse temperature of β = 4/t, obtained on N = 16 × 4 clusters with \({t}^{{\prime} }=-0.25t\), U = 6t, and Ω = t/2. Each row in (a) shows the real-space static staggered spin-spin correlation function at different values of λ. The panels in (b) show the corresponding density-density correlation functions.

Full size image

For λ = 0 [top row, Fig. 5a, b], we observe fluctuating spin and charge stripe correlations consistent with prior work21,25,26. The spin correlations are evident from the short-range AFM correlations (blue) surrounded by other AFM domains where the magnetic correlations are flipped (red). At this temperature, the charge stripes are weak but still present.

As with the VMC results for this value of \({t}^{{\prime} }\), we find that the spin correlations are reduced when we introduce the e-ph coupling. This suppression occurs gradually for weak coupling, but it accelerates as λ increases. For example, for λ = 0.5, we already see that the red domains in \({C}_{{{{{{{{\rm{spin}}}}}}}}}^{{{{{{{{\rm{stag}}}}}}}}}({{{{{{{{\bf{r}}}}}}}}}_{i})\) are nearly absent while the AFM correlations persist over longer length scales. As λ increases further, the AFM correlations extend over a larger distance. This behavior is presumably due to the attractive interaction mediated by the phonons, which counteracts the correlations generated by the Hubbard U. In contrast to the spin correlations, the charge correlations remain more robust for λ ≤ 0.5 but begin to develop an additional short-range Q = (π, π) modulation. These new modulations signal the formation of local bipolarons59, which tend to arrange themselves in local checkerboard-like order. Finally, the (π, π) modulation dominates over the entire cluster for the largest value of λ studied here.

So far, we have focused on results for Ω = t/2; however, we have obtained similar results for Ω = t and 2t (see Supplementary Note 6). The trends across the data set are more easily summarized by examining the momentum-dependent static spin S(Q, iωn = 0) and charge N(Q, iωn = 0) susceptibilities, shown in Fig. 6. These quantities are obtained by Fourier transforming the unequal time spin-spin and density-density correlation functions and integrating over imaginary time.

Fig. 6: Determinant quantum Monte Carlo results for the fluctuating stripe correlations in momentum space.
figure 6

All results were obtained at a filling of \(\langle \hat{n}\rangle =0.8\) and an inverse temperature of β = 4/t using N = 16 × 4 clusters with \({t}^{{\prime} }=-0.25t\) and U = 6t. Panels (a) through (d) show the static spin susceptibility for different values of λ. Panels (e) through (h) show the corresponding charge susceptibility. Results are shown for Ω = t/2, t, and 2t, as indicated in the legends provided in the second column.

Full size image

The spin stripe correlations manifest in S(Q, iωn = 0) as incommensurate peaks at Q = (π ± δs, π). As with the real-space picture, we find that δs is reduced for small λ, causing the double peaks to merge into a single broad peak centered at (π, π). However, in most cases, we can still discern two components by fitting a set of lorentzian functions to the data (see Supplementary Note 6). For large λ, S(Q, 0) approaches a single AFM peak, which is suppressed as Ω increases due to the reduction of Ueff and increasing competition with the competing CDW phase.

The charge stripes manifest in N(Q, iωn = 0) as incommensurate peaks centered at Q = (δc, 0)26. In the absence of e-ph coupling, we clearly observe this structure with δs/δc ≈ 0.45 at β = 4/t, consistent with ref. 26. These peaks remain well defined for λ ≤ 0.5 for all values of Ω, while the overall magnitude of the charge correlations increases uniformly with decreasing Ω. It is only for stronger e-ph coupling (λ = 0.75) that δc is shifted towards π as the phonon frequency is decreased, signaling a suppression of the charge stripes. We also note that increasing λ enhances the Q = (π, π) charge correlations due an increased tendency towards bipolaron formation. In fact, the (π, π) correlations become significantly larger than the (δc, 0) ones if λ is too large, consistent with the real space picture shown in Fig. 5.

The overall picture obtained from our DQMC results (\({t}^{{\prime} }=-0.25t\)) is that the Holstein interaction tends to suppress the fluctuating stripe correlations but that the effect is more pronounced for the spin correlations at small Ω and weak to intermediate coupling. For strong coupling and/or large Ω, the e-ph interaction suppresses both channels due to the competition with (π, π) charge correlations, bipolaron tendencies, or an overall reduction in Ueff. These results are in agreement with our zero-temperature VMC results for \({t}^{{\prime} }=-0.25t\). These results demonstrate that the e-ph interaction can have a non-trivial effect on static and fluctuating stripe correlations.

Discussion

Several numerical methods have found evidence that a Holstein interaction can enhance d-wave pairing correlations in the doped singleband Hubbard-Holstein model49,54,55. Some researchers have linked this enhancement to a non-trivial screening of the e-ph interaction, which reduces large-q scattering relative to small q36,54,60. Our results suggest that the lattice can affect superconductivity in another way by altering the competing stripe phases. Different optical phonon branches can play different roles in this context: high-energy phonons generally suppress both spin and charge stripes. In contrast, low-energy phonons can enhance them by increasing the charge modulations. Since cuprate optical oxygen phonons have Ω ⩽ t/3, we expect that the latter regime is more relevant to these materials. Our results, therefore, provide a natural framework for understanding anomalous oxygen isotope effects observed in materials like La2−xSrxCuO461. They also suggest an explanation for why charge modulations tend to develop before spin modulations in the cuprates, even though fluctuating spin stripes appear to be stronger in the doped Hubbard model.

Why does the e-ph interaction primarily couple to the charge modulations? To some extent, this might be expected since the phonons couple directly to the local charge density. There are other factors to consider, however. For one, stripe formation is a form of phase separation that emerges as a compromise in balancing the doped carriers’ kinetic and potential energies. Several QMC studies of the Holstein model have found evidence that it is prone to phase separation when doped away from half-filling50,62,63, which is stronger for low-energy phonons at high temperatures50,63. An enhancement of the e-ph coupling at small-q can further exacerbate this tendency64,65. In the case of the doped Hubbard-Holstein model, these factors then cooperate in collecting the doped carriers into particular spatial regions of the system, which would explain our observations.

Many models and experimental measurements on the cuprates estimate the dimensionless e-ph coupling to the oxygen-derived phonon modes to be in the range λ ~ 0.3 − 131,32,33,34,36,37. Combined with our results, these estimates suggest that the e-ph coupling could play a role in shaping the stripe correlations. The relevant phonon frequencies in the real materials (Ω ≈ t/3) are smaller than the values considered here. With this in mind, our results for \({t}^{{\prime} }=-0.25t\) suggest that a Holstein interaction will be insufficient for stabilizing the superconducting state. However, more sophisticated e-ph models must also be examined before we can draw definitive conclusions about the real materials. For example, coupling to the bond-buckling modes occurs via the oxygen on-site (potential) energy and has a significant momentum dependence32,60, unlike the Holstein model studied here. Similarly, coupling to the bond-stretching modes occurs via a Su-Schrieffer-Heeger (SSH) type interaction32,60, which modulates the carrier’s kinetic energy66,67. (The latter case is particularly interesting since the largest phonon softening in the cuprates typically occurs for the high-energy bond-stretching modes in proximity to CDW order39,40). To fully understand the role of these interactions on cuprate stripes, it will be necessary to study generalizations of the three-band model, which can capture these aspects of the relevant phonon modes. Nevertheless, our results demonstrate that e-ph coupling cannot be neglected in any complete picture of stripe physics.

Methods

We study the singleband Hubbard-Holstein model, defined on a two-dimensional (2D) square lattice. Its Hamiltonian is H = Hel + Hph + He−ph, where

$${H}_{{{{{{{{\rm{el}}}}}}}}}=-\mu \mathop{\sum}\limits_{i}{\hat{n}}_{i}-\mathop{\sum}\limits_{i,j,\sigma }{t}_{ij}^{{\phantom{{\dagger}}} }{c}_{i,\sigma }^{{{{\dagger}}} }{c}_{j,\sigma }^{{\phantom{{\dagger}}} }+U\mathop{\sum}\limits_{i}{\hat{n}}_{i,\uparrow }{\hat{n}}_{i,\downarrow },$$

describes the electronic subsystem,

$${H}_{{{{{{{{\rm{ph}}}}}}}}}={{\Omega }}\mathop{\sum}\limits_{i}\left({b}_{i}^{{{{\dagger}}} }{b}_{i}^{{\phantom{{\dagger}}} }+\frac{1}{2}\right)$$

describes the phononic subsystem, and

$${H}_{e-{{{{{{{\rm{ph}}}}}}}}}=g\mathop{\sum}\limits_{i}{\hat{n}}_{i}\left({b}_{i}^{{{{\dagger}}} }+{b}_{i}^{{\phantom{{\dagger}}} }\right)$$

describes their coupling. Here, \({c}_{i,\sigma }^{{{{\dagger}}} }\) (\({c}_{i,\sigma }^{{\phantom{{\dagger}}} }\)) creates (annihilates) a spin-σ ( = ↑, ↓) electron on site i, \({b}_{i}^{{{{\dagger}}} }\) (\({b}_{i}^{{\phantom{{\dagger}}} }\)) creates (annihilates) a dispersionless optical phonon at lattice site i with energy Ω, \({\hat{n}}_{i,\sigma }={c}_{i,\sigma }^{{{{\dagger}}} }{c}_{i,\sigma }^{{\phantom{{\dagger}}} }\) and \({\hat{n}}_{i}={\sum }_{\sigma }{\hat{n}}_{i,\sigma }\), tij is the hopping integral between sites i and j, ri = a(ix, iy) (\({i}_{x(y)}\in {\mathbb{Z}}\)) is a lattice vector, μ is the chemical potential, U is the Hubbard repulsion, and g is the e-ph coupling strength. Throughout, we set tij = t for nearest neighbors, \({t}_{ij}={t}^{{\prime} }\) for next-nearest neighbors, and tij = 0 otherwise, and vary Ω and g over a range of values. Finally, we set t = a = 1 and adopt a standard parameterization of the dimensionless e-ph coupling with \(\lambda =\frac{2{g}^{2}}{W{{\Omega }}}\approx \frac{{g}^{2}}{4t{{\Omega }}}\), where W ≈ 8t is the electronic bandwidth.

We study the model using VMC and DQMC, two nonperturbative numerical methods capable of treating both the e-e and e-ph interactions on an equal footing. VMC is a zero-temperature method that uses Markov chain Monte Carlo to optimize a variational estimate for the system’s ground state wave function. Here, we use the method as described in ref. 50, applied to rectangular N = 16 × 6 clusters with periodic boundary conditions (PBC). We have considered two different variational wave functions which we label as uniform and stripe solutions. Our uniform wave function includes a (π, π) antiferromagnetic (AFM) order parameter and uniform d-wave pairing order. Our stripe wavefunction includes inhomogeneous state with both spin and charge density modulations. DQMC is a numerically exact auxiliary field method that solves finite-size clusters within the grand canonical ensemble. We use the technique as outlined in ref. 48, applied to rectangular N = 16 × 4 clusters with PBC. Additional details of our specific simulation parameters for both methods are provided in the Supplementary Notes 1 and 5.

We note that both DQMC and VMC are generally restricted to phonon energies Ω/t ≳ 1 for the values of the dimensionless coupling λ considered here. This restriction arises due to issues with the autocorrelation time of the simulations48,50. We will consider a range of Ω/t ∈ [1, 5] ( ∈ [0.5 − 2] for DQMC) to get a broad view of the physics, but the value relevant to the high-Tc cuprates is lower (Ω ≈ t/3, assuming t ≈ 300 meV). The reader should bear this in mind.