Two-copy Quantum Teleportation

Abstract

We investigate two-copy scenario of quantum teleportation based on Bell measurements. The detailed protocol is presented and the general expression of the corresponding optimal teleportation fidelity is derived, which is given by the two-copy fully entangled fraction that is invariant under local unitary transformations. We prove that under a specific case of the protocol, which is significant for improving the optimal fidelity, the set of states with their two-copy fully entangled fractions bounded by a threshold value that required for useful two-copy teleportation is convex and compact. Hence the witness operators exist to separate states that are useful for two-copy teleportation from the rest ones. Moreover, we show that the optimal fidelity of two-copy teleportation surpasses that of the original one copy teleportation.

Introduction

Quantum teleportation plays an important role in quantum information processing^1,2. It gives ways to transmit an unknown quantum state from a sender traditionally named “Alice” to a receiver “Bob” who are spatially separated, using classical communication and quantum resources^3,4,5,6. In^7,8, the authors consider the original one copy teleportation: Alice and Bob previously share a pair of particles in an arbitrary mixed entangled state χ. In order to teleport an unknown state to Bob, Alice first performs joint Bell measurement on her particles and tells the results to Bob by classical communication. Bob tries his best to choose particular unitary transformations to get the optimal transmission fidelity. The optimal transmission fidelity of such teleportation is given by the fully entangled fraction (FEF)⁹ of the quantum resource. It shows that when the resource χ is a maximally entangled pure state, the corresponding optimal fidelity is equal to 1. However, Alice and Bob usually share a mixed entangled state due to decoherence, and the optimal fidelity is less than 1.

One way to improve the fidelity of teleportation is to distill entanglement¹⁰, which refers to the procedure of converting mixed entangled states into singlets by using many copies of the entangled resources. The distillation of pure states is often referred to as entanglement concentration¹¹. For mixed states, since the distillation protocol presented in¹⁰, fruitful results have been obtained^12,13,14,15. However, the problem of distillation is that the complicated protocol may have to be repeated for infinitely many times to bring out a singlet. Moreover, in each round the desired results are usually obtained probabilistically, usually with an extremely low possibility to get an expected measurement outcome.

Inspired by this, to improve the teleportation fidelity, we propose two-copy quantum teleportation scenario directly, instead of bringing out a singlet as the resource of traditional one copy teleportation scenario. Specifically, we introduce a quantum teleportation protocol based on Bell measurements. The corresponding optimal teleportation fidelity is derived analytically. The fact that the optimal fidelity of the two-copy teleportation can surpass that in one copy scenario (the traditional quantum teleportation) is shown by analytical means together with numerical methods. In particular, we discuss a specific case which is significant for improving the optimal fidelity. It shows that the set of quantum states with their two-copy fully entangled fraction bounded by a threshold value required for useful two-copy teleportation is convex and compact, which demonstrates the existence of teleportation witness^16,17,18 in two-copy quantum teleportation.

Results

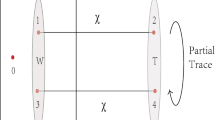
The two-copy teleportation protocol based on Bell measurements is as follows. Initially Alice and Bob share two pairs of entangled resources, see Fig. 1. Particles 1 and 2 (resp. 3 and 4) are in an entangled state χ. Particles 1 and 3 are in Alice’s side, while particles 2 and 4 are in Bob’s side. Alice wants to transmit an unknown state ρ_in of particle 0 to Bob. Firstly, Alice (resp. Bob) performs a joint local unitary operation W (resp. V) on particles 1 (resp. 2) and particle 3 (resp. 4). Then she makes joint Bell measurement on particles 0 and 1. She informs Bob the measurement results by classical means. According to these measurement results, Bob chooses corresponding unitary transformations {T} on particle 2 to achieve the optimal teleportation fidelity.

Figure 1

Scheme of two-copy teleportation protocol based on Bell measurements. Alice and Bob share two copies of entangled resource χ, with particles 1 and 3 in Alice’s side, and particles 2 and 4 in Bob’s side. Alice wants to transmit the unknown state ρ_in of particle 0 to Bob with optimal fidelity. The two-copy teleportation protocol based on Bell measurements is as follows: firstly, Alice (resp. Bob) performs a joint local unitary operation W (resp. V) on particles 1 (resp. 2) and particle 3 (resp. 4) to correlate these two particles. Then Alice makes joint Bell measurement on particles 0 and 1 and informs Bob the measurement results by classical communication. According to the measurement results, Bob chooses corresponding unitary transformations {T} on his particles 2 and 4 to restore the input state ρ_in on particle 2.

Let H denote an n-dimensional Hilbert space, with {|j〉, j = 0, ..., n − 1, n < ∞} as orthogonal normalized basis. A set of unitary matrices {U_st} in H can be written as: U_st = h^tg^s, where h and g are n × n matrices such that h|k〉 = |(k + 1)/mod n〉 and g|k〉 = w^k|k〉, with w = exp{−2iπ/n}. {U_st} has the following relations¹⁹: ${\rm{tr}}({U}_{st}^{\dagger }{U}_{s^{\prime} t^{\prime} })=n{\delta }_{tt^{\prime} }{\delta }_{ss^{\prime} }$, ${U}_{st}{U}_{st}^{\dagger }={I}_{n\times n}$, where I is the identity matrix. The generalized Bell states⁷ are given by $|{{\rm{\Phi }}}_{st}\rangle =({I}_{n\times n}\otimes {U}_{st})|{\rm{\Phi }}\rangle$, where $|{\rm{\Phi }}\rangle =|{{\rm{\Phi }}}_{{\rm{00}}}\rangle =\frac{1}{\sqrt{n}}{\sum }_{j=0}^{n-1}\,|jj\rangle$ is the maximally entangled pure state. The n² generalized Bell states $\{|{{\rm{\Phi }}}_{st}\rangle \}=\frac{1}{\sqrt{n}}{\sum }_{j,k}\,{({U}_{st})}_{jk}^{\ast }|jk\rangle$ form a complete orthogonal normalized basis of the H ⊗ H space. Throughout this paper we adopt the standard notations: for any matrix A∈End(H), A_a is an embedded operator in the tensor space H ⊗ H ⊗ … ⊗ H, which acts as A on the α-th space and as identity on the other spaces; and for any matrix U ∈ End(H ⊗ H), U_αβ is an embedded operator in H ⊗ H ⊗ … ⊗ H, which acts as identity on the spaces except for the α-th and β-th ones. After some tedious calculation, we get the output state under the scenario of two-copy teleportation:

Lemma 1

For any unknown input state ρ_in, the two-copy teleportation protocol under Bell measurements maps the state ρ_in to state ${{\rm{\Lambda }}}_{{\chi }^{\otimes 2}}^{\{{T}_{st},W,V\}}({\rho }_{in})$,

$$\begin{array}{rcl}{{\rm{\Lambda }}}_{{\chi }^{\otimes 2}}^{\{{T}_{st},W,V\}}({\rho }_{in}) & = & \frac{1}{{n}^{3}}\sum _{{s}_{1},{t}_{1}}\sum _{{s^{\prime} }_{1}{t^{\prime} }_{1}}\sum _{{s}_{2},{t}_{2}}\sum _{{s^{\prime} }_{2}{t^{\prime} }_{2}}\sum _{s,t}\,\langle {{\rm{\Phi }}}_{{s}_{1}{t}_{1}}|\chi |{{\rm{\Phi }}}_{{s^{\prime} }_{1}{t^{\prime} }_{1}}\rangle \langle {{\rm{\Phi }}}_{{s}_{2}{t}_{2}}|\chi |{{\rm{\Phi }}}_{{s^{\prime} }_{2}{t^{\prime} }_{2}}\rangle \\ & & \times \,{{\rm{tr}}}_{4}[({T}_{st}{)}_{2}{V}_{24}{({U}_{{s}_{1}{t}_{1}})}_{2}{({U}_{{s}_{2}{t}_{2}})}_{4}{W}_{24}{({U}_{st})}_{2}^{\dagger }{({\rho }_{in})}_{2}\\ & & \times \,{({U}_{st})}_{2}{W}_{24}^{\dagger }{({U}_{{s^{\prime} }_{1}{t^{\prime} }_{1}}^{\dagger })}_{2}{({U}_{{s^{\prime} }_{2}{t^{\prime} }_{2}})}_{4}^{\dagger }{V}_{24}^{\dagger }{({T}_{st})}_{2}^{\dagger }],\end{array}$$

(1)

where W and V are the unitary transformations Alice and Bob apply to their two particles, respectively. T_st ∈ {T} is the unitary operator that Bob performs on particle 2 to achieve the optimal teleportation fidelity.

Proof.

First consider that the unknown initial input state ρ_in that Alice wants to teleport is a pure state, $|\varphi \rangle ={\sum }_{\nu }{\alpha }_{\nu }|\nu \rangle$.

• 1). The two entangled resource states shared by Alice and Bob are pure: χ^⊗2 = |Ψ〉₁₂₃₄〈Ψ|, where $|{\rm{\Psi }}{\rangle }_{1234}={\sum }_{j,k=0}^{n-1}{\sum }_{l,m=0}^{n-1}\,{a}_{jk}|jk\rangle \otimes {a}_{lm}|lm\rangle ,\,{\sum }_{j,k=0}^{n-1}\,|{a}_{jk}{|}^{2}=1.$ Alice and Bob apply the unitary transformations W and V to their two resource particles respectively. Before the measurement, the initial state becomes $|\varphi {\rangle }_{0}{W}_{13}{V}_{24}|{\rm{\Psi }}{\rangle }_{1234}={\sum }_{j,k=0}^{n-1}{\sum }_{l,m=0}^{n-1}{\sum }_{j^{\prime} ,k^{\prime} }^{n-1}{\sum }_{l^{\prime} ,m^{\prime} }^{n-1}{\sum }_{\nu }^{n-1}{a}_{jk}{a}_{lm}{W}_{j^{\prime} l^{\prime} }^{jl}{V}_{k^{\prime} m^{\prime} }^{km}{\alpha }_{\nu }|\nu j^{\prime} k^{\prime} l^{\prime} m^{\prime} {\rangle }_{01234}$.

  After Alice’s joint Bell measurement based on |Φ_st〉 on particles 0 and 1, we get: ${\langle {{\rm{\Phi }}}_{st}{|}_{01}{(|\varphi \rangle }_{0}{W}_{13}{V}_{24}|{\rm{\Psi }}\rangle }_{1234})={V}_{24}{A}_{2}{A}_{4}{W}_{24}{({U}_{st}^{\dagger })}_{2}|\varphi {\rangle }_{2}|{\rm{\Phi }}{\rangle }_{34}$, where A is the n × n matrix with elements (A)_jk = a_jk. Receiving Alice’s measurement outcomes, correspondingly Bob applies unitary operators {T} on particle 2. The resulting state becomes ${({T}_{st})}_{2}{V}_{24}{A}_{2}{A}_{4}{W}_{24}{({U}_{st}^{\dagger })}_{2}|\varphi {\rangle }_{2}|{\rm{\Phi }}\rangle {}_{34}\mathrm{.}$ Taking partial trace over the spaces with respect to particles 3 and 4, we have

  $${{\rm{\Lambda }}}_{{\chi }^{\otimes 2}}^{\{{T}_{st},W,V\}}({\rho }_{in})=\sum _{s,t}\,\frac{1}{n}{{\rm{tr}}}_{4}{[({T}_{st}{)}_{2}{V}_{24}{A}_{2}{A}_{4}{W}_{24}{({U}_{st})}_{2}^{\dagger }|\varphi \rangle }_{2}\langle \varphi {|}_{2}{({U}_{st})}_{2}{W}_{24}^{\dagger }{A}_{2}^{\dagger }{A}_{4}^{\dagger }{V}_{24}^{\dagger }{({T}_{st})}_{2}^{\dagger }],$$

  (2)

  which is equivalent to Eq. (1).

• 2). Now consider the case of arbitrary entangled mixed resources, ${\chi }^{\otimes 2}={\sum }_{\alpha ,\beta }\,{P}_{\alpha }{P}_{\beta }|{{\rm{\Psi }}}_{\alpha \beta }\rangle \langle {{\rm{\Psi }}}_{\alpha \beta }|,$ where $|{{\rm{\Psi }}}_{\alpha \beta }\rangle ={\sum }_{j,k=0}^{n-1}{\sum }_{l,m=0}^{n-1}\,{a}_{jk}^{(\alpha )}|jk\rangle \otimes {a}_{lm}^{(\beta )}|lm\rangle ,$ 0 ≤ P_α(β) ≤ 1 and ${\sum }_{\alpha (\beta )}{P}_{\alpha (\beta )}=1$. Similar to the derivation of (2), we have

$${{\rm{\Lambda }}}_{{\chi }^{\otimes 2}}^{\{{T}_{st},W,V\}}({\rho }_{in})=\frac{1}{n}\sum _{s,t}\sum _{\alpha ,\beta }\,{P}_{\alpha }{P}_{\beta }{{\rm{tr}}}_{4}{[({T}_{st}{)}_{2}{V}_{24}{A}_{2}^{(\alpha )}{A}_{4}^{(\beta )}{W}_{24}{({U}_{st}^{\dagger })}_{2}|\varphi \rangle }_{2}\langle \varphi {|}_{2}{({U}_{st})}_{2}{W}_{24}^{\dagger }{A}_{2}^{(\alpha )\dagger }{A}_{4}^{(\beta )\dagger }{V}_{24}^{\dagger }{({T}_{st})}_{2}^{\dagger }],$$

where ${(A)}_{jk}^{(\alpha /\beta )}={a}_{jk}^{(\alpha /\beta )}$. Since each matrix A^(α) can be decomposed in the basis of U_st: ${A}^{(\alpha )}={\sum }_{s,t}\,{a}_{st}^{(\alpha )}{U}_{st}$, by using the relation⁷, $n{\sum }_{\alpha }\,{p}_{\alpha }{a}_{st}^{(\alpha )}{a}_{s^{\prime} t^{\prime} }^{(\alpha )\ast }=\langle {{\rm{\Phi }}}_{st}|\chi |{{\rm{\Phi }}}_{s^{\prime} t^{\prime} }\rangle$, we can straightforwardly show that Eq. (1) is also valid for any mixed input state ρ_in.$\square$

Remark

The two-copy teleportation scenario is trace preserving, $tr[{{\rm{\Lambda }}}_{{\chi }^{\otimes 2}}^{\{{T}_{st},W,V\}}({\rho }_{in})]=1$, see proof in Method.

Utilizing the output state, we get the optimal teleportation fidelity (see Method for detailed proof):

Theorem 1

The optimal teleportation fidelity f₂(χ)_max of the two-copy teleportation protocol is given by

$${f}_{2}{(\chi )}_{{\rm{\max }}}=\frac{n{F}_{2}(\chi )}{(n+\mathrm{1)}}+\frac{1}{n+1},$$

(3)

where ${{\rm{\Omega }}}_{13}^{T}={W}_{13}{({U}_{st})}_{1}{({T}_{st})}_{3}$, F₂(χ) is two-copy fully entangled fraction,

$${F}_{2}(\chi )=\mathop{{\rm{\max }}}\limits_{{\rm{\Omega }},V\in U({n}^{2})}\{{\langle {\rm{\Phi }}|}_{12}{{\rm{tr}}}_{34}[{{\rm{\Omega }}}_{13}{V}_{24}{\chi }_{12}{\chi }_{34}{{\rm{\Omega }}}_{13}^{\dagger }{V}_{24}^{\dagger }]{|{\rm{\Phi }}\rangle }_{12}\mathrm{\}.}$$

From Theorem 1 we see that the two-copy optimal teleportation fidelity f₂(χ) solely depends on the two-copy fully entangled fraction F₂(χ). It can be shown that F₂(χ) given by (3) is an invariant under local unitary transformations: ${\chi }_{12}{\chi }_{34}\to {({\mathfrak{U}})}_{13}{({\mathfrak{V}})}_{24}{\chi }_{12}{\chi }_{34}{({\mathfrak{U}})}_{13}^{\dagger }{({\mathfrak{V}})}_{24}^{\dagger }$, where ${\mathfrak{U}}$ and ${\mathfrak{V}}$ are unitary operators on H ⊗ H. Theorem 1 also tells us that a resource state χ is useful, namely, it gives better teleportation fidelity than classical channels, if ${F}_{2}(\chi ) > \frac{1}{n}$.

The original one copy optimal teleportation fidelity is given by^7,8

$${f}_{1}{(\chi )}_{{\rm{\max }}}=\frac{n{F}_{1}(\chi )}{n+1}+\frac{1}{n+1},$$

(4)

where ${F}_{1}(\chi )={{\rm{\max }}}_{U\in U(n)}\{{\langle {\rm{\Phi }}{|}_{12}{U}_{2}^{\dagger }{\chi }_{12}{U}_{2}|{\rm{\Phi }}\rangle }_{12}\}$ is the original fully entangled fraction. To show that the two-copy teleportation protocol is always better, or at least as good as the original one copy case, let us simply choose the unitary matrix ${W}_{13}={V}_{24}={I}_{{n}^{2}\times {n}^{2}}$ in Theorem 1, which does not necessarily reach the value of f₂(χ)_max.

Lemma 2

When choose the unitary matrix ${W}_{13}={V}_{24}={I}_{{n}^{2}\times {n}^{2}}$ in Theorem 1, the output state ${{\rm{\Lambda }}}_{{\chi }^{\otimes 2}}^{\{{T}_{st},W,V\}}({\rho }_{in})$ of the two-copy teleportation protocol reduces to the output state Λ^(χ)({T})(ρ) of one-copy teleportation protocol^7,8 (see Method).

Theorem 2

The two-copy optimal teleportation fidelity is always greater than or equal to that of the original one copy protocol, that is, for any arbitrary state χ,

$${f}_{2}{(\chi )}_{max}\ge {f}_{1}{(\chi )}_{max}\mathrm{.}$$

(5)

Proof.

From Eqs (3) and (4), one can see that both optimal teleportation fidelities for two-copy and onecopy teleportation protocols are linear functions of the corresponding fully entangled fractions. These fully entangled fractions characterize the usefulness of the entangled resource states in quantum teleportation. To compare f₂(χ)_max with f₁(χ)_max, one only needs to compare F₂(χ) with F₁(χ). Unfortunately, both F₂(χ) and F₁(χ) are formidably difficult to calculate analytically. Analytical formulae for F₁(χ) are only available for some special states^20,21. Generally one has only estimations of the upper and lower bounds of F₁(χ)^21,22. The computation of F₂(χ) is much more difficult than that of F₁(χ). However, if one takes W = V to be identity, or takes Ω and V in (3) to be the tensor of two unitary operators Υ ⊗ Γ with Υ, Γ ∈ U(n), then one gets F₂(χ) = F₁(χ). Thus the extreme value range of F₂ is larger than that of F₁. Therefore, for any arbitrary state χ, f₂(χ)_max ≥ f₁(χ)_max, i.e., the two-copy optimal teleportation fidelity is always greater than or equal to that of the original one copy protocol.$\square$

In the following, we give numerical calculations of F₂ and F₁ by using the Conjugate Gradient Algorithm^23,24. Following the modified Polak-Ribiere-Polyak method introduced in²⁴, we can get the numerical result of F₁.

Lemma 3

To simplify the computation, we take V = I_n×n to get a lower bound F′₂(χ) of F₂(χ):

$${F^{\prime} }_{2}(\chi )=\mathop{{\rm{\max }}}\limits_{{\rm{\Omega }}\in U({n}^{2})}\{\sum _{j}\,{\langle {\rm{\Phi }}{|}_{12}{\langle j{|}_{3}{{\rm{\Omega }}}_{23}{\chi }_{12}{{\rm{\Omega }}}_{23}^{\dagger }{\rho }_{3}^{\ast }|j\rangle }_{3}|{\rm{\Phi }}\rangle }_{12}\},$$

where ρ₃ = tr₄(χ₃₄); ${\rho }_{3}^{\ast }$ is the conjugate of ρ₃, see Method.

Denote ${{\mathfrak{F}}}_{2}(\chi )={\sum }_{j}\,{\langle {\rm{\Phi }}{|}_{12}{\langle j{|}_{3}{{\rm{\Omega }}}_{23}{\chi }_{12}{{\rm{\Omega }}}_{23}^{\dagger }{\rho }_{3}^{\ast }|j\rangle }_{3}|{\rm{\Phi }}\rangle }_{12}$. We get ${F^{\prime} }_{2}(\chi )={{\rm{\max }}}_{{{\rm{\Omega }}}_{23}\in U({n}^{2})}{{\mathfrak{F}}}_{2}$. Set ΔF = F′₂(χ) − F₁(χ). Figure 2 shows that for these randomly generated states, one has F′₂(χ) > F₁(χ), i.e., the lower bound of the optimal fidelity of two-copy teleportation is better than the optimal fidelity of the one-copy teleportation.

Figure 2

Hollow (solid) triangles stand for 3 (4)-dimensional randomly generated states. Horizontal axis is the one-copy fully entangled fraction F₁. Vertical axis denotes the difference ΔF = F′₂ − F₁. It is seen that ΔF > 0, and hence the lower bound of the optimal fidelity of the two-copy teleportation is better than the optimal fidelity of original one-copy teleportation for all these randomly generated states.

Let us investigate further the lower bound F′₂(χ) of the two-copy fully entangled fraction F₂(χ), obtained by setting V = I_n×n in the two-copy teleportation protocol. For the original one copy teleportation, it is shown that the resource states χ satisfying F₁(χ) > 1/n are useful for teleportation¹⁷. Since the set of states satisfying ${F}_{1}(\chi )\le \frac{1}{n}$ is convex and compact, there exist witness operators that detect entangled states which are useful for teleportation^17,18. Similarly, for the two-copy teleportation protocol with V = I_n×n, if resource states satisfying ${{F}^{{\rm{^{\prime} }}}}_{2}(\chi ) > \frac{1}{n}$, they are useful for teleportation. Denote ${\mathbb{S}}=\{\chi :{F^{\prime} }_{2}(\chi )\le \frac{1}{n}\}$. We have:

Theorem 3

The set ${\mathbb{S}}$ is convex and compact.

Proof.

• 1). The set ${\mathbb{S}}$ is convex: let χ_a and χ_b ∈ ${\mathbb{S}}$, namely, ${F^{\prime} }_{2}({\chi }_{a})\le \frac{1}{n}$, ${F^{\prime} }_{2}({\chi }_{b})\le \frac{1}{n}$. Consider χ_c = ξχ_a + (1 − ξ)χ_b, where ξ ∈ [0, 1]. By the definition of ${F^{\prime} }_{2}(\chi )={{\rm{\max }}}_{{{\rm{\Omega }}}_{23}\in U({n}^{2})}\{{\sum }_{j}{\langle {{\rm{\Phi }}}_{00}{|}_{12}{\langle j{|}_{3}{{\rm{\Omega }}}_{23}{\chi }_{12}{{\rm{\Omega }}}_{23}^{\dagger }{\rho }_{3}^{\ast }|j\rangle }_{3}|{{\rm{\Phi }}}_{00}\rangle }_{12}\}$, we get that ${F^{\prime} }_{2}({\chi }_{c})\le \xi {F^{\prime} }_{2}({\chi }_{a})+\mathrm{(1}-\xi ){F^{\prime} }_{2}({\chi }_{b})\le \frac{1}{n}\mathrm{.}$ Thus χ_c ∈ ${\mathbb{S}}$, i.e. ${\mathbb{S}}$ is convex.

• 2). The set ${\mathbb{S}}$ is compact: for finite dimensional Hilbert spaces, to show that a set is compact, it is enough to show the set is closed and bounded. ${\mathbb{S}}$ is bounded since the eigenvalues of χ lies in [0, 1]¹⁷. To see that it is closed, assume that for any two density matrices χ_a and χ_b, the value of F′₂(χ_a + χ_b) and F′₂(χ_a) are obtained at Ω_a+b and Ω_a respectively, where Ω ∈ U(n²). Therefore

$${F^{\prime} }_{2}({\chi }_{a}+{\chi }_{b})-{F^{\prime} }_{2}({\chi }_{a})\le {n}^{2}||{{\rm{\Omega }}}_{a+b}{||}^{2}\mathrm{(2||}{\chi }_{a}||+||{\chi }_{b}||)||{\chi }_{b}||,$$

(6)

where ||χ_a|| is the maximal eigenvalue of χ_a satisfying ||χ_a|| ≤ 1. Since the set of all unitary operators is bounded, ||Ω_{a + b}||² ≤ v, where v is a positive real number. Thus F′₂(χ_a + χ_b) − F′₂(χ_a) ≤ n²v(2 + ||χ_b||)||χ_b||.$\square$

Remark

For the two-copy teleportation protocol with V = I_n×n, there exist witnesses to identify the usefulness of an unknown resource state experimentally.

In fact, according to the Hahn-Banach theorem²⁵, any χ $\notin \,{\mathbb{S}}$ can be separated from ${\mathbb{S}}$ by a hyperplane. This feature enables for the existence of hermitian witness operators and thus experimental ways to detect the usefulness of an unknown resource state.$\square$

Discussion

To conclude, we have proposed a general two-copy quantum teleportation protocol based on Bell measurement systematically. The corresponding optimal teleportation fidelity have been analytically derived. Interestingly, the formulae of optimal teleportation fidelity in two-copy scenario and one-copy scenario are similar. Both of them are the one-variable linear function of the corresponding fully entangled fractions, which are invariants under local unitary transformations on the resource states. Analytical analysis together with numerical results illustrate that the optimal teleportation fidelity can be improved in two-copy teleportation protocol when compared with the onecopy scenario. Therefore, in order to improve the teleportation fidelity, two-copy teleportation protocol is significant both theoretically and experimentally. Furthermore, we have shown that in the context of two-copy teleportation protocol, if one considers a specific case that Bob conducts identity transformation on his resource states, the optimal fidelity can still be improved. The set of quantum states with their two-copy fully entangled fraction bounded by a threshold value required for useful two-copy teleportation is convex and compact. It demonstrates the existence of two-copy teleportation witness, completing the theory of two-copy quantum teleportation. Besides, this two-copy quantum teleportation protocol can be generalized to many-copy cases and may result in further improvement on the teleportation fidelity. Different protocols and methods are worthwhile to conceive and investigate in the future.

Methods

Proof of Remark

The output state ${{\rm{\Lambda }}}_{{\chi }^{\otimes 2}}^{\{{T}_{st},W,V\}}$ of the two-copy teleportation is trace preserving:

$$\begin{array}{rcl}{\rm{tr}}[{{\rm{\Lambda }}}_{{\chi }^{\otimes 2}}^{\{{T}_{st},W,V\}}({\rho }_{in})] & = & \frac{1}{{n}^{3}}\sum _{{s}_{1},{t}_{1}}\sum _{{s^{\prime} }_{1}{t^{\prime} }_{1}}\sum _{{s}_{2},{t}_{2}}\sum _{{s^{\prime} }_{2}{t^{\prime} }_{2}}\langle {{\rm{\Phi }}}_{{s}_{1}{t}_{1}}|\chi |{{\rm{\Phi }}}_{{s^{\prime} }_{1}{t^{\prime} }_{1}}\rangle \\ & & \times \,\langle {{\rm{\Phi }}}_{{s}_{2}{t}_{2}}|\chi |{{\rm{\Phi }}}_{{s^{\prime} }_{2}{t^{\prime} }_{2}}\rangle {{\rm{tr}}}_{2}\{({T}_{st}{)}_{2}{({U}_{{s}_{1}{t}_{1}})}_{2}{{\rm{tr}}}_{4}[({U}_{{s}_{2}{t}_{2}}{)}_{4}{W}_{24}{W}_{24}^{\dagger }{({U}_{{s^{\prime} }_{2}{t^{\prime} }_{2}})}_{4}^{\dagger }]({U}_{{s^{\prime} }_{1}{t^{\prime} }_{1}}^{\dagger }{)}_{2}{({T}_{st})}_{2}^{\dagger }\}\\ & = & \frac{1}{{n}^{2}}\sum _{{s}_{1},{t}_{1}}\sum _{{s^{\prime} }_{1}{t^{\prime} }_{1}}\sum _{{s}_{2},{t}_{2}}\sum _{{s^{\prime} }_{2}{t^{\prime} }_{2}}\langle {{\rm{\Phi }}}_{{s}_{1}{t}_{1}}|\chi |{{\rm{\Phi }}}_{{s^{\prime} }_{1}{t^{\prime} }_{1}}\rangle \\ & & \langle {{\rm{\Phi }}}_{{s}_{2}{t}_{2}}|\chi |{{\rm{\Phi }}}_{{s^{\prime} }_{2}{t^{\prime} }_{2}}\rangle t{r}_{2}[({U}_{{s}_{1}{t}_{1}}{)}_{2}{({U}_{{s^{\prime} }_{1}{t^{\prime} }_{1}}^{\dagger })}_{2}]{{\rm{tr}}}_{4}[({U}_{{s}_{2}{t}_{2}}{)}_{4}{({U}_{{s^{\prime} }_{2}{t^{\prime} }_{2}})}_{4}^{\dagger }]=\mathrm{1,}\end{array}$$

where in the first equality we have used the relation ${\sum }_{s,t}\,{U}_{st}^{\dagger }A{U}_{st}=n{\rm{tr}}(A){I}_{n\times n}$ for any n × n matrix A.$\square$

Proof of Theorem 1

Let U(n) be an irreducible n-dimensional representation of unitary group G. By using the Schur’s lemma

$${\int }_{G}dg({U}^{\dagger }(g)\otimes {U}^{\dagger }(g))\sigma (U(g)\otimes U(g))={\alpha }_{1}I\otimes I+{\alpha }_{2}P,$$

$${\alpha }_{1}=\frac{{n}^{2}{\rm{tr}}(\sigma )-n{\rm{tr}}(\sigma P)}{{n}^{2}({n}^{2}-\mathrm{1)}},\,{\alpha }_{2}=\frac{{n}^{2}{\rm{tr}}(\sigma P)-n{\rm{tr}}(\sigma )}{{n}^{2}({n}^{2}-\mathrm{1)}},$$

where σ is any operator acting on the tensor space, P is the flip operator, dg is the Haar measure on G normalized by ${\int }_{G}dg=1$, we get the fidelity of the two-copy teleportation protocol,

$$\begin{array}{rcl}{f}_{2}(\chi ) & = & \overline{\langle {\varphi }_{in}|{{\rm{\Lambda }}}_{{\chi }^{\otimes 2}}^{\{{T}_{st},W,V\}}({\rho }_{in})|{\varphi }_{in}\rangle }\\ & = & \frac{1}{{n}^{3}}\sum _{{s}_{1},{t}_{1}}\sum _{{s^{\prime} }_{1},{t^{\prime} }_{1}}\sum _{{s}_{2},{t}_{2}}\sum _{{s^{\prime} }_{2},{t^{\prime} }_{2}}\langle {{\rm{\Phi }}}_{{s}_{1}{t}_{1}}|\chi |{{\rm{\Phi }}}_{{s^{\prime} }_{1}{t^{\prime} }_{1}}\rangle \langle {{\rm{\Phi }}}_{{s}_{2}{t}_{2}}|\chi |{{\rm{\Phi }}}_{{s^{\prime} }_{2}{t^{\prime} }_{2}}\rangle \\ & & \times \,\sum _{s,t,j,k}\langle \mathrm{00|}{\int }_{G}[U{(g)}^{\dagger }\otimes U{(g)}^{\dagger }][\langle j{|}_{4}\\ & & \times \,{({T}_{st})}_{2}{V}_{24}{({U}_{{s}_{1}{t}_{1}})}_{2}{({U}_{{s}_{2}{t}_{2}})}_{4}{W}_{24}{({U}_{st})}_{2}^{\dagger }|k{\rangle }_{4}]\\ & & \otimes \,[\langle k{|}_{4}{({U}_{st})}_{2}{W}_{24}^{\dagger }{({U}_{{s^{\prime} }_{1}{t^{\prime} }_{1}}^{\dagger })}_{2}{({U}_{{s^{\prime} }_{2}{t^{\prime} }_{2}}^{\dagger })}_{4}{V}_{24}^{\dagger }\\ & & \times \,{({T}_{st})}_{2}^{\dagger }|j{\rangle }_{4}][U(g)\otimes U(g)]dg\mathrm{|00}\rangle \\ & = & \frac{1}{{n}^{4}(n+\mathrm{1)}}\sum _{{s}_{1},{t}_{1}}\sum _{{s^{\prime} }_{1},{t^{\prime} }_{1}}\sum _{{s}_{2},{t}_{2}}\sum _{{s^{\prime} }_{2},{t^{\prime} }_{2}}\langle {{\rm{\Phi }}}_{{s}_{1}{t}_{1}}|\chi |{{\rm{\Phi }}}_{{s^{\prime} }_{1}{t^{\prime} }_{1}}\rangle \langle {{\rm{\Phi }}}_{{s}_{2}{t}_{2}}|\chi |{{\rm{\Phi }}}_{{s^{\prime} }_{2}{t^{\prime} }_{2}}\rangle \\ & & \times \,\sum _{s,t,j,k,l,l^{\prime} }\{{{\rm{tr}}}_{2}[{\langle j{|}_{4}{({T}_{st})}_{2}{V}_{24}{({U}_{{s}_{1}{t}_{1}})}_{2}{({U}_{{s}_{2}{t}_{2}})}_{4}|l\rangle }_{4}\langle l{|}_{4}\\ & & \times \,{W}_{24}{({U}_{st})}_{2}^{\dagger }|k{\rangle }_{4}]{{\rm{tr}}}_{4}[\langle k{|}_{4}{({U}_{st})}_{2}{W}_{24}^{\dagger }\\ & & \times \,{({U}_{{s^{\prime} }_{1}{t^{\prime} }_{1}}^{\dagger })}_{2}{({U}_{{s^{\prime} }_{2}{t^{\prime} }_{2}})}_{4}^{\dagger }|l^{\prime} {\rangle }_{4}{\langle l^{\prime} {|}_{4}{V}_{24}^{\dagger }{({T}_{st})}_{2}^{\dagger }|j\rangle }_{4}]\\ & & +\,\frac{1}{{n}^{2}(n+\mathrm{1)}}\sum _{{s}_{1},{t}_{1}}\sum _{{s^{\prime} }_{1},{t^{\prime} }_{1}}\sum _{{s}_{2},{t}_{2}}\sum _{{s^{\prime} }_{2},{t^{\prime} }_{2}}\langle {{\rm{\Phi }}}_{{s}_{1}{t}_{1}}|\chi |{{\rm{\Phi }}}_{{s^{\prime} }_{1}{t^{\prime} }_{1}}\rangle \langle {{\rm{\Phi }}}_{{s}_{2}{t}_{2}}|\chi |{{\rm{\Phi }}}_{{s^{\prime} }_{2}{t^{\prime} }_{2}}\rangle \\ & & \times \,{{\rm{tr}}}_{2}[({U}_{{s}_{1}{t}_{1}}{)}_{2}{({U}_{{s^{\prime} }_{1}{t^{\prime} }_{1}}^{\dagger })}_{2}]{{\rm{tr}}}_{4}[({U}_{{s}_{2}{t}_{2}}{)}_{4}{({U}_{{s^{\prime} }_{2}{t^{\prime} }_{2}})}_{4}^{\dagger }]\\ & = & \frac{1}{{n}^{4}(n+\mathrm{1)}}\sum _{{s}_{1},{t}_{1}}\sum _{{s^{\prime} }_{1},{t^{\prime} }_{1}}\sum _{{s}_{2},{t}_{2}}\sum _{{s^{\prime} }_{2},{t^{\prime} }_{2}}\sum _{s,t,j,k}{\langle {\rm{\Phi }}|}_{12}{\langle {\rm{\Phi }}|}_{34}{{\rm{tr}}}_{24}{[{W}_{24}{({U}_{st})}_{2}^{\dagger }|k\rangle }_{4}\\ & & \times \,\langle j{|}_{4}{({T}_{st})}_{2}{V}_{24}{({U}_{{s}_{1}{t}_{1}})}_{2}{({U}_{{s}_{2}{t}_{2}})}_{4}]({U}_{{s}_{1}{t}_{1}}^{\dagger }{)}_{2}{({U}_{{s}_{2}{t}_{2}}^{\dagger })}_{4}{\chi }_{12}{\chi }_{34}\\ & & \times \,{{\rm{tr}}}_{24}{[{V}_{24}^{\dagger }{({T}_{st})}_{2}^{\dagger }|j\rangle }_{4}\langle k{|}_{4}{({U}_{st})}_{2}{W}_{24}^{\dagger }{({U}_{{s^{\prime} }_{1}{t^{\prime} }_{1}}^{\dagger })}_{2}{({U}_{{s^{\prime} }_{2}{t^{\prime} }_{2}})}_{4}^{\dagger }]\\ & & \times \,{({U}_{{s^{\prime} }_{1}{t^{\prime} }_{1}})}_{2}{({U}_{{s^{\prime} }_{2}{t^{\prime} }_{2}})}_{4}{|{\rm{\Phi }}\rangle }_{12}{|{\rm{\Phi }}\rangle }_{34}+\frac{1}{n+1}\\ & = & \frac{1}{(n+\mathrm{1)}}\sum _{s,t}{\langle {\rm{\Phi }}|}_{12}{\langle {\rm{\Phi }}|}_{34}{W}_{24}{({U}_{st})}_{2}^{\dagger }{({T}_{st})}_{2}\\ & & \times \,{{\rm{tr}}}_{4}[{V}_{24}{\chi }_{12}{\chi }_{34}{V}_{24}^{\dagger }]({T}_{st}{)}_{2}^{\dagger }{({U}_{st})}_{2}{W}_{24}^{\dagger }){|{\rm{\Phi }}\rangle }_{12}{|{\rm{\Phi }}\rangle }_{34}+\frac{1}{n+1},\end{array}$$

where $\overline{\langle {\varphi }_{in}|\mathrm{...}|{\varphi }_{in}\rangle }$ represents the average over all input states $|{\varphi }_{in}\rangle$.

Then the optimal teleportation fidelity is given by the maximal fidelity of f₂(χ),

$$\begin{array}{rcl}{f}_{2}{(\chi )}_{max} & = & \frac{{n}^{2}}{(n+\mathrm{1)}}\mathop{{\rm{\max }}}\limits_{{\rm{\Omega }},V\in U({n}^{2})}\{{\langle {\rm{\Phi }}|}_{12}{\langle {\rm{\Phi }}|}_{34}{{\rm{\Omega }}}_{24}{{\rm{tr}}}_{4}[{V}_{24}{\chi }_{12}{\chi }_{34}{V}_{24}^{\dagger }]{{\rm{\Omega }}}_{24}^{\dagger }{|{\rm{\Phi }}\rangle }_{12}{|{\rm{\Phi }}\rangle }_{34}\}+\frac{1}{n+1}\\ & = & \frac{{n}^{2}}{(n+\mathrm{1)}}\mathop{{\rm{\max }}}\limits_{{\rm{\Omega }},V\in U({n}^{2})}\{{\langle {\rm{\Phi }}|}_{12}{\langle {\rm{\Phi }}|}_{34}{{\rm{\Omega }}}_{13}^{T}{{\rm{tr}}}_{4}[{V}_{24}{\chi }_{12}{\chi }_{34}{V}_{24}^{\dagger }]{{\rm{\Omega }}}_{13}^{\ast }{|{\rm{\Phi }}\rangle }_{12}{|{\rm{\Phi }}\rangle }_{34}\}+\frac{1}{n+1},\end{array}$$

where Ω₂₄ = W₂₄(U_st)₂(T_st)₂. Rewriting Ω^T as Ω, we get (3).$\square$

Proof of Lemma 2

When we choose ${W}_{13}={V}_{24}={I}_{{n}^{2}\times {n}^{2}}$, the output state ${{\rm{\Lambda }}}_{{\chi }^{\otimes 2}}^{\{{T}_{st},W,V\}}({\rho }_{in})$ of the two-copy teleportation protocol reduces to that of one-copy teleportation protocol Λ^(χ)({T})(ρ) in⁷:

$$\begin{array}{c}{{\rm{\Lambda }}}_{{\chi }^{\otimes }2}^{\{{T}_{st},W,V\}}({\rho }_{in})\\ \begin{array}{rcl} & = & \frac{1}{{n}^{3}}\sum _{{s}_{1},{t}_{1}}\sum _{{s^{\prime} }_{1}{t^{\prime} }_{1}}\sum _{{s}_{2},{t}_{2}}\sum _{{s^{\prime} }_{2}{t^{\prime} }_{2}}\sum _{s,t}\,\langle {{\rm{\Phi }}}_{{s}_{1}{t}_{1}}|\chi |{{\rm{\Phi }}}_{{s^{\prime} }_{1}{t^{\prime} }_{1}}\rangle \langle {{\rm{\Phi }}}_{{s}_{2}{t}_{2}}|\chi |{{\rm{\Phi }}}_{{s^{\prime} }_{2}{t^{\prime} }_{2}}\rangle {{\rm{tr}}}_{4}[({T}_{st}{)}_{2}{({U}_{{s}_{1}{t}_{1}})}_{2}\\ & & \times \,{({U}_{{s}_{2}{t}_{2}})}_{4}{({U}_{st})}_{2}^{\dagger }|\varphi {\rangle }_{2}\langle \varphi {|}_{2}{({U}_{st})}_{2}{({U}_{{s^{\prime} }_{1}{t^{\prime} }_{1}}^{\dagger })}_{2}{({U}_{{s^{\prime} }_{2}{t^{\prime} }_{2}})}_{4}^{\dagger }{({T}_{st})}_{2}^{\dagger }]\\ & = & {{\rm{\Lambda }}}^{(\chi )}(\{T\})({\rho }_{in})\frac{1}{n}\sum _{{s}_{2},{t}_{2}}\sum _{{s^{\prime} }_{2},{t^{\prime} }_{2}}\,\langle {{\rm{\Phi }}}_{{s}_{2}{t}_{2}}|\chi |{{\rm{\Phi }}}_{{s^{\prime} }_{2}{t^{\prime} }_{2}}\rangle {\rm{tr}}[{U}_{{s}_{2}{t}_{2}}{U}_{{s^{\prime} }_{2}{t^{\prime} }_{2}}^{\dagger }]={{\rm{\Lambda }}}^{(\chi )}(\{T\})({\rho }_{in}\mathrm{).}\end{array}\end{array}$$

Proof of Lemma 3

$$\begin{array}{ccc}{{F}^{{\rm{^{\prime} }}}}_{2}(\chi ) & = & n\mathop{max}\limits_{{\rm{\Omega }}\in U({n}^{2})}\{{\langle {\rm{\Phi }}|}_{12}{\langle {\rm{\Phi }}|}_{34}{{\rm{\Omega }}}_{24}{\chi }_{12}{\rho }_{3}{{\rm{\Omega }}}_{24}^{\dagger }{|{\rm{\Phi }}\rangle }_{12}{|{\rm{\Phi }}\rangle }_{34}\}\\ & = & \mathop{max}\limits_{{\rm{\Omega }}\in U({n}^{2})}\{\sum _{j,{j}^{{\rm{^{\prime} }}}}\,{\langle {\rm{\Phi }}|}_{12}{\langle j|}_{4}{{\rm{\Omega }}}_{24}{\chi }_{12}{{\rm{\Omega }}}_{24}^{\dagger }{|{j}^{{\rm{^{\prime} }}}\rangle }_{4}{|{\rm{\Phi }}\rangle }_{12}{\langle j|}_{3}{\rho }_{3}{|{j}^{{\rm{^{\prime} }}}\rangle }_{3}\}\\ & = & \mathop{max}\limits_{{\rm{\Omega }}\in U({n}^{2})}{\{\sum _{j}{\langle {\rm{\Phi }}|}_{12}{\langle j|}_{3}{{\rm{\Omega }}}_{23}{\chi }_{12}{{\rm{\Omega }}}_{23}^{\dagger }{\rho }_{3}^{\ast }|j\rangle }_{3}{|{\rm{\Phi }}\rangle }_{12}\},\end{array}$$

where ρ₃ = tr₄(χ₃₄).

Numerical calculation for Fig. 2

Denote ${{\mathfrak{F}}}_{1}(\chi )={\langle {\rm{\Phi }}|}_{12}{U}_{2}^{\dagger }{\chi }_{12}{U}_{2}{|{\rm{\Phi }}\rangle }_{12}$. Since unitary U can be expressed as U = exp{i*ℍ}, where ℍ is the corresponding Hermitian matrix, we can get the increment of ${{\mathfrak{F}}}_{1}$

$$\begin{array}{ccc}{\rm{\Delta }}{{\mathfrak{F}}}_{1} & = & \frac{i}{2}[{\langle {\rm{\Phi }}|}_{12}({\rm{\Delta }}{{\mathbb{H}}}_{2}{U}_{2}+{U}_{2}{\rm{\Delta }}{{\mathbb{H}}}_{2}){\chi }_{12}{U}_{2}^{\dagger }{|{\rm{\Phi }}\rangle }_{12}\\ & & -\,{\langle {\rm{\Phi }}|}_{12}{U}_{2}{\chi }_{12}({\rm{\Delta }}{{\mathbb{H}}}_{2}{U}_{2}^{\dagger }+{U}_{2}^{\dagger }{\rm{\Delta }}{{\mathbb{H}}}_{2}){|{\rm{\Phi }}\rangle }_{12}]\\ & = & \frac{i}{2}{{\rm{t}}{\rm{r}}}_{2}\{{\rm{\Delta }}{{\mathbb{H}}}_{2}[{{\rm{t}}{\rm{r}}}_{1}{({U}_{2}{\chi }_{12}{U}_{2}^{\dagger }|{\rm{\Phi }}\rangle }_{12}{\langle {\rm{\Phi }}|}_{12})\\ & & +\,{{\rm{t}}{\rm{r}}}_{1}{({\chi }_{12}{U}_{2}^{\dagger }|{\rm{\Phi }}\rangle }_{12}{\langle {\rm{\Phi }}|}_{12}{U}_{2})\\ & & -\,{{\rm{t}}{\rm{r}}}_{1}{({U}_{2}^{\dagger }|{\rm{\Phi }}\rangle }_{12}{\langle {\rm{\Phi }}|}_{12}{U}_{2}{\chi }_{12})\\ & & -\,{{\rm{t}}{\rm{r}}}_{1}{(|{\rm{\Phi }}\rangle }_{12}{\langle {\rm{\Phi }}|}_{12}{U}_{2}{\chi }_{12}{U}_{2}^{\dagger })]\}\\ & = & \frac{i}{2}{{\rm{t}}{\rm{r}}}_{2}[{\rm{\Delta }}{{\mathbb{H}}}_{2}{G}_{2}({{\mathfrak{F}}}_{1})].\end{array}$$

Choosing ${G}_{2}({{\mathfrak{F}}}_{1})$ as the gradient of ${{\mathfrak{F}}}_{1}$, then following the MRPR Method introduced from Eq. (2.1) to Eq. (2.4) in²⁴, we can get the numerical result of F₁.

Using the same method, we can obtain the increment of ${{\mathfrak{F}}^{\prime} }_{2}(\chi )$:

$$\begin{array}{rcl}{\rm{\Delta }}{{\mathfrak{F}}^{\prime} }_{2} & = & \frac{i}{2}{{\rm{tr}}}_{23}\{{\rm{\Delta }}{{\mathbb{H}}}_{23}[{{\rm{tr}}}_{1}({{\rm{\Omega }}}_{23}{\chi }_{12}{{\rm{\Omega }}}_{23}^{\dagger }{\rho }_{3}^{\ast }{|{\rm{\Phi }}\rangle }_{12}{\langle {\rm{\Phi }}|}_{12})+{{\rm{tr}}}_{1}{({\chi }_{12}{{\rm{\Omega }}}_{23}^{\dagger }{\rho }_{3}^{\ast }|{\rm{\Phi }}\rangle }_{12}{\langle {\rm{\Phi }}|}_{12}{{\rm{\Omega }}}_{23})\\ & & -\,{{\rm{tr}}}_{1}({{\rm{\Omega }}}_{23}^{\dagger }{\rho }_{3}^{\ast }{|{\rm{\Phi }}\rangle }_{12}{\langle {\rm{\Phi }}|}_{12}{{\rm{\Omega }}}_{23}{\chi }_{12})-{{\rm{tr}}}_{1}({\rho }_{3}^{\ast }{|{\rm{\Phi }}\rangle }_{12}{\langle {\rm{\Phi }}|}_{12}{{\rm{\Omega }}}_{23}{\chi }_{12}{{\rm{\Omega }}}_{23}^{\dagger })]\}\\ & = & \frac{i}{2}{{\rm{tr}}}_{23}[{\rm{\Delta }}{{\mathbb{H}}}_{23}{G}_{23}({{\mathfrak{F}}^{\prime} }_{2}\mathrm{)].}\end{array}$$

Thus we can get a numerical lower bound of F′₂. We generate random 3d and 4d states by Mathematica and let ΔF = F′₂ − F₁, then can get into the result of Fig. 2.

Detailed proof of Theorem 3

$$\begin{array}{c}{{F}^{{\rm{^{\prime} }}}}_{2}({\chi }_{a}+{\chi }_{b})-{{F}^{{\rm{^{\prime} }}}}_{2}({\chi }_{a})\\ \begin{array}{ccc} & \le & \sum _{j}\,{\langle {{\rm{\Phi }}}_{00}|}_{12}{\langle j{|}_{3}{({{\rm{\Omega }}}_{a+b})}_{23}{({\chi }_{a})}_{12}{({{\rm{\Omega }}}_{a+b})}_{23}^{\dagger }{{\rm{t}}{\rm{r}}}_{4}{[({\chi }_{b}{)}_{34}]}^{\ast }|j\rangle }_{3}{|{{\rm{\Phi }}}_{00}\rangle }_{12}\\ & & +\,\sum _{j}\,{\langle {{\rm{\Phi }}}_{00}|}_{12}{\langle j{|}_{3}{({{\rm{\Omega }}}_{a+b})}_{23}{({\chi }_{b})}_{12}{({{\rm{\Omega }}}_{a+b})}_{23}^{\dagger }{{\rm{t}}{\rm{r}}}_{4}{[({\chi }_{a}{)}_{34}]}^{\ast }|j\rangle }_{3}{|{{\rm{\Phi }}}_{00}\rangle }_{12}\\ & & +\,\sum _{j}\,{\langle {{\rm{\Phi }}}_{00}|}_{12}{\langle j{|}_{3}{({{\rm{\Omega }}}_{a+b})}_{23}{({\chi }_{b})}_{12}{({{\rm{\Omega }}}_{a+b})}_{23}^{\dagger }{{\rm{t}}{\rm{r}}}_{4}{[({\chi }_{{\rm{b}}}{)}_{34}]}^{\ast }|{\rm{j}}\rangle }_{3}{|{{\rm{\Phi }}}_{00}\rangle }_{12}\\ & \le & \sum _{j,{j}^{{\rm{^{\prime} }}}}||{{\rm{\Phi }}}_{00}\rangle {||}^{2}||j{j}^{{\rm{^{\prime} }}}\rangle {||}^{2}||{{\rm{\Omega }}}_{a+b}{||}^{2}(2||{\chi }_{a}||+||{\chi }_{b}||)||{\chi }_{b}||\\ & = & {n}^{2}||{{\rm{\Omega }}}_{a+b}{||}^{2}(2||{\chi }_{a}||+||{\chi }_{b}||)||{\chi }_{b}||.\end{array}\end{array}$$