Introduction

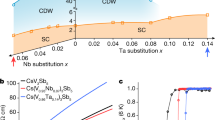
The kagome lattice, made of corner-shared triangles, is an exciting platform for emergent quantum phenomena1,2,3. Due to the wavefunction interference, the electronic structure of the kagome lattice features flat band, Dirac fermion, and van Hove singularities that result in a rich interplay between topology, geometry, and correlations4,5. For kagome metals near the van Hove singularities, the high density of states combining with the frustrated lattice geometry are predicted to support novel electronic orders6,7,8. Recently, in a topological kagome metal CsV3Sb5, superconductivity that possibly intertwines with charge density wave (CDW)9,10,11,12 (Fig. 1a), nematicity13,14,15,16 and loop current17,18 is observed. To date, the origin of superconductivity and its interplay with the other symmetry-breaking orders remain rigorous debate. Angle-resolved photoemission spectroscopy (ARPES) studies19,20 observed multiple van Hove singularities from V 3d-electrons near the Fermi level (EF), highlighting electronic driven instabilities6,8 (Fig. 1b). Furthermore, the density functional theory (DFT) calculated EPC strength21, λ~0.25, in CsV3Sb5 fails to support the superconducting transition temperature9, Tc~2.6 K, indicating unconventional pairing mechanism. However, a recent ARPES study of a cousin compound KV3Sb5 revealed a clear kink22 in the electronic band structure near the van Hove singularity, suggesting a moderate EPC. Therefore, an experimental estimation of orbital- and momentum-dependent λ and its possible connection with superconductivity are highly desired to understand the nature of the superconductivity in CsV3Sb5. Here we experimentally extract the orbital- and momentum-dependent λp,d(k) by determining the EPC-induced kinks in the electronic band structure. Our results reveal an intermediate EPC with λ=0.45–0.6 in CsV3Sb5, which can support a Tc on the same magnitude of the experimental value. Intriguingly, we find that λd is enhanced by about 50% in the isovalent-substituted Cs(V0.93Nb0.07)3Sb5 with an elevated Tc = 4.4 K. Our results suggest that EPC can play an important role on the superconductivity in CsV3Sb5.

Fig. 1: EPC-induced electronic kink in CsV3Sb5.
figure 1

a Schematic temperature versus pressure/doping phase diagram. The inset shows the crystal structure of CsV3Sb5. A top view of the V-Sb layer is zoomed in on the right panel. b Schematic of van Hove singularities at the M point of the Brillouin zone boundary which are connected by three nesting wavevectors. The von Hove singularities has mainly V 3d orbital characters (\({d}_{{x}^{2}-{y}^{2}}\) and \({d}_{{yz}}\)). The circular electronic pocket at Γ point has mainly Sb 5pz orbital character. c FS mapping with intensity integrated within EF ± 5 meV. The FS sheet in ky < 0 is symmetrized from the one in ky > 0 and a superposed FS sheet is collected from another independent sample. Dashed lines are DFT determined FSs. d ARPES intensity plot, corresponding to the black cut in c, showing a kink in the band dispersion. The band dispersion extracted from the MDCs is overlaid as a red curve. The dashed black line represents the bare band. The arrow indicates the position of the kink. The MDC at EF and its Lorentzian fit are shown as yellow and black lines, respectively. e Real-part self-energy ReΣ(ω=E-EF) and imaginary-part self-energy ImΣ(ω=E-EF). A background of ImΣother is subtracted for ImΣ(ω) (see supplementary note 2). The dashed black line marks the energy position of the kink.

Full size image

Results

Figure 1a, c shows the crystal structure and Fermi surface (FS) topology of CsV3Sb5, respectively. In agreement with previous DFT and ARPES studies12,19,20,21, the Sb 5p-band forms a circular FS, marked as α, at the BZ center and the V 3d bands yield hexagonal and triangle FSs, marked as β and δ in Fig. 1c, respectively. Figure 1d shows a typical ARPES intensity plot of the α band corresponding to the black cut shown in Fig. 1c. The coupling between electrons and bosonic modes is manifested by the intensity and dispersion anomalies, known as kink23,24, near a binding energy EB~32 meV. This many-body effect can be quantified by fitting the ARPES momentum distribution curves (MDCs) with a Lorentzian function25:

$$I({{{{{\boldsymbol{k}}}}}},\, \omega ) \propto A ({{{{{\boldsymbol{k}}}}}},\, \omega )=\frac{1}{\pi } \frac{{{{{{\rm{Im}}}}}} \Sigma (\omega )} {{(\omega -\varepsilon ({{{{{\boldsymbol{k}}}}}}) -{{{{{\rm{Re}}}}}} \Sigma (\omega ))}^{2}+{{{{{\rm{Im}}}}}} \Sigma {(\omega )}^{2}},$$
(1)

where ReΣ(ω = E-EF) and ImΣ(ω = E-EF) are the real and imaginary parts of the single-particle self-energy. ε(k) is the non-interacting bare band that can be approximated as a liner dispersion crossing EF23. Figure 1e demonstrates the extracted self-energy of the α band. We subtract a linear bare band from the experimentally extracted band to obtain ReΣ(ω) (see supplementary note 1). To extract the electron–boson coupling induced ImΣ(ω), the electron-electron and electron-impurity scatterings induced self-energy effects are removed, as suggested by previous practices23,26 (see supplementary note 2). At EB~32 meV, a peak near in ReΣ(ω) and a step jump in ImΣ(ω) prove strong many-body interactions. Since the self-energy anomalies persist above CDW transition temperature TCDW (supplementary Fig. S7), we attribute the self-energy anomaly to EPC.

Figure 2a–c compares the EPC-induced kinks on the α and β bands at 6 K. The ARPES intensity plots of the α and β bands shown in Fig. 2b correspond to the black cuts in Fig. 2a. While the kink near EB~32 meV is clear on both the α and β bands, an additional kink is observed at a lower EB~12 meV on the β band (Fig. 2c). The 12-meV kink is also prominent in ReΣ(ω). As we show in Fig. 2d, ReΣ(ω) of the β band shows a peak near EB~12 meV, proving strong d-electron–phonon coupling near 12 meV. In contrast, ReΣ(ω) of the α band only shows a broad shoulder.

Fig. 2: Orbital dependent EPC.
figure 2

a Contours of the FSs and the momentum location of the cuts shown in (b). b ARPES intensity plots the α and β bands. The momentum is rescaled with respect to their kF. c Extracted band dispersions of the \(\alpha\) and \(\beta\) bands. The arrows show the energy position of the kinks. The black lines are the corresponding bare bands. d Extracted ReΣ(ω) of the α and β bands. The error bars for ReΣ(ω) are determined from standard deviation of the MDC fits, which is converted to energy by multiplying velocity of bare band. The black lines are the ReΣ(ω) reproduced by maximum entropy method. e Extracted Eliashberg coupling functions \({\alpha }^{2}F\left(\omega \right)\) for the α and β bands. f λp,d estimated from \({\alpha }^{2}F\left(\omega \right)\) (purple circles) and λdev defined by the slope of ReΣ(ω) at EF (black circles). The error bars are determined by the standard deviation of the ReΣ(ω).

Full size image

The observation of clear EPC effects on both 5p and 3d bands points to a non-neglectable role of EPC for superconductivity in CsV3Sb5. To test the EPC-driven superconductivity, we extract the Eliashberg function, \({\alpha }^{2}F\left(\omega \right)\), at T = 6 K, slightly above Tc, using the maximum entropy method27,28 (see methods). A fit of the ReΣ(ω) and the extracted \({\alpha }^{2}F\left(\omega \right)\) are shown in Fig. 2d, e, respectively. λ and the logarithmic mean phonon frequency are obtained via28,29:

$$\lambda=2{\int }_{0}^{{\omega }_{\max }}\left[\frac{{\alpha }^{2}F\left(\omega \right)}{\omega }\right]{{{{{\rm{d}}}}}}\omega,$$
(2)
$${{{{{\rm{ln}}}}}}{\omega }_{\log}=2/\lambda {\int }_{0}^{\infty }{{{{{\rm{ln}}}}}}\omega \left[\frac{{\alpha }^{2}F(\omega )}{\omega }\right]{{{{{\rm{d}}}}}}\omega \left]\right.,$$
(3)

where \({\omega }_{\max }\) is the maximum frequency of the phonon spectrum. As shown in Fig. 2e, the orbital dependence of the EPC is mirrored in the different shapes of α2F(ω), where phonon modes near 32 meV are accounted for 70% of the total EPC strength on the α band, λp, but less than 50% for the EPC strength on the β band, λd. Interestingly, due to the spectral weight redistribution in \({\alpha }^{2}F\left(\omega \right)\) (shaded area in Fig. 2e), the extracted λp and λd are similar with λp,d~0.45 ± 0.05. We also employed the MEM fits the extracted ImΣ(ω), which yields a λ cοnsistent with the ReΣ(ω) fits (see supplementary note 4 and Fig. S2). Theoretically, \(\lambda\) can approximately be derived from a simpler approach29 following \({\lambda }_{{{{{{{\rm{dev}}}}}}}}={-\partial {{{{\mathrm{Re}}}}}\Sigma (\omega )/\partial \omega|}_{\omega={E}_{{{{{{\rm{F}}}}}}}}\cong \lambda\), when T is far lower than the Debye temperature. At T = 6 K, this method yields a \({\lambda }_{{{{{{{\rm{dev}}}}}}}}\)~0.6±0.1, qualitatively consistent with Eq. (2) within the experimental uncertainty (Fig. 3f).

Fig. 3: Momentum dependence of the electronic kink.
figure 3

a, b Representative ARPES intensity plots for the α and β bands as marked in c, respectively. d, e Extracted band dispersions for the representative α and β bands, respectively. The dashed black lines are bare bands. The red purple arrows indicate the 32-meV kink. These extracted dispersions are offset horizontally for a better view. f EPC strength λdev defined by the slope of ReΣ(ω) at EF plotted with FS angle \(\phi\)FS as the hollow markers. The solid markers are mirrored from the hollow makers for a better clarity. The square markers are the data collected from another independent sample (supplementary Fig. S4). The error bar for the λdev is determined by the standard deviation of the ReΣ(ω). The dashed yellow line represents the average value of λdev. The definition of the FS angle \(\phi\)FS is shown in c.

Full size image

Generally, EPC can exhibit momentum dependence. Figure 3 summarizes the momentum-dependent kinks on the α and β bands. The ARPES intensity plots and extracted band dispersions along representative directions are shown in Fig. 3a, b and Fig. 3d, e, respectively (see supplementary Fig. S3 for complete dataset). The extracted \({\lambda }_{{{{{{{\rm{dev}}}}}}}}({{{{{\boldsymbol{k}}}}}})\) for the α and β bands of two independent samples (supplementary Fig. S4) are summarized in Fig. 3f, which shows a nearly isotropic behavior within experimental uncertainties.

The orbital- and momentum-dependent results demonstrate that the EPC strength λ in CsV3Sb5 falls in the intermediate range of 0.45–0.6, which is about 2 times larger than the previous DFT predicted \({\lambda }_{{{{{{{\rm{DFT}}}}}}}}\)~0.25 (ref. 21). Using McMillan’s formula30 and taking the lower and upper limits of the experimentally estimated \(\lambda\) and the logarithmic mean phonon frequency ~17.1 meV obtained from Eq. (3), we derive Tc in a range from 0.8 K to 3 K (see supplementary note 6). The upper limit is comparable to the experimentally determined Tc in CsV3Sb5 (Fig. 4a). We shall note that the CDW gap near the M point20,31 (supplementary Fig. S6f) flattens the δ bands near EF, hindering the precise estimation of EPC strength. However, strong self-energy anomalies are observed on the δ bands and they have the same energy scales as the α and β bands (supplementary Fig. S6).

Fig. 4: Orbital and energy selective enhancement of EPC in Cs(V0.93Nb0.07)3Sb5.
figure 4

a Temperature dependence of magnetic susceptibility for pristine CsV3Sb5 and Cs(V0.93Nb0.07)3Sb5. Both zero-field cooling (ZFC) and field cooling (FC) curves are presented. b ARPES intensity plots of the α and β bands along the Γ−Κ direction for Cs(V0.93Nb0.07)3Sb5. The false color is adjusted for better visualization. c Extracted band dispersions for the α and β bands shown in (b). The black lines are the corresponding bare bands. d Extracted ReΣ(ω) for the α (top panel) and β (bottom panel) bands. The error bars for ReΣ(ω) are determined from the standard deviation of the MDC fits, which is converted to energy by multiplying the velocity of the bare band. The ReΣ(ω) of pristine CsV3Sb5 is plotted as colored shadows for a direct comparison. The solid black curves are the reproduced ReΣ(ω) via maximum entropy method. The dashed blue and gray line represents the slope of the ReΣ(ω) at EF in Cs(V0.93Nb0.07)3Sb5 and CsV3Sb5, respectively. e Extracted Eliashberg function α2F(ω). f EPC strength λ estimated from the α2F(ω), which is plotted as a function of Tc. The error bar for λ is determined by the standard deviation of the ReΣ(ω).

Full size image

As shown in Figs. 4a and 1a, Tc of CsV3Sb5 is increased with chemical substitutions or external pressure32,33,34,35. We thus continue to examine the EPC in a 7% Nb-doped Cs(V0.93Nb0.07)3Sb5 with Tc~4.4 K34. Electronic Kinks are observed on both the α and β bands as shown in Fig. 4b for the ARPES intensity plots and Fig. 4c for the extracted band dispersions. Figure 4d shows the extracted ReΣ(ω) on the α and β bands of Cs(V0.93Nb0.07)3Sb5. The shaded area corresponds to the ReΣ(ω) of the pristine CsV3Sb5. Remarkably, we observe that while ReΣ(ω) on the α band is similar in Cs(V0.93Nb0.07)3Sb5 and CsV3Sb5, on the β band, it shows a strong enhancement in the Nb-doped sample, especially near EB~10 meV. Based on the extracted α2F(ω), shown in Fig. 4e, we find that λd~0.75 ± 0.05 is enhanced by about 50% in Cs(V0.93Nb0.07)3Sb5 (Fig. 4f). Such giant enhancement is also manifested by the slope of ReΣ(ω) near EF (Fig. 4d). Consequently, the enhanced λd in Cs(V0.93Nb0.07)3Sb5 is expected to elevate Tc up to 4.5 K (see supplementary note 6), which is comparable to the experimental value of 4.4 K (Fig. 4a). Such synchronous enhancements of λd and Tc may indicate that the V 3d-electron–phonon couplings are the main driver of the superconductivity in CsV3Sb5.

Finally, we discuss the influences of CDW order on the quantitative extraction of λ at T < TCDW. The formation of a CDW gap will modify the bare band to deviate from a linear dispersion near EF. As we show in the supplementary Fig. S5, within the experimental resolution, we do not observe a CDW gap on the α and β bands. Therefore, for the α and β bands, the CDW modified bare band dispersion below TCDW is \(\sqrt{{{{\varepsilon }}}_{0}^{2}\left(k\right)+{\Delta }_{{{{{{\rm{CDW}}}}}}}^{2}}\,\cong \,{{{\varepsilon }}}_{0}(k)\), where \({{{\varepsilon }}}_{0}(k)={v}_{0}{{\hslash }}k\) is the linear bare band dispersion above TCDW. In this case, the linear bare band assumption used in our study is a good approximation. Indeed, the excellent agreement of ReΣ(ω) and ImΣ(ω) linked by Kramers-Kronig transformation23,26 validates the linear bare band assumption for the α and β bands (supplementary Figs. S1c-d). The linear bare band assumption, however, does not apply to the δ band that forms a CDW gap comparable to the kink energy20,31. We also note that the formation of CDW will also modify the electronic self-energy. As we show in the supplementary Fig. S7e, \({\lambda }_{{dev}}\) shows an inflection point at TCDW, which may suggest an enhanced EPC strength below TCDW. However, it can also be a consequence of the CDW-corrected electronic self-energy effect (see supplementary note 8).

In summary, by investigating the electronic kinks, we determined an intermediate EPC that is twice larger than the DFT calculated value in the kagome superconductor CsV3Sb5 and Cs(V0.93Nb0.07)3Sb5. Our results provide an important clue to understand the pairing mechanism in CsV3Sb5. The orbital, momentum of electronic kinks and their strengthening with the promoted Tc prove that the EPC in CsV3Sb5 is strong enough to support a Tc comparable to the experiment value and hence cannot be excluded as a possible pairing mechanism. While the exact microscopic pairing mechanism calls for further scrutiny, it is important to point out that the EPC-driven superconductivity is not incompatible with the recently observed pair-density wave (PDW) in CsV3Sb517. Indeed, PDW has been observed in another conventional superconductor NbSe2, where the pair-density modulation is due to the real space charge density modulations36. We also note that the EPC-driven superconductivity can coexist with the time-reversal symmetry-breaking (TRSB) orders or fluctuations18,37,38, as proposed by theoretical studies39,40,41. In those cases, the superconducting order parameter is expected to intertwine with the TRSB order parameter, which gives rise to an unconventional ground state.

Methods

Growth and characterization of single crystals

Single crystals of CsV3Sb5 were grown using CsSb2 alloy and Sb as flux. Cs, V, Sb elements and CsSb2 precursor were sealed in a Ta crucible in a molar ratio of 1:3:14:10, which was finally sealed in a highly evacuated quartz tube. The tube was heated up to 1273 K, maintained for 20 hours and then cooled down to 763 K slowly. Single crystals were separated from the flux by centrifuging. The single crystals of Cs(V0.93Nb0.07)3Sb5 were provided by Jinggong New Materials (Yangzhong) Co., Ltd. The growth and characterizations of Cs(V0.93Nb0.07)3Sb5 were present in ref. 34. The magnetic susceptibility was measured under magnetic field 20 Oe for CsV3Sb5 and 5 Oe for Cs(V0.93Nb0.07)3Sb5.

Laser-ARPES measurements

ARPES measurements were performed for the freshly cleaving surface with a Scienta-Omicron R4000 hemispherical analyzer with an ultraviolet laser (hν = 6.994 eV) at the Institute for Solid State Physics, the University of Tokyo42. The energy resolution was set to be 1.3 meV. The sample temperature was set to be 6 K if there is no special announcement. The samples were cleaved in situ and kept under a vacuum better than 3 × 10−11 torr during the experiments.

Maximum entropy method

The Eliashberg function \({\alpha }^{2}F\left(\omega ;\epsilon,\, {{{{{\boldsymbol{k}}}}}}\right)\) is related to the real part of the self-energy by the integration function

$${{{{{\rm{Re}}}}}}\Sigma \left(\epsilon,\, {{{{{\boldsymbol{k}}}}}}{{{{{\rm{;}}}}}} \, T\right)={\int }_{0}^{{{\infty }}}{{{{{\rm{d}}}}}}\omega \, {\alpha }^{2}F\left(\omega ; \epsilon,\, {{{{{\boldsymbol{k}}}}}}\right)K\left(\frac{\epsilon }{{k}_{B}T},\frac{\omega }{{k}_{B}T}\right),$$
(4)

where \(K\left(y,\, {y}^{{\prime} }\right)={\int }_{-\infty }^{\infty }{{{{{{\rm{d}}}}}}x}\frac{f\left(x-y\right)2{y}^{{\prime} }}{{x}^{2}-{y}^{2}}\) and \(f\left(x\right)\) is the Fermi distribution function. It is an ill-posed problem to obtain the Eliashberg function from Eq. (4). In this work, we adopted the maximum entropy method (MEM)27,28, which is frequently used to perform the analytic continuation43. By considering the energy resolution of the laser-ARPES, we estimated that the error bar of the real part of the self-energy was 1 meV. MEM requires a model default function to define the entropic prior. Here, we adopted the following model:

$$m\left(\omega \right)=\left\{\begin{array}{cc}{m}_{0}{\left(\frac{\omega }{{\omega }_{D}}\right)}^{2} & \omega \le {\omega }_{D}\\ {m}_{0} & {\omega }_{D}\le \omega \le {\omega }_{m}\\ 0 & \omega \, > \, {\omega }_{m}\end{array}\right.,$$
(5)

where \({m}_{0}\) = 15 meV, \({\omega }_{D}\) = 10 meV, and \({\omega }_{m}\) = 80 meV. This default model was also used in the previous study of the electron–phonon coupling on the Be surface28.