Introduction

In the last decade different material platforms have been proposed for the establishment of topological superconductivity. Among those are the semiconductor/superconductor1, the topological insulator/superconductor2, and the magnet/superconductor3,4,5,6,7 platforms. While the first two platforms require a magnetic field for the stabilization of a topological superconducting phase, which is difficult to reconcile with miniaturization requirements of microelectronics, this issue is circumvented in the latter platform, the MSHs, where the presence of a magnet within the system can provide the required time-reversal symmetry breaking. So far, attention has been mainly focused on using ferromagnetic (FM) components in 1D and 2D MSHs8,9,10,11,12,13,14, which are understood as gapped topological superconductors described by a non-zero Chern number15,16. However, hybrid systems with AFM order have not yet been considered. Another class of topological superconductivity, the gapless TNPSC phase, has been discussed extensively17,18,19,20,21,22,23,24,25,26, and recently its observation was reported for the transition metal dichalcogenide TaS227, in the form of low energy modes at step edges. However, for MSHs no evidence of TNPSC has been reported so far.

In this manuscript, we report the discovery of TNPSC in a 2D superconducting system with AFM order. We first introduce the theoretical description of such 2D TNPSC and then present its experimental realization in an AFM monolayer on top of an s-wave superconductor. The observed topological phase is established through the interplay of the pairing interaction of the substrate, the antiferromagnetic order of the magnetic monolayer, and the spin-orbit coupling (SOC) at the interface, which makes our results very general and not limited to a specific material system (see Supplementary Note 3 for a discussion on generality).

Results and discussion

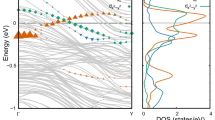
To investigate the properties of an AFM-MSH, we model a single layer of antiferromagnetically ordered magnetic adatoms with the symmetry of the (110) surface of a body-centered cubic (bcc) crystal. In such an AFM monolayer three different types of straight edges can form, as shown in Fig. 1a, which we name ferromagnetic (FM), zigzag (ZZ), and antiferromagnetic (AFM) edge in the following (the colors of the magnetic atoms in Fig. 1a indicate their spin orientations). We describe the electronic structure using a tight-binding model which reflects the presence of s-wave superconductivity, a Rashba spin-orbit interaction, and an AFM order of the magnetic moments, which interact with the electronic degrees of freedom via a magnetic exchange coupling4 (see “Methods” section for additional details). The resulting superconducting band structure of the system, shown in Fig. 1b, exhibits 8 nodal points (NP) located on the boundary of the magnetic Brillouin zone (BZ). The curved arrow at each NP indicates its topological charge, with anticlockwise arrows for winding number of +1 and clockwise arrows for −118,28 (see Supplementary Note 2 for details). Pairs of cones from neighboring NPs are connected via a van-Hove point, which we refer to as β modes (see Supplementary Note 1 for more details).

Fig. 1: Electronic properties of an AFM-based MSH.
figure 1

a Real space structure of an antiferromagnetically ordered mono-atomic layer with the symmetry of the (110) surface of a body-centered cubic crystal. b Superconducting quasiparticle dispersion. The dispersion shows nodal points on the boundary of the magnetic Brillouin zone, shown as a black rectangle (the structural Brillouin zone is shown as dashed lines). The winding number associated with each nodal point is indicated by the curved arrows (+1 red, −1 blue). c–e Spectral function for each edge type and f–h corresponding LDOS at (c, f) a FM edge, (d, g) a ZZ edge, and (e, h) an AFM edge. In addition to the edge modes, the spectral functions have contributions from the 2D bulk electronic structure, which originate from the projected bulk electronic band structure shown in b onto the respective momentum axes, as indicated by the colored lines in b (see Supplementary Fig. 2 for individual projections). A dispersive edge band connects the projected nodal points at the FM edge and a flat edge band for the ZZ edge (yellow arrows), resulting in strong low-energy peaks in the LDOS (black arrows). For the AFM edge, a state corresponding to the van-Hove singularity connecting two neighboring cones appears and is indicated by red arrows. Parameters for the model are given in the methods section.

Full size image

To model the three different types of edges, we consider semi-infinite systems29 and present the corresponding edge spectral functions in Fig. 1c–e as a function of energy and momentum. The location of the bulk NPs, projected onto the momenta parallel to the different edges, are indicated by colored spheres. In addition to the bulk states, the FM and ZZ edges exhibit low energy modes that connect two NPs with opposite topological charge, which we refer to as α modes. In the local density of states (LDOS) for the FM and ZZ edges, shown in Fig. 1f, g, these α modes give rise to strong low-energy peaks., We note that the weak dispersion of the α mode at the FM edge results in a splitting of the low-energy peak. Moreover, the projection of the electronic structure onto the FM edge, combined with the emergence of trivial edge states near the bulk gap, leads to a splitting of the superconducting coherence peaks. In contrast, at the AFM edge, no low energy mode is present, as shown in Fig. 1e, h, while only a peak arising from the β mode is visible in the LDOS. Although β is a bulk mode, it is much less prominent in the FM and ZZ edge. These distinct and qualitatively different features in the LDOS between the FM and ZZ edges on one hand, and the AFM edge on the other, are a characteristic and unique signature of the topological electronic structure of the system.

An experimental system with an AFM layer on a bcc(110) superconductor, as used in the model for AFM-TNPSC, is realized by a pseudomorphic Mn monolayer on Nb(110), which was found to exhibit c(2 × 2) AFM order30. As seen in Fig. 2a, Mn forms islands that are elongated along [001] directions. In addition to long [001] edges, island edges along [1–10] and [1–11] are observed, which are the different edge types discussed in Fig. 1, namely the FM, the ZZ, and the AFM edge, respectively (see Fig. 2b).

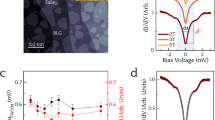
Fig. 2: Local tunneling spectroscopy on a Mn/Nb(110) island.
figure 2

a Topography image of a single Mn island with well-developed edges along the main crystallographic directions. b Schematics of the atomic and spin structure of a FM, ZZ, and AFM edge, respectively. c Tunneling point spectra acquired with a superconducting Nb-coated Cr tip at the positions indicated in panel a. d Deconvoluted LDOS obtained from the spectra displayed in panel c. α and β mark the states corresponding to calculated dispersive edge modes shown in Fig. 1. Tunneling parameters: a tunneling current It = 1 nA, bias U = 20 mV; c stabilization tunneling current Its = 1 nA, stabilization bias Ustab = 20 mV, modulation bias Umod = 100 µV, vertical tip shift towards the sample’s surface after stabilization Zapproach = 80 pm.

Full size image

Fig. 2c shows point spectra of the differential tunneling conductance (dI/dU) measured with a superconducting Nb-coated Cr tip at the positions indicated in Fig. 2a. Spectra taken on the clean Nb(110) (blue) show two coherence peaks at ±2.4 mV. With a Nb gap of 1.5 meV the tip gap amounts to Δtip = 0.9 meV. To be able to directly compare the experimental data with the calculated LDOS from our model in Fig. 1, we deconvolute all spectra and display them in Fig. 2d (see Methods section). This results in an LDOS for a clean Nb surface which has symmetric coherence peaks at ±1.5 meV validating the deconvolution procedure. We attribute the non-zero intensity within the superconducting gap of the bare Nb LDOS to our tip, which is not a bulk superconductor. The deconvoluted LDOS obtained in the middle of the Mn island (green) exhibits a prominent peak at 0.8 meV, which we ascribe to the β mode, while its negative energy counterpart (β−) has a very low intensity. This resembles the characteristic LDOS for the Mn monolayer we reported earlier30.

The LDOS of the three edges differ significantly: while the LDOS at the FM and the ZZ edges exhibits pronounced low-energy peaks, the LDOS at the AFM edge possesses only a peak that is located near the β+ peak of the Mn monolayer. These features are in good agreement with our theoretical results shown in Fig. 1f–h obtained for infinitely long edges and can thus be considered as characteristic spectroscopic signatures of the AFM-TNPSC state. There are, however, some small differences between the experimental and theoretical results. First, for the FM edge, for example, our theoretical results reveal a peak split symmetrically around the Fermi energy, α+ and α-, whereas the experimental LDOS (Fig. 2d) exhibits one broad peak in the middle of the gap. However, a closer inspection of the experimental raw data (Fig. 2c) indeed reveals the presence of two features close to Fermi level: one split between positive and negative side of the tip gap and one at around 1.2 mV; these are not as obvious after the deconvolution procedure. In addition, the theory predicts a splitting of the coherence peaks at the FM edge, whereas in the experimental data there is only one clear peak on either side of the gap. We propose that the intensity of some of the expected features is too small to resolve them. Second, at the ZZ edge, our theoretical results show a peak centered at zero energy, while the experimental observed peak in the LDOS is located at 90 µeV. Whether these small differences arise from the finite size of the experimentally investigated island (in contrast to the infinitely long edges considered theoretically) is presently unclear.

To investigate the spatial distribution of the spectroscopic fingerprints of our AFM-TNPSC we choose a Mn island with a particularly long FM edge, as shown in Fig. 3a. The insets show a spin-resolved constant-current image and a corresponding sketch of the antiferromagnetic order. Fig. 3b shows dI/dU maps related to α± and β±. We find that the β± state possesses the largest intensity in the interior of the Mn island, as expected for a bulk state, and in agreement with the spectra displayed in Fig. 2. In contrast, the α± state possesses its largest intensity along the FM edge, exhibits a weaker intensity along the ZZ edge, and is essentially absent along the AFM edge, as expected from the dI/dU spectra shown in Fig. 2. We note that the α± state also exhibits a weak spatially oscillating intensity in the interior of the island, which we attribute to confinement effects. These spatial intensity maps clearly reveal the edge and bulk character of the α± and β± states, respectively. Our experimental findings are in very good agreement with the theoretically computed maps shown in Fig. 3c, which was calculated for the same island shape and size as the experimental one, providing further support for the existence of the AFM-TNPSC state.

Fig. 3: Spatial dependence of tunneling spectroscopic features.
figure 3

a A topography image of a defect-free Mn island. The insets show an SP-STM image of part of the island and a sketch of the c(2 × 2) antiferromagnetic state. The green rectangle indicates the sample area of panel b. b Differential tunneling conductance maps acquired with a superconducting Nb-coated Cr tip at characteristic energies as indicated for α± and β±. c Tight-binding model calculated maps for the same spectroscopic features as in panel b. d Spectra along the line marked with a green arrow in panel a crossing one of the FM edges. e Deconvoluted LDOS obtained from the data in panel d. f Intensity of the zero-bias peak as a function of the distance from the edge. The green and orange curves are exponential decay fits to experimental data towards the Mn and Nb, respectively. The error bars represent measurement noise level. The inset shows a semi-log plot for experimental data and fits. g Spectra along the line marked with a blue arrow in panel a along one of the ferromagnetic edges. h Deconvoluted LDOS from panel g. Through the panels d–f the 0 on the x-axis is set to the half-height of the Mn island in the topography profile along the green arrow. Tunneling parameters: a It = 1 nA, U = 50 mV; b Its = 1 nA, Ustab = 20 mV, Umod = 100 µV, Zapproach = 80 pm, for α± U = ± 1.05 mV, for β± U = ± 1.9 mV; d Its = 1 nA, Ustab = 20 mV, Umod = 100 µV, Zapproach = 100 pm; g Its = 1 nA, Ustab = 20 mV, Umod = 100 µV, Zapproach = 100 pm.

Full size image

The localization of the α± state at the FM edge is also clearly visible in the dI/dU spectra taken along the green arrow in Fig. 3a, presented as a waterfall plot in Fig. 3d. Inside the Mn island the β+ state is dominant, while the α± state possesses its largest spectral weight only at the island’s edge. To quantify the localization we deconvolute the spectra, see Fig. 3e, and plot the resulting zero-bias intensity as a function of distance to the edge in Fig. 3f. The observed intensity decays exponentially on both sides of the edge, with a decay length of 1.5 nm towards the interior of the island, and of 1.0 nm on the Nb surface. The theoretically computed spatial dependence of the zero-bias intensity near the edge shows a very similar behavior, albeit with additional short-period oscillations on the Mn side.

Due to the finite size of the island, we also observe a spatial modulation of the intensity of the α± state along the edges. To visualize this modulation, spectra were taken along the straight bottom FM edge between the positions of structural imperfections, see blue arrow in Fig. 3a, and presented as waterfall plot in Fig. 3g. These plots reveal that the intensities of the α− or α+ branches are out-of-phase, exhibiting spatially alternating maxima. The corresponding deconvoluted data in Fig. 3h shows how the spectral weight of the α± branches shifts across zero-bias along this particular FM edge. The different spatial structure of the α± branches suggest that these branches are located at symmetric, non-zero energies. This, in turn, provides further evidence that the single peak in the experimentally obtained LDOS at the FM edge (see Fig. 2d) actually consists of two unresolved peaks that are located at symmetric energies around Fermi energy, in agreement with the theoretical results shown in Fig. 1f. Even though this modulation of the α± intensity along the FM edge is not captured by the model, the spatial distribution of the edge and bulk states is overall in very good agreement with the characteristic properties of AFM-TNPSC as predicted by the model. Additional measurements are shown in Supplementary Note 4.

In conclusion, we developed a general model of a TNPSC within a 2D-AFM/ s-wave superconductor hybrid system, which is a realization of a time reversal invariant topological superconductor. We experimentally characterized this emergent quantum phase in a real material system, confirming the nodal-point superconducting state by observing the different edge modes as predicted by our model. Due to the rising interest in AFM and superconducting systems, we expect our findings to trigger new experimental and theoretical research in superconducting spintronics and quantum materials.

Methods

Monolayer thick Mn islands were grown on top of an unreconstructed Nb(110) single crystal via physical vapor deposition from a crucible under UHV conditions with a base pressure of ~1.0 × 10−10 mbar following the procedure as described by Lo Conte et al.30. Before the Mn deposition, the Nb(110) substrate was cleaned by a series of 50 sec-long flashes in UHV with base pressure of 1.0 × 10−10 mbar, during which a maximum temperature of about 2400 °C is reached. Samples after growth were in situ transferred to a home-built LT STM system operated at 1.3 K in UHV.

The effective electronic temperature of the tunneling junction was 1.8 K as determined by a BCS fit to a dI/dU spectrum of superconducting Nb(110) acquired with a normal tip. All STM experiments presented in the work were caried out using a Nb-coated superconducting tip. The tip was made by indentation of a bulk Cr tip into clean Nb prior to the experiments. The dI/dU measurements were performed using a lock-in technique by adding a small modulation voltage with a frequency of 4333 Hz to the bias voltage. dI/dU maps were recorded in a constant-height mode after stabilization with the tip parked in the middle of the island. The acquired dI/dU spectra do not directly correspond to the LDOS of the sample, as for the case of normal metallic tips, but rather to the convolution of the density of states of the sample and of the superconducting tip. Accordingly, in order to retrieve the actual LDOS of the sample we perform a numerical deconvolution of the measured dI/dU spectra30,31,32. The tunneling conductance can be expressed as:

$$\frac{{{{{{{\mathrm{d}}}}}}I}}{{{{{{{\mathrm{d}}}}}}U}}\left(U,T\right)\propto {\int }_{-{{\infty }}}^{+{{\infty }}}{\rho }_{{{{{{\rm{S}}}}}}}\left(E\right)\frac{\partial {\rho }_{{{{{{\rm{T}}}}}}}\left(E-{eU}\right)}{\partial U}\left[f\left(E-{eU},T\right)-f\left(E,T\right)\right]{{{{{{\mathrm{d}}}}}}E} \\ +{\int }_{-{{\infty }}}^{+{{\infty }}}{\rho }_{{{{{{\rm{S}}}}}}}\left(E\right){\rho }_{{{{{{\rm{T}}}}}}}\left(E-{eU}\right)\frac{\partial f\left(E-{eU},T\right)}{\partial U}{{{{{{\mathrm{d}}}}}}E}$$
(1)

where ρS(E) is the energy-dependent LDOS of the sample below the tip apex, ρT(E) is the LDOS at the tip apex, f(E,T) is the Fermi-Dirac distribution function, T is the experimental temperature, and U is the applied bias between tip and sample.

We discretize the integral in Eq. (1) into N points in the interval U ϵ [−4, +4] meV. Treating the measured dI/dU spectrum as a column vector [dI/dU] of length N, Eq. (1) can be expressed as a multiplication of an (unknown) sample LDOS vector [ρS] of length N with an N × N matrix A:

$$\left[\frac{{{{{{\rm{d}}}}}}I}{{{{{{\rm{d}}}}}}U}\right]={{{{{\boldsymbol{A}}}}}}\left[{\rho }_{S}\right].$$
(2)

Accordingly, the matrix elements of A are:

$${A}_{{ij}}= \left(\frac{\partial {\rho }_{T}\left({E}_{j}-e{U}_{i}\right)}{\partial U}\left[f\left({E}_{j}-e{U}_{i},T\right)-f\left({E}_{j},T\right)\right] \right. \\ \left.+{\rho }_{T}\left({E}_{j}-e{U}_{i}\right)\frac{\partial f\left({E}_{j}-e{U}_{i},T\right)}{\partial U}\right)\times \delta E,$$
(3)

where Ej are the discrete energy values and Ui the bias voltage values of the acquired tunneling spectrum. Numerically computing a matrix inverse A−1 30,32 and multiplying the result with [dI/dU] yields an approximate sample LDOS:

$$\left[{\rho }_{S}\right] \sim {{{{{{\boldsymbol{A}}}}}}}^{-1}\left[\frac{{{{{{{\mathrm{d}}}}}}I}}{{{{{{{\mathrm{d}}}}}}U}}\right].$$
(4)

The DOS of the superconducting Nb-coated Cr tip is assumed to be a BCS DOS with a phenomenological broadening parameter Γ as discussed by Dynes et al.33:

$${\rho }_{T}(E)={\rho }_{0}Re\left[\frac{E-i\varGamma }{\sqrt{{(E-i\varGamma )}^{2}-{\varDelta }_{T}^{2}}}\right],$$
(5)

where ρ0 is the normal conducting DOS of the tip, and Re indicates the real part.

Parameters used for the deconvolution are as follows: T = 1.8 K, for ρT(E) we assume a superconducting DOS with a ∆T = 0.95 meV for measurements shown in Fig. 2 and ∆T = 1.05 meV for measurements shown in Fig. 3. To account for the thin Nb coating of the Cr tip the Γ parameter is set to 1e–4.

All data has been processed using a self-written python code.

The tight-binding model used is given by

$$H= `{t}_{0}\mathop{\sum}\limits_{\langle {{{{{\bf{r}}}}}},{{{{{{\bf{r}}}}}}}^{{{\hbox{'}}}}\rangle,\sigma }{c}_{{{{{{\bf{r}}}}}}{{{{{\rm{\sigma }}}}}}}^{{{\dagger}} }{c}_{{{{{{{\bf{r}}}}}}}^{{{\hbox{'}}}}{{{{{\rm{\sigma }}}}}}}+{t}_{1}\mathop{\sum}\limits_{\langle \langle {{{{{\bf{r}}}}}},{{{{{{\bf{r}}}}}}}^{{{\hbox{'}}}}\rangle \rangle,{{{{{\rm{\sigma }}}}}}}{c}_{{{{{{\bf{r}}}}}}{{{{{\rm{\sigma }}}}}}}^{{{\dagger}} }{c}_{{{{{{{\bf{r}}}}}}}^{{{\hbox{'}}}}{{{{{\rm{\sigma }}}}}}}+{t}_{2}\mathop{\sum}\limits_{\langle \langle \langle {{{{{\bf{r}}}}}},{{{{{{\bf{r}}}}}}}^{{{\hbox{'}}}}\rangle \rangle \rangle,{{{{{\rm{\sigma }}}}}}}{c}_{{{{{{\bf{r}}}}}}{{{{{\rm{\sigma }}}}}}}^{{{\dagger}} }{c}_{{{{{{{\bf{r}}}}}}}^{{{\hbox{'}}}}{{{{{\rm{\sigma }}}}}}}-\mu \mathop{\sum}\limits_{{{{{{\bf{r}}}}}}}{c}_{{{{{{\bf{r}}}}}}{{{{{\rm{\sigma }}}}}}}^{{{\dagger}} }{c}_{{{{{{\bf{r}}}}}}{{{{{\rm{\sigma }}}}}}}\\ -i\alpha \mathop{\sum}\limits_{\langle {{{{{\bf{r}}}}}},{{{{{{\bf{r}}}}}}}^{{{\hbox{'}}}}\rangle,\langle \langle {{{{{\bf{r}}}}}},{{{{{{\bf{r}}}}}}}^{{{\hbox{'}}}}\rangle \rangle,\alpha,\beta }{c}_{{{{{{\bf{r}}}}}}{{{{{\rm{\alpha }}}}}}}^{{{\dagger}} }{\left({{{{{{\boldsymbol{\sigma }}}}}}}_{\alpha \beta }\times \frac{{{{{{{\bf{r}}}}}}}^{{{\hbox{'}}}}-{{{{{\bf{r}}}}}}}{|{{{{{{\bf{r}}}}}}}^{{{\hbox{'}}}}-{{{{{\bf{r}}}}}}|}\right)}_{z}{c}_{{{{{{\bf{r}}}}}}\beta } \\ + J\mathop{\sum}\limits_{{{{{{\bf{r}}}}}}}{e}^{i{{{{{\bf{Q}}}}}}\cdot {{{{{\bf{r}}}}}}}\left({c}_{{{{{{\bf{r}}}}}}\uparrow }^{{{\dagger}} }{c}_{{{{{{\bf{r}}}}}}\uparrow }-{c}_{{{{{{\bf{r}}}}}}\downarrow }^{{{\dagger}} }{c}_{{{{{{\bf{r}}}}}}\downarrow }\right)+\Delta \mathop{\sum}\limits_{{{{{{\bf{r}}}}}}}\left({c}_{{{{{{\bf{r}}}}}}\uparrow }^{{{\dagger}} }{c}_{{{{{{\bf{r}}}}}}\downarrow }^{{{\dagger}} }+{c}_{{{{{{\bf{r}}}}}}\downarrow }{c}_{{{{{{\bf{r}}}}}}\uparrow }\right),$$
(6)

where t0, t1, t2 are the nearest, next-nearest, and next-next-nearest neighbor hopping parameters, respectively; μ is the chemical potential; α is the Rashba spin-orbit coupling; J is the exchange coupling; and Δ is the superconducting order parameter. The hopping directions are \((\pm \! \frac{a}{2},\pm \! \frac{b}{2})\) for nearest neighbor, (±a, 0) for next-nearest neighbor, and (0, ±b) for next-next-nearest neighbor. The ordering wavevector, Q, is \((\frac{2\pi }{a},0)\) or equivalently \((0,\frac{2\pi }{b})\). The parameters used in the main text are (t0, t1, t2, μ, α, J, Δ) = (1.1, 0.9, 1, 3.5, 0.5, 3.1, 1.5) × 1.7 meV. This set of parameters was chosen to reproduce the observed low-energy edge modes of the experimental data. In particular, the experimental results directly provide insight into the spatial structure of the magnetic order, as well as the magnitude of the superconducting gap. Moreover, the observation of low-energy edge modes along the FM and ZZ edges requires that nodal points are present on both edges of the magnetic Brillouin zone boundary. The absence of a low-energy edge mode along the AFM edge determines the relative position of the nodes as the projected nodal points must (closely) overlap when projected along the AFM edge direction. These observations constrain the ratios of the parameters that determine the normal state dispersion. The overall scale of these parameters relative to the superconducting order parameter is set by the observed localization length of the edge modes.

For an infinite system, Eq. (6) can be expressed in momentum space as

$$H=\mathop{\sum}\limits_{{{{{{\bf{k}}}}}}}{\psi }_{{{{{{\bf{k}}}}}}}^{{{\dagger}} }\{{\eta }_{0}{\tau }_{z}({\varepsilon }_{{{{{{\bf{k}}}}}}}{\sigma }_{0}+{\beta }_{{{{{{\bf{k}}}}}}}{\sigma }_{y})+{\eta }_{x}{\tau }_{z}({f}_{{{{{{\bf{k}}}}}}}{\sigma }_{0}+{{{{{{\boldsymbol{\alpha }}}}}}}_{{{{{{\bf{k}}}}}}}\cdot {{{{{\boldsymbol{\sigma }}}}}})+J{\eta }_{z}{\tau }_{0}{\sigma }_{z}+\Delta {\eta }_{0}{\tau }_{x}{\sigma }_{0}\}{\psi }_{{{{{{\bf{k}}}}}}}$$
(7)
$${\varepsilon }_{{{{{{\bf{k}}}}}}}=2{t}_{1}{{\cos }}\left({k}_{x}a\right)+2{t}_{2}{{\cos }}\left({k}_{y}b\right)-\mu$$
(7.1)
$${f}_{{{{{{\bf{k}}}}}}}=4{t}_{0}{{\cos }}\left(\frac{{k}_{x}a}{2}\right){{\cos }}\left(\frac{{k}_{y}b}{2}\right)$$
(7.2)
$${{{{{{\boldsymbol{\alpha }}}}}}}_{{{{{{\bf{k}}}}}}}=-4\alpha \left(\begin{array}{ccc}-\sqrt{\frac{2}{3}} \, {{\cos }}\left(\frac{{k}_{x}a}{2}\right){{\sin }}\left(\frac{{k}_{y}b}{2}\right),& \sqrt{\frac{1}{3}} \, {{\sin }}\left(\frac{{k}_{x}a}{2}\right){{\cos }}\left(\frac{{k}_{y}b}{2}\right),& 0\end{array}\right)$$
(7.3)
$${\beta }_{{{{{{\bf{k}}}}}}}=-2\alpha \, {{\sin }}\left({k}_{x}a\right)$$
(7.4)

where the spinor is given by

$${\psi }_{{{{{{\bf{k}}}}}}}^{{{\dagger}} }=\left(\begin{array}{cccccccc}{a}_{{{{{{\bf{k}}}}}}\uparrow }^{{{\dagger}} } & {a}_{{{{{{\bf{k}}}}}}\downarrow }^{{{\dagger}} } & {a}_{{{{{{\boldsymbol{-}}}}}}{{{{{\bf{k}}}}}}\downarrow } & {-a}_{{{{{{\boldsymbol{-}}}}}}{{{{{\bf{k}}}}}}\uparrow } & {b}_{{{{{{\bf{k}}}}}}\uparrow }^{{{\dagger}} } & {b}_{{{{{{\bf{k}}}}}}\downarrow }^{{{\dagger}} } & {b}_{{{{{{\boldsymbol{-}}}}}}{{{{{\bf{k}}}}}}\downarrow } & {-b}_{{{{{{\boldsymbol{-}}}}}}{{{{{\bf{k}}}}}}\uparrow }\end{array}\right).$$
(8)

akσ and bkσ are the annihilation operators for A (up moments) and B (down moments) sublattices, respectively; ηa, τa, and σa are Pauli matrices in sublattice, particle-hole, and spin space, respectively.