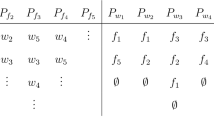Credibility, efficiency, and stability: a theory of dynamic matching markets

Abstract

We introduce a dynamic framework to analyze two-sided matching interactions that occur repeatedly over time, such as teacher–student matching or hospital–intern markets in Britain. We propose a dynamic concept of credible group stability and show that implementing a men-optimal stable matching in each period is credibly group-stable. The result holds for a women-optimal stable matching. A credibly group-stable dynamic matching is individually rational and immune to any defensible group deviations with an appropriate definition of defensibility. We obtain several policy implications for market design. Moreover, a sufficient condition for Pareto efficiency is given for finitely repeated markets.

A. Appendix: Proofs

1.1 A.1 Proposition 1

Consider a two-period dynamic market with $M=\{m_{1},m_{2}\}$ and $W=\{w_{1},w_{2},w_{3}\}$. The preferences are depicted in Fig. 9. In addition, the utility of being unmatched is 0 to each agent. Note that Fig. 9 just indicates the preferences for all agents, but does not show all matchings. There are 11 possible matchings. Denote $\mu _{ij}$ by the matching in which $m_{i}$ is matched with $w_{j}$ and the other agents are unmatched. Denote $\mu _{ij,k\ell }$ by the matching in which $m_{i}$ ($m_{k}$) is matched with $w_{j}$ ($w_{\ell }$) and the other agent is unmatched. Matching $\mu _{U}$ is the one where all agents are unmatched. In total, we have $121=11\times 11$ outcome paths. Out of them, we have 15 individual rational outcome paths: $(\mu _{11},\mu _{11})$, $(\mu _{11},\mu _{21})$, $(\mu _{13},\mu _{11})$, $(\mu _{13},\mu _{13})$, $(\mu _{13},\mu _{21})$, $(\mu _{13},\mu _{U})$, $(\mu _{21},\mu _{13})$, $(\mu _{21},\mu _{U})$, $(\mu _{U},\mu _{13})$, $(\mu _{U},\mu _{21})$, $(\mu _{U},\mu _{U})$; $(\mu _{21},\mu _{21})$, $(\mu _{21},\mu _{22})$, $(\mu _{22},\mu _{22})$,$(\mu _{11,22},\mu _{11,22})$. The first 11 outcome paths are blocked by the pair $(m_{2},w_{2})$ via $(\mu _{22},\mu _{22})$, and the last four are blocked by $(m_{1},w_{3})$ via $(\mu _{13},\mu _{13})$. $\square$

Fig. 9

The preferences in the period market

1.2 A.2 Proposition 4

1.2.1 A.2.1 Part (1)

It is sufficient to show that if a matching $\mu$ is individually rational, then [all blocking pairs are weak] $\Leftrightarrow$ [for each pairwise deviation $(A,{\hat{\mu }})$ from $\mu$, if $u_{i}({\hat{\mu }})>u_{i}(\mu )$ for each $i\in A$, then$(A,{\hat{\mu }})$ is not defensible]. Suppose that $\mu$ is individually rational.

First, we show the direction $(\Rightarrow )$. Suppose that all blocking pairs for $\mu$ are weak. Let $(A,{\hat{\mu }})$ be a pairwise deviation from $\mu$ such that for each $i\in A$, $u_{i}({\hat{\mu }})>u_{i}(\mu )$ (condition *). Without loss of generality, take $m\in A\cap M$. Since $\mu$ is individually rational, $u_{m}({\hat{\mu }})>u_{m}(\mu )\ge u_{m}(m)$. Thus, at ${\hat{\mu }}$, m is matched with some woman w. Thus, by the definition of group deviation, we have $A=\{m,w\}$. Thus, by condition (*), the pair (m, w) blocks $\mu$. Since by our assumption all blocking pairs for $\mu$ are weak, without loss of generality, there is $w^{\prime }\in W$ such that

$$\begin{aligned}&u_{m}(w^{\prime })>u_{m}(w)\text { and} \end{aligned}$$

(2)

$$\begin{aligned}&(m,w^{\prime })\text { is a blocking pair for }\mu . \end{aligned}$$

(3)

Consider a group deviation $(B,{\bar{\mu }})$ from ${\hat{\mu }}$, where $B:=\{m,w^{\prime }\}$ and ${\bar{\mu }}(m)=w^{\prime }$. Note that $A\cap B=\{m\}\ne \emptyset$ and $w^{\prime }\ne w$ by (2). We will show that for each $i\in B$, $u_{i}({\bar{\mu }})>u_{i}({\hat{\mu }})$, which means $(A,{\hat{\mu }})$ is not defensible. By the definition of pairwise deviation, under ${\hat{\mu }}$, $w^{\prime }$ is matched with $\mu (w)$. Then, it follows from (3) that $u_{w^{\prime }}(m)=u_{w^{\prime }}({\bar{\mu }})>u_{w^{\prime }}(\mu )=u_{w}({\hat{\mu }})$. Moreover, it follows from (2) that $u_{m}(w^{\prime })=u_{m}({\bar{\mu }})>u_{m}(w)=u_{m}({\hat{\mu }})$. Thus, the pairwise deviation $(A,{\hat{\mu }})$ is not defensible.

Next we show the other direction $(\Leftarrow )$. Suppose that the hypothesis is true. Let (m, w) be a blocking pair for $\mu$. Then consider the pairwise deviation $(\{m,w\},{\hat{\mu }})$ from $\mu$ with ${\hat{\mu }}(m)=w$. Then $u_{m}({\hat{\mu }})>u_{m}(\mu )$ and $u_{w}({\hat{\mu }})>u_{w}(\mu )$. By our hypothesis, the pairwise deviation is not defensible. Thus there is a group deviation $(B,{\bar{\mu }})$ from ${\hat{\mu }}$ with $\{m,w\}\cap B\ne \emptyset$ such that for each $i\in B$, $u_{i}({\bar{\mu }})>u_{i}({\hat{\mu }})$. Without loss of generality, take m in $\{m,w\}\cap B$. Then,

$$\begin{aligned} u_{m}({\bar{\mu }})>u_{m}({\hat{\mu }})>u_{m}(\mu )\ge u_{m}(m). \end{aligned}$$

(4)

The last inequality follows from individual rationality of $\mu$. By (4), m is matched with some woman $w^{\prime }\in B$ at ${\bar{\mu }}$ with $w^{\prime }\ne w$. Thus $u_{m}({\bar{\mu }})=u_{m}(w^{\prime })>u_{m}(w)\ge u_{m}({\hat{\mu }})$. It remains to show that the pair $(m,w^{\prime })$ is a blocking pair for $\mu$. First, by (4), $u_{m}(w^{\prime })=u_{m}({\bar{\mu }})>u_{m}(\mu )$. Lastly we need to show that $u_{w^{\prime }}(m)>u_{w^{\prime }}(\mu )$. By the definition of pairwise deviation $(\{m,w\},{\hat{\mu }})$, since $w\ne w^{\prime }$, $w^{\prime }$ is matched with $\mu (w^{\prime })$ under ${\hat{\mu }}$. Then, since $w^{\prime }\in B$, $u_{w}(m)=u_{w^{\prime }}({\bar{\mu }})>u_{w^{\prime }}({\hat{\mu }})=u_{w^{\prime }}(\mu )$. Therefore, $(m,w^{\prime })$ blocks $\mu$. $\square$

1.2.2 A.2.2 Part (3)

Consider a static market (Knuth 1996) with $M=\{m_{1},m_{2},$$m_{3},m_{4}\}$, $W=\{w_{1},w_{2},w_{3},w_{4}\}$ and the following preferences:

$m_{1}$	$m_{2}$	$m_{3}$	$m_{4}$	$w_{1}$	$w_{2}$	$w_{3}$	$w_{4}$
$\underline{w_{1}}$	$w_{2}$	$w_{3}$	$\underline{w_{4}}$	$m_{4}$	$\underline{m_{3}}$	$\underline{m_{2}}$	$m_{1}$
$w_{2}$	$w_{1}$	$w_{4}$	$w_{3}$	$m_{3}$	$m_{4}$	$m_{1}$	$m_{2}$
$w_{3}$	$w_{4}$	$w_{1}$	$w_{2}$	$m_{2}$	$m_{1}$	$m_{4}$	$m_{3}$
$w_{4}$	$\underline{w_{3}}$	$\underline{w_{2}}$	$w_{1}$	$\underline{m_{1}}$	$m_{2}$	$m_{3}$	$\underline{m_{4}}$

where each column indicates the preference of an agent in the first row, all mates are acceptable in each column, and an upper mate is preferred to a lower one. Consider the matching

$$\begin{aligned} \mu =\left( \begin{array}{cccc} m_{1} &{} m_{2} &{} m_{3} &{} m_{4}\\ w_{1} &{} w_{3} &{} w_{2} &{} w_{4} \end{array}\right) , \end{aligned}$$

where each of underlined cells in the table indicates his or her partner from this matching. Matching $\mu$ is not stable (for example, pair $(m_{2},w_{1})$ blocks it), but individually rational.

We show that $\mu$ is credibly group-stable. Suppose for a contradiction that there is a defensible group deviation $(A,{\hat{\mu }})$ such that for each $i\in A$, $u_{i}({\hat{\mu }})>u_{i}(\mu )$. Note that A does not contain agents $m_{1}$, $m_{4}$, $w_{2}$ nor $w_{3}$, because each has the best mate in matching $\mu$.

First, consider the case where A is a pair. Then, since A blocks $\mu$, A is $(m_{2},w_{1})$, $(m_{3},w_{1})$, $(m_{2},w_{4})$, or $(m_{3},w_{4})$. Thus, we have the following four cases.

A	$\{m_{2},w_{1}\}$	$\{m_{3},w_{1}\}$
${\hat{\mu }}$	$\left( \begin{array}{ccccc} m_{1} &{} m_{2} &{} m_{3} &{} m_{4} &{} (w_{3})\\ (m_{1}) &{} w_{1} &{} w_{2} &{} w_{4} &{} w_{3} \end{array}\right)$	$\left( \begin{array}{ccccc} m_{1} &{} m_{2} &{} m_{3} &{} m_{4} &{} (w_{2})\\ (m_{1}) &{} w_{3} &{} w_{1} &{} w_{4} &{} w_{2} \end{array}\right)$
Blocking pair	$(m_{3},w_{4})$	$(m_{3},w_{4})$
A	$\{m_{2},w_{4}\}$	$\{m_{3},w_{4}\}$
${\hat{\mu }}$	$\left( \begin{array}{ccccc} m_{1} &{} m_{2} &{} m_{3} &{} m_{4} &{} (w_{3})\\ w_{1} &{} w_{4} &{} w_{2} &{} (m_{4}) &{} w_{3} \end{array}\right)$	$\left( \begin{array}{ccccc} m_{1} &{} m_{2} &{} m_{3} &{} m_{4} &{} (w_{2})\\ w_{1} &{} w_{3} &{} w_{4} &{} (m_{4}) &{} w_{2} \end{array}\right)$
Blocking pair	$(m_{2},w_{1})$	$(m_{2},w_{4})$

Hence, deviation $(A,{\hat{\mu }})$ is not defensible, which is a contradiction.

If A consists of three agents, it is not defensible since the deviation is similar to pairwise ones, which is a contradiction. Thus $A=\{m_{2},m_{3},w_{1},w_{4}\}$. By defensibility, the restriction ${\hat{\mu }}|_{A}$ to A is stable in the restricted market consisting of A. Thus ${\hat{\mu }}|_{A}$ is either $\{(m_{2},w_{1}),(m_{3},w_{4})\}$ or $\{(m_{2},w_{4}),(m_{3},w_{1})\}$. In both cases, since $m_{4}$ is unmatched at ${\hat{\mu }}$, $(m_{4},w_{1})$ blocks ${\hat{\mu }}$. A contradiction.

1.2.3 A.2.3 Part (4)

Consider a static market with $M=\{m_{1},m_{2},m_{3},m_{4}\}$, $W=\{w_{1},w_{2},w_{3},w_{4}\}$, and the following preferences:

$m_{1}$	$m_{2}$	$m_{3}$	$m_{4}$	$w_{1}$	$w_{2}$	$w_{3}$	$w_{4}$
$\underline{w_{1}}$	$w_{1}$	$w_{4}$	$w_{1}$	$m_{3}$	$\underline{m_{2}}$	$\underline{m_{3}}$	$m_{2}$
	$w_{4}$	$w_{1}$	$\underline{w_{4}}$	$m_{4}$			$m_{3}$
	$\underline{w_{2}}$	$\underline{w_{3}}$		$m_{2}$			$\underline{m_{4}}$
				$\underline{m_{1}}$

where each column indicates the preference of an agent in the first row, only acceptable mates are listed in each column, and an upper mate is preferred to a lower one. Consider the matching

$$\begin{aligned} \mu =\left( \begin{array}{cccc} m_{1} &{} m_{2} &{} m_{3} &{} m_{4}\\ w_{1} &{} w_{2} &{} w_{3} &{} w_{4} \end{array}\right) . \end{aligned}$$

Each of boldfaced cells in the table indicates his or her partner from this matching. Note that matching $\mu$ is not stable but individually rational. All of blocking pairs are $(m_{2},w_{1})$, $(m_{3},w_{1})$, $(m_{4},w_{1})$, $(m_{2},w_{4})$, and $(m_{3},w_{4})$.

First, we show that $\mu$ is credibly pairwise-stable. Let $(A,{\hat{\mu }})$ be a pairwise deviation from $\mu$ such that for each $i\in A$, $u_{i}({\hat{\mu }})>u_{i}(\mu )$. We need to show that it is not defensible. Then, since A is a blocking pair, A is $\{m_{2},w_{1}\}$, $\{m_{3},w_{1}\}$, $\{m_{4},w_{1}\}$, $\{m_{2},w_{4}\}$, or $\{m_{3},w_{4}\}$. Thus we have the following five cases.

A	$\{m_{2},w_{1}\}$	$\{m_{3},w_{1}\}$
${\hat{\mu }}$	$\left( \begin{array}{ccccc} m_{1} &{} m_{2} &{} m_{3} &{} m_{4} &{} (w_{2})\\ (m_{1}) &{} w_{1} &{} w_{3} &{} w_{4} &{} w_{2} \end{array}\right)$	$\left( \begin{array}{ccccc} m_{1} &{} m_{2} &{} m_{3} &{} m_{4} &{} (w_{3})\\ (m_{1}) &{} w_{2} &{} w_{1} &{} w_{4} &{} w_{3} \end{array}\right)$
Blocking pair	$(m_{3},w_{4})$	$(m_{3},w_{4})$
A	$\{m_{4},w_{1}\}$	$\{m_{2},w_{4}\}$
${\hat{\mu }}$	$\left( \begin{array}{ccccc} m_{1} &{} m_{2} &{} m_{3} &{} m_{4} &{} (w_{4})\\ (m_{1}) &{} w_{2} &{} w_{3} &{} w_{1} &{} w_{4} \end{array}\right)$	$\left( \begin{array}{ccccc} m_{1} &{} m_{2} &{} m_{3} &{} m_{4} &{} (w_{2})\\ w_{1} &{} w_{4} &{} w_{3} &{} (m_{4}) &{} w_{2} \end{array}\right)$
Blocking pair	$(m_{3},w_{1})$	$(m_{2},w_{1})$
A	$\{m_{3},w_{4}\}$
${\hat{\mu }}$	$\left( \begin{array}{ccccc} m_{1} &{} m_{2} &{} m_{3} &{} m_{4} &{} (w_{3})\\ w_{1} &{} w_{2} &{} w_{4} &{} (m_{4}) &{} w_{3} \end{array}\right)$
Blocking pair	$(m_{2},w_{4})$

Hence, in any case, pair deviation $(A,{\hat{\mu }})$ is not defensible.

Next, we show that $\mu$ is not credibly group-stable. Consider the group deviation $(A,{\hat{\mu }})$ from $\mu$ where $A=\{m_{2},m_{3},w_{1},w_{4}\}$, ${\hat{\mu }}(m_{2})=w_{4}$, and ${\hat{\mu }}(m_{3})=w_{1}$. Note that under ${\hat{\mu }}$, all women are matched with the best mates. Thus, there is no profitable deviation from ${\hat{\mu }}$. Thus $(A,{\hat{\mu }})$ is defensible. Moreover, each agent in A is better off in ${\hat{\mu }}$ than in $\mu$. Hence $\mu$ is not credibly group-stable.

1.3 A.3 Proposition 5

Fix a stable matching $\mu$ and a defensible group deviation $(A,{\hat{\mu }})$ from $\mu$. Let B be the set of all agents outside A whose partner is not in A under $\mu$, and C be the set of all agents outside A whose partner is in A under $\mu$. Then, by Definition 4, ${\hat{\mu }}|_{B}=\mu |_{B}$ and all agents in C are unmatched at ${\hat{\mu }}$.

We first show that if C is empty then ${\hat{\mu }}$ is stable. Let C be empty. Then $M\cup W=A\cup B$. Suppose that some agent i blocks ${\hat{\mu }}$. If $i\in A$, the blocking contradicts the defensibility of $(A,{\hat{\mu }})$. If $i\in B$, since ${\hat{\mu }}|_{B}=\mu |_{B}$, i blocks $\mu$, which contradicts the stability of $\mu$. Thus no agent blocks ${\hat{\mu }}$. On the other hand, suppose that some pair (m, w) blocks ${\hat{\mu }}$. If either $m\in A$ or $w\in A$, then the blocking contradicts the defensibility of $(A,{\hat{\mu }})$. If $m\in B$ and $w\in B$, then since ${\hat{\mu }}|_{B}=\mu |_{B}$, (m, w) blocks $\mu$. This contradicts the stability of $\mu$. Hence no pair blocks ${\hat{\mu }}$. Therefore ${\hat{\mu }}$ is stable.

Thus, to prove that ${\hat{\mu }}$ is stable, we show that C is empty. Suppose for a contradiction that C is not empty. Without loss of generality, take a woman $w_{0}$ in $C\cap W$. We will recursively construct an infinite sequence $\{(m_{k},w_{k})\}_{k=1}^{\infty }$ of distinct pairs in $M\times W$ such that for each $k\in \{1,2,\ldots \}$ , (a) $\mu (m_{k})=w_{k-1}$, (b) ${\hat{\mu }}(m_{k})=w_{k}$, (c) $m_{k},w_{k}\in A$, (d) $u_{m_{k}}(\mu )<u_{m_{k}}({\hat{\mu }})$, (e) $u_{w_{k}}(\mu )>u_{w_{k}}({\hat{\mu }})$. This contradicts the finiteness of M and W.

First, construct $m_{1}$ and $w_{1}$ that satisfy conditions (a) to (e). Since $w_{0}\in C$, it follows from the definition of group deviation that $w_{0}$ is matched with some man in A at $\mu$. Denote this man by $m_{1}$ so that $m_{1}=\mu (w_{0})$ and thus (a) is satisfied. Since $w_{0}$ is unmatched at ${\hat{\mu }}$ and $\mu$ is individually rational, it follows from strict preferences that

$$\begin{aligned} u_{w_{0}}(m_{1})\equiv u_{w_{0}}(\mu )>u_{w_{0}}({\hat{\mu }})\equiv u_{w_{0}}(w_{0}). \end{aligned}$$

(5)

If $m_{1}$ were unmatched at ${\hat{\mu }}$, $u_{m_{1}}(w_{0})\equiv u_{m_{1}}(\mu )>u_{m_{1}}({\hat{\mu }})\equiv u_{m_{1}}(m_{1})$ by strict preferences and individual rationality of $\mu$. Then pair $(m_{1},w_{0})$ would block ${\hat{\mu }}$, violating defensibility of $(A,{\hat{\mu }})$ as $m_{1}\in A$. Thus, it follows from the definition of group deviation that $m_{1}$ is matched with some woman in A at ${\hat{\mu }}$. Denote this woman by $w_{1}$. Now, $m_{1},w_{1}\in A$ and $w_{1}={\hat{\mu }}(m_{1})$ so that (b) and (c) are satisfied. Note $w_{0}\ne w_{1}$. Since $w_{0}\ne w_{1}$, it follows from strict preferences that

$$\begin{aligned}&\text {either }\quad u_{m_{1}}(w_{0})\equiv u_{m_{1}}(\mu )>u_{m_{1}}(w_{1})\equiv u_{m_{1}}({\hat{\mu }}), \end{aligned}$$

(6)

$$\begin{aligned}&\text {or }\quad u_{m_{1}}(w_{0})\equiv u_{m_{1}}(\mu )<u_{m_{1}}(w_{1})\equiv u_{m_{1}}({\hat{\mu }}). \end{aligned}$$

(7)

If inequality (6) were true, then with (5), pair $(m_{1},w_{0})$ would block ${\hat{\mu }}$, violating the defensibility of $(A,{\hat{\mu }})$ as $m_{1}\in A$. Thus inequality (7) is true so that (d) is satisfied. Now, $\mu (w_{1})\ne {\hat{\mu }}(w_{1})\equiv m_{1}$, otherwise $w_{0}=w_{1}$, a contradiction. Since $\mu$ is stable, by inequality (7) and strict preferences, $u_{w_{1}}(\mu )>u_{w_{1}}({\hat{\mu }})$ so that (e) is satisfied. Now, $\{m_{1},w_{1},w_{0}\}$ satisfies conditions (a)–(e).

Suppose that we are given $w_{0}$ and $\{(m_{k},w_{k})\}_{k=1}^{K-1}$ which satisfy conditions (a) to (e) and all of whom are distinct. We construct $m_{K}$ and $w_{K}$ that satisfy the conditions. First, by our hypothesis,

$$\begin{aligned} u_{w_{K-1}}(\mu )>u_{w_{K-1}}({\hat{\mu }}). \end{aligned}$$

(8)

If $w_{K-1}$ were unmatched at $\mu$, then $w_{K-1}$ would block ${\hat{\mu }}$ by inequality (8), violating the defensibility of $(A,{\hat{\mu }})$ as $w_{K-1}\in A$ by our hypothesis. Thus $w_{K-1}$ is matched with some man at $\mu$. Denote this man by $m_{K}$ so that $m_{K}=\mu (w_{K-1})$ and so (a) is satisfied. Since by our hypothesis $w_{K-1}$ is different from $w_{1},\ldots ,w_{K-2}$ and $m_{k}=\mu (w_{k-1})$ for each $k\in \{1,\ldots ,K-1\}$, $m_{K}=\mu (w_{K-1})$ implies that $m_{K}\ne m_{1},\ldots ,m_{K-1}$ and thus $m_{1},\ldots ,m_{K}$ are distinct. If $m_{K}\not \in A$, then $m_{K}$ would be unmatched at ${\hat{\mu }}$ by the definition of group deviation. Then, since $\mu$ is individually rational, $u_{m_{K}}(w_{K-1})\equiv u_{m_{K}}(\mu )>u_{m_{K}}({\hat{\mu }})\equiv u_{m_{K}}(m_{K})$ from strict preferences. Thus, with inequality (8), pair $(m_{K},w_{K-1})$ would block ${\hat{\mu }}$, violating the defensibility as $w_{K-1}\in A$ by our hypothesis. Thus $m_{K}\in A$. If $m_{K}$ were unmatched at ${\hat{\mu }}$, then we would violate the defensibility like before. So, by the definition of group deviation, $m_{K}$ is matched with some woman in A at ${\hat{\mu }}$. Denote this woman by $w_{K}$ so that $w_{K}={\hat{\mu }}(m_{K})$ and $w_{K}\in A$, and now (b) and (c) are satisfied. Since $m_{1},\cdots ,m_{K}$ are distinct and $w_{k}={\hat{\mu }}(m_{k})$ for each $k\in \{1,\ldots ,K\}$, we have $w_{K}\ne w_{1},\ldots ,w_{K-1}$ and thus $w_{1},\ldots ,w_{K}$ are distinct. Now, because $w_{K-1}\ne w_{K}$, strict preferences imply that

$$\begin{aligned}&\text {either }\quad u_{m_{K}}(w_{K-1})\equiv u_{m_{K}}(\mu )>u_{m_{K}}(w_{K})\equiv u_{m_{K}}({\hat{\mu }}), \end{aligned}$$

(9)

$$\begin{aligned}&\text {or }\quad u_{m_{K}}(w_{K-1})\equiv u_{m_{K}}(\mu )<u_{m_{K}}(w_{K})\equiv u_{m_{K}}({\hat{\mu }}). \end{aligned}$$

(10)

If inequality (9) were true, then with (8), pair $(m_{K},w_{K-1})$ would block ${\hat{\mu }}$, violating the defensibility as $m_{K}$ and $w_{K-1}$ are in A. Thus inequality (10) holds so that (d) is satisfied. Finally, $\mu (w_{K})\ne m_{K}$ as $m_{K}$ is matched with $w_{K-1}\ne w_{K}$ at $\mu$. This implies from stability of $\mu$ and inequality (10) that $u_{w_{K}}(\mu )>u_{w_{K}}({\hat{\mu }})\equiv u_{w_{K}}(m_{K})$ so that (e) is satisfied. Now, we have the desired sequence. $\square$