Experimental and Computational Techniques of Free In-Plane Vibration of a Fixed Support Curved Beam with a Single Crack

Abstract

Purpose

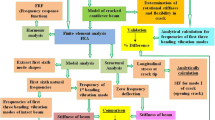
The present research paper focuses on the free in-plane vibrational analysis of a curved cracked cantilever beam using experimental and computational methods with reuse of aluminum scrape.

Method

The equation of motion governing free in-plane vibration of a curved cantilever beam with a single crack is solved numerically via DQEM (Differential Quadrature Element Method). Additionally, the fundamental frequencies and mode shape are investigated at various curvatures and depths of the curved cracked beam.

Results

The results are compared to the experimental model and finite element method to confirm the correctness of the suggested method of calculating its natural frequencies and mode shapes.

Conclusion

This study shows that the boundary conditions of the beam affect how a curved beam behaves concerning the mode transition event. The present method can also predict the impact of damage severity and location on a curved beam’s natural frequencies and mode shapes.

Appendices

Appendix 1

Differential quadrature element method

Differential equations are solve using a novel numerical method known as the DQEM. The DQEM is based on the DQ approach, which sums the values of the variables functions at all of the chosen accuracy points in the extraction axis to estimate the formulation of partial differential function at a position within the domain.

$$\frac{df}{dX}_{X={X}_{i}}=\sum_{j=1}^{N}{C}_{ij}^{(n)}{f}_{i} i,j=\mathrm{1,2},\mathrm{3,4}\dots \dots ..N$$

Equation X converts the physical coordinate to the natural coordinate.

$$\theta =\left(1-x\right){\theta }_{1}+x{\theta }_{N}$$

where ${\theta }_{1}$ and ${\theta }_{N}$ are the element’s first and Nth nodes angular coordinates. Keep in mind that any numbers between 0 and 1 are included in the values of $x$ ($0<X<1$).

Equation can be employed to apply this relation to obtain the differentiation with reference to $\theta$ (62).

Calculating the DQ weighting coefficients and choosing the precision points are two important stages in the DQ approach, as shown by Eq. (62). The weighted coefficients were calculated using Lagrangian functions, and the precision point was chosen using the Gauss-Lobatto Chebyshev polynomial.

Appendix 2

The element of [N], [J], [L], [R] and [V] as follows

$$\left[ N \right] = \left[ {\begin{array}{*{20}c} {m_{1} } & {m_{2} } & {m_{3} } & {m_{4} } & {m_{5} } & {m_{6} } \\ {m_{{1e^{{l_{1} \alpha }} }} } & {m_{{2e^{{l_{2} \alpha }} }} } & {m_{{3e^{{l_{3} \alpha }} }} } & {m_{{4e^{{l_{4} \alpha }} }} } & {m_{{5e^{{l_{5} \alpha }} }} } & {m_{{6e^{{l_{6} \alpha }} }} } \\ {n_{31} } & {n_{32} } & {n_{33} } & {n_{34} } & {n_{35} } & {n_{36} } \\ {n_{41} } & {n_{42} } & {n_{43} } & {n_{44} } & {n_{45} } & {n_{46} } \\ {n_{51} } & {n_{52} } & {n_{53} } & {n_{54} } & {n_{55} } & {n_{56} } \\ {n_{61} } & {n_{62} } & {n_{63} } & {n_{64} } & {n_{65} } & {n_{66} } \\ \end{array} } \right]$$

$${a}_{31}={u}_{1}\mathrm{sin}\aleph -\mathrm{cos}\aleph$$

$${n}_{32}={u}_{2}\mathrm{sin}\aleph -\mathrm{cos}\aleph$$

$${n}_{33}={u}_{3}\mathrm{sin}\aleph -\mathrm{cos}\aleph$$

$${n}_{34}={u}_{4}\mathrm{sin}\aleph -\mathrm{cos}\aleph$$

$${n}_{35}={u}_{5}\mathrm{sin}\aleph -\mathrm{cos}\aleph$$

$${n}_{36}={u}_{6}\mathrm{sin}\aleph -\mathrm{cos}\aleph$$

$${n}_{41}=\left({u}_{1}\mathrm{sin}\varepsilon -\mathrm{cos}\varepsilon \right){e}^{{l}_{1}\alpha }$$

$${n}_{42}=\left({u}_{2}\mathrm{sin}\varepsilon -\mathrm{cos}\varepsilon \right){e}^{{l}_{2}\alpha }$$

$${n}_{43}=\left({u}_{3}\mathrm{sin}\varepsilon -\mathrm{cos}\varepsilon \right){e}^{{l}_{3}\alpha }$$

$${n}_{44}=\left({u}_{4}\mathrm{sin}\varepsilon -\mathrm{cos}\varepsilon \right){e}^{{l}_{4}\alpha }$$

$${n}_{45}=\left({u}_{5}\mathrm{sin}\varepsilon -\mathrm{cos}\eta \right){e}^{{l}_{5}\alpha }$$

$${n}_{46}=\left({u}_{6}\mathrm{sin}\eta -\mathrm{cos}\eta \right){e}^{{l}_{6}\alpha }$$

$${n}_{51}={u}_{1}\mathrm{cos}\delta +\mathrm{sin}\delta$$

$${n}_{52}={u}_{2}\mathrm{cos}\delta +\mathrm{sin}\delta$$

$${n}_{53}={u}_{3}\mathrm{cos}\delta +\mathrm{sin}\delta$$

$${n}_{54}={u}_{4}\mathrm{cos}\delta +\mathrm{sin}\delta$$

$${n}_{55}={u}_{5}\mathrm{cos}\delta +\mathrm{sin}\delta$$

$${n}_{56}={u}_{6}\mathrm{cos}\delta +\mathrm{sin}\delta$$

$${n}_{61}=\left(-{u}_{1}\mathrm{cos}\eta +\mathrm{sin}\eta \right){e}^{{l}_{1}\alpha }$$

$${n}_{62}=\left(-{u}_{2}\mathrm{cos}\eta +\mathrm{sin}\eta \right){e}^{{l}_{2}\alpha }$$

$${n}_{63}=\left(-{u}_{3}\mathrm{cos}\eta +\mathrm{sin}\eta \right){e}^{{l}_{3}\alpha }$$

$${n}_{64}=\left(-{u}_{4}\mathrm{cos}\eta +\mathrm{sin}\eta \right){e}^{{l}_{4}\alpha }$$

$${n}_{65}=\left(-{u}_{5}\mathrm{cos}\eta +\mathrm{sin}\eta \right){e}^{{l}_{5}\alpha }$$

$${n}_{66}=\left(-{u}_{6}\mathrm{cos}\eta +\mathrm{sin}\eta \right){e}^{{l}_{6}\alpha }$$

$$\left[J\right]=\left[\begin{array}{ccc}{-m}_{1}{l}_{1}& {-m}_{2}{l}_{2}& \begin{array}{ccc}{-m}_{3}{l}_{3}& {-m}_{4}{l}_{4}& \begin{array}{cc}{-m}_{5}{l}_{5}& {-m}_{6}{l}_{6}\end{array}\end{array}\\ {{l}_{1}m}_{1{e}^{{l}_{1}\alpha }}& {{l}_{1}m}_{2{e}^{{l}_{2}\alpha }}& {l}_{1}\begin{array}{ccc}{m}_{3{e}^{{l}_{3}\alpha }}& {{l}_{1}m}_{4{e}^{{l}_{4}\alpha }}& \begin{array}{cc}{{l}_{1}m}_{5{e}^{{l}_{5}\alpha }}& {{l}_{1}m}_{6{e}^{{l}_{6}\alpha }}\end{array}\end{array}\\ \begin{array}{c}{j}_{31}\\ {j}_{41}\\ \begin{array}{c}{j}_{51}\\ {j}_{61}\end{array}\end{array}& \begin{array}{c}{j}_{32}\\ {j}_{42}\\ \begin{array}{c}{j}_{52}\\ {j}_{62}\end{array}\end{array}& \begin{array}{c}\begin{array}{ccc}{j}_{33}& {j}_{34}& \begin{array}{cc}{j}_{35}& {j}_{36}\end{array}\end{array}\\ \begin{array}{ccc}{j}_{43}& {j}_{44}& \begin{array}{cc}{j}_{45}& {j}_{46}\end{array}\end{array}\\ \begin{array}{c}\begin{array}{ccc}{j}_{53}& {j}_{54}& \begin{array}{cc}{j}_{55}& {j}_{56}\end{array}\end{array}\\ \begin{array}{ccc}{j}_{63}& {j}_{64}& \begin{array}{cc}{j}_{65}& {j}_{66}\end{array}\end{array}\end{array}\end{array}\end{array}\right]$$

$${p}_{31}={q}_{1}\mathrm{sin}\delta +{t}_{1}\mathrm{cos}\delta$$

$${p}_{32}={q}_{2}\mathrm{sin}\delta +{t}_{2}\mathrm{cos}\delta$$

$${p}_{33}={q}_{3}\mathrm{sin}\delta +{t}_{3}\mathrm{cos}\delta$$

$${p}_{34}={q}_{4}\mathrm{sin}\delta +{t}_{4}\mathrm{cos}\delta$$

$${p}_{35}={q}_{5}\mathrm{sin}\delta +{t}_{5}\mathrm{cos}\delta$$

$${p}_{36}={q}_{1}\mathrm{sin}\delta +{t}_{6}\mathrm{cos}\delta$$

$${p}_{41}=\left({q}_{1}\mathrm{sin}\eta -{t}_{1}\mathrm{cos}\eta \right){e}^{{l}_{1}\alpha }$$

$${p}_{42}=\left({q}_{2}\mathrm{sin}\eta -{t}_{2}\mathrm{cos}\eta \right){e}^{{l}_{2}\alpha }$$

$${p}_{43}=\left({q}_{3}\mathrm{sin}\eta -{t}_{3}\mathrm{cos}\eta \right){e}^{{l}_{3}\alpha }$$

$${p}_{44}=\left({q}_{4}\mathrm{sin}\eta -{t}_{4}\mathrm{cos}\eta \right){e}^{{l}_{4}\alpha }$$

$${p}_{45}=\left({q}_{5}\mathrm{sin}\eta -{t}_{5}\mathrm{cos}\eta \right){e}^{{l}_{5}\alpha }$$

$${p}_{46}=\left({q}_{6}\mathrm{sin}\eta -{t}_{6}\mathrm{cos}\eta \right){e}^{{l}_{6}\alpha }$$

$${p}_{51}={-q}_{1}\mathrm{cos}\delta +{t}_{1}\mathrm{sin}\delta$$

$${p}_{52}={-q}_{2}\mathrm{cos}\delta +{t}_{2}\mathrm{sin}\delta$$

$${p}_{53}={-q}_{3}\mathrm{cos}\delta +{t}_{3}\mathrm{sin}\delta$$

$${p}_{54}={-q}_{4}\mathrm{cos}\delta +{t}_{4}\mathrm{sin}\delta$$

$${p}_{55}={-q}_{5}\mathrm{cos}\delta +{t}_{5}\mathrm{sin}\delta$$

$${p}_{56}={-q}_{6}\mathrm{cos}\delta +{t}_{6}\mathrm{sin}\delta$$

$${p}_{61}=\left(-{q}_{1}\mathrm{cos}\eta +{t}_{1}\mathrm{sin}\eta \right){e}^{{l}_{1}\alpha }$$

$${p}_{62}=\left(-{q}_{2}\mathrm{cos}\eta +{t}_{2}\mathrm{sin}\eta \right){e}^{{l}_{2}\alpha }$$

$${p}_{63}=\left(-{q}_{3}\mathrm{cos}\eta +{t}_{3}\mathrm{sin}\eta \right){e}^{{l}_{3}\alpha }$$

$${p}_{64}=\left(-{q}_{4}\mathrm{cos}\eta +{t}_{4}\mathrm{sin}\eta \right){e}^{{l}_{4}\alpha }$$

$${p}_{65}=\left(-{q}_{5}\mathrm{cos}\eta +{t}_{5}\mathrm{sin}\eta \right){e}^{{l}_{5}\alpha }$$

$${p}_{66}=\left(-{q}_{6}\mathrm{cos}\eta +{t}_{6}\mathrm{sin}\eta \right){e}^{{l}_{6}\alpha }$$

$$[C] = \left[\begin{array}{*{20}{c}} {{c_{1,1}}}&{{c_{1,2}}}&{{c_{1,3}}}&{{c_{1,4}}}&{{c_{1,5}}}&{{c_{1,6}}}&0&0&0&0&0&0\\ 0&0&0&0&0&0&{{c_{2,7}}}&{{c_{2,8}}}&{{c_{2,9}}}&{{c_{2,10}}}&{{c_{2,11}}}&{{c_{2,12}}}\\ {{c_{3,1}}}&{{c_{3,2}}}&{{c_{3,3}}}&{{c_{3,4}}}&{{c_{3,5}}}&{{c_{3,6}}}&0&0&0&0&0&0\\ 0&0&0&0&0&0&{{c_{4,7}}}&{{c_{4,8}}}&{{c_{4,9}}}&{{c_{4,10}}}&{{c_{4,11}}}&{{c_{4,12}}}\\ {{c_{5,1}}}&{{c_{5,2}}}&{{c_{5,3}}}&{{c_{5,4}}}&{{c_{5,5}}}&{{c_{5,6}}}&0&0&0&0&0&0\\ 0&0&0&0&0&0&{{c_{6,7}}}&{{c_{6,8}}}&{{c_{6,9}}}&{{c_{6,10}}}&{{c_{6,11}}}&{{c_{6,12}}}\\ {{c_{7,1}}}&{{c_{7,2}}}&{{c_{7,3}}}&{{c_{7,4}}}&{{c_{7,5}}}&{{c_{7,6}}}&{ - 1}&{ - 1}&{ - 1}&{ - 1}&{ - 1}&{ - 1}\\ {{c_{8,1}}}&{{c_{8,2}}}&{{c_{8,3}}}&{{c_{8,4}}}&{{c_{8,5}}}&{{c_{8,6}}}&{{c_{8,7}}}&{{c_{8,8}}}&{{c_{8,9}}}&{{c_{8,10}}}&{{c_{8,11}}}&{{c_{8,12}}}\\ {{c_{9,1}}}&{{c_{9,2}}}&{{c_{9,3}}}&{{c_{9,4}}}&{{c_{9,5}}}&{{c_{9,6}}}&{{c_{9,7}}}&{{c_{9,8}}}&{{c_{9,9}}}&{{c_{9,10}}}&{{c_{9,11}}}&{{c_{9,12}}}\\ {{c_{10,1}}}&{{c_{10,2}}}&{{c_{10,3}}}&{{c_{10,4}}}&{{c_{10,5}}}&{{c_{10,6}}}&{{c_{10,7}}}&{{c_{10,8}}}&{{c_{10,9}}}&{{c_{10,10}}}&{{c_{10,11}}}&{{c_{10,12}}}\\ {{c_{11,1}}}&{{c_{11,2}}}&{{c_{11,3}}}&{{c_{11,4}}}&{{c_{11,5}}}&{{c_{11,6}}}&{{c_{11,7}}}&{{c_{11,8}}}&{{c_{11,9}}}&{{c_{11,10}}}&{{c_{11,11}}}&{{c_{11,12}}}\\ {{c_{12,1}}}&{{c_{12,2}}}&{{c_{12,3}}}&{{c_{12,4}}}&{{c_{12,5}}}&{{c_{12,6}}}&{{c_{12,7}}}&{{c_{12,8}}}&{{c_{12,9}}}&{{c_{12,10}}}&{{c_{12,11}}}&{{c_{12,12}}} \end{array}\right]$$

$${c}_{\mathrm{1,1}}={m}_{1}$$

$${c}_{\mathrm{1,2}}={m}_{1}$$

$${c}_{\mathrm{1,3}}={m}_{1}$$

$${c}_{\mathrm{1,4}}={m}_{1}$$

$${c}_{\mathrm{1,5}}={m}_{1}$$

$${c}_{\mathrm{1,6}}={m}_{1}$$

$${c}_{\mathrm{2,7}}={m}_{1{e}^{{l}_{1}\alpha }}$$

$${c}_{\mathrm{2,8}}={m}_{1{e}^{{l}_{2}\alpha }}$$

$${c}_{\mathrm{2,9}}={m}_{3{e}^{{l}_{3}\alpha }}$$

$${c}_{\mathrm{2,10}}={m}_{3{e}^{{l}_{4}\alpha }}$$

$${c}_{\mathrm{2,11}}={m}_{5{e}^{{l}_{5}\alpha }}$$

$${c}_{\mathrm{2,12}}={m}_{5{e}^{{l}_{6}\alpha }}$$

$${c}_{\mathrm{3,1}}={u}_{1}\mathrm{sin}\delta -\mathrm{cos}\delta$$

$${c}_{\mathrm{3,2}}={u}_{2}\mathrm{sin}\delta -\mathrm{cos}\delta$$

$${c}_{\mathrm{3,3}}={u}_{3}\mathrm{sin}\delta -\mathrm{cos}\delta$$

$${c}_{\mathrm{3,4}}={u}_{4}\mathrm{sin}\delta -\mathrm{cos}\delta$$

$${c}_{\mathrm{3,5}}={u}_{5}\mathrm{sin}\delta -\mathrm{cos}\delta$$

$${c}_{\mathrm{3,6}}={u}_{6}\mathrm{sin}\delta -\mathrm{cos}\delta$$

$${c}_{\mathrm{4,1}}=\left({u}_{1}\mathrm{sin}\eta +\mathrm{cos}\eta \right){e}^{{l}_{1}\alpha }$$

$${c}_{\mathrm{4,2}}=\left({u}_{2}\mathrm{sin}\eta +\mathrm{cos}\eta \right){e}^{{l}_{2}\alpha }$$

$${c}_{\mathrm{4,3}}=\left({u}_{3}\mathrm{sin}\eta +\mathrm{cos}\eta \right){e}^{{l}_{3}\alpha }$$

$${c}_{\mathrm{4,4}}=\left({u}_{4}\mathrm{sin}\eta +\mathrm{cos}\eta \right){e}^{{l}_{4}\alpha }$$

$${c}_{\mathrm{4,5}}=\left({u}_{5}\mathrm{sin}\eta +\mathrm{cos}\eta \right){e}^{{l}_{5}\alpha }$$

$${c}_{\mathrm{4,6}}=\left({u}_{6}\mathrm{sin}\eta +\mathrm{cos}\eta \right){e}^{{l}_{6}\alpha }$$

$${c}_{\mathrm{5,1}}={u}_{1}\mathrm{cos}\delta +\mathrm{sin}\delta$$

$${c}_{\mathrm{5,2}}={u}_{2}\mathrm{cos}\delta +\mathrm{sin}\delta$$

$${c}_{\mathrm{5,3}}={u}_{3}\mathrm{cos}\delta +\mathrm{sin}\delta$$

$${c}_{\mathrm{5,4}}={u}_{4}\mathrm{cos}\delta +\mathrm{sin}\delta$$

$${c}_{\mathrm{5,5}}={u}_{5}\mathrm{cos}\delta +\mathrm{sin}\delta$$

$${c}_{\mathrm{5,6}}={u}_{6}\mathrm{cos}\delta +\mathrm{sin}\delta$$

$${c}_{\mathrm{6,1}}=\left({-u}_{1}\mathrm{cos}\eta +\mathrm{sin}\eta \right){e}^{{l}_{1}\alpha }$$

$${c}_{\mathrm{6,2}}=\left({-u}_{2}\mathrm{cos}\eta +\mathrm{sin}\eta \right){e}^{{l}_{2}\alpha }$$

$${c}_{\mathrm{6,3}}=\left({-u}_{3}\mathrm{cos}\eta +\mathrm{sin}\eta \right){e}^{{l}_{3}\alpha }$$

$${c}_{\mathrm{6,4}}=\left({-u}_{4}\mathrm{cos}\eta +\mathrm{sin}\eta \right){e}^{{l}_{4}\alpha }$$

$$c=\left({-u}_{5}\mathrm{cos}\eta +\mathrm{sin}\eta \right){e}^{{l}_{5}\alpha }$$

$${c}_{\mathrm{6,6}}=\left({-u}_{6}\mathrm{cos}\eta +\mathrm{sin}\eta \right){e}^{{l}_{6}\alpha }$$

$${c}_{\mathrm{7,1}}={e}^{{l}_{1}\beta }$$

$${c}_{\mathrm{7,2}}={e}^{{l}_{2}\beta }$$

$${c}_{\mathrm{7,3}}={e}^{{l}_{3}\beta }$$

$${c}_{\mathrm{7,4}}={e}^{{l}_{4}\beta }$$

$${c}_{\mathrm{7,5}}={e}^{{l}_{5}\beta }$$

$${zc}_{\mathrm{7,6}}={e}^{{l}_{6}\beta }$$

$${c}_{\mathrm{8,1}}={{u}_{1}e}^{{l}_{1}\beta }$$

$${c}_{\mathrm{8,2}}={{u}_{2}e}^{{l}_{2}\beta }$$

$${c}_{\mathrm{8,3}}={{u}_{3}e}^{{l}_{3}\beta }$$

$${c}_{\mathrm{8,4}}={{u}_{4}e}^{{l}_{4}\beta }$$

$${c}_{\mathrm{8,5}}={{u}_{5}e}^{{l}_{5}\beta }$$

$${c}_{\mathrm{8,6}}={{u}_{6}e}^{{l}_{6}\beta }$$

$${c}_{\mathrm{8,7}}=-{u}_{1}$$

$${c}_{\mathrm{8,8}}=-{u}_{2}$$

$${c}_{\mathrm{8,9}}=-{u}_{3}$$

$${c}_{\mathrm{8,10}}=-{u}_{4}$$

$${c}_{\mathrm{8,11}}=-{u}_{5}$$

$${c}_{\mathrm{8,12}}=-{u}_{6}$$

$${c}_{\mathrm{9,1}}=-\frac{{m}_{1}{e}^{{l}_{1}\beta }}{R}-\frac{EI{R}^{2}{l}_{1}{m}_{1}{e}^{{l}_{1}\beta }}{K}$$

$${c}_{\mathrm{9,2}}=-\frac{{m}_{2}{e}^{{l}_{2}\beta }}{R}-\frac{EI{R}^{2}{l}_{2}{m}_{2}{e}^{{l}_{2}\beta }}{K}$$

$${c}_{\mathrm{9,3}}=-\frac{{m}_{3}{e}^{{l}_{3}\beta }}{R}-\frac{EI{R}^{2}{l}_{3}{m}_{3}{e}^{{l}_{3}\beta }}{K}$$

$${c}_{\mathrm{9,4}}=-\frac{{m}_{4}{e}^{{l}_{4}\beta }}{R}-\frac{EI{R}^{2}{l}_{4}{m}_{4}{e}^{{l}_{4}\beta }}{K}$$

$${c}_{\mathrm{9,5}}=-\frac{{m}_{5}{e}^{{l}_{5}\beta }}{R}-\frac{EI{R}^{2}{l}_{5}{m}_{5}{e}^{{l}_{5}\beta }}{K}$$

$${c}_{\mathrm{9,6}}=-\frac{{m}_{6}{e}^{{l}_{2}\beta }}{R}-\frac{EI{R}^{2}{l}_{6}{m}_{6}{e}^{{l}_{6}\beta }}{K}$$

$${c}_{\mathrm{9,7}}=\frac{{m}_{1}}{R}$$

$${c}_{\mathrm{9,8}}=\frac{{m}_{2}}{R}$$

$${c}_{\mathrm{9,9}}=\frac{{m}_{3}}{R}$$

$${c}_{\mathrm{9,10}}=\frac{{m}_{4}}{R}$$

$${c}_{\mathrm{9,11}}=\frac{{m}_{5}}{R}$$

$${c}_{\mathrm{9,12}}=\frac{{m}_{6}}{R}$$

$${c}_{\mathrm{10,1}}=-\frac{EI}{{R}^{3}}{{t}_{1}e}^{{l}_{1}\beta }$$

$${c}_{\mathrm{10,2}}=-\frac{EI}{{R}^{3}}{{t}_{2}e}^{{l}_{2}\beta }$$

$${c}_{\mathrm{10,3}}=-\frac{EI}{{R}^{3}}{{t}_{3}e}^{{l}_{3}\beta }$$

$${c}_{\mathrm{10,4}}=-\frac{EI}{{R}^{3}}{{t}_{4}e}^{{l}_{4}\beta }$$

$${c}_{\mathrm{10,5}}=-\frac{EI}{{R}^{3}}{{t}_{5}e}^{{l}_{5}\beta }$$

$${c}_{\mathrm{10,6}}=-\frac{EI}{{R}^{3}}{{t}_{6}e}^{{l}_{6}\beta }$$

$${c}_{\mathrm{10,7}}=-\frac{EI}{{R}^{3}}{t}_{1}$$

$${c}_{\mathrm{10,8}}=-\frac{EI}{{R}^{3}}{t}_{2}$$

$${c}_{\mathrm{10,9}}=-\frac{EI}{{R}^{3}}{t}_{3}$$

$${c}_{\mathrm{10,10}}=-\frac{EI}{{R}^{3}}{t}_{4}$$

$${c}_{\mathrm{10,11}}=-\frac{EI}{{R}^{3}}{t}_{5}$$

$${c}_{\mathrm{10,12}}=-\frac{EI}{{R}^{3}}{t}_{6}$$

$${c}_{\mathrm{11,1}}=-\frac{EI}{{R}^{3}}{{q}_{1}e}^{{l}_{1}\beta }$$

$${c}_{\mathrm{11,2}}=-\frac{EI}{{R}^{3}}{{q}_{2}e}^{{l}_{2}\beta }$$

$${c}_{\mathrm{11,3}}=-\frac{EI}{{R}^{3}}{{q}_{3}e}^{{l}_{3}\beta }$$

$${c}_{\mathrm{11,4}}=-\frac{EI}{{R}^{3}}{{q}_{4}e}^{{l}_{4}\beta }$$

$${c}_{\mathrm{11,5}}=-\frac{EI}{{R}^{3}}{{q}_{5}e}^{{l}_{5}\beta }$$

$${c}_{\mathrm{11,6}}=-\frac{EI}{{R}^{3}}{{q}_{1}e}^{{l}_{6}\beta }$$

$${c}_{\mathrm{11,7}}=-\frac{EI}{{R}^{3}}{{q}_{7}e}^{{l}_{7}\beta }$$

$${c}_{\mathrm{11,8}}=-\frac{EI}{{R}^{3}}{{q}_{8}e}^{{l}_{8}\beta }$$

$${c}_{\mathrm{11,9}}=-\frac{EI}{{R}^{3}}{{q}_{9}e}^{{l}_{9}\beta }$$

$${c}_{\mathrm{11,10}}=-\frac{EI}{{R}^{3}}{{q}_{10}e}^{{l}_{10}\beta }$$

$${c}_{\mathrm{11,11}}=-\frac{EI}{{R}^{3}}{{q}_{11}e}^{{l}_{11}\beta }$$

$${c}_{\mathrm{11,12}}=-\frac{EI}{{R}^{3}}{{q}_{12}e}^{{l}_{12}\beta }$$

$${c}_{\mathrm{12,1}}=-\frac{EI}{{R}^{2}}{{q}_{1}{m}_{1}e}^{{l}_{1}\beta }$$

$${c}_{\mathrm{12,2}}=-\frac{EI}{{R}^{2}}{{q}_{2}{m}_{2}e}^{{l}_{2}\beta }$$

$${c}_{\mathrm{12,3}}=-\frac{EI}{{R}^{2}}{{q}_{3}{m}_{3}e}^{{l}_{3}\beta }$$

$${c}_{\mathrm{12,4}}=-\frac{EI}{{R}^{2}}{{q}_{4}{m}_{4}e}^{{l}_{4}\beta }$$

$${c}_{\mathrm{12,5}}=-\frac{EI}{{R}^{2}}{{q}_{5}{m}_{5}e}^{{l}_{5}\beta }$$

$${c}_{\mathrm{12,6}}=-\frac{EI}{{R}^{2}}{{q}_{6}{m}_{6}e}^{{l}_{6}\beta }$$

$${c}_{\mathrm{12,7}}=-\frac{EI}{{R}^{2}}{q}_{1}{m}_{1}$$

$${c}_{\mathrm{12,8}}=-\frac{EI}{{R}^{2}}{q}_{2}{m}_{2}$$

$${c}_{\mathrm{12,9}}=-\frac{EI}{{R}^{2}}{q}_{3}{m}_{3}$$

$${c}_{\mathrm{12,10}}=-\frac{EI}{{R}^{2}}{q}_{4}{m}_{4}$$

$${c}_{\mathrm{12,11}}=-\frac{EI}{{R}^{2}}{q}_{5}{m}_{5}$$

$${c}_{\mathrm{12,12}}=-\frac{EI}{{R}^{2}}{q}_{6}{m}_{6}$$

$$\left[R\right]=\left[\begin{array}{c}\begin{array}{c}{S}_{0}(\mathrm{1,1}:12)\\ {S}_{0}(\mathrm{8,1}:12)\end{array}\\ \begin{array}{ccc}\begin{array}{cc}\begin{array}{c}{\left[O\right]}_{1x3}\\ {\left[O\right]}_{1x5}\end{array}& \begin{array}{c}1\\ 1\end{array}\end{array}& \begin{array}{c}{\left[O\right]}_{1x4}\\ {\left[O\right]}_{1x4}\end{array}& \begin{array}{cc}\begin{array}{c}-1\\ -1\end{array}& \begin{array}{c}{\left[O\right]}_{1x3}\\ {\left[O\right]}_{1x1}\end{array}\end{array}\end{array}\\ \begin{array}{c}{S}_{0}\left(\mathrm{4,1}:12\right)-{S}_{0}(\mathrm{9,1}:12)\\ \begin{array}{c}{S}_{0}\left(\mathrm{6,1}:12\right)-{S}_{0}(\mathrm{11,1}:12)\\ \begin{array}{c}{S}_{0}\left(\mathrm{2,1}:12\right)+{S}_{0}(\mathrm{7,1}:12)\\ {g}_{\mathrm{8,1} }{g}_{\mathrm{8,2} {g}_{\mathrm{8,3}: }{g}_{\mathrm{8,6} {g}_{\mathrm{8,7}:12}}}\end{array}\end{array}\end{array}\end{array}\right]$$

$${g}_{\mathrm{8,1}}=R{S}_{0}(\mathrm{2,1})$$

$${g}_{\mathrm{8,2}}=R{S}_{0}\left(\mathrm{2,1}\right)+\frac{K}{R}$$

$${g}_{\mathrm{8,3}:5}=R{S}_{0}(\mathrm{2,3}:6)$$

$${g}_{\mathrm{8,6}}=R{S}_{0}(\mathrm{2,7})+\frac{K}{R}$$

$${g}_{\mathrm{8,7}:12}=R{S}_{0}(\mathrm{2,8}:12)$$

$$\left[ V \right] = \left[\begin{array}{*{20}{c}} {{v_{1,1}}}&{{v_{1,2}}}&{{v_{1,3}}}&{{v_{1,4}}}&{{v_{1,5}}}&{{v_{1,6}}}&0&0&0&0&0&0\\ {{v_{2,1}}}&{{v_{2,2}}}&{{v_{2,3}}}&{{v_{2,4}}}&{{v_{2,5}}}&{{v_{2,6}}}&0&0&0&0&0&0\\ {{v_{3,1}}}&{{v_{3,2}}}&{{v_{3,3}}}&{{v_{3,4}}}&{{v_{3,5}}}&{{v_{3,6}}}&0&0&0&0&0&0\\ 0&0&0&0&0&0&{{v_{4,7}}}&{{v_{4,8}}}&{{v_{4,9}}}&{{v_{4,10}}}&{{v_{4,11}}}&{{v_{4,12}}}\\ 0&0&0&0&0&0&{{v_{5,7}}}&{{v_{5,8}}}&{{v_{5,9}}}&{{v_{5,10}}}&{{v_{5,11}}}&{{v_{5,12}}}\\ 0&0&0&0&0&0&{{v_{6,7}}}&{{v_{6,8}}}&{{v_{6,9}}}&{{v_{6,10}}}&{{v_{6,11}}}&{{v_{6,12}}}\\ {{v_{7,1}}}&{{v_{7,2}}}&{{v_{7,3}}}&{{v_{7,4}}}&{{v_{7,5}}}&{{v_{7,6}}}&{{v_{7,7}}}&{{v_{7,8}}}&{{v_{7,9}}}&{{v_{7,10}}}&{{v_{7,11}}}&{{v_{7,12}}}\\ {{v_{8,1}}}&{{v_{8,2}}}&{{v_{8,3}}}&{{v_{8,4}}}&{{v_{8,5}}}&{{v_{8,6}}}&{{v_{8,7}}}&{{v_{8,8}}}&{{v_{8,9}}}&{{v_{8,10}}}&{{v_{8,11}}}&{{v_{9,12}}}\\ {{v_{9,1}}}&{{v_{9,2}}}&{{v_{9,3}}}&{{v_{9,4}}}&{{v_{9,5}}}&{{v_{9,6}}}&{{v_{9,7}}}&{{v_{9,8}}}&{{v_{9,9}}}&{{v_{10,10}}}&{{v_{11,11}}}&{{v_{12,12}}}\\ {{v_{10,1}}}&{{v_{10,2}}}&{{v_{10,3}}}&{{v_{10,4}}}&{{v_{10,5}}}&{{v_{10,6}}}&{{v_{10,7}}}&{{v_{10,8}}}&{{v_{10,9}}}&{{v_{10,10}}}&{{v_{10,11}}}&{{v_{10,12}}}\\ {{v_{11,1}}}&{{v_{11,2}}}&{{v_{11,3}}}&{{v_{11,4}}}&{{v_{11,5}}}&{{v_{11,6}}}&{{v_{11,7}}}&{{v_{11,8}}}&{{v_{11,9}}}&{{v_{11,10}}}&{{v_{11,11}}}&{{v_{11,12}}}\\ {{v_{12,1}}}&{{v_{12,2}}}&{{v_{12,3}}}&{{v_{12,4}}}&{{v_{12,5}}}&{{v_{12,6}}}&{{v_{12,7}}}&{{v_{12,8}}}&{{v_{12,9}}}&{{v_{12,10}}}&{{v_{12,11}}}&{{v_{12,12}}} \end{array}\right]$$

The seventh through twelfth row $\left[S\right]$ and $\left[Z\right]$ are the same and other element of $\left[S\right]$ are considered as follows

$${s}_{\mathrm{1,1}}=-{m}_{1}{l}_{1}$$

$${s}_{\mathrm{1,2}}=-{m}_{2}{l}_{2}$$

$${s}_{\mathrm{1,3}}=-{m}_{3}{l}_{3}$$

$${s}_{\mathrm{1,4}}=-{m}_{4}{l}_{4}$$

$${s}_{\mathrm{1,5}}=-{m}_{5}{l}_{5}$$

$${s}_{\mathrm{1,6}}=-{m}_{1}{l}_{6}$$

$${s}_{\mathrm{2,1}}={q}_{1}\mathrm{sin}\rho +{t}_{1}\mathrm{cos}\rho$$

$${s}_{\mathrm{2,2}}={q}_{2}\mathrm{sin}\rho +{t}_{2}\mathrm{cos}\rho$$

$${s}_{\mathrm{2,3}}={q}_{3}\mathrm{sin}\rho +{t}_{3}\mathrm{cos}\rho$$

$${s}_{\mathrm{2,4}}={q}_{4}\mathrm{sin}\rho +{t}_{4}\mathrm{cos}\rho$$

$${s}_{\mathrm{2,5}}={q}_{5}\mathrm{sin}\rho +{t}_{5}\mathrm{cos}\rho$$

$${s}_{\mathrm{2,6}}={q}_{6}\mathrm{sin}\rho +{t}_{6}\mathrm{cos}\rho$$

$${s}_{\mathrm{3,1}}={q}_{1}\mathrm{cos}\rho +{t}_{1}\mathrm{sin}\rho$$

$${s}_{\mathrm{3,2}}={q}_{2}\mathrm{cos}\rho +{t}_{2}\mathrm{sin}\rho$$

$${s}_{\mathrm{3,3}}={q}_{3}\mathrm{cos}\rho +{t}_{3}\mathrm{sin}\rho$$

$${s}_{\mathrm{3,4}}={q}_{4}\mathrm{cos}\rho +{t}_{4}\mathrm{sin}\rho$$

$${s}_{\mathrm{3,5}}={q}_{5}\mathrm{cos}\rho +{t}_{5}\mathrm{sin}\rho$$

$${s}_{\mathrm{3,6}}={q}_{6}\mathrm{cos}\rho +{t}_{6}\mathrm{sin}\rho$$

$${s}_{\mathrm{4,7}}=-{{l}_{1}{m}_{1}e}^{{l}_{1}\alpha }$$

$${s}_{\mathrm{4,2}}=-{{l}_{2}{m}_{2}e}^{{l}_{2}\alpha }$$

$${s}_{\mathrm{4,9}}=-{{l}_{3}{m}_{3}e}^{{l}_{3}\alpha }$$

$${s}_{\mathrm{4,4}}=-{{l}_{4}{m}_{4}e}^{{l}_{4}\alpha }$$

$${s}_{\mathrm{4,11}}=-{{l}_{5}{m}_{5}e}^{{l}_{5}\alpha }$$

$${s}_{\mathrm{4,6}}=-{{l}_{6}{m}_{6}e}^{{l}_{6}\alpha }$$

$${s}_{\mathrm{5,7}}=\left({q}_{1}\mathrm{sin}\eta -{t}_{1}\mathrm{cos}\eta \right){e}^{{l}_{1}\alpha }$$

$${s}_{\mathrm{5,2}}=\left({q}_{2}\mathrm{sin}\eta -{t}_{2}\mathrm{cos}\eta \right){e}^{{l}_{2}\alpha }$$

$${s}_{\mathrm{5,9}}=\left({q}_{3}\mathrm{sin}\eta -{t}_{3}\mathrm{cos}\eta \right){e}^{{l}_{3}\alpha }$$

$${s}_{\mathrm{5,4}}=\left({q}_{4}\mathrm{sin}\eta -{t}_{4}\mathrm{cos}\eta \right){e}^{{l}_{4}\alpha }$$

$${s}_{\mathrm{5,11}}=\left({q}_{5}\mathrm{sin}\eta -{t}_{5}\mathrm{cos}\eta \right){e}^{{l}_{5}\alpha }$$

$${s}_{\mathrm{5,6}}=\left({q}_{6}\mathrm{sin}\eta -{t}_{6}\mathrm{cos}\eta \right){e}^{{l}_{6}\alpha }$$

$${s}_{\mathrm{6,7}}=\left({q}_{1}\mathrm{cos}\eta +{t}_{1}\mathrm{sin}\eta \right){e}^{{l}_{1}\alpha }$$

$${s}_{\mathrm{6,2}}=\left({q}_{2}\mathrm{cos}\eta +{t}_{2}\mathrm{sin}\eta \right){e}^{{l}_{2}\alpha }$$

$${s}_{\mathrm{6,9}}=\left({q}_{3}\mathrm{cos}\eta +{t}_{3}\mathrm{sin}\eta \right){e}^{{l}_{3}\alpha }$$

$${s}_{\mathrm{6,4}}=\left({q}_{4}\mathrm{cos}\eta +{t}_{4}\mathrm{sin}\eta \right){e}^{{l}_{4}\alpha }$$

$${s}_{\mathrm{6,11}}=\left({q}_{5}\mathrm{cos}\eta +{t}_{5}\mathrm{sin}\eta \right){e}^{{l}_{5}\alpha }$$

$${s}_{\mathrm{6,6}}=\left({q}_{6}\mathrm{cos}\eta +{t}_{6}\mathrm{sin}\eta \right){e}^{{l}_{6}\alpha }$$