Introduction

By means of transducers made of piezoelectric materials, mechanical deformations can be generated by an electrical voltage [1, 2]. The application of a high-frequency alternating voltage (> 20 kHz) to piezoelectric transducers leads to ultrasonic vibrations. Ultrasound is used in many areas of technology, such as medical diagnostics and non-destructive materials testing [3]. Power ultrasound with high sound intensities offers a wide range of applications such as medical devices, ultrasonic cleaning, ultrasonic welding and sonochemistry [4, 5].

Commonly used are piezo-ceramics such as lead zirconate titanate PZT, in which piezoelectric powder materials are sintered with a binder [6]. Piezo-ceramics such as PZT4 and PZT8 are used for power ultrasonic transducers [7].

A widely used piezoelectric ultrasonic transducer is the bolt-clamped Langevin transducer, in which the piezo-ceramics are clamped between two end masses by means of a screw connection [1].

Piezoelectric ultrasonic transducers exhibit a temperature-dependent behavior. This was experimentally determined by Upadhye et al. [6]. Their resonance frequencies change due to the temperature dependence of the materials [6]. Since Langevin transducers have their highest efficiency in resonance [1], the exact determination and prediction of the resonance frequency is important for their design and development. In many technical applications, the resonance frequency must be determined during operation in order to optimally control the transducers. The aim of this contribution is to predict the resonance frequency of Langevin transducers and its temperature dependence in order to improve the accuracy of the design.

The temperature dependency of piezoelectric transducers can be described by equivalent electrical circuits and mathematical models [8,9,10,11,12].

Another common method for describing ultrasonic transducers is the finite-element method FEM [4, 5, 13,14,15]. By means of this method, geometrically complex shapes such as Langevin transducers in combination with horns can also be simulated. In order to represent the temperature dependence of the materials, it is necessary to use temperature-dependent functions of the material coefficients. Due to the sharp shape of the resonance curve, even slight deviations lead to extreme efficiency reduction of the transducer. Therefore, it is important to employ exact material coefficients in the FEM Model [16].

In a first step of this contribution, a FEM base model is created employing measured material data from approved previous investigations. Then, the material coefficients with an influence on the resonance frequency are examined. These are modified by an optimization algorithm to fit the experimental results and then validated to require material coefficients with improved accuracy.

In order to determine material- and geometry-related influences on the resonance frequency and its temperature dependence, six variants of transducers are experimentally examined between 30 °C and 80 °C. The minimum temperature of 30 °C is restricted because of inevitably heating of transducers above room temperature due to losses during their operation. The maximum temperature of 80 °C is limited by the heat resistance of the insulating material which is necessary to prevent a short circuit. The transducers differ in three geometries and in two materials of the end masses and ultrasonic horn booster.

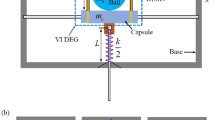
Langevin transducers without horn (type 1) and two Langevin transducers with differently shaped horns (types 2 and 3) are examined. They are shown in Table 1 and Figs. 1 and 2.

Table 1 Overview of the examined Langevin transducers
Full size table
Fig. 1
figure 1

Langevin transducer type 1: (1) screw M8 × 65, (2) washer, (3) end mass, (4) electrode, (6) piezo-ceramic PZT8, (7) nut

Full size image
Fig. 2
figure 2

Langevin transducer with horn types 2 and 3: (1) screw M8 × 65, (2) washer, (3) end mass, (4) electrode, (5) support ring, (6) piezo-ceramic PZT8, (7) top: stepped horn—type 3/bottom: round horn—type 2

Full size image

The Langevin transducers without a horn consist of two oppositely polarized piezo-ceramics clamped between two end masses (Fig. 1). They are clamped using a screw and a nut with a pretensioning force of 15 kN. The screw is separated from the electrodes by insulating material.

In addition to the Langevin transducer without horn, Langevin transducers in combination with two horn geometries (ultrasonic horn booster) are examined (Fig. 2). The stepped and rounded horns amplify the vibration amplitudes [15, 17].

The end masses and horns of the transducers are made of the structural steel S235 for the variants S and of the titanium alloy Ti6Al4V for the variants T. The different variants of the examined Langevin transducers are listed in Table 1.

Experimental Investigation of the Temperature Dependence

Within the scope of the experimental investigation, the resonance frequency of the Langevin transducers is determined by an impedance frequency response. The impedance is the ratio of the complex amplitude of the applied sinusoidal voltage and the complex amplitude of the flowing current. The frequency at the impedance minimum corresponds to the mechanical resonance frequency of the Langevin transducer [18].

Experimental Setup

The setup for the experimental investigation is shown in Fig. 3. The Langevin transducers are heated to temperatures of 30, 40, 50, 60, 70 and 80 °C in a heating chamber. The temperature is measured using an infrared thermometer (Voltcraft IR 500-12D). The measuring point at the piezo-ceramic (emissivity 0.95) is shown in Fig. 3. An ultrasound generator and a measuring computer are used for control and data acquisition.

Fig. 3
figure 3

Measuring setup for examining the vibration behavior consisting of an ultrasound generator, Langevin converter (without horn), measuring computer and temperature measurement with an IR thermometer

Full size image

To determine the resonance frequency, a frequency response in the range of 2 kHz around the resonance frequency is recorded with an RMS sine voltage of 10 V applied to the piezo-ceramics. With the measurement setup, the resonance frequency can be determined with a deviation of ± 0.01%.

Temperature-Dependent Resonance Measurement

As a result of the experimental investigation, the temperature-dependent shift of the resonance frequency of each shape is examined. Figure 4 shows an example of the impedance curves of shape T3 (see Table 1) at temperatures from 30 °C to 80 °C.

Fig. 4
figure 4

Mechanical resonance of T3 with increasing temperature

Full size image

The mechanical resonance frequencies are determined on the basis of the impedance minima. The resonance frequency decreases by approximately 870 Hz when the temperature increases from 30 °C to 80 °C.

The temperature-dependent resonance frequency curves of the various types in Figs. 5 and 6 show that all types have a descending resonance frequency as the temperature increases. The comparison of the types shows that the resonance frequencies of the types made of Ti6Al4V (T1-3) are higher than those of the types made of S235 (S1–3).

Fig. 5
figure 5

Descending resonance frequency with increasing temperature of the types T1–3

Full size image
Fig. 6
figure 6

Descending resonance frequency with increasing temperature of the types S1–3

Full size image

Within a material, the Langevin transducers without horn (type T1 and S1) have the highest resonance frequencies. The Langevin transducers with a stepped horn (type T3 and S3) have the lowest resonance frequencies.

The gradient of the resonance frequency of the individual shapes from the point at 30 °C to the point at 80 °C of the experiments can be taken from Fig. 7. It is approximately linear according to Figs. 5 and 6. The types made of Ti6Al4V with horn T2 and T3 show a higher temperature dependence of the resonance frequency of − 19.4 Hz/°C and − 17.4 Hz/°C than the types made of S235 with horn S2 and S3. The latter have a resonance frequency gradient of − 5.0 Hz/°C and − 6.0 Hz/°C. The resonance frequency of the types without horn T1 and S1 have a similar dependence on temperature with a gradient of − 5.6 Hz/°C and − 5.0 Hz/°C, respectively.

Fig. 7
figure 7

Gradient of resonance frequency of types T1–3 and S1–3 between 30 °C and 80 °C in the experiments Exp

Full size image

FEM Model

Harmonic calculations are performed in ANSYS to describe and predict the resonance frequency of the piezoelectric Langevin transducers using FEM. The types are considered as rotationally symmetrical 2D geometries.

Temperature-Dependent Material Models

In the FEM model, different material models are used to describe the piezo-materials and the metallic materials.

Piezo-ceramics such as PZT8 have an anisotropic material behavior. The metallic materials are assumed to be isotropic.

All material coefficients required for the description of the material model are parameterized. Temperature-dependent functions are assigned to them in the parameter set. The temperature is defined as a parameter on which all material-related parameters depend.

Piezo-materials

The piezo-elements made of the piezo-material PZT8 can be calculated by the (Application Customization Toolkit ACT) extension PiezoAndMEMS and by inserting piezoelectric properties via the ANSYS Parametric Design Language APDL [19, 20].

The piezoelectric coupled equations of state in matrix notation with the piezoelectric coupling matrix are used in FEM calculations to describe the piezoelectric properties [21]. It is shown in Eq. 1 and connects the mechanical stress tensor T as well as the electrical stress tensor D with the mechanical strain S and the electrical field E:

$$\begin{gathered} T = c^{E} S - eE \hfill \\ D= eS + \varepsilon^{S} E. \hfill \\ \end{gathered}$$
(1)

Here, cE is the elastic stiffness matrix at constant electric field E. The clamped dielectric matrix εS describes the charge displacement in piezoelectric materials in an electric field E at constant mechanical strain S [21].

The structural and quasi-electrostatic fields are coupled in piezoelectric analyses by the piezoelectric constant e according to Eq. 2 with Rayleigh damping DR, dielectric dissipation DF, mass M, force F and negative electric charge Q:

$$\left[\begin{array}{cc}{c}^{E} & e\\ e& {-\varepsilon }^{S}\end{array}\right]\left\{\begin{array}{c}S\\ D\end{array}\right\}+\left[\begin{array}{cc}{D}_{R}& 0\\ 0& -{D}_{F}\end{array}\right]\left\{\begin{array}{c}\dot{S}\\ \dot{D}\end{array}\right\}+\left[\begin{array}{cc}M& 0\\ 0& 0\end{array}\right]\left\{\begin{array}{c}\ddot{S}\\ \ddot{D}\end{array}\right\}=\left\{\begin{array}{c}F\\ Q\end{array}\right\}.$$
(2)

To define the anisotropic material behavior of PZT8 in ANSYS, a symmetric 6 × 6 matrix of stiffnesses cE is assigned to the piezoelectric elements via the APDL. The piezoelectric coefficients e and the dielectric coefficients εS are inserted via the PiezoAndMEMS extension using piezoelectric bodies. The density of the piezo-elements is defined in the material data set.

The coefficients of the mechanical stiffness, piezoelectric and dielectric matrices are temperature-dependent and have been experimentally determined for different piezoelectric materials by various scientists using the resonant ultrasound spectroscopy RUS [22,23,24], the resonance and anti-resonance method [25,26,27,28,29,30,31] and the quasi-static method [25].

A complete set of temperature-dependent coefficients in the e-form could be determined by Tang et al. for PZT4 [22, 23] and by Zhang et al. for PZT8 [24] using RUS.

According to the literature, the dielectric coefficients ε in PZT increase with increasing temperature [22,23,24,25,26]. The piezoelectric coefficients e and the stiffness coefficients cE also increase in piezoelectric materials with the increase in temperature [22,23,24]. No temperature-dependent data are available for the density ρ of PZT8, which is 7750 kg/m3 [32].

The functions of the temperature-dependent material properties of PZT8 obtained by experimental investigations of other scientists are listed in Table 2.

Table 2 Material coefficients of the piezo-material PZT8 as a function of the temperature in °C and their percentage change from 30 °C to 80 °C [21, 24, 32]
Full size table

Metallic Materials

The metallic components made of structural steel S235, the titanium alloy Ti6Al4V and copper Cu have an isotropic material behavior. To describe the isotropic material model, the material coefficients Young’s modulus Y, Poisson’s ratio ν and density ρ are required.

The material coefficients of the titanium alloy Ti6Al4V, structural steel S235 and copper Cu are temperature-dependent. Within their work, Bartsch et al. carried out an extensive literature search of experimental work with temperature-dependent material coefficients of the titanium alloy Ti6Al4V, summarized them and formed functions from them (see Table 3) [33]. The works of Chen et al. [34] and Savas [35] contain tables for all temperature-dependent material coefficients of the structural steel S235 required in the isotropic material model. The linear functions shown in Table 4 are approximated to these. Temperature-dependent values for the Young’s modulus and Poisson’s ratio of copper are collected from various other works by Ledbetter et al. [36]. In their work, Cahill et al. present date for the temperature-dependent density of copper [37]. Using the data for Young’s modulus, Poisson’s ratio and density of copper at different temperatures linear temperature-dependent functions are formed, which can be seen in Table 5.

Table 3 Material coefficients of the titanium alloy Ti6Al4V as a function of the temperature in °C and their percentage change from 30 °C to 80 °C [33]
Full size table
Table 4 Material coefficients of structural steel S235 as a function of the temperature in °C and their percentage change from 30 °C to 80 °C [34, 35]
Full size table
Table 5 Material coefficients of copper Cu as a function of temperature in °C and their percentage change from 30 °C to 80 °C [36, 37]
Full size table

For the metallic materials considered, the Young’s moduli Y and the densities ρ decrease with increasing temperature, while the Poisson’s ratios ν increase. From 30 °C to 80 °C, the Young’s moduli decrease by up to 4.72% and the densities by up to 0.33%. The Poisson’s ratios increase by up to 1.54% in the temperature range mentioned [33,34,35,36,37].

Damping

The energy dissipation of vibrations, for example due to losses in the material and joints, is defined in FEM calculations via damping [2].

A widely used damping model is the Rayleigh damping from Eq. 3. With the mass matrix M and the stiffness matrix C, the Rayleigh damping DR is divided into a mass and a stiffness-proportional component [2]:

$${D}_{R}=\alpha \underline{M}+\beta \underline{C}.$$
(3)

The damping of piezoelectric materials or Langevin transducers can be determined by taking into account the quality factor Qm and the frequency ω. The stiffness-proportional component of the Rayleigh damping β is calculated according to Eq. 4 [2]:

$$\beta = \frac{1}{{Q_{m} \omega }}.$$
(4)

Viscous damping normally occurs in piezoelectric transducers. For these, α = 0 applies to the mass-proportional component of the Rayleigh damping [2].

The quality factor Qm can be approximately determined from the experimental frequency response H(ω) with the resonance frequency ωr and the two frequencies ω1 and ω2 using the 3 dB method according Eq. 5 [38, 39]:

$$Q_{m} = \frac{{\omega_{r} }}{{\omega_{2} - \omega_{1} }}$$
(5)

with \(\left| {H\left( {\omega_{1} } \right)} \right| = \left| {H\left( {\omega_{2} } \right)} \right| = \sqrt{\frac{1}{2}} \left| {H\left( {\omega_{r} } \right)} \right|.\)

This approximation provides the quality factor Qm with a deviation of up to 20% [38].

The experimentally determined stiffness-proportional component of the Rayleigh damping β averaged over the six types is 1.5E−8.

Contacts

In the harmonic analysis, the linear contact formulation “no separation” is used, which does not allow the contact pairs to separate in normal direction while tangential movements are allowed. The chosen contact algorithm is the augmented Lagrangian method. The contact surfaces can move freely in the tangential direction. The threaded connection between the horn and the screw is assumed to be a bonded contact where the contact surfaces are firmly connected in all directions.

The contact stiffness is expressed by the normal stiffness factor FKN. For bulk deformation, a FKN of 1 is used [40].

The contact elements are the 2D 3 node surface-to-surface contact elements CONTA172 and the associated target surface elements TARGE169.

Boundary Conditions

The Langevin transducers T1 and S1 are constrained in their center with a fixed support applied to the outer line of the copper electrode. The Langevin transducers with horn S2, S3, T2 and T3 are constrained on the support ring and the central ring of the horn, since they are supported at these parts in the experiments.

The voltage on the piezo-elements is applied via the boundary condition Voltages on the surfaces of the piezo-elements are opposite to each other in the axial direction. A voltage with a real value of 14.14 V is applied to one of the two surfaces corresponding to 10 V RMS. The opposite surface has a voltage of 0 V.

Solver

The solution method is harmonic with full matrices. With this method, the electrical voltage applied to the piezo-elements can be taken into account. In addition, it offers a higher accuracy than modal superposition, which is the alternative method available in ANSYS.

Meshing

The metallic components are meshed with higher order 2D 6-node and 8-node elements PLANE183, which have a quadratic displacement behavior. The piezo-ceramics are meshed with 8-node 2D elements PLANE223, which have piezoelectric capabilities. For curvatures, mesh nodes are generated every 15°. The element size considered in the convergence analysis refers to the maximal allowed element size. The growth rate between the refined curvatures and the maximum element size is 1.2.

The meshing of type T3 with maximum element sizes from 5 mm to 0.75 mm are shown exemplary in Fig. 8 (the colors distinguish the individual materials of the Langevin transducer, light-grey: Ti6Al4V/S235, grey: S235, orange: PZT8, blue: Cu). The other types are meshed with the same element sizes for the convergence analysis. The resonance frequency of the impedance curves converges with increasing number of elements for all types. Figure 9 shows the resonance frequency of the impedance curves of type T3 as an example. Convergence is achieved when the frequency shift is less than 21 Hz. The frequency shift between the element sizes 1.25 mm (1207 elements) and 0.75 mm (2046 elements) is 20 Hz, which indicates convergence. In percentage terms, the frequency shift is less than 0.06%.

Fig. 8
figure 8

a 3D image of rotary symmetry of Langevin transducer type T3 with (1) screw M8 × 65, (2) washer, (3) end mass, (4) electrode, (5) support ring, (6) piezo-ceramic PZT8, (7) stepped horn; b 2D meshing of the type T3 with the maximum element sizes 5 mm, 2 mm, 1.25 mm and 0.75 mm

Full size image
Fig. 9
figure 9

Convergence of resonance frequency of T3

Full size image

Procedure for Describing the Temperature-Dependent Resonance Frequency in FEM Calculations

The procedure for describing the temperature influence on the resonance frequency in FEM calculations is shown in Fig. 10.

Fig. 10
figure 10

Method to fit a FEM model to experimental data in 2 steps (Optimization 1, Optimization 2) based on a parameter correlation and a FEM base model with measured temperature-dependent material data

Full size image

First, a FEM base model is formed from measured temperature-dependent functions of the material coefficients.

The resonance frequency calculated with the base model at a constant temperature of 30 °C is compared with the experiments. If the deviation is more than 0.5% (approx. 175 Hz), the functions of the material coefficients from Tables 2, 3, 4 and 5 with influence on the resonance frequency are modified in optimization step 1. For this purpose, the material coefficients with influence on the resonance frequency are first identified in a parameter correlation. Their functions are then optimized so that the deviation of the calculated resonance frequency from the experiments at 30 °C is minimized.

In the second optimization step, the deviation of the calculated resonance frequency change from 30 °C to 80 °C to the experiments is minimized.

FEM Base Model

The measured temperature-dependent material coefficients described in Tables 2, 3, 4 and 5 are defined as functions in ANSYS in order to simulate the temperature dependence of the Langevin transducers using the FEM.

Parameter Correlation

The influence of the dielectric coefficients εS, the piezoelectric coefficients e and the stiffnesses cE of the piezoelectric material as well as the density ρ, the Young’s modulus Y and the Poisson’s ratio ν of the metallic materials on the resonance frequency in FEM calculations is investigated by means of a parameter correlation. The material coefficients are varied according to the maximum change in the temperature range from 30 °C to 80 °C described in Tables 2, 3, 4 and 5. There is no temperature-dependent function for the density of the piezo-material PZT8. In order to examine the influence of the density of the piezo-material on the resonance frequency, the percentage change in density from 30 °C to 80 °C is assumed to be 0.33% in the parameter correlation.

The Spearman correlation coefficient is used to describe the relationships between the material coefficients and the resonance frequencies. It evaluates the monotonic association of two parameters on a scale from -1 to 1. If it is positive, the parameters behave in the same direction. If it is negative, they behave in opposite directions. If the value of the Spearman coefficient is < 0.1, the correlation between the parameters is negligible. If it is > 0.9, the correlation is very strong. Between 0.1 and 0.9, the Spearman coefficient can be divided into different levels from weak to strong, whereby their boundaries should be interpreted as indistinct. A low correlation also means that the parameter under consideration depends on other parameters [41].

Preliminary tests and examination of the relationships between the parameters during the correlation confirm the monotonic relationship of the examined parameters.

Optimization 1: Resonance Frequency at Constant Temperature of 30 °C

Within the optimization, the functions of the material coefficients from Tables 2, 3 and 4 are adjusted or new linear functions are assigned to them so that the results of the simulation model are fitted to the experimental data.

The aim is to test whether it is possible to optimize the model by describing the stiffness using linear functions while at the same time reducing its complexity.

The fitted functions of the material coefficients ffit(T) are structured as in Eq. 6. Parameter b30°C is the material coefficient at 30 °C and parameter a is the linear change of the material coefficient with a temperature change:

$${f}_{fit}\left(T\right)={b}_{30^\circ C}+a \left(T-30^\circ C\right).$$
(6)

The aim of optimization 1 is to modify the material parameters b30°C is such a way that the resonance frequencies of the types T1, T2, S1 and S2 from the FEM calculations approximate the tests to below 175 Hz.

Optimization 2: Temperature Dependence of the Resonance Frequency from 30 °C to 80 °C

The aim of optimization 2 is to determine the parameter a, which describes the linear change of the material coefficients with change of temperature. For this purpose, parameter a is modified in such a way that the average deviation of the resonance frequency gradient between 30 °C and 80 °C from the FEM calculation to that from the experiments is minimized.

In the optimization, the material coefficients are modified with which the resonance frequency shows a correlation with a Spearman coefficient greater than 0.2. The optimizations are carried out with the types T1, T2, S1 and S2. The linear functions obtained from the optimization are applied to the types T3 and S3 and validated with the experiments.

To describe the dependence of the resonance frequency on the material coefficients, response surfaces are formed for each optimization step. They are based on multiple FEM calculations of samples with different values of the coefficients generated by a Design of Experiment DoE method. Optimal space-filling with the design type maximum entropy is chosen as the DoE method [42]. The advantage of space-filling designs over classical DoE methods such as central composite design is that the samples are distributed more densely over the entire design space. Simulative computer experiments more often exhibit systematic error than random error, which is why a densely filled design space yields better results [43]. The response surface algorithm is Genetic Aggregation, which automatically selects, configures and creates the most appropriate type of response surface for each output parameter [42].

On the response surfaces created, the resonance frequency is described mathematically as a function of the selected material coefficients. Thus, the optimization can be carried out without further, time-consuming FEM calculations. The accuracy of the response surfaces is checked with validation points.

Multi-Objective Genetic Algorithm MOGA is chosen as the optimization algorithm, which can take several objectives into account [42]. This is necessary due to the consideration of the four resonance frequencies of the shapes S1, S2, T1 and T2 as objectives.

Results

The results of the FEM calculations with the base model, the parameter correlation and the calculations with the fitted model are listed below.

FEM Base Model

In the FEM base model, the temperature dependence of the resonance frequency is described by the known functions from the Tables 2, 3, 4 and 5.

A comparison of the temperature-dependent resonance frequencies of the types made of Ti6Al4V (T1–3) calculated by the FEM base model with the experiments is shown in Fig. 11.

Fig. 11
figure 11

Temperature-dependent resonance frequencies of the types T1–T3 from experiment (Exp) and FEM calculation with the base model (Base)

Full size image

The gradients of the functions in the test and FEM calculation show similarities, especially for the type T1. The values of the resonance frequencies at 30 °C deviate from each other by up to 928 Hz, so that there is a shift of the functions on the ordinate. This shows that the FEM base model can qualitatively represent the temperature behavior of Ti6Al4V, but the quantitative values deviate.

The comparison of the temperature-dependent resonance frequencies of the types made of S235 (4–6) calculated by the FEM base model with the experiments is shown in Fig. 12.

Fig. 12
figure 12

Temperature-dependent resonance frequencies of the types S1–S3 from experiment (Exp) and FEM calculation with the base model (Base)

Full size image

In contrast to the experiment, the FEM calculations of the types S1–S3 with the base model show no significant change in the resonance frequency with temperature. Quantitatively, the types S1–S3 show a deviation of the resonance frequency at 30 °C up to 310 Hz.

This shows that the functions of the material coefficients for S235 on which the base model is based deviate from the material characteristics of the real material both in their temperature behavior and in their value at 30 °C.

Parameter Correlation

The resonance frequencies and their temperature dependence calculated with the FEM base model deviate from the test results. To minimize the deviation, the material coefficients with an influence on the resonance frequency are determined using a parameter correlation. Afterward, these are specifically modified in optimization steps 1 and 2.

The Spearman correlation of the material coefficients with the resonance frequencies of the different types is shown in Fig. 13.

Fig. 13
figure 13

Spearman correlation of material coefficients with the resonance frequency for shapes T1–3 and S1–3

Full size image

The resonance frequencies show a correlation with the stiffness cE11, cE13 and cE33 of the piezo-material. The correlations of cE13 and cE33 are high, up to 0.68 and 0.58, respectively. The correlation coefficient of cE11 is significantly lower at up to 0.22.

The correlation of cE13 with the resonance frequency for the types with horns S2, S3, T2 and T3 is opposite, while their correlation with the stiffnesses cE33 and cE11 is the same direction. From the literature data, it appears that the stiffnesses change similarly with a change in temperature. Thus, their influence on the resonance frequency of the Langevin transducer with horn is balanced out, so that overall they are only slightly dependent on the temperature behavior of the piezoelectric material.

For the types without a horn, the stiffnesses cE11, cE13 and cE33 behave in the same direction as the resonance frequency. Therefore, the influence of the temperature behavior of the piezoelectric material on these shapes is greater.

The resonance frequency shows a strong correlation of up to 0.82 with the Young’s moduli of the end masses and horns. The resonance frequency behaves in the same direction as the Young’s moduli for all types.

The resonance frequency shows no or a negligible correlation to the other material coefficients.

Fitted Functions of the Temperature-Dependent Material Coefficients

The temperature-dependent material coefficients are optimized based on the function described in Eq. 6.

The fitted functions of the material coefficients with influence on the resonance frequency are shown in Table 6. The other functions of the material coefficients are not changed and are consistent with Tables 2, 3, 4 and 5.

Table 6 Fitted material coefficients obtained as a function of temperature in °C
Full size table

The results of the two optimization steps for the model fit are described below.

Optimization 1: Resonance Frequency at Constant Temperature of 30 °C

Compared to the FEM calculations with the base model, the calculated resonance frequencies at 30 °C with the fitted model are closer to those of the experiments. This applies in particular for the types T1 and S1, where the deviation of the resonance frequency at 30 °C is 5 Hz and 6 Hz, respectively (see Fig. 14). The resonance frequencies of the types T2 and S2 at 30 °C calculated with the fitted model also show a small deviation of 22 and 21 Hz with respect to the experiments. For the types T3 and S3, which were not considered in the optimization, the deviation between the FEM calculation with the fitted model and the experiments are somewhat higher. They are 166 Hz for T3 and 105 Hz for S3. Compared to the results with the base model, the resonance frequencies calculated with the fitted model are 134 Hz to 906 Hz closer to the experimental results. This corresponds to a reduction of the average deviation of the resonance frequency from 1.5% (base model) to 0.2% (fitted model) with respect to the experiments.

Fig. 14
figure 14

Deviation of the resonance frequencies at 30 °C of the types T1–3 and S1–3 between the experiments and the FEM calculation with the base model (Base) and the fitted model (Fit) in Hz

Full size image

In Fig. 15, the impedance curves of type T1 at 30 °C are plotted against the frequency from the test and the FEM calculation with the base model and the fitted model in the range of the resonance frequency. An improvement of the results with the fitted model in relation to the base model can be seen. Overall, the impedance curves of all types at 30 °C can be described well with the fitted model.

Fig. 15
figure 15

Comparison of the frequency response of impedance of the type T1 at 30 °C between experiment (Exp) and the FEM calculation with the base model (Base) and the fitted model (Fit)

Full size image

Optimization 2: Temperature Dependence of the Resonance Frequency from 30 °C to 80 °C

The comparison of the temperature-dependent resonance frequencies between the experiment and the FEM calculation with the fitted model is shown for the types made of Ti6Al4V (T1–3) in Fig. 16 and for the types made of S235 (S1–3) in Fig. 17. The temperature dependence of the types T2, T3 and S1 in the range from 30 °C to 50 °C can be described well by the fitted model. In this range, the average deviation of the resonance frequency temperature gradient is 10%.

Fig. 16
figure 16

Temperature-dependent resonance frequencies of types T1–T3 from experiment (Exp) and FEM calculation with the fitted model (Fit)

Full size image
Fig. 17
figure 17

Temperature-dependent resonance frequencies of types S1–S3 from experiment (Exp) and FEM calculation with the fitted model (Fit)

Full size image

Figure 18 shows the comparison of the temperature gradients of the resonance frequencies between 30 °C and 80 °C for types T1–3 and S1–3 in the experiments and the calculations with the base model and the fitted model.

Fig. 18
figure 18

Comparison of the temperature gradients of the resonance frequency in Hz/°C between 30 °C and 80 °C for the types T1–3 and S1–3 between the experiments (Exp), the FEM calculations with the base model (Base) and the fitted model (Fit)

Full size image

The fitted model shows a uniform temperature-dependent behavior of the types S1–3 made of S235 which can be compared to the experiments. The calculations with the base model do not show a uniform temperature-dependent behavior of the types S1–3. In addition, the calculated temperature gradients of the resonance frequencies with this model are very small.

In comparison with the FEM calculations with the base model, the temperature gradients of the resonance frequencies calculated with the fitted model in the range from 30 °C to 80 °C have come closer to the tests for all shapes except T1 (see Fig. 17). They are in the range from − 2.6 Hz/°C (S1) to − 13.2 Hz/°C (T3).

The temperature gradient of the resonance frequency of types S1-3, which is not represented by the base model (deviation 106%), can be determined by the fitted model with an average deviation of 42%. The average deviation of the resonance frequency temperature gradient of the types T1-3 can be reduced from 40 to 35% by the fitted model.

The fitted model has a geometry and material-dependent temperature gradient of the resonance frequency. Within the same material, type 1 has the lowest gradients and type 3 has the highest gradients. The resonance frequencies of the type T made of Ti6Al4V show a higher dependency with the temperature than the type S made of S235.

Conclusion

Experiments show that the resonance frequency of Langevin transducers decreases with increasing temperature. The temperature-dependent reduction as well as the magnitude of the resonance frequency depends on the geometry and the material of the transducers.

The aim of this contribution is to reproduce this experimentally determined temperature dependence as accurately as possible with the FEM simulation.

A first FEM model does not calculate the results satisfactorily in our temperature and frequency range.

Therefore, a method is developed to analytically optimize the existing material data, respectively, its coefficients.

In the first step, a parameter correlation is performed to determine the coefficients with the largest influences on the resonant frequency change.

In the second step, the temperature-dependent functions describing the coefficients are optimized using the FEM. For this purpose, a specific method is developed which is based on the Multi-Objective Genetic Algorithm MOGA.

The FEM calculations with these methodically optimized functions of the material coefficients show an improved agreement with the experimental data.

With the fitted functions, the resonance frequency of all examined types at a temperature range from 30 °C to 80 °C can be determined with an average deviation of 0.13% for Steel (S235) and 0.39% for Titanium (Ti6Al4V).

In subsequent work, it can be examined whether a further improvement of the results can be achieved by means of this approach and using higher order functions to describe the temperature-dependent resonance behavior.

The shown optimization method using ANSYS FEM can be used by other scientists to obtain complex material coefficients which are difficult to measure directly.