Bond-Based Peridynamics with Stretch and Rotation Kinematics for Opening and Shearing Modes of Fracture

Abstract

This study presents a new bond-based peridynamic approach for modeling the elastic deformation of isotropic materials with bond stretch and rotation, thus removing the constraint on the Poisson’s ratio. The resulting PD equilibrium equations derived under the assumption of small deformation are solved by employing implicit techniques. The bond constants associated with stretch and rotation kinematic are directly related to the constitutive relations of stress and strain components in continuum mechanics. Also, the expressions for the critical stretch and critical relative rotation are derived in terms of mode I and mode II critical energy release rates, respectively. Lastly, it does not require a surface correction procedure, and the displacement and traction type boundary conditions are directly imposed without introducing fictitious regions in the domain. The capability of this approach is first demonstrated by capturing the correct deformation of plate type structures under general loading conditions. Subsequently, its capability for failure prediction is established by simulating the response of a double cantilever beam (DCB) under mode I type loading and compact shear specimen under mode II type loading.

Appendices

Appendix 1

Peridynamic Functions

For three dimensional analysis, the matrix ${\mathbf{G}}({{\varvec{\upxi}}})$ and vector ${\mathbf{g}}({{\varvec{\upxi}}})$ are defined as

$${\mathbf{G}} = \left[ {\begin{array}{*{20}c} {g_{2}^{200} ({{\varvec{\upxi}}})} & {g_{2}^{110} ({{\varvec{\upxi}}})} & {g_{2}^{101} ({{\varvec{\upxi}}})} \\ {g_{2}^{110} ({{\varvec{\upxi}}})} & {g_{2}^{020} ({{\varvec{\upxi}}})} & {g_{2}^{011} ({{\varvec{\upxi}}})} \\ {g_{2}^{101} ({{\varvec{\upxi}}})} & {g_{2}^{011} ({{\varvec{\upxi}}})} & {g_{2}^{002} ({{\varvec{\upxi}}})} \\ \end{array} } \right]$$

(149)

and

$${\mathbf{g}} = \left\{ {\begin{array}{*{20}c} {g_{2}^{100} ({{\varvec{\upxi}}})} \\ {g_{2}^{010} ({{\varvec{\upxi}}})} \\ {g_{2}^{001} ({{\varvec{\upxi}}})} \\ \end{array} } \right\}$$

(150)

where the components of matrix ${\mathbf{G}}({{\varvec{\upxi}}})$ and the vector ${\mathbf{g}}({{\varvec{\upxi}}})$ are explicitly given by Madenci et al. [25, 26]. Their derivation is based on the PD differential operator, and they only depend on the spatial relation of the material points in the family of point, ${\mathbf{x}}$. The superscripts denote the order of differentiation with respect to the variable $x_{{{i}}}$ with ${{i}} = 1,2,3$ and the subscript 2 represents the order of Taylor Series Expansion (TSE) in the construction of the PD functions.

For 2D analysis, they reduce to

$${\mathbf{G}} = \left[ {\begin{array}{*{20}c} {g_{2}^{20} ({{\varvec{\upxi}}})} & {g_{2}^{11} ({{\varvec{\upxi}}})} \\ {g_{2}^{11} ({{\varvec{\upxi}}})} & {g_{2}^{02} ({{\varvec{\upxi}}})} \\ \end{array} } \right]$$

(151)

and

$${\mathbf{g}} = \left\{ {\begin{array}{*{20}c} {g_{2}^{10} ({{\varvec{\upxi}}})} \\ {g_{2}^{01} ({{\varvec{\upxi}}})} \\ \end{array} } \right\}$$

(152)

The PD functions can be analytically evaluated if a material point is located symmetrically at the center of a circular interaction domain.

For 3D analysis, the matrix ${\mathbf{G}}({{\varvec{\upxi}}})$ and the vector ${\mathbf{g}}({{\varvec{\upxi}}})$ can be evaluated as

$${\mathbf{G}} = \frac{15}{{\pi \delta^{7} }}w_{2} (\left| {{\varvec{\upxi}}} \right|)({{\varvec{\upxi}}} \otimes {{\varvec{\upxi}}}) - \frac{3}{{\pi \delta^{7} }}w_{2} (\left| {{\varvec{\upxi}}} \right|)({{\varvec{\upxi}}} \cdot {{\varvec{\upxi}}}){\mathbf{I}}$$

(153)

$$\text{tr}{\mathbf{G}} = \frac{6}{{\pi\delta^{7} }}w_{2} (\left| {{\varvec{\upxi}}} \right|)({{\varvec{\upxi}}} \cdot {{\varvec{\upxi}}})$$

(154)

$${\mathbf{g}} = \frac{9}{{4\pi \delta^{5} }}w_{1} (\left| {{\varvec{\upxi}}} \right|){{\varvec{\upxi}}}$$

in which the weight function is specified as

$$w_{n} (|{{\varvec{\upxi}}}|) = \frac{{\delta^{n + 1} }}{{\left| {{\varvec{\upxi}}} \right|^{n + 1} }}$$

(155)

With the substitution of the weight function, the PD representation of $({\mathbf{n}} \otimes {\mathbf{n}})$ and $({\mathbf{I}} - {\mathbf{n}} \otimes {\mathbf{n}})$ can be derived as

$$\left( {{\mathbf{n}} \otimes {\mathbf{n}}} \right) = \frac{{\pi \delta^{4} }}{30}\left( {{\text{tr}}{\mathbf{GI}} + 2{\mathbf{G}}} \right)\left| {{\varvec{\upxi}}} \right|$$

(156)

and

$$\left( {{\mathbf{I}} - \left( {{\mathbf{n}} \otimes {\mathbf{n}}} \right)} \right) = \frac{{\pi \delta^{4} }}{15}\left( {{\text{2tr}}{\mathbf{GI}} - {\mathbf{G}}} \right)\left| {{\varvec{\upxi}}} \right|$$

(157)

For 2D analysis, the matrix ${\mathbf{G}}({{\varvec{\upxi}}})$ and the vector ${\mathbf{g}}({{\varvec{\upxi}}})$ can be evaluated as

$${\mathbf{G}}= \frac{12}{{\pi h\delta^{6} }}w_{2} (\left| {{\varvec{\upxi}}} \right|)({{\varvec{\upxi}}} \otimes {{\varvec{\upxi}}}) - \frac{3}{{\pi h\delta^{6} }}w_{2} (\left| {{\varvec{\upxi}}} \right|)({{\varvec{\upxi}}} \cdot {{\varvec{\upxi}}}){\mathbf{I}}$$

(158)

$$\text{tr}{\mathbf{G}} = \frac{6}{{\pi h\delta^{6} }}w_{2} (\left| {{\varvec{\upxi}}} \right|)({{\varvec{\upxi}}} \cdot {{\varvec{\upxi}}})$$

(159)

and

$${\mathbf{g}} = \frac{2}{{\pi h\delta^{4} }}w_{1} (\left| {{\varvec{\upxi}}} \right|){{\varvec{\upxi}}}$$

(160)

The PD representation of $({\mathbf{n}} \otimes {\mathbf{n}})$ and $({\mathbf{I}} - {\mathbf{n}} \otimes {\mathbf{n}})$ can be derived as

$$\left( {{\mathbf{n}} \otimes {\mathbf{n}}} \right) = \frac{{\pi h\delta^{3} }}{24}\left( {{\text{tr}}{\mathbf{GI}} + 2{\mathbf{G}}} \right)\left| {{\varvec{\upxi}}} \right|$$

(161)

and

$$\left( {{\mathbf{I}} - \left( {{\mathbf{n}} \otimes {\mathbf{n}}} \right)} \right) = \frac{{\pi h\delta^{3} }}{24}\left( {{\text{3tr}}{\mathbf{GI}} - 2{\mathbf{G}}} \right)\left| {{\varvec{\upxi}}} \right|$$

(162)

Appendix 2

Balance Laws

Based on the identity $({\mathbf{a}} \otimes {\mathbf{b}}){\mathbf{c}} = ({\mathbf{b}} \times {\mathbf{c}}){\mathbf{a}}$ along with $\left( {{\mathbf{n}} \otimes {\mathbf{n}}} \right){{\varvec{\upomega}}}({\mathbf{x}}){\mathbf{n}} = 0$, the force density vector given in Eq. (26) can be expressed as

$$\mathbf {f(u - u^{\prime},x^{\prime} - x) }= (c - d)\mathbf{\frac{1}{{\left| {{\varvec{\upxi}}} \right|}}\left( {(u(x^{\prime}) - u(x)) \cdot n} \right)n} + d\mathbf{\frac{1}{{\left| {{\varvec{\upxi}}} \right|}}\left( {(u(x^{\prime}) - u(x)) - {{\varvec{\upomega}}}(x){{\varvec{\upxi}}}} \right)}$$

(163)

With ${\mathbf{u}}({\mathbf{x^{\prime}}}) - {\mathbf{u}}({\mathbf{x}}) = ({\mathbf{y^{\prime}}} - {\mathbf{y}}) - {{\varvec{\upxi}}}$ and ${{\varvec{\upomega}}}({\mathbf{x}}){{\varvec{\upxi}}} = \Omega \times {{\varvec{\upxi}}}$, this expression can be rewritten as

$$\mathbf{ f(u - u^{\prime},x^{\prime} - x)} = (c - d)\mathbf{\frac{1}{{\left| {{\varvec{\upxi}}} \right|}}\left( {(u(x^{\prime}) - u(x)) \cdot n} \right)n }+ d\mathbf{\frac{{(y^{\prime}(x^{\prime}) - y(x)) - {{\varvec{\upxi}}}}}{{\left| {{\varvec{\upxi}}} \right|}} - d\mathbf{{\kern 1pt} {{\varvec{\Omega}}}(x) \times n}}$$

(164)

where the rigid body rotation vector of the bond is defined as

$${{\varvec{\Omega}}}({\mathbf{x}}) = \left\{ {\begin{array}{*{20}c} {\omega_{32} } \\ {\omega_{13} } \\ {\omega_{21} } \\ \end{array} } \right\}$$

(165)

Invoking the assumption of small deformation, ${\mathbf{y^{\prime}}} - {\mathbf{y}} \approx \left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right|{\mathbf{n}}$, Eq. (164) reduces to

$$\mathbf{f(u - u^{\prime},x^{\prime} - x)} = (c - d)\mathbf{\frac{1}{{\left| {{\varvec{\upxi}}} \right|}}\left( {(u(x^{\prime}) - u(x)) \cdot n} \right)n} + d\mathbf{\frac{{\left| {y^{\prime} - y} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}n} - d\mathbf{{\kern 1pt} {{\varvec{\Omega}}}(x) \times n}$$

(166)

In accordance with Eq. (13), it can be recast as

$${\mathbf{f}}({\mathbf{u}} - {\mathbf{u^{\prime}}},{\mathbf{x^{\prime}}} - {\mathbf{x}}) = c\frac{{\left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}{\mathbf{n}} - d{\kern 1pt} {{\varvec{\Omega}}}({\mathbf{x}}) \times {\mathbf{n}}$$

(167)

or

$${\mathbf{f}}({\mathbf{u}} - {\mathbf{u^{\prime}}},{\mathbf{x^{\prime}}} - {\mathbf{x}}) = c\frac{{\left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}\frac{{({\mathbf{x^{\prime}}} - {\mathbf{x}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}} - d{\kern 1pt} {{\varvec{\Omega}}}({\mathbf{x}}) \times \frac{{({\mathbf{x^{\prime}}} - {\mathbf{x}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}$$

(168)

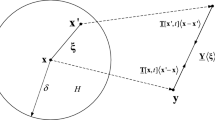
The linear momentum, ${\mathbf{L}}$, and angular momentum (about the coordinate origin), ${\mathbf{H}}_{0}$, of a fixed set of particles at time $t$ in volume $V$ are given by

$${\mathbf{L}} = \int\limits_{V} {\rho ({\mathbf{x}})\,{\dot{\mathbf{u}}}\left( {{\mathbf{x}},t} \right)dV}$$

(169)

and

$${\mathbf{H}}_{0} = \int\limits_{V} {{\mathbf{y}}({\mathbf{x}},t) \times \rho ({\mathbf{x}})\,{\dot{\mathbf{u}}}\left( {{\mathbf{x}},t} \right)dV}$$

(170)

The total force, ${\mathbf{F}}$, and torque, ${{\varvec{\Pi}}}_{0}$, about the origin can be expressed as

$${\mathbf{F}} = \int\limits_{V} {{\mathbf{b}}({\mathbf{x}},t)dV} + c\int\limits_{V} {\int\limits_{{H_{{\mathbf{x}}} }} {\frac{{\left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}\frac{{({\mathbf{x^{\prime}}} - {\mathbf{x}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}} \,dH_{{{\mathbf{x^{\prime}}}}} } dV - d\int\limits_{V} {{{\varvec{\Omega}}}({\mathbf{x}}) \times \int\limits_{{H_{{\mathbf{x}}} }} {{\mathbf{n}}\frac{{({\mathbf{x^{\prime}}} - {\mathbf{x}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}} \,dH_{{{\mathbf{x^{\prime}}}}} } dV$$

(171)

and

$$\begin{gathered} {{\varvec{\Pi}}}_{0} = \int\limits_{V} {{\mathbf{y}}({\mathbf{x}}) \times {\mathbf{b}}({\mathbf{x}})dV} + c\int\limits_{V} {{\mathbf{y}}({\mathbf{x}}) \times \int\limits_{{H_{{\mathbf{x}}} }} {\frac{{\left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}\frac{{({\mathbf{x^{\prime}}} - {\mathbf{x}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}} \,dH_{{{\mathbf{x^{\prime}}}}} } dV \hfill \\ \, - d\int\limits_{V} {{\mathbf{y}}({\mathbf{x}}) \times {{\varvec{\Omega}}}({\mathbf{x}}) \times \int\limits_{{H_{{\mathbf{x}}} }} {\frac{{({\mathbf{x^{\prime}}} - {\mathbf{x}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}} \,dH_{{{\mathbf{x^{\prime}}}}} } dV \hfill \\ \end{gathered}$$

(172)

in which the evaluation of the integral $\int\limits_{{H_{{\mathbf{x}}} }} {\mathbf{n}} \,dH_{{{\mathbf{x^{\prime}}}}} = 0$. Therefore, the total force and torque can be rewritten in the form

$${\mathbf{F}} = \int\limits_{V} {{\mathbf{b}}({\mathbf{x}},t)dV} + \frac{c}{2}\int\limits_{V} {\int\limits_{{H_{{\mathbf{x}}} }} {\frac{{\left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}\frac{{({\mathbf{x^{\prime}}} - {\mathbf{x}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}} \,dH_{{{\mathbf{x^{\prime}}}}} dV} - \frac{c}{2}\int\limits_{V} {\int\limits_{{H_{{\mathbf{x}}} }} {\frac{{\left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}\frac{{({\mathbf{x}} - {\mathbf{x^{\prime}}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}} \,dH_{{{\mathbf{x^{\prime}}}}} dV}$$

(173)

and

$$\begin{gathered} {{\varvec{\Pi}}}_{0} = \int\limits_{V} {{\mathbf{y}}({\mathbf{x}}) \times {\mathbf{b}}({\mathbf{x}})dV} + \frac{c}{2}\int\limits_{V} {{\mathbf{y}}({\mathbf{x}}) \times \int\limits_{{H_{{\mathbf{x}}} }} {\frac{{\left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}\frac{{({\mathbf{x^{\prime}}} - {\mathbf{x}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}} \,dH_{{{\mathbf{x^{\prime}}}}} dV} \hfill \\ \, - \frac{c}{2}\int\limits_{V} {{\mathbf{y}}({\mathbf{x}}) \times \int\limits_{{H_{{\mathbf{x}}} }} {\frac{{\left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}\frac{{({\mathbf{x}} - {\mathbf{x^{\prime}}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}} \,dH_{{{\mathbf{x^{\prime}}}}} dV} \hfill \\ \end{gathered}$$

(174)

Because ${\mathbf{f}}({\mathbf{x^{\prime}}} - {\mathbf{x}}\,) = {\mathbf{f}}({\mathbf{x}} - {\mathbf{x^{\prime}}}\,) = {\mathbf{0}}$ for ${\mathbf{x^{\prime}}} \notin H_{{\mathbf{x}}}$, these equations can be recast to include all of the material points in volume $V$ as

$${\mathbf{F}} = \int\limits_{V} {{\mathbf{b}}({\mathbf{x}},t)dV} + \frac{c}{2}\int\limits_{V} {\int\limits_{V} {\frac{{\left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}\frac{{({\mathbf{x^{\prime}}} - {\mathbf{x}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}} \,dV^{\prime}dV} - \frac{c}{2}\int\limits_{V} {\int\limits_{V} {\frac{{\left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}\frac{{({\mathbf{x}} - {\mathbf{x^{\prime}}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}dV^{\prime}dV} }$$

(175)

and

$$\begin{gathered} {{\varvec{\Pi}}}_{0} = \int\limits_{V} {{\mathbf{y}}({\mathbf{x}}) \times {\mathbf{b}}({\mathbf{x}})dV} + \frac{c}{2}\int\limits_{V} {{\mathbf{y}}({\mathbf{x}}) \times \int\limits_{V} {\frac{{\left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}\frac{{({\mathbf{x^{\prime}}} - {\mathbf{x}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}} \,dV^{\prime}dV} \hfill \\ \, - \frac{c}{2}\int\limits_{V} {{\mathbf{y}}({\mathbf{x}}) \times \int\limits_{V} {\frac{{\left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}\frac{{({\mathbf{x}} - {\mathbf{x^{\prime}}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}} \,dV^{\prime}dV} \hfill \\ \end{gathered}$$

(176)

If the parameters ${\mathbf{x}}$ and ${\mathbf{x^{\prime}}}$ in the second integral on the right-hand side of these equations are exchanged, these integrals become

$$\int\limits_{V} {\int\limits_{V} {\frac{{\left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}\frac{{({\mathbf{x}} - {\mathbf{x^{\prime}}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}dV^{\prime}dV} } = \int\limits_{V} {\int\limits_{V} {\frac{{\left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}\frac{{({\mathbf{x^{\prime}}} - {\mathbf{x}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}} \,dVdV^{\prime}}$$

(177)

and

$$\int\limits_{V} {{\mathbf{y}}({\mathbf{x}}) \times \int\limits_{V} {\frac{{\left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}\frac{{({\mathbf{x}} - {\mathbf{x^{\prime}}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}} \,dV^{\prime}dV = } \int\limits_{V} {{\mathbf{y}}({\mathbf{x^{\prime}}}) \times \int\limits_{V} {\frac{{\left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}\frac{{({\mathbf{x^{\prime}}} - {\mathbf{x}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}} \,dVdV^{\prime}}$$

(178)

Therefore, the total force and torque simplify to

$${\mathbf{F}} = \int\limits_{V} {{\mathbf{b}}({\mathbf{x}},t)dV}$$

(179)

and

$${{\varvec{\Pi}}}_{0} = \int\limits_{V} {{\mathbf{y}}({\mathbf{x}}) \times {\mathbf{b}}({\mathbf{x}})dV} - \int\limits_{V} {\left( {{\mathbf{y}}({\mathbf{x^{\prime}}}) - {\mathbf{y}}({\mathbf{x}})} \right) \times \int\limits_{V} {\frac{{\left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right| - \left| {{\varvec{\upxi}}} \right|}}{{\left| {{\varvec{\upxi}}} \right|}}\frac{{({\mathbf{x^{\prime}}} - {\mathbf{x}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}} \,dVdV^{\prime}}$$

(180)

Invoking the assumption of small deformation, ${\mathbf{y^{\prime}}} - {\mathbf{y}} \approx \left| {{\mathbf{y^{\prime}}} - {\mathbf{y}}} \right|{\mathbf{n}}$ and noting that $\int\limits_{V} {\mathbf{n}} \,dV = 0$, the expression for torque can be simplified as

$$\begin{gathered} {{\varvec{\Pi}}}_{0} = \int\limits_{V} {{\mathbf{y}}({\mathbf{x}}) \times {\mathbf{b}}({\mathbf{x}})dV} - \int\limits_{V} {\int\limits_{V} {\frac{{\left( {{\mathbf{y}}({\mathbf{x^{\prime}}}) - {\mathbf{y}}({\mathbf{x}})} \right) \times \left( {{\mathbf{y}}({\mathbf{x^{\prime}}}) - {\mathbf{y}}({\mathbf{x}})} \right)}}{{\left| {{\varvec{\upxi}}} \right|}}} \,dVdV^{\prime}} \hfill \\ \, + \int\limits_{V} {\left( {{\mathbf{y}}({\mathbf{x^{\prime}}}) - {\mathbf{y}}({\mathbf{x}})} \right) \times \int\limits_{V} {\frac{{({\mathbf{x^{\prime}}} - {\mathbf{x}})}}{{\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|}}} \,dVdV^{\prime}} \hfill \\ \end{gathered}$$

(181)

The balance of linear momentum, ${\dot{\mathbf{L}}} = {\mathbf{F}}$, and angular momentum, ${\dot{\mathbf{H}}}_{0} = {{\varvec{\Pi}}}_{0} ,$ results in

$$\int\limits_{V} {\left( {\rho ({\mathbf{x}}){\mathbf{\ddot{u}}}({\mathbf{x}},t) - {\mathbf{b}}({\mathbf{x}},t)} \right)dV} = 0$$

(182)

and

$$\int\limits_{V} {{\mathbf{y}}({\mathbf{x}}) \times \left( {\rho ({\mathbf{x}}){\mathbf{\ddot{u}}}({\mathbf{x}},t) - {\mathbf{b}}({\mathbf{x}},t)} \right)dV} = 0$$

(183)

Appendix 3

Matrices

For 3D analysis, the matrices appearing in Eq. (38) are defined as

$${\mathbf{M}} = \left[ {\begin{array}{*{20}c} {n_{1}^{4} } & {n_{1}^{2} n_{2}^{2} } & {n_{1}^{2} n_{3}^{2} } & {n_{1}^{3} n_{2}^{{}} } & {n_{1}^{2} n_{2}^{{}} n_{3}^{{}} } & {n_{1}^{3} n_{3}^{{}} } \\ {n_{1}^{2} n_{2}^{2} } & {n_{2}^{4} } & {n_{2}^{2} n_{3}^{2} } & {n_{1}^{{}} n_{2}^{3} } & {n_{2}^{3} n_{3}^{{}} } & {n_{1}^{{}} n_{2}^{2} n_{3}^{{}} } \\ {n_{1}^{2} n_{3}^{2} } & {n_{2}^{2} n_{3}^{2} } & {n_{3}^{4} } & {n_{1}^{{}} n_{2}^{{}} n_{3}^{2} } & {n_{2}^{{}} n_{3}^{3} } & {n_{1}^{{}} n_{3}^{3} } \\ {n_{1}^{3} n_{2}^{{}} } & {n_{1}^{{}} n_{2}^{3} } & {n_{1}^{{}} n_{2}^{{}} n_{3}^{2} } & {n_{1}^{2} n_{2}^{2} } & {n_{1}^{{}} n_{2}^{2} n_{3}^{{}} } & {n_{1}^{2} n_{2}^{{}} n_{3}^{{}} } \\ {n_{1}^{2} n_{2}^{{}} n_{3}^{{}} } & {n_{2}^{3} n_{3}^{{}} } & {n_{2}^{{}} n_{3}^{3} } & {n_{1}^{{}} n_{2}^{2} n_{3}^{{}} } & {n_{2}^{2} n_{3}^{2} } & {n_{1}^{{}} n_{2}^{{}} n_{3}^{2} } \\ {n_{1}^{3} n_{3}^{{}} } & {n_{1}^{{}} n_{2}^{2} n_{3}^{{}} } & {n_{1}^{{}} n_{3}^{3} } & {n_{1}^{2} n_{2}^{{}} n_{3}^{{}} } & {n_{1}^{{}} n_{2}^{{}} n_{3}^{2} } & {n_{1}^{2} n_{3}^{2} } \\ \end{array} } \right]$$

(184)

and

$${\mathbf{N}} = \left[ {\begin{array}{*{20}c} {n_{1}^{2} } & 0 & 0 & {\frac{{n_{1}^{{}} n_{2}^{{}} }}{2}} & 0 & {\frac{{n_{1}^{{}} n_{3}^{{}} }}{4}} \\ 0 & {n_{2}^{2} } & 0 & {\frac{{n_{1}^{{}} n_{2}^{{}} }}{2}} & {\frac{{n_{2}^{{}} n_{3}^{{}} }}{2}} & 0 \\ 0 & 0 & {n_{3}^{2} } & 0 & {\frac{{n_{2}^{{}} n_{3}^{{}} }}{2}} & {\frac{{n_{1}^{{}} n_{3}^{{}} }}{2}} \\ {\frac{{n_{1}^{{}} n_{2}^{{}} }}{2}} & {\frac{{n_{1}^{{}} n_{2}^{{}} }}{2}} & 0 & {\frac{{n_{1}^{2} + n_{2}^{2} }}{4}} & {\frac{{n_{1}^{{}} n_{3}^{{}} }}{4}} & {\frac{{n_{2}^{{}} n_{3}^{{}} }}{4}} \\ 0 & {\frac{{n_{2}^{{}} n_{3}^{{}} }}{2}} & {\frac{{n_{2}^{{}} n_{3}^{{}} }}{2}} & {\frac{{n_{1}^{{}} n_{3}^{{}} }}{4}} & {\frac{{n_{2}^{2} + n_{3}^{2} }}{4}} & {\frac{{n_{1}^{{}} n_{2}^{{}} }}{4}} \\ {\frac{{n_{1}^{{}} n_{3}^{{}} }}{2}} & 0 & {\frac{{n_{1}^{{}} n_{3}^{{}} }}{2}} & {\frac{{n_{2}^{{}} n_{3}^{{}} }}{4}} & {\frac{{n_{1}^{{}} n_{2}^{{}} }}{4}} & {\frac{{n_{1}^{2} + n_{3}^{2} }}{4}} \\ \end{array} } \right]$$

(185)

Their integration over a spherical interaction domain results in

$$\int\limits_{{V_{{\mathbf{x}}} }} {{\mathbf{M}}\left| {{\varvec{\upxi}}} \right|dV_{{{\mathbf{x^{\prime}}}}} } = \frac{{\pi \delta^{4} }}{15}\left[ {\begin{array}{*{20}c} 3 & 1 & 1 & 0 & 0 & 0 \\ 1 & 3 & 1 & 0 & 0 & 0 \\ 1 & 1 & 3 & 0 & 0 & 0 \\ 0 & 0 & 0 & 1 & 0 & 0 \\ 0 & 0 & 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 0 & 0 & 1 \\ \end{array} } \right]$$

(186)

and

$$\int\limits_{{V_{{\mathbf{x}}} }} {{\mathbf{N}}\left| {{\varvec{\upxi}}} \right|dV_{{{\mathbf{x^{\prime}}}}} } = \frac{{\pi \delta^{4} }}{6}\left[ {\begin{array}{*{20}c} 2 & 0 & 0 & 0 & 0 & 0 \\ 0 & 2 & 0 & 0 & 0 & 0 \\ 0 & 0 & 2 & 0 & 0 & 0 \\ 0 & 0 & 0 & 1 & 0 & 0 \\ 0 & 0 & 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 0 & 0 & 1 \\ \end{array} } \right]$$

(187)

For 2D analysis, these matrices reduce to

$${\mathbf{M}} = \left[ {\begin{array}{*{20}c} {n_{1}^{4} } & {n_{1}^{2} n_{2}^{2} } & {n_{1}^{3} n_{2}^{{}} } \\ {n_{1}^{2} n_{2}^{2} } & {n_{2}^{4} } & {n_{1}^{{}} n_{2}^{3} } \\ {n_{1}^{3} n_{2}^{{}} } & {n_{1}^{{}} n_{2}^{3} } & {n_{1}^{2} n_{2}^{2} } \\ \end{array} } \right]$$

(188)

and

$${\mathbf{N}} = \left[ {\begin{array}{*{20}c} {n_{1}^{2} } & 0 & {\frac{{n_{1}^{{}} n_{2}^{{}} }}{2}} \\ 0 & {n_{2}^{2} } & {\frac{{n_{1}^{{}} n_{2}^{{}} }}{2}} \\ {\frac{{n_{1}^{{}} n_{2}^{{}} }}{2}} & {\frac{{n_{1}^{{}} n_{2}^{{}} }}{2}} & \frac{1}{4} \\ \end{array} } \right]$$

(189)

Their integration over a circular interaction domain results in

$$\int\limits_{{V_{{\mathbf{x}}} }} {{\mathbf{M}}\left| {{\varvec{\upxi}}} \right|dV_{{{\mathbf{x^{\prime}}}}} } = \frac{{\pi h\delta^{3} }}{12}\left[ {\begin{array}{*{20}c} 3 & 1 & 0 \\ 1 & 3 & 0 \\ 0 & 0 & 1 \\ \end{array} } \right]$$

(190)

and

$$\int\limits_{{V_{{\mathbf{x}}} }} {{\mathbf{N}}\left| {{\varvec{\upxi}}} \right|dV_{{{\mathbf{x^{\prime}}}}} } = \frac{{\pi h\delta^{3} }}{6}\left[ {\begin{array}{*{20}c} 2 & 0 & 0 \\ 0 & 2 & 0 \\ 0 & 0 & 1 \\ \end{array} } \right]$$

(191)

Appendix 4

Relative Rotation Angle of Bonds

The relative rotation angle between any two bonds is derived by generalizing the skew angle concept introduced by Zhang and Qiao [5]. As illustrated in Fig. 27, the two bonds, ${\mathbf{\xi^{\prime}}} = {\mathbf{x^{\prime}}} - {\mathbf{x}}$ and ${\mathbf{\xi^{\prime\prime}}} = {\mathbf{x^{\prime\prime}}} - {\mathbf{x}}$, experience deformation and become ${\mathbf{y^{\prime}}} = {\mathbf{\xi^{\prime}}} + {\mathbf{u^{\prime}}}$ and ${\mathbf{y^{\prime\prime}}} = {\mathbf{\xi^{\prime\prime}}} + {\mathbf{u^{\prime\prime}}}$, respectively, in the deformed state. The unit vector ${\mathbf{N}}$ is perpendicular to the plane formed by bonds ${\mathbf{\xi^{\prime}}}$ and ${\mathbf{\xi^{\prime\prime}}}$. The unit vectors ${\mathbf{n'}}$ and ${\mathbf{t^{\prime}}}$, both in the plane formed by bonds ${\mathbf{\xi^{\prime}}}$ and ${\mathbf{\xi^{\prime\prime}}}$, are in the direction and perpendicular to ${\mathbf{\xi^{\prime}}}$, respectively.

Fig. 27

The relative rotation (skew) angle, $\gamma$ between bonds ${\mathbf{\xi^{\prime}}}$ and ${\mathbf{\xi^{\prime\prime}}}$ connected at material point x

The position of the bond, ${\mathbf{y^{\prime}}}$, can be described by

$${\mathbf{y^{\prime}}} = {\mathbf{R}}\,{\mathbf{\xi^{\prime}}}$$

(192)

in which ${\mathbf{R}}$ is the rotation matrix in the counterclockwise direction defined as

$${\mathbf{R}} = \left[ {\begin{array}{*{20}c} {\cos \varphi } & { - N_{3} \sin \varphi } & {N_{2} \sin \varphi } \\ {N_{3} \sin \varphi } & {\cos \varphi } & { - N_{1} \sin \varphi } \\ { - N_{2} \sin \varphi } & {N_{1} \sin \varphi } & {\cos \varphi } \\ \end{array} } \right]{ + }\left[ {\begin{array}{*{20}c} {N_{1} N_{1} } & {N_{1} N_{2} } & {N_{1} N_{3} } \\ {N_{1} N_{2} } & {N_{2} N_{2} } & {N_{2} N_{3} } \\ {N_{1} N_{3} } & {N_{2} N_{3} } & {N_{3} N_{3} } \\ \end{array} } \right](1 - \cos \varphi )$$

(193)

which reduces to

$${\mathbf{R}} = \left[ {\begin{array}{*{20}c} {\cos \varphi } & { - \sin \varphi } & 0 \\ {\sin \varphi } & {\cos \varphi } & 0 \\ 0 & 0 & 1 \\ \end{array} } \right]$$

(194)

for two-dimensional analysis with $N_{1} = N_{2} = 0$ and $N_{3} = 1$. The angle between ${\mathbf{\xi^{\prime}}}$ and ${\mathbf{y^{\prime}}}$ is defined as $\varphi$, and it can be determined from

$$\varphi = \cos^{ - 1} \left( {\frac{{\xi^{\prime} \times y^{\prime}}}{{\left| {\xi^{\prime}} \right|\left| {y^{\prime}} \right|}}} \right)$$

(195)

The position of bond ${\mathbf{y^{\prime\prime}}}$ with respect to the undeformed state can be obtained as

$${\mathbf{z^{\prime\prime}}} = {\mathbf{R}}^{T} \,{\mathbf{y^{\prime\prime}}}$$

(196)

As shown in Fig. 27, the relative rotation angle of bond, ${\mathbf{\xi^{\prime}}}$, with respect to ${\mathbf{\xi^{\prime\prime}}}$ can be expressed as

$$\gamma = \frac{{\left| {(z^{\prime\prime} - \xi^{\prime\prime}) \times n^{\prime}} \right|}}{{\left| {\xi^{\prime\prime} \times t^{\prime}} \right|}}$$

(197)