1 Introduction

In magnetized fusion plasmas, we often have a magnetic island produced by either intrinsic tearing instabilities (Furth et al. 1963; Wilson 1996) or by externally imposed magnetic field perturbations (Hahm and Kulsrud 1985; Fitzpatrick 1993; Waelbroeck 2009). The magnetic island primarily degrades the plasma confinement by parallel transport along the reconnected magnetic field lines and sometimes even leads to a plasma disruption (Wesson 1989; Boozer 2012; Strait 2019), but could serve positive roles in the magnetic fusion plasma operation. An important example is that the resonant magnetic perturbation (RMP)-induced suppression of edge localized modes (ELMs) in H-mode plasmas (Evans 2006), which enables sustainable H-mode operation, highly relies on the opening of a magnetic island near the pedestal top (Nazikian 2015; Hu 2019). Moreover, an interesting experimental observation (Ida 2004) suggests that a magnetic island can stimulate the transition to an enhanced confinement state with internal transport barrier formation (Hahm 2002; Wolf 2003). It is widely accepted that the leading mechanism of the transport barrier formation in magnetized fusion plasmas is the E\(\times\)B flow shear suppression of turbulent transport (Burrell 1997, 2020). The large scale E\(\times\)B flow here, characterized by flux function electrostatic potential \(\Phi (\psi )\) or equivalently radial electric field \(E_r \propto \Phi ' (\psi )\), stream on magnetic surfaces of a confined magnetized plasma, which Therefore does not induce transport by itself while shearing the turbulence eddies in a binormal direction (Biglari et al. 1990; Hahm and Burrell 1995; Terry 2000).

In general two classes of the E\(\times\)B shear flows are involved in the transition, the macroscale E\(\times\)B flow determined by the radial force balance, and the mesoscale E\(\times\)B flow self-generated from turbulence (Lin 1998, 2002; Diamond 2005; Itoh 2006). As long as the time increase of external heat input is moderate, the self-generation of the mesoscale zonal flows triggers, in other words, initiates the transition followed by the limit-cycle oscillation which is a representative signal of the turbulence-zonal flow interaction (Miki 2012; Schmitz 2017). Interestingly, extensive nonlinear gyrokinetic simulations studies of tokamak turbulence in the presence of a magnetic island have shown that E\(\times\)B vortex flows circulating on the magnetic island contours (Estrada 2016) could also be self-generated, showing close similarity to the zonal flows circulating on the magnetic surfaces in the nested tori configuration (Hornsby 2010; Navarro 2017; Kwon 2018; Fang and Lin 2019; Muto et al. 2022; Li 2023). Motivated by these findings, there has been recent theoretical progress on the time evolution of the self-generated vortex flows extending the zonal flow theories to the magnetic island geometry, identifying differences from the tokamak zonal flows rooted in the geometrical effect (Choi and Hahm 2022; Leconte and Cho 2023; Choi 2023). In this paper, we review the theoretical achievements and discuss their implications and impact on magnetic fusion energy research.

Theoretically, the self-generated E\(\times\)B vortex flow is defined as a perturbed electrostatic potential which is a flux function in the helical magnetic flux, i.e., a function depending on the helical magnetic flux only. In the vicinity of a magnetic island in a confined toroidal magnetized plasma, due to the difference in the magnetic topology from the usual nested tori magnetic field configuration, the proper magnetic surface label is the helical magnetic flux (Hazeltine and Meiss 2003)

$$\begin{aligned} \psi _h = \psi - \chi /q_s, \end{aligned}$$
(1)

where \(\psi\) is the poloidal magnetic flux typically used as a proper magnetic surface label in the confined toroidal fusion plasmas with nested tori magnetic configuration, \(\chi\) is the toroidal magnetic flux, and \(q_s\) is the safety factor (in the absence of the magnetic island) at the mode rational surface which corresponds to the magnetic island center. Recall that the original definition of the safety factor is \(q \equiv d\chi / d\psi\) (D’haeseleer et al. 1991) so that the helical magnetic flux \(\psi _h\) captures the difference in the pitch of the helical magnetic field line from the reference value. For convenience, we use a normalized helical magnetic flux

$$\begin{aligned} X = - \psi _h / \tilde{\psi }, \end{aligned}$$
(2)

where \(\tilde{\psi }\) is the amplitude of the magnetic island perturbation

$$\begin{aligned} \delta \psi = \tilde{\psi } \cos {(m\alpha )}, \end{aligned}$$
(3)

which is considered to be a constant in accordance with the constant-\(\psi\) approximation (Furth et al. 1963; Rutherford 1973) widely valid in realistic \(\beta\) and flow regimes (Fitzpatrick 2022). In Eq. (3), \(m\alpha = m\theta - n\zeta\) so that \(\alpha = \theta - \zeta /q_s\) is called helical angle, where \(\theta\) and \(\zeta\) are poloidal and toroidal angles, m and n are poloidal and toroidal mode numbers, and \(q_s = m/n\). For simplicity, we consider a circular-concentric background tokamak magnetic field, neglecting shaping and Shafranov shift. In this case, the magnetic surface label for the tokamak magnetic field is reduced to radial position r. Expanding \(\psi\) and \(\chi\) in r with respect to the mode rational surface \(r_s\) up to the second order, we obtain

$$\begin{aligned} X = \frac{2x^2}{w^2} - \cos {(ky)}, \end{aligned}$$
(4)

where \(x=r-r_s\) is the distance from the mode rational surface, \(w = 2(q_s \tilde{\psi } / \hat{s} B_\zeta )^{1/2}\) is the magnetic island half-width and \(\hat{s} = r_s q_s ' / q_s\) is the magnetic shear. Note that we have replaced the helical angle \(\alpha\) to a length variable y by \(m\alpha = ky\), where \(k = m/r_s\), to formally consider local Cartesian coordinates (x, y, z) in the vicinity of a magnetic island. Here, z is in the direction of the reference magnetic field (“guide field”). From Eq. (4), it is clear that \(X\in [-1,1]\) inside the magnetic island. Note that this normalized helical magnetic flux could be used outside (but still in the vicinity of) the magnetic island so that \(X \in [-1,\infty ]\) from the point of view of the ‘inner solution’. But in this study, we focus on the physics inside the magnetic island only.

In this paper, we show that this self-generated vortex flow evolves to a more complex flow structure, and it is convenient to use the eikonal representation as follows to study the long-term evolution.

$$\begin{aligned} \delta \phi (\textbf{x},t) = \phi (\textbf{x},t)e^{iS(\textbf{x})}. \end{aligned}$$
(5)

In gyrokinetics, we decompose the particle position \(\textbf{x}\) to the gyrocenter position \(\textbf{R}\) and the gyroradius vector \(\varvec{\rho }\) as \(\textbf{x} = \textbf{R} + \varvec{\rho }\). Then, after expansion in \(\varvec{\rho }\), the gyroaveraged potential is approximated to

$$\begin{aligned} \langle \delta \phi \rangle _g (\textbf{R},\mu ,t) \simeq J_0 (k_\perp \rho ) \phi (\textbf{R},t) e^{iS(\textbf{R})}, \end{aligned}$$
(6)

where \(\langle \cdots \rangle _g\) denotes the gyroaverage, \(\mu = mv_\perp ^2 / 2B\) is the magnetic moment which is the first adiabatic invariant, \(J_0\) is the zeroth order Bessel function of the first kind that captures the finite Larmor radius (FLR) effect, and \(\textbf{k} = \varvec{\nabla } S (\textbf{R})\) is the wavevector. With Eq. (6), we consider an initial gyro averaged potential appropriate for a self-generated vortex flow

$$\begin{aligned} \langle \delta \phi \rangle _g (X,\mu ,t=0) = J_0 (k_\perp \rho ) \phi (t=0) e^{iS(X)}, \end{aligned}$$
(7)

with initially uniform envelope \(\phi (t=0)\).

In the intermediate time scale longer than the ion bounce period but shorter than the toroidal precession time, the envelope of the self-generated vortex flow approaches a residual level after fast collisionless damping, \(\phi = \phi _\textrm{R}(X)>\phi _\textrm{RH}\), which is moderately higher than the Rosenbluth-Hinton level \(\phi _\textrm{RH}\) predicted in the tokamak geometry (Rosenbluth and Hinton 1998). Note that the residual envelope \(\phi _\textrm{R} (X)\) is spatially inhomogeneous.

In the longer term, with a small background vortex flow, the residual envelope undergoes further damping and evolves to a flux function in x, i.e., \(\psi\), by the homogenization on the tokamak magnetic surfaces by the toroidal precession. That is, the flow envelope becomes a zonal quantity \(\phi =\phi _\textrm{L} (\psi )\). As a result, the residual vortex flow becomes a zonal-vortex mixture

$$\begin{aligned} \langle \delta \phi \rangle _g = J_0 (k_\perp \rho ) \phi _\textrm{L} (\psi ) e^{iS(X)}, \end{aligned}$$
(8)

with \(\psi\)-dependent envelope and X-dependent eikonal part. This zonal-vortex mixture flow has open streamlines around the X-point, which allows particles, fluid elements, and turbulence eddies to move across the magnetic island boundary, advecting in and out of the island. It should also be noted that the amplitude of the flow mixture is \(\phi _\textrm{L}/\phi (t=0) \sim (k_\perp \rho )^2\) which is much smaller than the residual level \(\phi _\textrm{R}/\phi (t=0) \sim \sqrt{\epsilon }/q^2\) for the long wavelength vortex flows considered in this paper. The time scale for the long-term evolution is characterized by the secular magnetic drift frequency \(\overline{\omega }_\textrm{D} = \textbf{k} \cdot \overline{\textbf{v}}_d\), where \(\overline{\textbf{v}}_d\) is the secular magnetic drift velocity. An interesting point is that this resembles the stellarator zonal flow problem (Sugama and Watanabe 2005, 2006a; Helander 2011; Nicolau 2021) where long-term zonal flow damping occurs by the secular radial magnetic drift frequency \(\overline{\omega }_\textrm{D} = k_r \overline{v}_{dr}\). The crucial difference is that while the nonaxisymmetric 3D magnetic field effect is contained in the orbit center velocity \(\overline{v}_{dr}\) for the stellarator zonal flow, it is in the wavevector \(\textbf{k}\) for our vortex flow in a tokamak magnetic island. In short, the tokamak vortex flow and the stellarator zonal flow share a common physics of secular drift frequency-induced long-term collisionless flow damping.

This long-term damping and structure deformation of the self-generated E\(\times\)B vortex flow could be significantly reduced in the presence of a large enough background vortex flow. It is clear from the previous study without the background flow that the difference between the geometry of the self-generated vortex flow and the orbit center trajectory is the origin of the non-trivial long-term evolution. Therefore, the larger the background vortex flow, the smaller the difference between the orbit center trajectory and the self-generated vortex flow, leading to less flow damping and deformation. A rough criterion for the significant reduction of the long-term damping of the self-generated vortex flow is \(e\Phi _0 / T > \epsilon w/r\), where \(\Phi _0(X)\) is the electrostatic potential for the background vortex flow. This synergistic effect between the background and the self-generated vortex flows indicates that the transition, or in other words the bifurcation to the locally enhanced confinement state in the vicinity of a magnetic island likely occurs in the region of strong background E\(\times\)B flow, regardless of the speed of the time increase of the external heat input.

In the remaining part of this paper, we explain the details of calculations, interpretations, and discussions that have been summarized in the Introduction. It is organized as follows. In Sect. 2, we present a gyrokinetic description of the self-generated vortex flow in a stationary tokamak magnetic island. In Sect. 3, we discuss the bounce/transit motion of the gyrocenter and the secular motion of the orbit center with the concept of the drift surface. In Sect. 4, we explicitly calculate the residual vortex flow level and discuss the difference from the tokamak residual zonal flow. In Sect. 5, we explicitly calculate the final flow envelope level achieved after the long-term evolution of the residual vortex flow with negligible and significant background vortex flows. In Sect. 6, we discuss the impact of the gyrokinetic theory of self-generated vortex flows on magnetic fusion energy research. In Sect. 7, we close the paper with a summary.

2 Gyrokinetic equations for the self-generated vortex flow

Nonlinear gyrokinetics (Frieman and Chen 1982; Hahm 1988; Brizard and Hahm 2007) is a well-established formulation for appropriate kinetic description of low-frequency perturbations and long-term behaviors in magnetized plasma. Systematically extracting fast and tedious gyromotion from more interesting slower phenomena based on the conservation of the first adiabatic invariant, the magnetic moment \(\mu\), gyrokinetics have served as a rigorous and practically useful tool for the physics study of magnetized fusion plasmas.

While modern gyrokinetics with full gyrocenter distribution F in \((\mu , v_\parallel )\) velocity space has been extensively used in global gyrokinetic codes (Idomura 2008; Chang and Ku 2008; Obrejan 2017; Michels 2021) due to the benefit of intrinsic satisfaction of conservation laws, here we use the conventional gyrokinetic equations (Frieman and Chen 1982) with a decomposition of the perturbed particle distribution \(\delta f\) in \((\mu , \mathcal {E})\) velocity space in eikonal form which is useful for analytic theory developments. Indeed, there have been plenty of analytic theories that have used Frieman-Chen conventional nonlinear gyrokinetics to study self-generated zonal flows (Rosenbluth and Hinton 1998; Sugama and Watanabe 2005, 2006a; Helander 2011; Chen et al. 2000; Qiu et al. 2016; Wang 2017; Chen and Chen 2022). The Frieman-Chen gyrokinetic Vlasov equation (or simply the gyrokinetic equation in conventional treatment) for electrostatic perturbations, appropriate for the drift wave turbulence and zonal flows (Horton 1999) in magnetic fusion plasmas, is (Frieman and Chen 1982; Brizard and Hahm 2007)

$$\begin{aligned}{} & {} \frac{d \delta g}{dt} = - \left( e \frac{\partial \langle \delta \phi \rangle _g}{\partial t} \frac{\partial }{\partial \mathcal {E}} + \frac{\textbf{b}}{B} \times \varvec{\nabla } \langle \delta \phi \rangle _g \cdot \varvec{\nabla } \right) F_0 - \frac{\textbf{b}}{B} \times \varvec{\nabla } \langle \delta \phi \rangle _g \cdot \varvec{\nabla } \delta g, \end{aligned}$$
(9)

where d/dt is the unperturbed material derivative, e is the species electric charge (not electron charge), \(\mathcal {E}\) is the unperturbed guiding-center energy and \(\textbf{b} = \textbf{B} / B\) is a unit vector in the magnetic field direction. In Eq. (9), \(\delta g\) is the gyro-angle independent part of the non-adiabatic response defined as follows.

$$\begin{aligned}{} & {} \delta f = \left( e\delta \phi \frac{\partial }{\partial \mathcal {E}} + \frac{e}{B} \delta \phi \frac{\partial }{\partial \mu } \right) F_0 \nonumber \\{} & {} \quad + e^{-\varvec{\rho } \cdot \varvec{\nabla }} \left( \delta g - \frac{e\langle \delta \phi \rangle _g}{B} \frac{\partial F_0}{\partial \mu }\right) . \end{aligned}$$
(10)

Here, \(\delta f\) is the perturbed part of the particle distribution \(f=F_0 + \delta f\) in the particle phase space \((\textbf{x},\textbf{v})\). Using eikonal representations for \(\delta \phi (\textbf{x},t)\) as shown in Eqs. (5) and (6) and for \(\delta g\),

$$\begin{aligned} \delta g = g (\textbf{R},\mu ,t) e^{iS(\textbf{R})}, \end{aligned}$$
(11)

assuming Maxwellian unperturbed distribution \(F_0 \propto \exp {(-\mathcal {E}/T)}\), Eq. (10) becomes

$$\begin{aligned} \delta f \simeq - \frac{e\delta \phi (\textbf{x},t)}{T} F_0 + \delta g (\textbf{R},\mu ,t) e^{-ik_\perp \rho }. \end{aligned}$$
(12)

Accordingly, the gyrokinetic Vlasov equation shown in Eq. (9) becomes

$$\begin{aligned}&\frac{\partial g}{\partial t} + (v_\parallel \textbf{b} + \textbf{u}_\textrm{E0} + \textbf{v}_d)\cdot \varvec{\nabla } g + i(\textbf{k} \cdot \textbf{u}_\textrm{E0} + \omega _\textrm{D}) g \nonumber \\&\quad = J_0 (k_\perp \rho ) \frac{e}{T} \frac{\partial \phi }{\partial t} F_0 - \frac{\textbf{b}}{B} \times \varvec{\nabla } J_0 (k_\perp \rho ) \phi \cdot \varvec{\nabla } F_0 - \frac{\textbf{b}}{B} \times i \textbf{k} J_0 (k_\perp \rho ) \phi \cdot \varvec{\nabla } F_0 \nonumber \\&\qquad + \frac{1}{B} \sum _{\textbf{k} = \textbf{k} ' + \textbf{k} ''} [\textbf{b} \cdot (\textbf{k}' \times \textbf{k} '')] J_0 (k_\perp ' \rho ) \phi _{\textbf{k} '} g_{\textbf{k} ''}, \end{aligned}$$
(13)

where the subscripts \(\textbf{k}'\) and \(\textbf{k}''\) have been attached to the envelopes g and \(\phi\) consisting of the E\(\times\)B nonlinearity, the last term in the right-hand side (RHS) of Eqs. (9) and (13), to distinguish the ambient perturbations from the perturbation of our interest. In Eq. (13), \(v_\parallel (\mu , \mathcal {E}) = \sqrt{2(\mathcal {E} - e\Phi _0 - \mu B) / M}\) is the parallel streaming along the magnetic field line, where M is the species mass, \(\textbf{u}_\textrm{E0} = \textbf{b} \times \varvec{\nabla } \Phi _0 / B\) is the background E\(\times\)B flow velocity, \(\textbf{v}_d\) is the magnetic drift velocity and \(\omega _\textrm{D} = \textbf{k} \cdot \textbf{v}_d\) is the magnetic drift frequency.

To understand the behaviors of the self-generated vortex flow and its synergism with the background vortex flow \(\Phi _0 (X)\) by analytic calculations, we simplify the system with uniform, or moderately varying, density \(n_0\) and temperature T profiles. Then, Eq (13) is simplified as follows.

$$\begin{aligned} \frac{\partial g}{\partial t} + (v_\parallel \textbf{b} +\textbf{u}_\textrm{E0} + \textbf{v}_d)\cdot \varvec{\nabla } g + i\omega _\textrm{D} g = J_0 (k_\perp \rho ) \frac{e}{T} \frac{\partial \phi }{\partial t} F_0 + N F_0. \end{aligned}$$
(14)

Here, the last term of the RHS of Eq. (14), which is a shortened expression of the E\(\times\)B nonlinearity in Eq. (13). Note that after the pull-back transformation of the gyrocenter distribution into the particle and the polarization parts (Hahm 1988; Brizard and Hahm 2007), the E\(\times\)B nonlinearity can be divided into the Navier–Stokes and the Hasegawa-Mima nonlinearities, respectively (Chen and Chen 2022). The latter one is a well-known origin of the E\(\times\)B flow self-generation from microturbulence through the modulational instability (Chen et al. 2000; Champeaux and Diamond 2001; Diamond 2001, 2005; Ishizawa et al. 2019). In this work, we neglect the Navier–Stokes nonlinearity and focus on the Hasegawa-Mima nonlinearity (Hasegawa and Mima 1977). The motivations for this are discussed in the later part of this section.

In the later sections, we present explicit calculations of the basic physics property of time evolution of the self-generated vortex flow by considering an impulse source

$$\begin{aligned} N F_0 = \delta f_g (t=0) \delta (t) = - \langle \delta n_p (t=0) \rangle \frac{F_0}{n_0} \delta (t), \end{aligned}$$
(15)

where \(\delta f_g\) is the perturbed gyrocenter distribution, \(\delta n_p\) is the (classical) polarization density and \(\langle \cdots \rangle\) is the flux surface average (on the helical magnetic surface here for the vortex flow in the magnetic island), which has been proven to be useful in plenty of previous theoretical works (Rosenbluth and Hinton 1998; Sugama and Watanabe 2005, 2006a; Helander 2011; Xiao and Catto 2006; Wang and Hahm 2009; Hahm 2013; Guo 2017; Choi and Hahm 2018; Cho and Hahm 2019; Singh and Diamond 2021). The influence of the source distribution function has been discussed in detail in Refs. (Kim and Jhang 2020; Kim et al. 2022).

So far we have presented the gyrokinetic Vlasov equation, which corresponds to the particle equation describing how fields affect particles. The self-consistent gyrokinetic system is constructed by closing the system with the field equation that describes how the particles affect the fields. For the case of the electrostatic perturbation, it is the gyrokinetic Poisson equation in the modern gyrokinetic terminology (Brizard and Hahm 2007), which is an equivalent of the quasi-neutrality condition

$$\begin{aligned} \delta n_e = Z_i \delta n_i, \end{aligned}$$
(16)

for the case of single-species ions which will be assumed in this paper. In conventional gyrokinetics, the quasi-neutrality condition shown in Eq. (16) could be rewritten as

$$\begin{aligned} n_{e0} \frac{|e|\delta \phi }{T_e} + \int d^3 v \, J_0 (k_\perp \rho _e) \delta g_e = -n_{e0} \frac{Z_i |e|\delta \phi }{T_i} + \int d^3 v \, J_0 (k_\perp \rho _i) \delta g_i, \end{aligned}$$
(17)

where we have used the equilibrium part of the quasi-neutrality condition \(n_{e0} = Z_i n_{i0}\).

There are two main motivations for the use of the specific form of the initial impulse (‘kick’) vortex flow source shown in Eq. (15). First, its direct proportionality to the minus perturbed polarization density \(-\delta n_p\) has been highly motivated by theoretical treatments of the ion temperature gradient (ITG) turbulence, which is a representative turbulence type in magnetized fusion plasmas (Horton 1999) and has Therefore been considered as a primary candidate self-generating the mesoscale E\(\times\)B flows (Lin 1998; Rosenbluth and Hinton 1998). Since a non-trivial electron contribution is not essential, though could be significant (Lin 2007), in the physics mechanism of the linear ITG mode drive, the electron response has typically been assumed to be adiabatic, i.e., \(\delta \tilde{n}_e = |e|\delta \tilde{\phi }/T_e\), based on an ordering \(\omega \ll k_\parallel v_{Te}\). Here, we have decomposed perturbations as \(A = \langle A \rangle + \tilde{A}\). With the adiabatic electrons, the lowest-order incompressible part of the flux-surface-averaged perturbed continuity equation

$$\begin{aligned} \frac{\partial \langle \delta n_e \rangle }{\partial t} + \langle \delta \tilde{\textbf{v}}_\textrm{E} \cdot \varvec{\nabla } \delta \tilde{n}_e \rangle = 0, \end{aligned}$$
(18)

yields zero particle density response \(\langle \delta n_e \rangle = 0\). (Recall that we consider a quasi-neutral plasma in this paper as shown in Eq. (16).) The compressible part \(\langle \delta \tilde{n}_e \varvec{\nabla } \cdot \delta \tilde{\textbf{v}}_\textrm{E} \rangle\) due to non-uniform B is responsible for the geodesic acoustic mode (GAM) (Winsor et al. 1968) which is not our interest as a source. In the presence of a significant wave-particle resonance of electrons or in the hydrodynamic electron regime, the non-adiabatic electron response leads to zonal density dynamics resulting in the density profile corrugation (Singh and Diamond 2021). This is relevant to the Navier–Stokes nonlinearity in the gyrokinetic equation, Eq. (13), mentioned previously. Back to the adiabatic electrons, since the perturbed particle density \(\delta n = \delta n_g + \delta n_p\) can be divided by the gyrocenter density \(\delta n_g\) and the polarization density \(\delta n_p\) by the pull-back transformation (Hahm 1988; Brizard and Hahm 2007; Wang and Hahm 2009; Dubin 1983), we have \(\langle \delta n_g \rangle = - \langle \delta n_p \rangle\) with \(\langle \delta n \rangle =0\). Note that by integrating Eq. (14) over the velocity space, we obtain the perturbed gyrocenter continuity equation for \(\delta n_g\), and then Eq. (15) yields the initial impulse gyrocenter density source \(\langle \delta n_g (t=0) \rangle \delta (t) = -\langle \delta n_p(t=0) \rangle \delta (t)\).

Second, the direct proportionality of the impulse vortex flow source shown in Eq. (15) to the unperturbed distribution function \(F_0\), typically assumed to be Maxwellian, is to minimize the kinetic effects coming from the source and to focus on the gyrokinetic plasma response. In general, the velocity space structure of the source could affect the time evolution of a self-generated E\(\times\)B flow (Falessi and Zonca 2019).

Note that in this paper, we address analytic calculations of long-wavelength vortex flows satisfying an ordering

$$\begin{aligned} \rho _e< \Delta _{e}< \rho _i< \Delta _i< |\textbf{k} |^{-1}< w < r_s, \end{aligned}$$
(19)

where \(\Delta\) is the orbit width (banana width for trapped particles). Based on this ordering, for simplicity, we neglect electron FLR and finite orbit width (FOW) effects while considering ion FLR and FOW effects. As a consequence, the Bessel function \(J_0 (k_\perp \rho _e) \rightarrow 1\) and the gyrocenter density is assumed to be the same with the particle density \(\delta n_{eg} \rightarrow \delta n_e\) for electrons, and as a result the impulse vortex flow source comes only from the ions.

We take the bounce/transit average to Eq. (14) for the study of the time evolution of the initially given vortex flow and calculate the residual vortex flow level after a fast collisionless damping. Then, for the long-term evolution of the residual vortex flow, we take the drift average to the bounce/transit-averaged equation and calculate the flow level after the long-term damping and deformation. Mathematical definitions of the bounce/transit average and the drift average inside the magnetic island are presented in the next section.

3 Single particle motion, bounce/transit and drift averages

Following previous works (Rosenbluth and Hinton 1998; Sugama and Watanabe 2005, 2006a), we seek to obtain a bounce/transit averaged kinetic equation for an analytic study of the long-term evolution of the self-generated vortex flow after fast collisionless damping by toroidicity-induced linear coupling with GAM that undergoes ion transit resonance (Sugama and Watanabe 2006b). In the magnetic island geometry, the parallel streaming is mostly in the z-direction, the direction of the reference (guide) magnetic field, and the projected streaming along the magnetic island contour in the \(x-y\) plane is way slower. Given this time scale disparity, we define the bounce/transit average in the magnetic island geometry as (Wilson 1996; Shaing 2001)

$$\begin{aligned} \overline{A} \equiv \left. \oint _{b/t} \frac{dz}{v_\parallel } A \Bigg / \oint _{b/t} \frac{dz}{v_\parallel } \right. , \end{aligned}$$
(20)

where b/t represents the trajectory of the lowest order guiding center motion which is determined by the streaming along the z-direction. A different point of view of Eq. (20) as an approximation of a precise bounce/transit average is presented in Appendix A.

Note that Eq. (20) still holds even in the presence of a large background E\(\times\)B flow. This is quite a different situation from the tokamak geometry with a large background \(\textrm{E}_r\times\)B flow. In the tokamak geometry with axisymmetry in the toroidal direction, the bounce/transit motion is determined in the poloidal direction. Therefore, we have the contribution from the background \(\textrm{E}_r\times\)B flow comparable to the projected parallel streaming \(v_\parallel B_\theta /B\) on the poloidal cross-section, resulting in the modification of the denominator of the bounce/transit integral \(v_\parallel \rightarrow v_\parallel + E_r/B_\theta\). Through this contribution the background \(\textrm{E}_r\times\)B flow affects the ion FOW effect, so-called ‘orbit squeezing’ (Shaing and Hazeltine 1992; Hinton and Kim 1995; Baek et al. 2006), leading to a modification of the residual level of tokamak zonal flows (Kagan and Catto 2009; Landreman and Catto 2010). In contrast, in the magnetic island geometry, the lowest-order bounce/transit motion is determined in the z-direction, the direction of the reference helical magnetic field \(\textbf{B}_s\), completely orthogonal to the background vortex flow with \(\Phi _0 (X)\). Therefore, from Eqs. (14) and (20), it is clear that the background E\(\times\)B flow does not contribute to the bounce/transit averaging and the residual level for the case of the vortex flows in a tokamak magnetic island.

Considering the parallel velocity \(v_\parallel (\mu ,\mathcal {E}) = \sqrt{2(\mathcal {E} - e\Phi -\mu B)/2}\), we neglect the contribution of the magnetic island perturbation \(\delta \psi\), or \(\delta \textbf{B} = \varvec{\nabla } \zeta \times \varvec{\nabla } \delta \psi = \delta B_x \hat{x}\), since the inhomogeneity of the magnetic field strength B coming from the perturbed part \(\delta \textbf{B}\) is much smaller than one from the equilibrium part \(\textbf{B}\) as shown in Eq. (A13). So, the parallel velocity is approximated to

$$\begin{aligned} v_\parallel \simeq \textrm{sgn}(v_\parallel )\sqrt{\frac{2}{M}\left[ \mathcal {E} - e\Phi - \mu B_0 (1-\epsilon \cos {\theta })\right] }. \end{aligned}$$
(21)

The same approximation neglecting the \(\delta \textbf{B}\) contribution to B applies to the magnetic drift velocity

$$\begin{aligned} \textbf{v}_d = -\left( \frac{\mu B}{M} + v_\parallel ^2\right) \frac{\varvec{\nabla } B \times \textbf{B}}{\Omega _c B^2}, \end{aligned}$$
(22)

is proportional to \(\varvec{\nabla } B \times \textbf{B}\) in the low-\(\beta\) limit. Here, \(\Omega _c = eB/M\) is the gyrofrequency. That is, the gyrocenter trajectories in the magnetic island are almost the same as the ones in axisymmetric tokamak geometry without the island. One can check orderings that the contributions of \(\varvec{\nabla } \delta B \times \textbf{B}\) and \(\varvec{\nabla } B_0 \times \delta \textbf{B}\) to the magnetic drift frequency \(\omega _\textrm{D}\) are much smaller by \(\mathcal {O}((w/r)^4 \epsilon )\) and \(\mathcal {O}((w/r)^3 \epsilon ^2)\), respectively, compared to the contribution from unperturbed part \(\varvec{\nabla } B_0 \times \textbf{B}_0\). Consequently, the main non-trivial features of the time evolution of the self-generated vortex flow originate from the geometrical property of the vortex flow perturbation \(\delta \phi (X)\) contained in \(\textbf{k}\). The single particle trajectory is treated to be the same as the axisymmetric tokamak case without the magnetic island.

In a longer-term \(t>\overline{\omega }_\textrm{D}^{-1}\), the residual vortex flow undergoes further time evolution due to the finite secular magnetic drift frequency \(\overline{\omega }_\textrm{D}=\textbf{k} \cdot \overline{\textbf{v}}_d\). As explained in the previous paragraph, it is mainly due to the geometrical property contained in \(\textbf{k}\), which is different from the long-term evolution of the stellarator zonal flows where \(\overline{\textbf{v}}_d\) makes the distinction from the tokamak zonal flow (Sugama and Watanabe 2005, 2006a; Helander 2011). Here, the long-term evolution of the vortex flows is determined by the next-order gyrocenter motions slower than the parallel streaming, i.e., slower than the bounce/transit motion. These could be properly analyzed by focusing on the trajectories of the centers of the bonuce/transit orbit, so-called ‘orbit centers’ (Wang and Hahm 2009), finding the drift surface and its action-angle pair \((\Omega ,\varphi )\) (Cary and Brizard 2009). We rewrite the bounce/transit-averaged unperturbed propagator as

$$\begin{aligned} \left( \overline{v}_\parallel \textbf{b}_\perp + \overline{\textbf{u}}_\textrm{E0} + \overline{\textbf{v}}_{d}\right) \cdot \varvec{\nabla } = \omega _\varphi \left. \frac{\partial }{\partial \varphi } \right| _{\Omega ,\mathcal {E},\mu }, \end{aligned}$$
(23)

where \(\omega _\varphi\) is the drift frequency which corresponds to \(\dot{\varphi }\) and \(\textbf{b}_\perp = (B_x \hat{x} + B_y \hat{y})/B\) is the projection of the unit vector for the magnetic field line \(\textbf{b}\) on the \(x-y\) plane, where

$$\begin{aligned} \frac{B_x}{B_s} = \frac{w^2}{4L_s} k\sin {(ky)}, \quad \frac{B_y}{B_s} = -\frac{x}{L_s}. \end{aligned}$$
(24)

Applying Eq. (23) to the drift surface label \(\Omega\), we have

$$\begin{aligned} \left( \overline{v}_\parallel - \frac{R_a \Phi _0}{\tilde{\psi }} \right) \frac{B_x}{B_s} \frac{\partial \Omega }{\partial x} + \left\{ \left( \overline{v}_\parallel - \frac{R_a \Phi _0}{\tilde{\psi }} \right) \frac{B_y}{B_s} + \overline{v}_d \right\} \frac{\partial \Omega }{\partial y} =0, \end{aligned}$$
(25)

where \(R_a\) is the major radius at the magnetic axis, and we have used \(\overline{\textbf{v}}_d \cdot \varvec{\nabla } = \overline{v}_d \partial /\partial y\). The explicit expressions of the precession velocity \(\overline{v}_{d}\) and the bounce/transit-averaged parallel velocity are

$$\begin{aligned} \overline{v}_{d}&= - \frac{1}{R \Omega _{c}} \frac{\mu B_a}{M} \left\{ 2\frac{E(\kappa )}{K(\kappa )} -1 \right\} \quad \text {(trapped)}, \end{aligned}$$
(26)
$$\begin{aligned}&=- \frac{1}{R \Omega _{c}} \frac{\mu B_a}{M} \left\{ 2\kappa ^2 \frac{E(\kappa ^{-1})}{K(\kappa ^{-1})} + 1 - 2\kappa ^2 \right\} \quad \text {(passing)} , \end{aligned}$$
(27)

and

$$\begin{aligned} \overline{v}_\parallel&= 0 \quad \text {(trapped)}, \end{aligned}$$
(28)
$$\begin{aligned}&= \textrm{sgn} (v_\parallel ) \sqrt{\epsilon } \sqrt{\frac{\mu B_a}{M}} \pi \frac{\kappa }{K(\kappa ^{-1})} \quad \text {(passing)}, \end{aligned}$$
(29)

where \(\kappa\) is the velocity pitch angle parameter defined as follows in the high-aspect-ratio tokamaks.

$$\begin{aligned} \kappa ^2 = \frac{\mathcal {E} - e \Phi _0 - \mu B_a (1-\epsilon )}{2\epsilon \mu B_a}, \end{aligned}$$
(30)

and K(k) and E(k) are the complete elliptic integral of the first and the second kind, respectively. Note that for Eqs. (26)-(29), we have used

$$\begin{aligned} v_\parallel&= \textrm{sgn}(v_\parallel )\sqrt{\frac{4\epsilon \mu B_a}{M}} \kappa \cos {\vartheta } \quad \text {(trapped)}, \end{aligned}$$
(31)
$$\begin{aligned}&= \textrm{sgn}(v_\parallel )\sqrt{\frac{4\epsilon \mu B_a}{M}} \kappa \sqrt{1 - \kappa ^{-2} \sin ^2{\vartheta }} \quad \text {(passing)}, \end{aligned}$$
(32)

where the bounce/transit angle \(\vartheta\) is given by (Wang and Hahm 2009)

$$\begin{aligned} \vartheta&= \arcsin {(\kappa ^{-1}\sin {(\theta /2)})} \quad \text {(trapped)}, \end{aligned}$$
(33)
$$\begin{aligned}&= \theta /2 \quad \text {(passing)} . \end{aligned}$$
(34)

Meanwhile, on the drift surface \(\Omega =\text {const}\),

$$\begin{aligned} d\Omega = \frac{\partial \Omega }{\partial x} dx + \frac{\partial \Omega }{\partial y} dy =0. \end{aligned}$$
(35)

Note that here we have used the fact that \(\mathcal {E}=\text {const}\) and \(\mu =\text {const}\) for a single particle. Substituting Eq. (25) into Eq. (35),

$$\begin{aligned} - \left( \overline{v}_\parallel \frac{B_y}{B_s} + \overline{v}_{d} \right) dx + \frac{B_x}{B_s} dy = 0. \end{aligned}$$
(36)

To the lowest order in w/r, neglecting spatial variations of \(\overline{v}_{d}\) and \(\overline{v}_\parallel\) in Eq. (36) we could rewrite it as

$$\begin{aligned} \left( \overline{v}_\parallel - \frac{R_a}{\tilde{\psi }} \Phi _0' \right) dX - \overline{v}_{d} \frac{4L_s}{w^2} dx = 0. \end{aligned}$$
(37)

Note that Eq. (37) yields a general drift surface label

$$\begin{aligned} \Omega = X - \frac{R_a}{\tilde{\psi }} \frac{\Phi }{\overline{v}_\parallel } - \frac{4L_s}{w^2} \frac{\overline{v}_{d}}{\overline{v}_\parallel } x, \end{aligned}$$
(38)

which is consistent with previous drift-kinetic calculations (Imada 2018; Dudkovskaia 2021). In this paper, to further address analytic kinetic calculations, we go to limiting cases to get analytically tractable expressions of the action-angle pair. The relative amplitude of the second term in Eq. (37) compared to the third is, using Eqs. (26) and (27),

$$\begin{aligned} \frac{\text {(second term)}}{\text {(third term)}} \sim \frac{R_a \Phi _0 / \tilde{\psi }}{4L_s \overline{v}_d/w} \sim \frac{e\Phi _0/T}{\epsilon w/r} = \frac{\Phi _0}{\Phi _{cr}}, \end{aligned}$$
(39)

where the asymptotic critical amplitude of the background vortex flow is

$$\begin{aligned} \frac{e\Phi _{cr}}{T} = \epsilon \frac{w}{r}. \end{aligned}$$
(40)

Note that the critical background flow level is proportional to the inverse aspect ratio \(\epsilon\) and the relative island width w/r. For the passing particles, since \(\overline{v}_\parallel \ne 0\) as shown in Eq. (29), we should also compare the ratio of the first and the third terms in Eq. (37),

$$\begin{aligned} \frac{\text {(first term)}}{\text {(third term)}} \sim \frac{w}{L_s} \frac{\sqrt{\epsilon }v_\perp }{v_\perp ^2 /R\Omega _{c}} \sim \frac{\sqrt{\epsilon }\hat{s} w}{q\rho _T} = \frac{w}{w_{cr}}, \end{aligned}$$
(41)

where \(v_\perp\) is the perpendicular particle velocity. The asymptotic critical magnetic island half-width is

$$\begin{aligned} w_{{cr}} = \frac{q\rho _{T}}{\sqrt{\epsilon } \hat{s}} \sim \frac{\Delta _b}{\hat{s}}. \end{aligned}$$
(42)

Based on the orderings shown in Eqs. (39) and (41), we consider three asymptotic regimes of the orbit center trajectory depending on the background vortex potential \(\Phi\) and the magnetic island half-width w as shown in Table 1.

Table 1 Asymptotic regimes of the orbit center trajectory
Full size table

One should be aware that we have simplified the argument considering only the representative quantities, while the relative amplitudes of the three terms in Eq. (37) depends on the energy \(\mathcal {E}\) and the velocity pitch \(\kappa\) in a precise sense. In the following subsections, we present approximate expressions of the drift surface label \(\Omega\) and the drift angle \(\varphi\) in each regime shown in Table 1, obtained focusing on the effect of the toroidal precession which is the very non-trivial feature of the tokamak magnetic island compared to the usual slab magnetic island.

3.1 Toroidal regime

In the toroidal regime, solely the toroidal procession, the third term of the LHS of Eq. (37) is dominant. Therefore, we can simply approximate the drift surface label to the tokamak magnetic surface label,

$$\begin{aligned} \Omega = \psi , \end{aligned}$$
(43)

which is equivalent to x in the magnetic island geometry, and \(\varphi = \zeta\) (Choi and Hahm 2022) keeping only the toroidal precession in the orbit center motion. Here, we have

$$\begin{aligned} \omega _\varphi = n\omega _{pr} \simeq k\overline{v}_{d}, \end{aligned}$$
(44)

where \(\omega _{pr}\) is the well-known toroidal precession frequency.

3.2 Shifted vortex regime

In the shifted vortex regime, the background vortex flow, the second term in Eq. (37), dominates the toroidal precession and the projected streaming. Since the background vortex flow and the projected streaming are both flux functions in X as shown in Eq. (37), we neglect the projected streaming while keeping the toroidal precession. As a result, we have

$$\begin{aligned} \Omega = \frac{2(x-d)^2}{w^2} - \cos {(ky)}, \end{aligned}$$
(45)

which is radially shifted from the helical magnetic flux X by

$$\begin{aligned} d = -\frac{L_s \overline{v}_d}{R_a \Phi '/\tilde{\psi }} = -\frac{\overline{v}_\textrm{d}}{4\Phi ' /Bw} w \sim \frac{\overline{v}_\textrm{d}}{u_\textrm{E}} w, \end{aligned}$$
(46)

The radial shift \(d/w \sim \overline{v}_\textrm{d} / u_\textrm{E}\) reveals that the deviation of the particle trajectory from the helical magnetic surface is determined by the competition between the toroidal precession on the unperturbed tokamak magnetic surface and the background vortex flow on the helical island magnetic surface. Note that \(|d|\ll w\), which indicates that a large background vortex flow reduces the finite surface deviation (FSD) of the drift surface from the magnetic surface. This leads to a reduction of the long-term damping and structure deformation of the self-generated flow as will be presented in the next section. Similar to the case of the original helical magnetic surface (Rutherford 1973; Hahm 2021) shown in Eq. (A3), the drift surface label, Eq. (45), could be described by polar coordinates

$$\begin{aligned} \eta \cos {\varphi } = \frac{x-d}{w}, \quad \eta \sin {\varphi } = \sin {\left( \frac{ky}{2}\right) }, \quad \eta ^2 = \frac{\Omega +1}{2}. \end{aligned}$$
(47)

In general, the expression of the drift frequency \(\omega _\varphi\) can be obtained by applying the bounce/transit-averaged unperturbed propagator, i.e., Eq. (23), to the drift angle \(\varphi\).

$$\begin{aligned} \omega _\varphi = \left( \overline{v}_\parallel \textbf{b}_\perp + \overline{\textbf{u}}_\textrm{E0} + \overline{\textbf{v}}_\textrm{d}\right) \cdot \varvec{\nabla } \varphi . \end{aligned}$$
(48)

An approximate expression of the drift frequency \(\omega _\varphi\) appropriate to the shifted vortex regime can be obtained from Eqs. (24), (47) and (48) as follows, neglecting the projected streaming term.

$$\begin{aligned} \omega _\varphi \simeq \left( \overline{\textbf{u}}_\textrm{E0} + \overline{\textbf{v}}_\textrm{d}\right) \cdot \varvec{\nabla } \varphi = \frac{2k\Phi '}{Bw} \sqrt{1-\eta ^2 \sin ^2{\varphi }} \left( 1 - d_x \sin ^2{\varphi }\right) , \end{aligned}$$
(49)

where \(d_x \equiv \partial d / \partial x\) which is typically small by \(\mathcal {O}(d/w)\).

3.3 Shifted island regime

For the passing particles, the projected streaming along the magnetic island contour in the \(x-y\) plane could be dominant in the shifted island regime as shown in Table 1. In this regime, following the same argument as in the shifted vortex regime, we neglect the advection by the background vortex flow, while keeping the projected streaming and the toroidal precession. Then, the approximate expression of the drift surface label becomes

$$\begin{aligned} \Omega = \frac{2(x-d)^2}{w^2} - \cos {(ky)}, \end{aligned}$$
(50)

where

$$\begin{aligned} d = \frac{L_s \overline{v}_\textrm{d}}{\overline{v}_\parallel } \end{aligned}$$
(51)

is the radial shift of the drift surface \(\Omega\) from the magnetic surface X due to the toroidal precession. Again, \(|d| \ll w\). Equation (51) shows that in this regime, it is the competition between the toroidal precession on the tokamak magnetic surface and the projected streaming on the island’s magnetic surface which determines the deviation of the particle trajectory from the magnetic surface. The expression of the drift frequency \(\omega _\varphi\) for the shifted island regime is obtained using Eqs. (24), (47) and (48) as follows.

$$\begin{aligned} \omega _\varphi \simeq \left( \overline{v}_\parallel \textbf{b}_\perp + \overline{\textbf{v}}_\textrm{d}\right) \cdot \varvec{\nabla } \varphi = - \frac{kw}{2L_s} \overline{v}_\parallel \sqrt{1-\eta ^2 \sin ^2{\varphi }} \left( 1-d_x \sin ^2{\varphi }\right) . \end{aligned}$$
(52)

4 Residual vortex flow level

In the intermediate time scale slower than the ion bounce motion but faster than the secular magnetic drift \(\omega _{bi}^{-1}< t < \overline{\omega }_\textrm{D}^{-1}\) (note that the bounce frequency \(\omega _b\) is slower than the transit frequency \(\omega _t\)), the initially self-generated vortex flow \(\delta \phi\) approaches to its residual level. In this section, we show this with derivation and analytic calculation by approximating the gyrokinetic equations ignoring the secular magnetic drift frequency \(\overline{\omega }_\textrm{D}\) while keeping the fastly varying part that gives the finite orbit width (FOW) effect. This time scale separation of the magnetic drift frequency is formally achieved as (Helander 2011)

$$\begin{aligned} \omega _\textrm{D} = v_\parallel \partial _z Q + \overline{\omega }_\textrm{D}, \end{aligned}$$
(53)

where \(Q \sim k_X \Delta\) is the term that gives the FOW effect. Here, \(k_X \equiv |\textbf{k}|\) is the amplitude of the wavevector \(\textbf{k} = \varvec{\nabla }S(X)\) of the vortex flow perturbation \(\delta \phi (X)\) which should not be confused with the wavenumber \(k=m/r_s\) of the magnetic island perturbation \(\delta \psi (y)\), and \(\Delta\) is the radial width of the bounce/transit orbit. We substitute Eq. (53) into the gyrokinetic Vlasov equation for the self-generated vortex flow, Eq. (14), and expand the equation and g in \(\omega / \omega _{b/t}\) considering behaviors \(\omega\) slower than the bounce/transit motion \(\omega _{b/t}\) only. Then, the lowest-order equation is

$$\begin{aligned} v_\parallel \partial _z g_0 + i (v_\parallel \partial _z Q)g_0 = 0, \end{aligned}$$
(54)

which gives a formal solution (Rosenbluth and Hinton 1998)

$$\begin{aligned} g_0 = h\exp {(-iQ)}, \end{aligned}$$
(55)

where \(h=h(x,y)\) is the bounce/transit-angle-independent part as \(z \propto \theta\) has a one-to-one correspondence to the bounce/transit angle (Wang and Hahm 2009). The next order equation is

$$\begin{aligned}&\frac{\partial g_0}{\partial t} + v_\parallel \partial _z g_1 + i(v_\parallel \partial _z Q) g_1 + i\overline{\omega }_\textrm{D} g_0 \nonumber \\&\qquad + (v_\parallel \textbf{b}_\perp + \textbf{u}_\textrm{E0} + \textbf{v}_d) \cdot \varvec{\nabla } g_0 \nonumber \\&\quad = J_0 \frac{e}{T} F_0 \frac{\partial \phi }{\partial t} + N F_0 . \end{aligned}$$
(56)

Multiplying Eq. (56) by \(\exp {(iQ)}\) and taking the bounce/transit average shown in Eq. (20), we obtain the bounce/transit-kinetic equation as follows.

$$\begin{aligned}{} & {} \frac{\partial h}{\partial t} + i\overline{\omega }_\textrm{D} h + \overline{e^{iQ} (v_\parallel \textbf{b}_\perp + \textbf{u}_\textrm{E0} + \textbf{v}_d) \cdot \varvec{\nabla } (he^{-iQ})} \nonumber \\{} & {} \quad = \frac{e}{T} F_0\overline{e^{iQ} J_0 \frac{\partial \phi }{\partial t}} + \overline{e^{iQ} N}F_0. \end{aligned}$$
(57)

We take the long-wavelength approximation to the bounce/transit-averaged advection terms in LHS of Eq. (57) for simplicity so that

$$\begin{aligned}{} & {} \frac{\partial h}{\partial t} + i\overline{\omega }_\textrm{D} h + \left( \overline{v}_\parallel \textbf{b}_\perp + \overline{\textbf{u}}_\textrm{E0} + \overline{\textbf{v}}_\textrm{d} \right) \nonumber \\{} & {} \quad \cdot \varvec{\nabla } h = \frac{e}{T} F_0\overline{e^{iQ} J_0 \frac{\partial \phi }{\partial t}} + \overline{e^{iQ} N}F_0. \end{aligned}$$
(58)

It should be emphasized that we keep the general form of the RHS of Eq. (57) which is necessary to properly describe the polarization shielding (Rosenbluth and Hinton 1998; Sugama and Watanabe 2005, 2006a). In the intermediate time scale \(\omega _{b}^{-1}< t < \overline{\omega }_\textrm{D}^{-1}\), we further neglect all the slower terms of the LHS of Eq. (58) leaving only the first term, resulting in

$$\begin{aligned} \frac{\partial h}{\partial t} = \frac{e}{T}F_0 \overline{e^{iQ} J_0 \frac{\partial \phi }{\partial t}} + \overline{e^{iQ} N} F_0, \end{aligned}$$
(59)

presuming weak inhomogeneity of the envelope h guaranteed from the initial condition. The formal solution of Eq. (59) is

$$\begin{aligned} h = \frac{e}{T} F_0 \overline{e^{iQ} J_0 \phi } + \overline{e^{iQ} P} F_0, \end{aligned}$$
(60)

where \(P = \int dt N\) represents the amount of the vortex flow production through the E\(\times\)B nonlinearity. Substituting Eq. (60) into Eq. (16), the quasi-neutrality condition, and taking the flux-surface average, we obtain

$$\begin{aligned} \frac{Z_i |e|}{T_i} \phi \left\langle 1 - \int d^3 v \frac{F_{0i}}{n_{i0}} J_0 e^{-iQ} \overline{e^{iQ} J_0 } \right\rangle = \left\langle P\right\rangle . \end{aligned}$$
(61)

Hereafter, \(J_0\) and Q are only for ions, as we neglect the electron FLR and FOW effects as mentioned previously. In Eq. (61), the flow production term P is

$$\begin{aligned} P = \int d^3 v \frac{F_{0i}}{n_{i0}} J_0 e^{-iQ} \overline{e^{iQ}J_0 P_{i}} - \int d^3 v \frac{F_{0e}}{n_{e0}} P_{e}. \end{aligned}$$
(62)

We consider an initial kick as shown in Eq. (15), which gives

$$\begin{aligned} \langle P \rangle = \frac{Z_i|e|}{T_i} \left\langle k_X^2 \rho _{Ti}^2\right\rangle \phi (t=0), \end{aligned}$$
(63)

using the long-wavelength expression of the polarization density (Xiao and Catto 2006; Wang and Hahm 2009). In Eq. (63), \(\rho _{Ti}\) is the ion thermal Larmor radius. Substituting Eq. (63) into Eq. (61), we obtain a formal expression of the residual vortex flow level

$$\begin{aligned} R = \frac{\phi }{\phi (t=0)} = \frac{\chi _\textrm{cl}}{\chi _\textrm{cl} + \chi _\textrm{nc}}, \end{aligned}$$
(64)

where

$$\begin{aligned}{} & {} \chi _\textrm{cl} = \left\langle k_X^2 \rho _{Ti}^2 \right\rangle , \nonumber \\{} & {} \chi _\textrm{nc} = \left\langle \int d^3 v \frac{F_{0i}}{n_{i0}} \left( \overline{Q_i^2} - \overline{Q}_i^2 \right) \right\rangle , \end{aligned}$$
(65)

are the classical and neoclassical susceptibilities for polarization shielding (Xiao and Catto 2006; Wang and Hahm 2009), which originate from the FLR and FOW effects.

We perform explicit analytic calculations of the classical and neoclassical susceptibilities and the residual vortex flow level shown in Eqs. (64) and (65) for a magnetic island in a circular concentric tokamak plasma. From Eq. (4), we have

$$\begin{aligned} \textbf{k} = \varvec{\nabla } S(X) = S' \left[ \hat{x} \frac{4x}{w^2} + \hat{y} k \sin {(ky)} \right] , \end{aligned}$$
(66)

where \(S' = dS/dX\). In Eq. (66) and Fig. 1, it is clear that the vortex flow wavevector \(\textbf{k}\) has not only the radial x component but also the helical angle y component. So the vortex flow wavevector is neither radial nor isotropic.

Fig. 1
figure 1

Vortex flow wavevector \(\textbf{k}\) (black arrows) plotted on the magnetic island contour X (colormap) in the case of \(kw = 1\), showing finite helical angle y component

Full size image

Therefore, from Eqs. (26), (27) and (66), we obtain the explicit expression of the secular magnetic drift frequency \(\overline{\omega }_\textrm{D} = \textbf{k} \cdot \overline{\textbf{v}}_d\) as follows.

$$\begin{aligned} \overline{\omega }_\textrm{D}&= - \frac{S'}{R_a \Omega _{ca}} \frac{\mu B_a}{M} k\sin {(ky)} \left[ 2\frac{E(\kappa )}{K(\kappa )} -1 \right] \quad \text {(trapped)} , \end{aligned}$$
(67)
$$\begin{aligned}&= - \frac{S'}{R_a \Omega _{ca}} \frac{\mu B_a}{M} k\sin {(ky)} \left[ 2\kappa ^2 \frac{E(\kappa ^{-1})}{K(\kappa ^{-1})} +1-2\kappa ^2 \right] \quad \text {(passing)} . \end{aligned}$$
(68)

Substituting Eqs. (67) and (68) into Eq. (53), we obtain an explicit expression of the FOW factor Q as follows.

$$\begin{aligned} Q = Q_0 + Q_1, \end{aligned}$$
(69)

where

$$\begin{aligned} Q_0&= \frac{B_a S'}{\Omega _{ca}} \frac{4x}{w^2} \frac{qR_a}{r} \frac{v_\parallel }{B}, \end{aligned}$$
(70)
$$\begin{aligned} Q_1&= - \sigma \frac{S'}{\Omega _{ca}} \frac{q}{\sqrt{\epsilon }} \sqrt{\frac{\mu B_a}{M}} 2k\sin {(ky)} \left[ \textrm{E}(\vartheta ,\kappa ) - \frac{E(\kappa )}{K(\kappa )} \textrm{F}(\vartheta ,\kappa ) \right] \quad \text {(trapped)}, \end{aligned}$$
(71)
$$\begin{aligned}&= - \sigma \frac{S'}{\Omega _{ca}} \frac{q}{\sqrt{\epsilon }} \sqrt{\frac{\mu B_a}{M}} 2k\sin {(ky)} \kappa \left[ \textrm{E}(\vartheta ,\kappa ^{-1}) - \frac{E(\kappa ^{-1})}{K(\kappa ^{-1})} \textrm{F}(\vartheta ,\kappa ^{-1}) \right] \,\, \text {(passing)}. \end{aligned}$$
(72)

Here, \(\textrm{F}(x,k)\) and \(\textrm{E}(x,k)\) are the incomplete elliptic integral of the first and the second kinds, respectively. Note that \(Q_0\) comes from the unperturbed tokamak magnetic field \(\textbf{B}_0\), and \(Q_1\) is from the magnetic island perturbation \(\delta \textbf{B}\).

We now move on to the explicit expression of the flux surface average \(\langle \cdots \rangle\) in the magnetic island geometry,

$$\begin{aligned} \left\langle A \right\rangle (X) = \left\langle \left\langle A (x,y,z) \right\rangle _z \right\rangle _y, \end{aligned}$$
(73)

which could be decomposed into two parts

$$\begin{aligned}&\left\langle A \right\rangle _z = \frac{1}{2\pi } \oint d\theta \, A, \end{aligned}$$
(74)
$$\begin{aligned}&\left\langle A \right\rangle _y = \left. \oint _X \frac{dy\, A}{\pm \sqrt{X+\cos {(ky)}}} \Bigg / \oint _X \frac{dy}{\pm \sqrt{X+\cos {(ky)}}} \right. . \end{aligned}$$
(75)

One should be aware that the y-average \(\langle \cdots \rangle _y\) shown in Eq. (75) is done on a constant helical magnetic flux X surface, not on a constant-x surface. The integration range is limited to \([-y_t,y_t]\), where \(X + \cos {(ky)} =0\) at \(y=\pm y_t\). Note that it does not mean that the orbit center trajectory is limited on a constant-X surface, i.e., an island contour. It means that the island contour is ranged over \([-y_t,y_t]\) in the y-space. The + sign in the integrand denotes the outer half of the integration curve along the island contour, and the - sign denotes the inner half. The expression and the meaning of the y-average become more transparent with the use of the polar coordinates \((\rho ,\chi )\) presented in Eq. (A3) as follows.

$$\begin{aligned} \left\langle A \right\rangle _y = \left. \oint _\rho \frac{d\chi \, A}{\sqrt{1-\rho ^2 \sin ^2{\chi }}} \Bigg / \oint _\rho \frac{d\chi }{\sqrt{1-\rho ^2 \sin ^2{\chi }}} \right. . \end{aligned}$$
(76)

From Eq. (76) it is clear that the y-average is done along the entire curve of a specific magnetic island contour characterized by \(\rho\), i.e., the helical magnetic surface X.

Using Eqs. (66), (73), (74) and (76), we obtain an explicit expression of the classical susceptibility for the polarization shielding of the self-generated vortex flow from Eq. (65) as follows.

$$\begin{aligned} \chi _\textrm{cl} = \left[ G_0 (\rho ) + G_1 (\rho ) \right] k_w^2 \rho _{Ti}^2, \end{aligned}$$
(77)

where \(k_w \equiv 4S'/w\) denotes a characteristic wavenumber scale of the vortex flow, and the geometrical factors \(G_0\) and \(G_1\) are given by

$$\begin{aligned} G_0 (\rho )&= \left\langle \frac{x^2}{w^2}\right\rangle _y = \frac{E(\rho )}{K(\rho )} - 1 +\rho ^2, \end{aligned}$$
(78)
$$\begin{aligned} G_1( \rho )&= \left\langle \frac{1}{4}\left( \frac{kw}{2}\right) ^2 \sin ^2{(ky)}\right\rangle _y = \left( \frac{kw}{2}\right) ^2\frac{1}{3}\left[ (2\rho ^2-1)\frac{E(\rho )}{K(\rho )} + 1 -\rho ^2 \right] . \end{aligned}$$
(79)

For an explicit calculation of the neoclassical susceptibility \(\chi _\textrm{nc}\), we need to calculate the velocity space integrations for trapped and passing particles separately. The process could be simplified by using \((\mu ,\kappa )\) velocity space variables and switching the z-average and \(\kappa\)-integration as follows. We start with

$$\begin{aligned} \int d^3 v = \int _{\sigma >0} \frac{2\pi B d\mu d\mathcal {E}}{M^2 |v_\parallel |} + \int _{\sigma <0} \frac{2\pi B d\mu d\mathcal {E}}{M^2 |v_\parallel |}. \end{aligned}$$
(80)

Note that \(d\mu d\mathcal {E} = 2\epsilon \mu B_a d\mu d(\kappa ^2)\) from Eq. (30), and for trapped particles

$$\begin{aligned} \int _{-\pi }^{\pi } d\theta \int _{\kappa _0}^{1} d(\kappa ^2) = \int _{0}^{1} d(\kappa ^2) \int _{-\theta _t}^{\theta _t} d\theta = \int _{0}^{1} d(\kappa ^2) \int _{-\pi /2}^{\pi /2} \frac{2\kappa \cos {\vartheta } d\vartheta }{\sqrt{1-\kappa ^2 \sin ^2{\vartheta }}}, \end{aligned}$$
(81)

where \(\kappa _0 = \sin {(\theta /2)}\), while for passing particles

$$\begin{aligned} \int _{-\pi }^{\pi } d\theta \int _{1}^{\infty } d(\kappa ^2) = \int _1^{\infty } d(\kappa ^2) \int _{-\pi }^{\pi } 2d\vartheta . \end{aligned}$$
(82)

Here, we have used the bounce/transit angle \(\vartheta\) of which expressions given in Eqs. (33) and (34). Using Eqs. (31), (32), (69)-(76), (80)-(82), we obtain explicit expressions of the trapped and passing particle contributions to the neoclassical susceptibility \(\chi _\textrm{nc}\) as follows from Eq. (65).

$$\begin{aligned} \chi _\textrm{nc}^t&= \left[ 1.20 G_0 (\rho ) + 0.15 G_1 (\rho ) \right] \frac{q^2}{\sqrt{\epsilon }} k_w^2 \rho _{Ti}^2, \end{aligned}$$
(83)
$$\begin{aligned} \chi _\textrm{nc}^p&= \left[ 0.43 G_0 (\rho ) + 0.09 G_1 (\rho ) \right] \frac{q^2}{\sqrt{\epsilon }} k_w^2 \rho _{Ti}^2 . \end{aligned}$$
(84)

Adding Eqs. (83) and (84) together, we obtain an explicit expression of the neoclassical susceptibility for the polarization shielding of the self-generated vortex flow as follows.

$$\begin{aligned} \chi _\textrm{nc} = \chi _\textrm{nc}^t +\chi _\textrm{nc}^p = \left[ 1.63 G_0 (\rho ) + 0.24 G_1 (\rho ) \right] \frac{q^2}{\sqrt{\epsilon }} k_w^2 \rho _{Ti}^2. \end{aligned}$$
(85)

Here, the numerical coefficient 1.63 for \(G_0\) is the same as one for the neoclassical susceptibility for the tokamak zonal flow (Rosenbluth and Hinton 1998), consistent with the fact that both originate from the FOW effect by radial magnetic drift \(v_{dx}\). The small numerical coefficient 0.24 for \(G_1\), which originates from the helical angle component of the magnetic drift \(v_{dy} \propto \cos {\theta }\), is due to the even parity of \(v_{dy}\) in \(\theta\). Consequently, the helical angle component mainly gives mean precession of the orbit center and has a minor contribution to the orbit width.

Now, substituting Eqs. (77) and (85) into Eq. (64), we finally obtain an explicit expression of the residual vortex flow level (Choi and Hahm 2022)

$$\begin{aligned} \frac{\phi _\textrm{R}(X)}{\phi (t=0)} = \left. 1 \Bigg / \left[ 1+\frac{1.63G_0 (\rho ) + 0.24G_1 (\rho )}{G_0(\rho ) + G_1 (\rho )}\frac{q^2}{\sqrt{\epsilon }} \right] \right. . \end{aligned}$$
(86)

Note that in the limit of zero magnetic island perturbation \(\delta \psi \rightarrow 0\), which is equivalent to the zero magnetic island width \(w \rightarrow 0\), the geometrical factor \(G_1\) vanishes and we recover the widely-recognized Rosenbluth-Hinton residual level for a self-generated E\(\times\)B flow in a tokamak plasma (Rosenbluth and Hinton 1998). Recall that \(G_1 \propto w^2 \propto \tilde{\psi }\) from Eq. (79). In the presence of a magnetic island, due to the small numerical coefficient 0.24 for \(G_1\) in the neoclassical susceptibility \(\chi _\textrm{nc}\) shown in Eq. (85), the neoclassical enhancement of the polarization shielding of the self-generated vortex flow is weak. This leads to a higher residual vortex flow level compared to the Rosenbluth-Hinton as shown in Eq. (86). The thicker the magnetic island is, the higher the residual vortex flow level. We again emphasize that the non-trivial feature of the residual vortex flow level presented here purely comes from the geometrical property of the self-generated vortex flow contained in the wavevector \(\textbf{k}\) in the magnetic drift frequency \(\omega _\textrm{D} = \textbf{k} \cdot \textbf{v}_d\). The distortion of the particle trajectory by the magnetic island gives negligible effect.

5 Deformation of residual vortex flow

In the longer time scale \(\overline{\omega }_\textrm{D}^{-1}<t\), the secular magnetic drift frequency \(\overline{\omega }_\textrm{D}\) affect the vortex flow evolution. The toroidal precession results in the finite \(\overline{\omega }_\textrm{D}\), and it leads to the deviation of the orbit center trajectory, characterized by the drift surface \(\Omega\), from the helical magnetic surface X. This effect is quantified by the general finite surface deviation (FSD) factor

$$\begin{aligned} \Lambda \equiv \int _\Omega d\varphi \frac{\overline{\omega }_\textrm{D}}{\omega _\varphi }, \end{aligned}$$
(87)

where the expressions of the drift surface \(\Omega\) and the drift frequency \(\omega _\varphi\) has been presented in Sec. 3. Note that the secular magnetic drift frequency is, in general,

$$\begin{aligned} \overline{\omega }_\textrm{D} = S' \overline{v}_d k\sin {(ky)}. \end{aligned}$$
(88)

Therefore, substituting Eq. (88) and \(\omega _\varphi \simeq k\overline{v}_\textrm{d}\) into Eq. (87), we obtain the FSD factor for the toroidal regime as follows (Choi and Hahm 2022).

$$\begin{aligned} \Lambda \simeq -S' \cos {(ky)}. \end{aligned}$$
(89)

Meanwhile, in the shifted vortex regime and the shifted island regime, from Eqs. (47), (49), (52) and (87), we obtain

$$\begin{aligned} \Lambda \simeq 4S' \frac{d}{w} \eta \cos {\varphi } = 4S'\frac{d(x-d)}{w^2}. \end{aligned}$$
(90)

Comparing Eqs. (89) and (90), we find a crucial difference between the two cases in the FSD factor is large \(\Lambda \lesssim \mathcal {O}(1)\) in the toroidal regime, while it is much smaller by \(\Lambda \sim \mathcal {O}(d/w) \ll 1\) in the shifted vortex and the shifted island regimes. Here, we have applied a subsidiary ordering that \(S' \lesssim \mathcal {O}(1)\), that is, \(k_X^{-1} \lesssim w\), in addition to the basic ordering shown in Eq. (19). This indicates that a strong enough background vortex flow (shifted vortex regime) or averaged parallel streaming (shifted island regime) results in a significant reduction of the deviation of the drift surface from the magnetic surface.

Using the drift action-angle pair \((\Omega ,\varphi )\) and the FSD factor \(\Lambda\) presented in Eqs. (23) and (87), we rewrite Eq. (58), the bounce/transit-kinetic equation, as follows.

$$\begin{aligned} \frac{\partial h}{\partial t} + \omega _\varphi \left. \frac{\partial h}{\partial \varphi } \right| _\Omega + i \omega _\varphi \left. \frac{\partial \Lambda }{\partial \varphi } \right| _\Omega h =\frac{e}{T} F_0\overline{e^{iQ} J_0 \frac{\partial \phi }{\partial t}} + \overline{e^{iQ} N}F_0. \end{aligned}$$
(91)

Multiplying Eq. (91) by \(\exp {(i\Lambda )}\) and taking the drift average (Cary and Brizard 2009)

$$\begin{aligned}{}[A]\equiv \left. \oint _\Omega \frac{d\varphi }{\omega _\varphi } A \Bigg / \oint _\Omega \frac{d\varphi }{\omega _\varphi } \right. , \end{aligned}$$
(92)

we obtain the drift-averaged kinetic equation

$$\begin{aligned} \frac{\partial H}{\partial t} = \frac{e}{T}\left[ e^{i\Lambda } \overline{F_0 e^{iQ} J_0 \frac{\partial \phi }{\partial t}} \right] + \left[ e^{i\Lambda }\overline{e^{iQ} N F_0}\right] , \end{aligned}$$
(93)

where \(h = H \exp {(-i\Lambda })\), which gives a solution

$$\begin{aligned} g = e^{-iQ} e^{-i\Lambda } \left[ e^{i\Lambda } \overline{F_0 e^{iQ} J_0 \frac{e}{T}\phi } \right] + e^{-iQ} e^{-i\Lambda } \left[ e^{i\Lambda } \overline{e^{iQ} f_g (0)}\right] , \end{aligned}$$
(94)

where we have assumed that \(g_1\) has already been damped out in the intermediate time scale. Substituting Eq. (94) into the quasi-neutrality condition, Eq. (16), we obtain the general formal solution for the long-term flow potential as follows (Choi 2023).

$$\begin{aligned} \mathcal {D} \delta \phi = \mathcal {I}, \end{aligned}$$
(95)

where the dielectricity operator \(\mathcal {D}\) is defined as

$$\begin{aligned} \mathcal {D} \delta \phi&= \sum _{j=e,i} \mathcal {D}_j \delta \phi \nonumber \\&= \sum _{j=e,i} \frac{e}{T_j} \left\langle \int d^3 v \frac{F_{0j}}{n_{0}} \left\{ \delta \phi - J_0 e^{-iQ} e^{-i\Lambda } \left[ e^{i\Lambda } \overline{e^{iQ} J_0 }\phi \right] e^{iS} \right\} \right\rangle _z , \end{aligned}$$
(96)

and the source term is

$$\begin{aligned} \mathcal {I} = \frac{e}{T_i} \left\langle \int d^3 v \frac{F_{0i}}{n_0} J_0 e^{-iQ} e^{-i\Lambda }\left[ e^{i\Lambda } \overline{e^{iQ}}\chi _\textrm{cl}\phi (0)\right] e^{iS}\right\rangle _z. \end{aligned}$$
(97)

Note that \(\phi\) is outside of the bounce average in Eqs. (96) and (97), provided that it is independent of z in the long term as the GAM sidebands, originating from the flow compressibility due to the inhomogeneity of B along the reference magnetic field (that is, in the z-direction) in our system, were already damped by transit resonance (Sugama and Watanabe 2006b). Now, we obtain explicit expressions of the long-term potential by approximating Eqs. (96) and (97) for narrow (\(w_{\textrm{cr},e} \ll w \ll w_{cr,i}\)) and thick (\(w_{cr,i} \ll w\)) magnetic islands.

5.1 Narrow magnetic island

5.1.1 Weak background flow

In a narrow magnetic island \(w_{cr,e} \ll w \ll w_{cr,i}\) with a weak background E\(\times\)B vortex flow \(\Phi _0 \ll \Phi _{cr}\), ions and trapped electrons are in the toroidal regime and passing electrons are in the shifted island regime. Therefore, we have a large FSD factor \(\Lambda \lesssim \mathcal {O}(1)\) for ions, and then the ion FLR and FOW effects become subdominant in the dielectricity \(\mathcal {D}_i\) shown in Eq. (96). Since the fraction of the trapped electrons having a large FSD factor is small by \(\mathcal {O}(\sqrt{\epsilon })\) and the passing electrons have small FSD factor, we have \(|\mathcal {D}_i |\sim \mathcal {O}(1) \gg |\mathcal {D}_e |\). Therefore, what mainly happens is the homogenization of the residual vortex flow potential envelope on the tokamak magnetic surface \(\psi\) by the ion toroidal precession. Taking the flux surface average to Eq. (95) over the unperturbed magnetic surface, we obtain an explicit expression of the long-term potential envelope as follows.

$$\begin{aligned} \frac{\phi _\textrm{L}(\psi )}{\phi (t=0)} = k_w^2 \rho _{Ti}^2 \frac{J_0^2 (S')}{1-J_0^2(S')} \left[ \frac{4x^2}{w^2} + \left( \frac{kw}{2}\right) ^2 \frac{J_0(S')+J_2(S')}{2J_0(S')} \right] . \end{aligned}$$
(98)

Eq. (98) shows that the long-term flow level is much smaller than the residual vortex flow level in the intermediate time scale, according to the ordering \(|Q |\ll 1\) used for the long-wavelength vortex flow. More interestingly, Eq. (98) indicates that the residual vortex flow evolves to a zonal-vortex flow mixture (Choi and Hahm 2022) in a longer term,

$$\begin{aligned} \delta \phi = \phi _\textrm{L} (\psi ) e^{iS(X)}, \end{aligned}$$
(99)

consisting of the zonal envelope \(\phi _\textrm{L} (\psi )\) and the vortex eikonal part \(\exp {[iS(X)]}\).

5.1.2 Strong enough background flow

On the other hand, in the presence of a strong enough background E\(\times\)B vortex flow \(\Phi \gg \Phi _{cr}\), the ions and the trapped electrons are now in the shifted vortex regime, and consequently have reduced FSD factors \(\Lambda \sim \mathcal {O}(d/w) \ll 1\) from Eqs. (46) and (90), in comparison to the toroidal regime. Applying a subsidiary ordering \(e\Phi _\textrm{cr}/T \gg w_{\textrm{cr},e}/w\), we find that the passing electrons are still in the shifted island regime, grounded on the maximal ordering for the background E\(\times\)B flows \(e\Phi _0/T \sim \mathcal {O}(1)\) which is smaller than \(\Phi _{cr}w/w_{cr,e}\) (See Table 1.). The passing electrons have an even smaller FSD factor compared to ions, resulting in \(1 \gg |\mathcal {D}_i |\gg |\mathcal {D}_e |\).

Note that in the shifted vortex regime,

$$\begin{aligned} \langle d \rangle _v \equiv \left\langle \int d^3 v \frac{F_0}{n_0} d \right\rangle _z = 0, \end{aligned}$$
(100)

which means that we have no average deviation of the trajectories of the flow-carrying particles from the magnetic surface X. This property is a result of an exact cancellation of the contributions from the trapped and barely passing particles. As a consequence, the potential contour (\(\sim\) streamline) of the self-generated vortex flow in the \(x-y\) plane is maintained to be the same as the magnetic island contour X in the long term, up to the linear order of \(\mathcal {O}(d/w)\). In other words, the flow structure is unchanged from the concentric vortex monopole. Based on this observation, we readily obtain an explicit expression of the long-term flow level. From Eqs. (96) and (97), taking the flux surface average, we have

$$\begin{aligned} \frac{\phi _\textrm{L}(X)}{\phi (t=0)} = \frac{\chi _\textrm{cl}}{\chi _\textrm{cl} + \chi _\textrm{nc} + \chi _\textrm{d}}, \end{aligned}$$
(101)

where we have the drift susceptibility (Choi 2023)

$$\begin{aligned} \chi _\textrm{d} = \left\langle \langle [\Lambda ^2 ] \rangle _v - \langle [\Lambda ]^2 \rangle _v \right\rangle , \end{aligned}$$
(102)

which characterizes the enhancement of the polarization shielding of the residual vortex flow due to the magnetic precession, controlled by the large enough background vortex flow as shown in Eqs. (46) and (90). Here, electron contributions to \(\chi _\textrm{d}\) have been neglected since they give only minor corrections as explained. Then, an explicit expression of the drift susceptibility could be obtained as follows.

$$\begin{aligned} \chi _\textrm{d} = k_w^2 D_i^2 G_0 (\rho ), \end{aligned}$$
(103)

where

$$\begin{aligned} D \equiv \sqrt{\langle d^2 \rangle _v} \simeq 1.97 \frac{V_D}{V_E}\epsilon ^{1/4} w, \end{aligned}$$
(104)

captures the leading non-trivial FSD effect. In Eq. (104),

$$\begin{aligned} V_D \equiv \frac{T}{eBR}, \quad V_E \equiv \frac{4\Phi '}{Bw}, \end{aligned}$$
(105)

represent magnitudes of the toroidal precession and the background E\(\times\)B vortex flow, respectively. It is noteworthy that from Eqs. (103) and (104), we have decreasing drift susceptibility \(\chi _\textrm{d} \propto 1/V_E^2\) with the background flow velocity. It clearly reveals that a finite background vortex flow is beneficial to maintain the self-generated vortex flow. In the strong background flow limit \(V_E \rightarrow \infty\), we have a vanishing drift susceptibility \(\chi _\textrm{d} \rightarrow 0\). As a consequence, we recover the residual vortex flow \(\phi _\textrm{L} \rightarrow \phi _\textrm{R}\) even in the long term \(t>\overline{\omega }_\textrm{D}^{-1}\). That is, there is no further damping, i.e., shielding, of the residual vortex flow in the presence of a very strong background vortex flow.

5.2 Thick magnetic island

In the case of a thick magnetic island \(w \gg w_{\textrm{cr},i}\) in a collisionless tokamak plasma, we find similar features in the long-term evolution of the self-generated vortex flow with those in a narrow magnetic island studied in the previous subsection. (In reality, the parallel collisional relaxation along the magnetic island contour (Fitzpatrick 1995) enters as another defender of the long-term flow structure deformation in a thick magnetic island (Choi and Hahm 2022), but this effect will not be analyzed here.) The main difference is a significant trapped particle contribution over the passing particles and in accordance equal role of electrons with ions, except for a strong background vortex flow.

5.2.1 Weak background flow

In a thick magnetic island \(w \gg w_{\textrm{cr},i}\) with a weak background vortex flow \(\Phi \ll \Phi _\textrm{cr}\), we have trapped particles in the toroidal regime, and passing particles in the shifted island regime. Accordingly, from Eqs. (51), (89) and (90), we have much smaller FSD \(\Lambda \sim \mathcal {O}(d/w)\) for the passing particles compared to the trapped particles \(\Lambda \lesssim \mathcal {O}(1)\). As a result, we have a dominant trapped particle contribution to the plasma dielectricity \(\mathcal {D}\), which yields

$$\begin{aligned}{} & {} \frac{\phi _\textrm{L}(\psi )}{\phi (t=0)} = \frac{k_w^2 \rho _{Ti}^2}{1+T_i/T_e} \frac{J_0^2 (S')}{1-J_0^2(S')} \left[ \frac{4x^2}{w^2} + \left( \frac{kw}{2}\right) ^2 \frac{J_0(S')+J_2(S')}{2J_0(S')} \right] . \end{aligned}$$
(106)

That is, in the absence of a strong background vortex flow and a collisional relaxation, toroidal precession again leads to the formation of the zonal-vortex flow mixture in a thick magnetic island. The main difference from the narrow island case is that here the electrons also contribute to the dielectricity \(\mathcal {D}\) in addition to the ions as shown in the factor \(1+T_i/T_e\) in Eq. (106), which gives a lower mixture flow level compared to the one in a narrow magnetic island.

5.2.2 Moderately strong background flow

With a moderate background vortex flow \(\Phi _\textrm{cr} \ll \Phi \ll \Phi _\textrm{cr} w/w_{\textrm{cr},i}\) in a thick magnetic island, we have trapped particles in the shifted vortex regime while passing particles in the shifted island regime due to a stronger effect from the averaged streaming. Then, the FSD factor \(\Lambda\) for the passing particles is smaller than that for the trapped particles. As a result, we have a dominant trapped particle contribution to the long-term flow, which leads to Eq. (101), but with a different expression of the drift susceptibility

$$\begin{aligned} \chi _\textrm{d} = k_w^2 (D_i^2 + D_e^2) G_0 (\rho ), \end{aligned}$$
(107)

which contains contributions from both ions and electrons, where

$$\begin{aligned} D = 1.41 \frac{V_D}{V_E} \epsilon ^{1/4} w, \end{aligned}$$
(108)

contains only trapped particle contribution to the FSD factor.

5.2.3 Very strong background flow

In the presence of a very strong background vortex flow \(\Phi \gg \Phi _\textrm{cr} w/w_{\textrm{cr},i}\) in a thick magnetic island, ions and trapped electrons are in the shifted vortex regime, and passing electrons are in the shifted island regime. This is the same condition as the case of a strong background vortex flow in a narrow magnetic island. As a result, we have the same long-term potential shown in Eqs. (101) and (103).

Fig. 2
figure 2

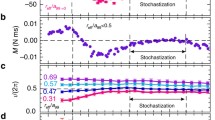
Levels of the residual vortex flow (black), long-term vortex with a moderately strong background vortex flow (blue), and the long-term zonal-vortex flow mixture with negligible background flow (red) in a thick magnetic island. They have been normalized by the Rosenbluth-Hinton residual flow level. Here, we have used an example parameter set \(S' = \pi /4\), \(e\Phi _0' / T = 1/8\), \(r=0.6\) m, \(R=3\) m, \(w=0.2\) m, \(T=10\) keV, \(B=2\) T

Full size image

Figure 2 shows an example of the levels of the residual vortex flow, long-term vortex flow in the presence of a moderately strong background vortex flow, and the long-term zonal-vortex flow mixture with negligible background flow in a thick magnetic island studied in Sec. 5.2. It is obvious that the background vortex flow plays a positive role in maintaining the self-generated vortex flow in the long term. It could be maintained in the same order as the Rosenbluth-Hinton residual flow level. We emphasize that the relative strength of the shearing rate (Hahm 2021) of the long-term vortex \(\delta \phi _\textrm{L}\) with background flow, the essential quantity for turbulence suppression (Biglari et al. 1990; Hahm and Burrell 1995), is the same as that of the potential level shown in Fig. 2. It is comparable to the shearing rate of the residual vortex \(\delta \phi _\textrm{R}\). This is because the inhomogeneities of the potential envelopes \(\phi _\textrm{R}\) and \(\phi _\textrm{L}\) are not drastic to violate the assumption of the eikonal representation, Eq. (5), that \(\partial _\rho ^2 \delta \phi \simeq -(\partial _\rho S)^2 \delta \phi\) so that the vortex flow shearing rate \(\omega _\textrm{E} \propto \delta \phi\) in this paper.

6 Discussions and Conclusion

As presented in the previous sections, a self-generated vortex flow in a magnetic island in a tokamak plasma shows a highly non-trivial behavior compared to one in a slab plasma. The reason is the magnetic drift \(\textbf{v}_d \propto \textbf{b} \times \varvec{\nabla } B\) by the inhomogeneous tokamak magnetic field \(B\simeq B_a (1-\epsilon \cos {\theta })\) which brings a mismatch between the vortex flow streamlines and the trajectories of the flow-carrying particles. In the intermediate time scale, the influence of this mismatch could be suppressed due to the presence of quasi-closed bounce/transit orbits that attenuate the effects of the magnetic drift, leading to only a moderate difference in the residual flow level \(\phi _\textrm{R}/\phi (t=0) = \chi _\textrm{cl}/(\chi _\textrm{cl} + \chi _\textrm{nc})\) from the well-known Rosenbluth-Hinton level \(1/(1+1.6q^2/\sqrt{\epsilon })\) (Rosenbluth and Hinton 1998) as shown in Eq. (64). However, in the long time scale, the slowly changing part of the magnetic drift \(\overline{\textbf{v}}_d\), the magnetic precession in the toroidal \(\zeta\) direction, eventually breaks the helical symmetry of the self-generated vortex flow \(\delta \phi (X)\). This toroidicity-induced symmetry breaking induces long-term collisionless flow damping toward a zonal-vortex flow mixture with \(\phi _\textrm{L}/\phi (t=0) \sim \chi _\textrm{cl}\) much smaller than the residual level as shown in Fig. 2.

Fig. 3
figure 3

Structure of the zonal-vortex flow mixture in the absence of a background flow. Here, we have used the same example parameter set as Fig. 2

Full size image

Figure 3 shows the structure of the zonal-vortex flow mixture that we arrive at after the long-term evolution \(t\gg \overline{\omega }_\textrm{D}^{-1}\) in the absence of a strong background flow \(\Phi _0 \ll \Phi _{cr}\). Note that the initial monopolar vortex evolves to a dipolar flow mixture in the long term. More interestingly, the flow mixture has a significant amount of open streamlines around the X-points which effectively reduces the isolated region inside the magnetic island. The particles, fluid elements, and perturbations including turbulence eddies can move in and out of the magnetic island by the advection along the mixture streamlines. This could be a candidate mechanism for experimentally observed turbulence invasion into the magnetic island through the X-point regions (Choi 2021a, b) which is different from the turbulence spreading (Hahm et al. 2004; Lin and Hahm 2004) across the E\(\times\)B flow shear layer (Wang 2007; Hahm and Diamond 2018; Ida 2018). Note that a recent analytic work based on the two-point decorrelation theory has proposed turbulence spreading into a magnetic island by a vanishing vortex flow shearing rate at the X-points as a candidate mechanism (Hahm 2021). Another interesting feature of the zonal-vortex flow mixture shown in Fig. 3 is that far from the X-point, the mixture flow forms a localized flow shear layer near the island separatrix. This kind of self-generated E\(\times\)B flow shear layer near the island boundary has also been found in numerical simulations (Ishizawa et al. 2019; Ishizawa and Nakajima 2009).

In general, the non-trivial features of the kinetic evolution of the self-generated vortex flow originating from the toroidicity-induced magnetic drift presented in this paper could affect the magnetic island evolution, described by the Rutherford equation (Rutherford 1973), through two different paths, closing the interaction loop among the magnetic island, the microturbulence, and the vortex flow. One is by the flow-induced (Cole and Fitzpatrick 2006; Park 2022) and the turbulent transport-induced (Fitzpatrick 2022) modifications of the tearing mode stability index \(\Delta '\), and another one is by the polarization current contribution (Smolyakov 1993; Fitzpatrick and Waelbroeck 2009). As long as the secular magnetic drift frequency \(\overline{\omega }_\textrm{D}\) for the long-term evolution of the self-generated vortex flow is faster than the inverse resistive diffusion time \(\eta /\mu _0 w^2\) which characterizes the nonlinear magnetic island growth in the Rutherford regime (Rutherford 1973), our results for the long-term evolution can readily be applied to the Rutherford equation, guaranteed from the time scale separation. When \(\overline{\omega }_\textrm{D}\) is too slow, the gyrokinetic evolution in the intermediate time scale toward the vortex flow residual shall be relevant, provided that the nonlinear magnetic island evolution is still slower than the ion bounce frequency \(\omega _{bi}\). It is worth noting that the ordering for the validity of the fluid description of the magnetic island is \(\omega \gg \omega _{ti}\) (Smolyakov et al. 2007). Note that the toroidicity-induced magnetic drift has also been found to affect the neoclassical tearing mode threshold (Imada 2018; Dudkovskaia 2021), and the toroidal precession works together with the E\(\times\)B shear flow suppressing turbulent transport (Choi and Hahm 2016).

The long-term damping of the self-generated vortex flow by the toroidicity-induced helical symmetry breaking with a structural change to a zonal-vortex mixture makes it harder for the turbulence-induced modulational growth (Chen et al. 2000; Champeaux and Diamond 2001; Diamond 2001) (self-generation) of the vortex flow to overcome the flow damping. As a consequence, we have a higher transition threshold to a locally enhanced confinement state in the vicinity of a tokamak magnetic island, compared to the zonal flow-triggered transition (Diamond 2005). In the realistic tokamak plasmas, the parallel collisional relaxation of the flow would change back the flow structure from the zonal-vortex mixture to the concentric vortex (Choi and Hahm 2022). But in terms of the flow amplitude, this is a negative synergism.

Fortunately, in the presence of a strong enough background vortex flow \(e\Phi _0/T \gg \epsilon w/r\), the long-term flow deformation and damping are significantly reduced so that the long-term flow structure is maintained to be the same with the concentric vortex up to \(\mathcal {O}(d/w)\), and the flow level \(\phi _\textrm{L}/\phi (t=0) \sim \chi _\textrm{cl}/(\chi _\textrm{cl}+\chi _\textrm{nc}+\chi _\textrm{d})\) remains comparable to the residual vortex flow level as also shown in Fig. 2. In addition, the parallel collisional relaxation of the long-term flow is also prevented, and the dominant collisional flow damping becomes a slower one by the neoclassical friction between trapped and passing particles (Hinton and Rosenbluth 1999). Consequently, the background E\(\times\)B vortex flow not only suppresses microturbulence by itself but also helps the triggering of the transition to a locally enhanced confinement state. This kind of positive synergism between the profile-induced and the turbulence-induced E\(\times\)B flows has been previously studied for the case of tokamak zonal flows (Kagan and Catto 2009; Landreman and Catto 2010) in terms of the finite orbit width (FOW) effect. For our case of the tokamak vortex flow the synergism appears in the finite surface deviation (FSD) of the flow streamlines from the magnetic island contours.

The presented theory suggests that a thick magnetic island is better for the internal transport barrier formation with a local enhancement of the confinement than a narrow island, taking into account experimental observations (Ida 2002, 2004) and simulation results (Ishizawa 2012) that a significant background electric field shear appears only for thick enough magnetic islands. However, the global tokamak confinement is likely degraded with the thick magnetic island due to the profile flattening over the wide radial domain inside the island. A strategy to overcome this disadvantage of a thick magnetic island could be mitigation or suppression of the magnetic island by the application of the electron cyclotron current drive (ECCD) (La Haye 2006; De Lazzari and Westerhof 2009; Kim 2015) or the resonant magnetic perturbation (Scoville and La Haye 2003; Park 2011; Paz-Soldan 2014) after achieving the internal transport barrier. Owing to the hysteresis of the transport barrier in response to the external input (Thomas 1998; Hubbard 2002; Malkov and Diamond 2008; Kim 2011; Miki 2013), one could minimize the shortcoming of a thick magnetic island maintaining strength. The findings from the theory and experiments also propose that the magnetic island boundary is a prominent location for transition to a locally enhanced confinement state, not only due to a significant background E\(\times\)B flow shear suppression of turbulent transport but also due to an easier triggering of the bifurcation via the vortex flow self-generation. For reference, the magnetic island boundary has also been considered a prominent location for the profile-induced polarization current generation (Fitzpatrick et al. 2006).

An important extension of our theory to be done in the near future would be the inclusion of the profile inhomogeneities other than the background E\(\times\)B flow. From a gyrokinetic point of view, it should enter through the unperturbed distribution function \(F_0\) which could be extended from the Maxwellian to the shifted local Maxwellian considering inhomogeneous background density \(n_0\), temperature T and parallel flow \(u_\parallel\). Note that the background parallel flow \(u_\parallel\) itself, directly related to the background parallel current \(j_\parallel\), does not affect the time evolution of the self-generated vortex flow considering \(\cdot \varvec{\nabla } F_0\) in the gyrokinetic Vlasov equation, Eq, (13), unless it is comparable to or faster than the parallel thermal velocity. Only its shear is important. Also, the natural frequency of a magnetic island \(\omega _0\), related to the density and temperature gradients (Fitzpatrick et al. 2001), can be absorbed into the helical angle as \(ky \rightarrow m\theta - n\zeta - \omega _0 t\), going to the frame co-moving with the magnetic island. Therefore, only its shear, or in other words the second-order gradients of the density and temperatures, gives a non-trivial change to the dynamics of the self-generated vortex flow. Another important extension would be considering the intermediate \(k_X \rho _i< 1 < Q_i\) and the short wavelength \(1<k_X \rho _i\) vortex flow regimes (Wang and Hahm 2009), to be applicable to future high-performance tokamak plasmas with magnetic island.

In addition, it is desirable to pursue theoretical studies on the competition between self-generations of the tearing parity island flow and the twisting parity vortex flow from turbulence. Note that the vortex flow defined by a flux-function potential \(\delta \phi (X)\) is a twisting parity flow with an even parity potential \(\delta \phi (-x) = \delta \phi (x)\). The flow keeps the twisting parity even after deformation to a zonal-vortex mixture (recall Eqs. (98) and (106)). While robust self-generation of this twisting parity vortex flow has been observed in gyrokinetic simulations (Hornsby 2012; Navarro 2017; Muto et al. 2022; Li 2023), several fluid simulations (Sato and Ishizawa 2017; Ishizawa et al. 2019; Hu 2020; Zhang 2023) have reported self-generation of a tearing parity E\(\times\)B flow in the context of the parity instability and nonlinear magnetic island formation. Also, neoclassical profile solutions have shown tearing parity background E\(\times\)B flow (Kwon 2018; Dudkovskaia 2021). At this point, note that experiments have observed E\(\times\)B flows of either parity in different machines and plasma conditions (Ida 2001; Estrada 2016, 2021). A deeper understanding of control parameters and conditions for having an E\(\times\)B flow of specific parity is needed.

Since the presented work is an electrostatic gyrokinetic theory, it would be good to make a brief remark on the roles of turbulent magnetic fluctuations on the E\(\times\)B flow self-generation around a magnetic island. While tokamak microturbulence is intrinsically electrostatic (Horton 1999), its magnetic field component could have interesting influences on E\(\times\)B flows in the magnetic island region. It has been found from gyrokinetic simulations that electromagnetic ITG turbulence nonlinearly produces small-scale tearing modes (Doerk 2011; Hatch 2013) which is a natural consequence of parity mixing (Sato and Ishizawa 2017). The small-scale tearing parity magnetic fluctuations could form a stochastic magnetic field and act toward a decrease of a self-generated vortex flow, either by Maxwell stress (Ishizawa et al. 2019; Cao and Diamond 2022) or kinetic damping (Terry 2013). Or, the small-scale tearing modes could merge to self-generate a large-scale magnetic island (Ishizawa and Nakajima 2010; Ishizawa and Waelbroeck 2013) with accompanied E\(\times\)B flow. The turbulent magnetic fluctuations could work either way depending on the situation.

To summarize, we have presented a recently developed gyrokinetic theory of time evolution of an E\(\times\)B vortex flow self-generated from turbulence in a tokamak magnetic island. In the intermediate time scale slower than the ion bounce motion but faster than the magnetic precession, the initially self-generated vortex flow approaches a residual level after fast collisionless damping, which is higher than the well-known Rosenbluth-Hinton level due to the geometrical property of the vortex flow wavevector originating from the magnetic island perturbation. This residual vortex flow level is not affected by the background vortex flow. In the long time scale slower than the magnetic precession, the residual vortex flow undergoes further damping with deformation toward a zonal-vortex mixture due to a toroidicity-induced breaking of the helical symmetry. In the presence of a strong enough background vortex flow with \(e\Phi _0 /T>\epsilon w/r\), the long-term flow damping and deformation is significantly reduced as a result of the reduction of the finite surface deviation (FSD) of the vortex flow streamlines from the magnetic island contours. Subsequently, the parallel collisional relaxation of the long-term flow along the magnetic island contours is also prevented which further improves the situation. The positive roles of the background vortex flow in sustaining the self-generated vortex flow lead to a more favorable condition for the transition to an enhanced confinement state in the vicinity of a magnetic island, because the net self-generation rate of the vortex flow plays is crucial in triggering the transition. The synergism between the two vortex flows that we have addressed in this paper could work together with previously suggested mechanisms relying on the profile-induced background E\(\times\)B flow, converging to the same statement with previous works (Ida 2015, 2020) that the magnetic island boundary region is a prominent location for bifurcations.