Abstract
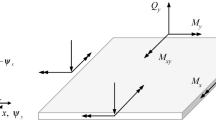
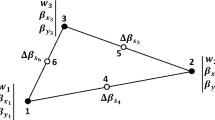
Despite the high importance of orthotropic bending plate, up to now, only a few studies have been performed based on the analytical solution for analysis of these structures. In this paper, an efficient element is proposed by utilizing the hybrid-Trefftz method to analyze structures. The proposed quadrilateral element (QHT-OR) has four nodes and twelve degrees of freedom. Moreover, two independent displacement fields are defined for inner and boundaries of element. The internal field is achieved by solving the governing equation of orthotropic plates. Furthermore, the boundary field is related to the nodal degrees of freedom by using interpolation functions. It should be mentioned that the interpolation functions of two-node beam elements are applied for boundary field. The responses obtained by using numerical tests show the high accuracy of the QHT-OR element even for coarse meshes.








Similar content being viewed by others
References
Albuquerque E, Sollero P, Venturini W, Aliabadi M (2006) Boundary element analysis of anisotropic Kirchhoff plates. Int J Solids Struct 43:4029–4046
An C, Gu J, Su J (2016) Exact solution of bending problem of clamped orthotropic rectangular thin plates. J Braz Soc Mech Sci Eng 38:601–607
Bao G, Jiang W, Roberts J (1997) Analytic and finite element solutions for bending and buckling of orthotropic rectangular plates. Int J Solids Struct 34:1797–1822
Bhaskar K, Kaushik B (2004) Simple and exact series solutions for flexure of orthotropic rectangular plates with any combination of clamped and simply supported edges. Compos Struct 63:63–68
Choo YS, Choi N, Lee BC (2010) A new hybrid-Trefftz triangular and quadrilateral plate elements. Appl Math Model 34:14–23
Dong C, Lo S, Cheung Y, Lee K (2004) Anisotropic thin plate bending problems by Trefftz boundary collocation method. Eng Anal Boundary Elem 28:1017–1024
dos Reis A, Lima Albuquerque É, Luiz Torsani F, Palermo L Jr, Sollero P (2011) Computation of moments and stresses in laminated composite plates by the boundary element method. Eng Anal Boundary Elem 35:105–113
dos Reis A, Lima Albuquerque É, Palermo Júnior L (2013) The boundary element method applied to orthotropic shear deformable plates. Eng Anal Boundary Elem 37:738–746
Herrera I (1984) Boundary methods: an algebraic theory. Pitman Advanced Pub. Program, New York
Jirousek J, Guex L (1986) The hybrid-Trefftz finite element model and its application to plate bending. Int J Numer Methods Eng 23:651–693
Jirousek J, Leon N (1977) A powerful finite element for plate bending. Comput Methods Appl Mech Eng 12:77–96
Jirousek J, N’Diaye M (1990) Solution of orthotropic plates based on p-extension of the hybrid-Trefftz finite element model. Comput Struct 34:51–62
Mbakogu F, Pavlović M (2000) Bending of clamped orthotropic rectangular plates: a variational symbolic solution. Comput Struct 77:117–128
Petrolito J (1996) Triangular thick plate elements based on a hybrid-Trefftz approach. Comput Struct 60:883–894
Petrolito J (2011) Analysis of orthotropic thick plates using hybrid-Trefftz elements. In: Australian conference of the mechanics of structures and materials, Melbourne, pp 105–109
Petrolito J (2014) Vibration and stability analysis of thick orthotropic plates using hybrid-Trefftz elements. Appl Math Model 38:5858–5869
Qin Q-H (2005) Trefftz finite element method and its applications. Appl Mech Rev 58:316–337
Reddy JN (2004) Mechanics of laminated composite plates and shells: theory and analysis. CRC Press, Boca Raton
Rezaiee-Pajand M, Karkon M (2012) Two efficient hybrid-Trefftz elements for plate bending analysis. Latin Am J Solids Struct 9:43–67
Rezaiee-Pajand M, Karkon M (2013) An effective membrane element based on analytical solution. Euro J Mech A/Solids 39:268–279
Rezaiee-Pajand M, Karkon M (2014) Two higher order hybrid-Trefftz elements for thin plate bending analysis. Finite Elem Anal Des 85:73–86
Rezaiee-Pajand M, Yaghoobi M, Karkon M (2012) Hybrid Trefftz formulation for thin plate analysis. Int J Comput Methods 9(04):1250053. https://doi.org/10.1142/S0219876212500533
Shi G, Bezine G (1988) A general boundary integral formulation for the anisotropic plate bending problems. J Compos Mater 22:694–716
Shimpi R, Patel H (2006) A two variable refined plate theory for orthotropic plate analysis. Int J Solids Struct 43:6783–6799
Sladek J, Sladek V, Zhang C, Krivacek J, Wen P (2006) Analysis of orthotropic thick plates by meshless local Petrov–Galerkin (MLPG) method. Int J Numer Methods Eng 67:1830–1850
Thai H-T, Kim S-E (2012) Analytical solution of a two variable refined plate theory for bending analysis of orthotropic Levy-type plates. Int J Mech Sci 54:269–276
Timoshenko SP, Woinowsky-Krieger S (1959) Theory of plates and shells. McGraw-Hill, New York
Timoshenko S, Woinowsky-Krieger S, Woinowsky-Krieger S (1959) Theory of plates and shells, vol 2. McGraw-Hill, New York
Ugural A (1999) Stresses in plates and shells. McGraw-Hill, New York
Wang J, Huang M (1991) Boundary element method for orthotropic thick plates. Acta Mech Sin 7:258–266
Wang J, Schweizerhof K (1996) Fundamental solutions and boundary integral equations of moderately thick symmetrically laminated anisotropic plates. Commun Numer Methods Eng 12:383–394
Wang C, Wang X, Zhang X, Hu P (2017) Assumed stress quasi-conforming technique for static and free vibration analysis of Reissner-Mindlin plates. Int J Numer Methods Eng 112(4):303–337
Wilt T, Saleeb A, Chang T (1990) A mixed element for laminated plates and shells. Comput Struct 37:597–611
Wünsche M, García-Sánchez F, Sáez A (2012) Analysis of anisotropic Kirchhoff plates using a novel hypersingular BEM. Comput Mech 49:629–641
Zhang Y, Kim K (2004) Two simple and efficient displacement-based quadrilateral elements for the analysis of composite laminated plates. Int J Numer Methods Eng 61:1771–1796
Author information
Authors and Affiliations
Corresponding author
Appendix: The Interpolation Matrix of the Boundary Displacement Field
Appendix: The Interpolation Matrix of the Boundary Displacement Field
The entries of the interpolation matrix of the boundary displacement field (45) have the coming appearance:
Rights and permissions
About this article
Cite this article
Karkon, M., Rezaiee-Pajand, M. Finite Element Analysis of Orthotropic Thin Plates Using Analytical Solution. Iran J Sci Technol Trans Civ Eng 43, 125–135 (2019). https://doi.org/10.1007/s40996-018-0128-x
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s40996-018-0128-x