Skip to main content
SpringerLink
Log in

Endogenous market structures in a mixed oligopoly with a public firm whose managerial contract is based on welfare and bargaining over the managerial contract of a private firm

  • Published:
Economia Politica Aims and scope Submit manuscript

Abstract

We consider the endogenous choice problem of strategic contracts for public and private firms in a managerial mixed duopoly with differentiated goods. Focusing on the situation wherein the weighted sum of social welfare and quantity is adopted as its delegation contract within the public firm, we investigate the situation wherein the managerial delegation contract of the public firm is determined by maximizing social welfare, which is equal to the objective function of its owner, and the managerial delegation contract of the private firm is determined by bargaining over the content of the managerial delegation contract between its owner and manager. This paper clarifies that the equilibrium market structure depends on the bargaining power of the manager within the private firm. More concretely, when the bargaining power is sufficiently low, no equilibrium market structure exists. Furthermore, when the bargaining power is moderate (sufficiently high), the game wherein the public firm chooses a quantity contract and the private firm chooses a price contract and (both firms choose price contracts) becomes the unique equilibrium market structure.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

Notes

  1. Barros (1995) is a seminal paper on a mixed duopoly with a managerial delegation; he considered both the strategic effects and the asymmetric information of the firms’ sales delegation contracts in the fashion of Fershtman and Judd (1987), Sklivas (1987), and Vickers (1985). Subsequently, White (2001) and Bárcena-Ruiz (2009) consider a similar problem after Barros (1995).

  2. In the recent works on the endogenous selection problem of strategic contracts, there exist Scrimitore (2013) which considered the case of production subsidization, Haraguchi and Matsumura (2014) with foreign investors, and Haraguchi and Matsumura (2016) with multiple public firms and private firms.

  3. In their recent work, by reflecting modern corporate governance clauses, Van Witteloostuijn et al. (2007) studied the bargaining between a private firm’s owner and manager over sales delegation parameters in order to explain how the disclosure of managerial delegation contracts prescribed in modern corporate governance affects market outcomes. More specifically, they showed that when the sales delegation contract is considered, the relative bargaining power of managers to owners is positively associated with social welfare, while it is negatively associated with each firm’s profit.

  4. More precisely, although the delegation contract of the public firm is slightly different from that in Heywood and Ye (2009), it is common that social welfare, which is equal to the payoff of the public firm, is similarly reflected to their delegation contracts in both the models. It is natural that the delegation contract of the public firm’s manager includes social welfare since the payoff of the owner of the public firm is equal to social welfare. In Japan, although the public firms which are located in each local area mainly conducts the water and sever works system in the relevant area, their businesses were able to be consigned to a local private firm by revising the Water Supply Act of Japan in 2001. Similarly, in the water and sever works system, the public firm delegates the managerial decision to the relevant private firm through the delegation contracts such that social welfare enhances in France and the United States, too. In particular, in the Japanese water and sever works system, the owner of the local public firm delegates the managerial decision into the manager of her relevant firm through its delegation contract. Then, the owner of the local private firm delegates the managerial contract such that the raise in social welfare is included, which is provided by the owner of the public firm in such a industry.

  5. Compared with Nakamura (2015b), this fact yields different results for the equilibrium market structure when the relative bargaining power of the manager to that of the owner within the private firm becomes above a threshold value.

  6. Although we define this in detail in Sect. 3, we suppose that the i–j game means the subgame in which the public firm chooses the i contract and the private firm chooses the j contract, \(\left( i = p, q \right)\).

  7. As described below, the partial intuition behind this result is given by the strength of the “production substitution effect.” More precisely , when the relative bargaining power of the manger of the private firm manager to that of the owner is relatively high, since such a effect is weaker in this paper than in Nakamura (2015b), the public firm owner selects its quantity contract if the private firm selects its price contract.

  8. Therefore, in all the areas of the relative bargaining power and of the degree of product differentiation, the q–p game is not socially preferable market structure with respect to social welfare. This fact is also strikingly different from Nakamura (2015b).

  9. Moreover, the long numerators in the payoffs of the owners in the four games are arranged and located in the "Appendix".

  10. Throughout this paper, we restrict the interval of parameter \(\delta\) to \(\left( 0, 1 \right)\), implying that the goods produced by firms 0 and 1 are substitutable. In addition, in order for firm 1 to be active in the four games, we assume \(\alpha _{1} - \alpha _{0} \delta - c \left( 1 - \delta \right) > 0\). This assumption is equivalent to \(\alpha - c > 0\) if \(\alpha := \alpha _{0} = \alpha _{1}\), implying that market size \(\alpha\) is sufficiently large.

  11. In Lambertini (2000a, b), although \(\pi _{i} + \theta _{i} q_{i}\) is adopted within firm i as another type of sales delegation, it was shown that the effect of \(\pi _{i} + \theta _{i} q_{i}\) on the market outcomes are similar to that in the case of Nakamura (2015b) as in Fershtman and Judd (1987), Sklivas (1987), and Vickers (1985). Thus, the slight change of the delegation type from \(\theta _{i} \pi _{i} + \left( 1 - \theta _{i} \right) S_{i}\) to \(\pi _{i} +\theta _{i} q_{i}\) within private firm i does not alter the equilibrium market structure so much. Thus,. such a change does not influences the equilibrium market outcomes and the equilibrium market structures so much.

  12. This fact can be supported by the assumption that the payoff to the manager of firm i is defined as \(\lambda _{i} + \mu _{i} V_{i}\), for some real number \(\lambda _{i}\) and some positive real number \(\mu _{i}\), \(\left( i = 0, 1 \right)\). In addition, Heywood and Ye (2009) investigated the situation wherein the delegation contract within the public firm is the weighted sum of social welfare and its profit. In this paper, for tractability, we suppose the weighted sum of social welfare and the quantity of public firm 0 as its delegation contract.

  13. The bargaining between the owner and manager within firm 1 is modeled on the generalized Nash bargaining solution in Binmore et al. (1986). In addition, more recently, in particular within the private firm, such a bargaining à la Van Witteloostuijn et al. (2007) reflects the modern phenomenon that the opportunistic behavior by her manager is problematic.

  14. More precisely, public firm 0’s manger determines her delegation parameter with respect to the maximization of social welfare, while the delegation parameter is determined through the bargaining over the delegation parameter within private firm 1.

  15. Note that \(W^{ij}\), which denotes social welfare in the i–j game, is defined as the sum of \(CS^{ij}\) and \(PS^{ij} \equiv \pi ^{ij}_{0} + \pi ^{ij}_{1}\), \(\left( i, j = p, q \right)\).

  16. Under the setting of this paper, we give the ranking order of consumer surplus and producer surplus among the four games by assuming \(\alpha _{0} = \alpha _{1} = a\). Thus, when \(\alpha _{0} = \alpha _{1} = a\), we have

    • \(PS^{pp}> PS^{pq}> PS^{qq} > PS^{qp}\).

    • \(CS^{qq}> CS^{pq}> CS^{qp} > CS^{pp}\).

    Therefore, the ranking orders of social welfare which is equal to the payoff of the owner of public firm 0 is not explained by the sole ranking order of consumer surplus and producer surplus among the four games, respectively.

  17. Similar to this paper, also in Nakamura (2015b), when \(\alpha _{0} = \alpha _{1} = a\), we have

    • \(PS^{qp}_{A}> PS^{pq}_{A} > \max \left\{ PS^{qq}_{A}, PS^{pp}_{A} \right\}\)

    • \(CS^{qp}_{A} > \max \left\{ CS^{qq}_{A}, CS^{pp}_{A} \right\}\) and \(\min \left\{ CS^{qq}_{A}, CS^{pp}_{A} \right\} > CS^{pq}_{A}\).

    Note that when \(\delta\) and \(\gamma\) are sufficiently high, \(CS^{qq}_{A} > CS^{pp}_{A}\), whereas \(CS^{pp}_{A} > CS^{qq}_{A}\), otherwise. Therefore, similar to the case of in this paper, the ranking orders of social welfare which is equal to the owner of public firm 0 is not explained by the sole ranking order of consumer surplus and producer surplus among the four games, respectively.

  18. In Nakamura (2015b), under the setting that \(\alpha _{0} = \alpha _{1} = a\), al the equilibrium market outcomes.

  19. We can recognize that \(\theta ^{pq}_{0}\) is the lowest among those in the four games from Lemma 1. When \(\gamma\) is sufficiently low (near zero), the production substitution effect works similarly to Nakamura (2015b).

  20. Although Nakamura (2015a) considered the situation that the weighted sum of social welfare and the difference between the consumer surplus and the producer surplus is the delegation contract of the public firm, while the delegation parameter of the private firm is determined through the maximization of the sales delegation contract à la Fershtman and Judd (1987), Sklivas (1987), and Vickers (1985) without bargaining over it, he showed that there are no equilibrium market structures under the pure strategic contract class for all areas of parameters. In addition, when the rival firm’s strategic contract is fixed, the optimal strategic contract of the relevant firm is the same between this paper and Nakamura (2015a).

  21. From easy calculations, we have

    $$\begin{aligned} \left( \partial \theta ^{qq}_{1} / \partial \gamma \right) \gtreqless \left( \partial \theta ^{qp}_{1} / \partial \gamma \right) \iff \delta ^{4} + \gamma ^{2} \delta ^{4} - 2 \gamma (8 - 8 \delta ^{2} + \delta ^{4}) \gtreqless 0. \end{aligned}$$

    Thus, when \(\gamma\) is more than a moderate value, which depends on \(\delta\), \(\partial \theta ^{qp}_{1} / \gamma > \partial \theta ^{qq}_{1} / \partial \gamma\).

  22. In this case, the p–q game does not become the equilibrium market structure in this paper, which is different from Nakamura (2015b), because the strength of the production substitution effect is different between this paper and Nakamura (2015b).

  23. In fact, from easy calculations, we get \(\partial \left( q^{qp}_{0} - q^{pp}_{0}\right) / \partial \gamma < 0\) and \(\partial \left( q^{pp}_{1} - q^{qp}_{1} \right) / \partial \gamma < 0\) if \(\gamma\) is sufficiently high.

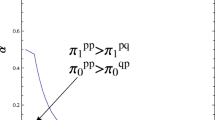
  24. Note that in Nakamura (2015b), \(\delta\) is sufficiently low, there exists the area such that the equilibrium market structure coincides the socially preferable market structure, i.e., the p–q game. As in this paper, in the case wherein the owner of the public firm provided to his manager to the linear weighted sum of social welfare and the owner of the public firm provided to his manager to the sales delegation contract, the government should caution the free selection of the strategic contracts by the owners of both the public firm and the private firm from the viewpoint of social welfare in the case wherein \(\gamma\) is over relatively high.

  25. Result 2. Proposition 1 in Nakamura (2015b)

    In a managerial mixed duopoly, where only the manager of private firm 1 can have a positive bargaining power relative to that of the owner in the determination of its sales delegation parameter, the equilibrium market structures, classified using \(\delta\) and \(\gamma\), are observed in the following scenarios:

    $$\begin{aligned} {\left\{ \begin{array}{ll} {\text{the}}\ p{\text{-}}q\ {\text{game}}& {} \quad {\text{if}} \; \gamma \left[ 8 - 8 \delta ^{2} + \delta ^{4} - 4 (2 - \delta ^{2}) \sqrt{1 - \delta ^{2}} \right] / \delta ^{4}, \\ {\text{the}}\ p{\text{-}}q\ \text{game} \,{\text{and the }} q{\text{-}}q \,{\text{game}}& {}\quad {\text{if}} \; \gamma = \left[ 8 - 8 \delta ^{2} + \delta ^{4} - 4 (2 - \delta ^{2})\sqrt{1 - \delta ^{2}} \right] / \delta ^{4}, \\ {\text{Nothing}},& {}\quad {\text{otherwise}}. \end{array}\right.} \end{aligned}$$

  26. Strictly speaking, although it was shown that the q–q game can become the equilibrium market structure in Nakamura (2015b), this phenomenon is a measure-zero event: \(\gamma = \left[ 8 - 8 \delta ^{2} + \delta ^{4} - 4 \sqrt{1 - \delta ^{2}} \left( 2 - \delta ^{2} \right) \right] / \delta ^{4}\).

  27. Nakamura (2015b) which considered the in a managerial mixed duopoly à la Fershtman and Judd (1987), Sklivas (1987), and Vickers (1985) showed that the equilibrium market structure, i.e., the p–q game coincides with the socially preferable market structure with respect to social welfare, when \(\gamma\) is sufficiently low, not zero.

  28. Moreover, we have \(d \theta ^{pp}_{0} \left( \theta _{1} \right) / d \theta _{1} = - (\delta - \delta ^{3}) / (4 - 3 \delta ^{2}) < 0\) and \(d \theta ^{pp}_{1} \left( \theta _{0} \right) / d \theta _{0} = \gamma \lesseqgtr \delta ^{2} / (2 - \delta ^{2})\).

  29. Moreover, we have \(d \theta ^{pq}_{0} \left( \theta _{1} \right) / d \theta _{1} = \delta / (4 - 3 \delta ^{2}) > 0\) and \(d \theta ^{pq}_{1} \left( \theta _{0} \right) / d \theta _{0} = - \gamma \delta < 0\).

  30. Moreover, we have \(d \theta ^{qp}_{0} \left( \theta \right) / d \theta _{1} = - (\delta - \delta ^{3}) / (4 - 3 \delta ^{2}) < 0\) and \(d \theta ^{qp}_{1} \left( \theta _{0} \right) / d \theta _{0} = - \gamma \delta / (1 - \delta ^{2}) < 0\).

  31. Moreover, we have \(d \theta ^{qq}_{0} \left( \theta _{1} \right) / d \theta _{1} = \delta (1 - \delta ^{2}) / (4 - 3 \delta ^{2}) > 0\) and \(d \theta ^{qq}_{1} \left( \theta _{0} \right) / d \theta _{0} = - \left[ \delta ^{3} + \gamma \delta (2 - \delta ^{2}) \right] /2 (1 - \delta ^{2})) < 0\).

References

  • Bárcena-Ruiz, J. C. (2009). The decision to hire managers in mixed markets under bertrand competition. Japanese Economic Review, 60, 376–388.

    Article  Google Scholar 

  • Barros, F. (1995). Incentive schemes as strategic variables: An application to a mixed duopoly. International Journal of Industrial Organization, 13, 373–386.

    Article  Google Scholar 

  • Binmore, K., Rubinstein, A., & Wolinsky, A. (1986). The nash bargaining solution in economic modelling. RAND Journal of Economics, 17, 176–188.

    Article  Google Scholar 

  • Chirco, A., Colombo, C., & Scrimitore, M. (2014). Organizational Structure and the choice of price vs. quantity in a mixed duopoly. Japanese Economic Review, 65, 521–542.

    Article  Google Scholar 

  • Dixit, A. (1979). A model of duopoly suggesting a theory of entry barriers. Bell Journal of Economics, 10, 20–32.

    Article  Google Scholar 

  • Fershtman, C., & Judd, K. (1987). Equilibrium incentives in oligopoly. American Economic Review, 77, 927–940.

    Google Scholar 

  • Haraguchi, J., & Matsumura, T. (2014). Price versus quantity in a mixed duopoly with foreign penetration. Research in Economics, 68, 338–353.

    Article  Google Scholar 

  • Haraguchi, J., & Matsumura, T. (2016). Cournot-bertrand comparison in a mixed oligopoly. Journal of Economics, 117, 117–136.

    Article  Google Scholar 

  • Heywood, J. S., & Ye, G. (2009). Delegation in a mixed oligopoly: The case of multiple private firms. Managerial and Decision Economics, 30, 71–82.

    Article  Google Scholar 

  • Lambertini, L. (2000a). Extended games played by managerial firms. Japanese Economic Review, 51, 274–283.

    Article  Google Scholar 

  • Lambertini, L. (2000b). Strategic delegation and the shape of market competition. Scottish Journal of Political Economy, 47, 550–570.

    Article  Google Scholar 

  • Matsumura, T., & Ogawa, A. (2012). Price versus quantity in a mixed duopoly. Economics Letters, 116, 174–177.

    Article  Google Scholar 

  • Nakamura, Y. (2011a). Bargaining over managerial delegation contracts and merger incentives with asymmetric costs. Research in Economics, 65, 47–61.

    Article  Google Scholar 

  • Nakamura, Y. (2011b). Bargaining over managerial delegation contracts and merger incentives in an international oligopoly. Manchester School, 79, 718–739.

    Article  Google Scholar 

  • Nakamura, Y. (2015a). Endogenous choice of strategic variables in an asymmetric duopoly with respect to the demand functions that firms face. International Review of Economics and Finance, 35, 262–277.

    Article  Google Scholar 

  • Nakamura, Y. (2015b). Endogenous choice of strategic contracts in a mixed duopoly with bargaining over managerial delegation contracts. IAustralian Economic Papers, 54, 121–134.

    Article  Google Scholar 

  • Scrimitore, M. (2013). Price or quantity? The strategic choice of subsidized firms in a mixed duopoly. Economics Letters, 118, 337–341.

    Article  Google Scholar 

  • Singh, N., & Vives, X. (1984). Price and quantity competition in a differentiated duopoly. RAND Journal of Economics, 15, 546–554.

    Article  Google Scholar 

  • Sklivas, S. D. (1987). The strategic choice of management incentives. RAND Journal of Economics, 18, 452–458.

    Article  Google Scholar 

  • Van Witteloostuijn, A., & Jansen V. T., Lier, A. V. (2007). Bargaining over Managerial Contracts in Delegation Games: Managerial Power, Contract Disclosure, and Cartel Behavior. Managerial and Decision Economics, 28, 897–904.

  • Vickers, J. Delegation and the theory of the firm. Economic Journal 95, 138–147.

  • White, M. D. (2001). Managerial incentives and the decision to hire managers in markets with public and private firms. European Journal of Political Economy, 17, 877–896.

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. College of Economics, Nihon University, 1-3-2 Misaki-cho, Chiyoda-ku, Tokyo, 101-8360, Japan

    Yasuhiko Nakamura

Authors
  1. Yasuhiko Nakamura
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yasuhiko Nakamura.

Additional information

We are grateful to two anonymous referees for their helpful comments and suggestions. In addition, we are grateful for the financial supports of the Inamori Foundation, the Seimeikai Foundation, and KAKENHI (16K03665). All remaining errors are our own.

Electronic supplementary material

Below is the link to the electronic supplementary material.

Supplementary material 1 (PDF 59 kb)

Appendix

Appendix

1.1 The values of each term within the payoffs of firms 0 and 1 in each game

We here give the values of each term in the numerator within the payoffs of firms 0 and 1 in the four game.

1.1.1 The values of the terms of the numerator of the payoff of firms 0 in the p–p game

$${\left\{ \begin{array}{ll} PP^{0}_{1} = \left[ 8 - 2 \left( 5 + \gamma ^{2}\right) \delta ^{2} + \left( 1 + \gamma \right) ^{2} \delta ^{4} \right] , \\ PP^{0}_{2} = \left[ 28 + 32 \delta - 13 \delta ^{2} - 16 \delta ^{3} + 2 \delta ^{4} + 2 \delta ^{5} + 2 \gamma \left( 4 - 11 \delta ^{2} - 8 \delta ^{3} + 2 \delta ^{4} + 2 \delta ^{5}\right) - \gamma ^{2} \left( 4 - 3 \delta ^{2} - 2 \delta ^{4} - 2 \delta ^{5}\right) \right] , \\ PP^{0}_{3} = \left[ 12 - 21 \delta ^{2} + 9 \delta ^{4} - \delta ^{6} + 2 \gamma \left( 4 - 7 \delta ^{2} + 5 \delta ^{4} - \delta ^{6}\right) - \gamma ^{2} \left( 4 - 3 \delta ^{2} - \delta ^{4} + \delta ^{6}\right) \right] , \\ PP^{0}_{4} = \left[ 12 - 21 \delta ^{2} + 9 \delta ^{4} - \delta ^{6} + 2 \gamma \left( 4 - 7 \delta ^{2} + 5 \delta ^{4} - \delta ^{6}\right) - \gamma ^{2} \left( 4 - 3 \delta ^{2} - \delta ^{4} + \delta ^{6}\right) \right] , \\ PP^{0}_{5} = \left[ 16 + 4 \left( 1 - \gamma \right) ^{2} \delta - 8 \left( 3 + \gamma \right) \delta ^{2} - 3 \left( 1 - \gamma \right) ^{2} \delta ^{3} + \left( 9 + 10 \gamma + \gamma ^{2}\right) \delta ^{4} - \left( 1 + \gamma \right) ^{2} \delta ^{6} \right] , \\ PP^{0}_{6} = \left[ 12 - 21 \delta ^{2} + 9 \delta ^{4} - \delta ^{6} + 2 \gamma \left( 4 - 7 \delta ^{2} + 5 \delta ^{4} - \delta ^{6}\right) - \gamma ^{2} \left( 4 - 3 \delta ^{2} - \delta ^{4} + \delta ^{6}\right) \right] . \end{array}\right. }$$

1.1.2 The values of the terms of the numerator of the payoff of firms 0 in the p–q game

$${\left\{ \begin{array}{ll} PQ^{0}_{1} = \left[ 8 - 2 \left( 5 - 4 \gamma + \gamma ^{2}\right) \delta ^{2} + 3 \left( 1 - \gamma \right) ^{2} \delta ^{4} \right] , \\ PQ^{0}_{2} = \left[ 12 - 17 \delta ^{2} + 6 \delta ^{4} + \gamma \left( 8 - 6 \delta ^{2}\right) - \gamma ^{2} \left( 4 - 7 \delta ^{2} + 2 \delta ^{4}\right) \right] , \\ PQ^{0}_{3} = \big [12 - 17 \delta ^{2} + 6 \delta ^{4} + \gamma \left( 8 - 6 \delta ^{2} \right) - \gamma ^{2} \left( 4 - 7 \delta ^{2} + 2 \delta ^{4}\right) \big ], \\ PQ^{0}_{4} = \big [28 + 4 \delta - 41 \delta ^{2} - 7 \delta ^{3} + 15 \delta ^{4} + 3 \delta ^{5} + 2 \gamma \left( 4 - 4 \delta + \delta ^{2} + 7 \delta ^{3} - 3 \delta ^{4} - 3 \delta ^{5}\right) \\ \qquad - \gamma ^{2} \left( 4 - 4 \delta - 7 \delta ^{2} + 7 \delta ^{3} + \delta ^{4} - 3 \delta ^{5}\right) \big ], \\ PQ^{0}_{5} = \left[ 16 + 4 \left( 1 - \gamma \right) ^{2} \delta - 8 \left( 3 - \gamma \right) \delta ^{2} - 7 \left( 1 - \gamma \right) ^{2} \delta ^{3} + \left( 3 - \gamma \right) ^{2} \delta ^{4} + 3 \left( 1 - \gamma \right) ^{2} \delta ^{5} \right], \\ PQ^{0}_{6} = \left[ 12 - 17 \delta ^{2} + 6 \delta ^{4} + \gamma \left( 8 - 6 \delta ^{2}\right) - \gamma ^{2} \left( 4 - 7 \delta ^{2} + 2 \delta ^{4}\right) \right] . \end{array}\right. }$$

1.1.3 The values of the terms of the numerator of the payoff of firms 0 in the q–q game

$${\left\{ \begin{array}{ll} QP^{0}_{1} = \left[ 4 - \left( 3 + \gamma ^{2}\right) \delta ^{2} \right] , \\ QP^{0}_{2} = \left[ 12 - 9 \delta ^{2} + \gamma \left( 8 - 6 \delta ^{2}\right) - \gamma ^{2} \left( 4 + \delta ^{2}\right) \right] , \\ QP^{0}_{3} = \left[ 12 - 9 \delta ^{2} + \gamma \left( 8 - 6 \delta ^{2}\right) - \gamma ^{2} \left( 4 + \delta ^{2}\right) \right] , \\ QP^{0}_{4} = \left[ 28 - 24 \delta - 21 \delta ^{2} + 18 \delta ^{3} + 2 \gamma \left( 4 - 8 \delta - 3 \delta ^{2} + 6 \delta ^{3}\right) - \gamma ^{2} \left( 4 - 8 \delta + 5 \delta ^{2} - 2 \delta ^{3}\right) \right] , \\ QP^{0}_{5} = \left[ 16 - 4 \left( 3 + 2 \gamma - \gamma ^{2}\right) \delta - 4 \left( 3 + \gamma ^{2}\right) \delta ^{2} + \left( 3 + \gamma \right) ^{2} \delta ^{3} \right] , \\ QP^{0}_{6} = \left[ 12 - 9 \delta ^{2} + \gamma \left( 8 - 6 \delta ^{2}\right) - \gamma ^{2} \left( 4 + \delta ^{2}\right) \right] . \end{array}\right. }$$

1.1.4 The values of the terms of the numerator of the payoff of firms 0 in the q–q game

$${\left\{ \begin{array}{ll} QQ^{0}_{1} = \left[ 4 - \left( 3 - 4 \gamma + \gamma ^{2}\right) \delta ^{2} + \left( 1 - \gamma \right) ^{2} \delta ^{4} \right] , \\ QQ^{0}_{2} = \left[ 12 - 5 \delta ^{2} + \delta ^{4} + \gamma \left( 8 + 2 \delta ^{2} - 2 \delta ^{4}\right) - \gamma ^{2} \left( 4 - 3 \delta ^{2} - \delta ^{4}\right) \right] , \\ QQ^{0}_{3} = \left[ 12 - 5 \delta ^{2} + \delta ^{4} + \gamma \left( 8 + 2 \delta ^{2} - 2 \delta ^{4}\right) - \gamma ^{2} \left( 4 - 3 \delta ^{2} - \delta ^{4}\right) \right] , \\ QQ^{0}_{4} = \left[ 28 + 4 \delta - 13 \delta ^{2} - 3 \delta ^{3} + 2 \delta ^{4} + \gamma \left( 8 - 8 \delta + 10 \delta ^{2} + 6 \delta ^{3} - 4 \delta ^{4}\right) - \gamma ^{2} \left( 4 - 4 \delta - 3 \delta ^{2} + 3 \delta ^{3} - 2 \delta ^{4}\right) \right] , \\ QQ^{0}_{5} = \left[ 16 + 4 \left( 1 - \gamma \right) ^{2} \delta - 8 \left( 1 - \gamma \right) \delta ^{2} - 3 \left( 1 - \gamma \right) ^{2} \delta ^{3} + \left( 1 - \gamma \right) ^{2} \delta ^{4} \right] , \\ QQ^{0}_{6} = \left[ 12 - 5 \delta ^{2} + \delta ^{4} + \gamma \left( 8 + 2 \delta ^{2} - 2 \delta ^{4}\right) - \gamma ^{2} \left( 4 - 3 \delta ^{2} - \delta ^{4}\right) \right] . \end{array}\right. }$$

1.2 Deriving process of market outcomes in the four games

Here, we provide the process of deriving the market outcomes obtained in the four market structures.

1.2.1 p–p game

In the third stage, the reaction functions of firms 0 and 1 are given in the p–p game:

$${\left\{ \begin{array}{ll} p_{0} \left( p_{1} \right) = c - c \delta + p_{1} \delta - \theta _{0}, \\ p_{1} \left( p_{0} \right) = \left( c + \alpha _{1} + p_{0} \delta - \alpha _{0} \delta - \theta 1\right) / 2, \end{array}\right. }$$

yielding

$$\begin{aligned} p^{pp}_{0} \left( \theta _{0}, \theta _{1} \right)&= \left[ \alpha _{1} \delta - \alpha _{0} \delta ^{2} - 2 \theta _{0} + \delta \theta _{1} + c \left( 2 - \delta \right) \right] / \left( 2 - \delta ^{2}\right) , \\ p^{pp}_{1} \left( \theta _{0}, \theta _{1} \right)&= \left[ \alpha _{1} - \alpha _{0} \delta - \delta \theta _{0} - \theta _{1} + c \left( 1 + \delta - \delta ^{2}\right) \right] / \left( 2 -\delta ^{2}\right) . \end{aligned}$$

Moreover, we have

$$\begin{aligned}&\pi ^{pp}_{1} \left( \theta _{0}, \theta _{1} \right) = \left[ \alpha _{1} - c (1 - \delta ) - \alpha _{0} \delta - \delta \theta _{0} - \theta _{1} \right] (\alpha _{1} - c + c \delta - \alpha _{0} \delta - \delta \theta _{0} + \theta _{1} - \delta ^{2} \theta _{1})\\&/ \beta (2 - \delta ^{2})^{2} (1 - \delta ^{2}), \\&V^{pp}_{1} \left( \theta _{0}, \theta _{1} \right) = (c - \alpha _{1} - c \delta + \alpha _{0} \delta + \delta \theta _{0} - \theta _{1} + \delta ^{2} \theta _{1})^{2} / \beta (2 - \delta ^{2})^{2} (1 - \delta ^{2}). \end{aligned}$$

In the second stage, the owner maximizes social welfare, which is equal to his objective function, within firm 0, and the bargaining problem between the manager and owner within firm 1 are given as follows:

$${\left\{ \begin{array}{ll} \partial W^{pp} \left( \theta _{0}, \theta _{1} \right) / \partial \theta = 0 \iff \theta ^{pp}_{0} \left( \theta _{1} \right) = \delta \left( 1 - \delta ^{2}\right) \left[ \alpha _{1} - c \left( 1 - \delta \right) - \alpha _{0} \delta - \theta _{1} \right] / \left( 4 - 3 \delta ^{2}\right) , \\ \partial B^{pp}_{1} \left( \theta _{0}, \theta _{1} \right) / \partial \theta _{1} = 0 \iff \theta ^{pp}_{1} \left( \theta _{0} \right) = - \left[ \delta ^{2} - \gamma \left( 2 - \delta ^{2}\right) \right] \left[ \alpha _{1} - c \left( 1 - \delta \right) - \delta \left( \alpha _{0} + \theta _{0}\right) \right] / 2 \left( 1 - \delta ^{2}\right) , \end{array}\right. }$$

Note that \(B^{pp}_{1} \left( \theta _{0}, \theta _{1} \right) = \left[ V^{pp}_{1} \left( \theta _{0}, \theta _{1} \right) \right] ^{\gamma } \cdot \left[ \pi ^{pp}_{i} \left( \theta _{0}, \theta _{1} \right) \right] ^{1 - \gamma }\).Footnote 28 The delegation parameters of firms 0 and 1 in the p-p game are given as follows:

$$\begin{aligned}&\theta ^{pp}_{0} = \frac{\left( 1 - \gamma \right) \delta \left[ \alpha _{1} - c \left( 1 - \delta \right) - \alpha _{0} \delta \right] }{4 - \left( 1 + \gamma \right) \delta ^{2}}, \quad \\&\theta ^{pp}_{1} = - \frac{\left[ \alpha _{1} - c \left( 1 - \delta \right) - \alpha _{0} \delta \right] \left( 2 - \delta ^{2} \right) \left[ \delta ^{2} - \gamma \left( 2 - \delta ^{2} \right) \right] }{\left( 1 - \delta ^{2} \right) \left[ 4 - \left( 1 + \gamma \right) \delta ^{2} \right] }. \end{aligned}$$

Moreover, we obtain the following equilibrium market outcomes in the p–p game:

$$\begin{aligned} p^{pp}_{0}&= \frac{\left( 1 - \gamma \right) \delta \left( \alpha _{1} - \alpha _{0} \delta \right) + c \left[ 4 - \left( 1 - \gamma \right) \delta - 2 \left( 2 + \gamma \right) \delta ^{2} + \left( 1 + \gamma \right) \delta ^{4} \right] }{\left( 1 - \delta ^{2} \right) \left[ 4 - \left( 1 + \gamma \right) \delta ^{2} \right] }, \\ p^{pp}_{1}&= \frac{\left( 1 - \gamma \right) \left( \alpha _{1} - \alpha _{0} \delta \right) \left( 2 - \delta ^{2}\right) + c \left( 1 - \delta \right) \left[ 2 + 4 \delta - \delta ^{3} + \gamma \left( 2 - 2 \delta ^{2} - \delta ^{3}\right) \right] }{\left( 1 - \delta ^{2}\right) \left[ 4 - \left( 1 + \gamma \right) \delta ^{2} \right] }, \\ \pi ^{pp}_{0}&= \frac{ \begin{array}{c} \left( 1 - \gamma \right) \delta \left[ \alpha _{1} - c \left( 1 - \delta \right) - \alpha _{0} \delta \right] \left\{ 2 \alpha _{0} \left( 2 - \delta ^{2} \right) - \alpha _{1} \delta \left( 3 + \gamma - \delta ^{2} - \gamma \delta ^{2} \right) - c \left( 1 - \delta \right) \left[ 4 + \left( 1 - \gamma \right) \delta - \left( 1 + \gamma \right) \delta ^{2} \right] \right\} \end{array} }{\beta \left( 1 - \delta ^{2} \right) ^{2} \left[ 4 - \left( 1 + \gamma \right) \delta ^{2} \right] ^{2}}, \\ CS^{pp}&= \frac{ \begin{array}{c} \alpha _{0}^{2} \left( 2 - \delta ^{2} \right) ^{2} PP^{CS}_{1} - 2 \alpha _{0} \alpha _{1} \delta \left( 2 - \delta ^{2} \right) PP^{CS}_{2} + c^{2} \left( 1 - \delta \right) ^{2} PP^{CS}_{3} + \alpha _{1}^{2} PP^{CS}_{4} - 2 c \left( 1 - \delta \right) \left[ \alpha _{0} \left( 2 - \delta ^{2} \right) PP^{CS}_{5} + \alpha _{1} PP_{6} \right] \end{array} }{2 \beta \left( 1 - \delta ^{2} \right) ^{2} \left[ 4 - \left( 1 + \gamma \right) \delta ^{2} \right] ^{2}}, \\&PS^{pp} \\&= \frac{\left( 1 - \gamma \right) \left[ \alpha _{1} - c \left( 1 - \delta \right) - \alpha _{0} \delta \right] \left\{ \alpha _{0} \delta \left( 2 - \delta ^{2}\right) \left[ \delta ^{2} - \gamma \left( 2 - \delta ^{2}\right) \right] - c \left( 1 - \delta \right) PP^{PS}_{1} + \alpha _{1} PP^{PS}_{2} \right\} }{\beta \left( 1 - \delta ^{2}\right) ^{2} \left[ 4 - \left( 1 + \gamma \right) \delta ^{2} \right] ^{2}}, \end{aligned}$$

where

$$\begin{aligned} {\left\{ \begin{array}{ll} PP^{CS}_{1} = \left[ 4 + \left( 3 + 2 \gamma - \gamma ^{2} \right) \delta ^{2} \right] , \\ PP^{CS}_{2} = \left[ \left( 2 - \delta ^{2} \right) ^{2} + 2 \gamma \left( 1 - 3 \delta ^{2} + \delta ^{4} \right) + \gamma ^{2} \left( 2 - 2 \delta ^{2} + \delta ^{4 }\right) \right] , \\ PP^{CS}_{3} =\left[ 20 + 24 \delta - 7 \delta ^{2} - 14 \delta ^{3} + 2 \delta ^{5} + 2 \gamma \left( 4 + 4 \delta - 9 \delta ^{2} - 8 \delta ^{3} + 2 \delta ^{4} + 2 \delta ^{5} \right) + \gamma ^{2} \left( 4 - 7 \delta ^{2} - 2 \delta ^{3} + 4 \delta ^{4} + 2 \delta ^{5} \right) \right] , \\ PP^{CS}_{4} = \left[ 4 - 7 \delta ^{2} + 5 \delta ^{4} - \delta ^{6} + 2 \gamma \left( 4 - 9 \delta ^{2} + 5 \delta ^{4} - \delta ^{6} \right) + \gamma ^{2} \left( 4 - 7 \delta ^{2} + 5 \delta ^{4} - \delta ^{6} \right) \right] , \\ PP^{CS}_{5} = \left[ 8 + 2 \left( 2 - \gamma - \gamma ^{2}\right) \delta - 6 \left( 1 + \gamma \right) \delta ^{2} - 2 \left( 1 - \gamma ^{2}\right) \delta ^{3} + \left( 1 + \gamma \right) ^{2} \delta ^{4} \right] , \\ PP^{CS}_{6} = \left[ 4 - 4 \delta - 11 \delta ^{2} + \delta ^{3} + 6 \delta ^{4} - \delta ^{6} - 2 \gamma \left( 4 + 2 \delta - 7 \delta ^{2} + 5 \delta ^{4} - \delta ^{6} \right) - \gamma ^{2} \left( 4 - 7 \delta ^{2} - \delta ^{3} + 4 \delta ^{4} - \delta ^{6} \right) \right] , \\ PP^{PS}_{1} = \left[ 4 + 4 \delta - 3 \delta ^{2} - \delta ^{3} + \delta ^{4} + \gamma \left( 4 - 5 \delta ^{2} - \delta ^{3} + \delta ^{4}\right) \right] , \\ PP^{PS}_{2} = \left[ 4 - 7 \delta ^{2} + 2 \delta ^{4} + \gamma \left( 4 - 5 \delta ^{2} + 2 \delta ^{4}\right) \right] . \end{array}\right. } \end{aligned}$$

1.2.2 p–q game

In the third stage, the reaction functions of firms 0 and 1 are given in the p–q game:

$${\left\{ \begin{array}{ll} p^{pq}_{0} \left( q_{1} \right) = c - \theta _{0}, \\ q^{pq}_{1} \left( p_{0} \right) = \left( c - \alpha _{1} - p_{0} \delta + \alpha _{0} \delta - \theta _{1} \right) / 2 \beta \left( 1 - \delta ^{2}\right) , \end{array}\right. }$$

yielding

$$\begin{aligned}&p^{pq}_{0} \left( \theta _{0} \right) = c - \theta _{0}, \\&q^{pq}_{1} \left( \theta _{0}, \theta _{1} \right) = \left( \alpha _{1} + c \delta - \alpha _{0} \delta - \delta \theta _{0} + \theta _{1} - c \right) / \left( 2 \beta - 2 \beta \delta ^{2} \right) . \end{aligned}$$

Moreover, we have

$$\begin{aligned}&\pi ^{pq}_{1} \left( \theta _{0}, \theta _{1} \right) = \left[ \alpha _{1} - c (1 - \delta ) - \alpha _{0} \delta - \delta \theta _{0} - \theta _{1} \right] \\&\left[ \alpha _{1} - c (1 - \delta ) - \alpha _{0} \delta - \delta \theta _{0} + \theta _{1} \right] \\&/ 4 \beta (1 - \delta ^{2}), \\&V^{pq}_{1} \left( \theta _{0}, \theta _{1} \right) = \left[ \alpha _{1} - c (1 - \delta ) - \alpha _{0} \delta - \delta \theta _{0} + \theta _{1} \right] ^{2} / 4 \beta (1 - \delta ^{2}). \end{aligned}$$

In the second stage, the owner maximizes social welfare, which is equal to his objective function, within firm 0, and the bargaining problem between the manager and owner within firm 1 are given as follows:

$${\left\{ \begin{array}{ll} \partial W^{pq} \left( \theta _{0}, \theta _{1} \right) / \partial \theta _{0} = 0 \iff \theta ^{pq}_{0} \left( \theta _{1} \right) = \delta \left[ \alpha _{0} \delta + \theta _{1} - \alpha _{1} + c \left( 1 - \delta \right) \right] / \left( 4 - 3 \delta ^{2}\right) , \\ \partial B^{pq}_{1} \left( \theta _{0}, \theta _{1} \right) / \partial \theta _{1} = 0 \iff \theta ^{pq}_{1} \left( \theta _{0} \right) = \gamma \left[ \alpha 1 - c \left( 1 - \delta \right) - \delta \left( \alpha _{0} + \theta _{0}\right) \right] . \end{array}\right. }$$

Note that \(B^{pq}_{1} \left( \theta _{0}, \theta _{1} \right) = \left[ V^{pq}_{1} \left( \theta _{0}, \theta _{1} \right) \right] ^{\gamma } \cdot \left[ \pi ^{pq}_{1} \left( \theta _{0}, \theta _{1} \right) \right] ^{1 - \gamma }\).Footnote 29 The delegation parameters of firms 0 and 1 in the p-q game are given as follows:

$$\begin{aligned} \theta ^{pq}_{0} = - \frac{\left( 1 - \gamma \right) \delta \left[ \alpha _{1} - c \left( 1 - \delta \right) - \alpha _{0} \delta \right] }{4 - \left( 3 - \gamma \right) \delta ^{2}}, \quad \theta ^{pq}_{1} = \frac{2 \gamma \left[ \alpha _{1} - c \left( 1 - \delta \right) - \alpha _{0} \delta \right] \left( 2 - \delta ^{2}\right) }{4 - \left( 3 - \gamma \right) \delta ^{2}}. \end{aligned}$$

Moreover, we obtain the following equilibrium market outcomes in the p–q game:

$$\begin{aligned} p^{pq}_{0}&= c + \frac{\left( 1 - \gamma \right) \delta \left[ \alpha _{1} - c \left( 1 - \delta \right) - \alpha _{0} \delta \right] }{4 - \left( 3 - \gamma \right) \delta ^{2}}, \quad q^{pq}_{1} = \frac{\left( 1 + \gamma \right) \left[ \alpha _{1} - c \left( 1 - \delta \right) - \alpha _{0} \delta \right] \left( 2 - \delta ^{2}\right) }{\beta \left( 1 - \delta ^{2}\right) \left[ 4 - \left( 3 - \gamma \right) \delta ^{2} \right] }, \\ \pi ^{pq}_{0}&= \frac{ \begin{array}{c} \left( 1 - \gamma \right) \delta \left[ \alpha _{1} - c \left( 1 - \delta \right) - \alpha _{0} \delta \right] \left\{ \alpha _{1} \delta \left( 3 + \gamma - 2 \delta ^{2}\right) - \alpha _{0} \left( 2 - \delta ^{2}\right) \left[ 2 - \left( 1 - \gamma \right) \delta ^{2} \right] - c \left( 1 - \delta \right) \left[ 4 + \delta - \gamma \delta - \left( 3 - \gamma \right) \delta ^{2} - \left( 1 - \gamma \right) \delta ^{3} \right] \right\} \end{array} }{\beta \left( 1 - \delta ^{2}\right) \left[ 4 - \left( 3 - \gamma \right) \delta ^{2} \right] ^{2}}, \\ CS^{pq}&= \frac{ \begin{array}{c} \alpha _{0}^{2} \left( 2 - \delta ^{2}\right) ^{2} PQ^{CS}_{1} - 2 \alpha _{0} \alpha _{1} \delta \left( 2 - \delta ^{2}\right) PQ^{CS}_{2} + \alpha _{1}^{2} PQ^{CS}_{3} + c^{2} \left( 1 - \delta \right) PQ^{CS}_{4} + 2 c \left( 1 - \delta \right) \left[ \alpha _{0} \left( 2 - \delta ^{2}\right) PQ^{CS}_{5} + \alpha _{1} PQ^{CS}_{6} \right] \end{array} }{2 \beta \left( 1 - \delta \right) \left( 1 + \delta \right) \left[ 4 - \left( 3 - \gamma \right) \delta ^{2} \right] ^{2}}, \\ PS^{pq}&= \frac{\left( 1 - \gamma \right) \left[ \alpha _{1} - c \left( 1 - \delta \right) - \alpha _{0} \delta \right] \left\{ \begin{array}{c} \alpha _{1} \left[ 4 - 3 \delta ^{2} + \gamma \left( 4 - \delta ^{2}\right) \right] - 2 \alpha _{0} \gamma \delta \left( 2 - \delta ^{2}\right) - c \left[ 4 - 3 \delta ^{2} + \gamma \left( 4 - 4 \delta - \delta ^{2} + 2 \delta ^{3}\right) \right] \end{array} \right\} }{\beta \left[ 4 - \left( 3 - \gamma \right) \delta ^{2} \right] ^{2}}, \end{aligned}$$

where

$${\left\{ \begin{array}{ll} PQ^{CS}_{1} = \left[ 4 - \left( 3 - 2 \gamma - \gamma ^{2}\right) \delta ^{2} \right] , \\ PQ^{CS}_{2} =\left[ 4 + 2 \gamma - 3 \delta ^{2} + \gamma ^{2} \left( 2 - \delta ^{2}\right) \right] , \\ PQ^{CS}_{3} =\left[ 4 - 3 \delta ^{2} + 2 \gamma \left( 4 - 5 \delta ^{2} + 2 \delta ^{4}\right) + \gamma ^{2} \left( 4 - 3 \delta ^{2}\right) \right] , \\ PQ^{CS}_{4} =\big [20 + 4 \delta - 27 \delta ^{2} - 7 \delta ^{3} + 9 \delta ^{4} + 3 \delta ^{5} + 2 \gamma \left( 4 - \delta ^{2} + \delta ^{3} - \delta ^{4} - \delta ^{5}\right) \\ \qquad + \gamma ^{2} \left( 4 - 4 \delta - 3 \delta ^{2} + 5 \delta ^{3} + \delta ^{4} - \delta ^{5}\right) \big ], \\ PQ^{CS}_{5} =\left[ 8 + 2 \left( 2 - \gamma - \gamma ^{2}\right) \delta - 2 \left( 3 - \gamma \right) \delta ^{2} - \left( 3 - 2 \gamma - \gamma ^{2}\right) \delta ^{3} \right] , \\ PQ^{CS}_{6} =\left[ 4 - 4 \delta - 7 \delta ^{2} + 3 \delta ^{3} + 3 \delta ^{4} + \gamma \left( 8 + 4 \delta - 6 \delta ^{2} - 4 \delta ^{3}\right) + \gamma ^{2} \left( 4 - 3 \delta ^{2} + \delta ^{3} + \delta ^{4}\right) \right] . \end{array}\right. }$$

1.2.3 q–p game

In the third stage, the reaction functions of firms 0 and 1 are given in the q–p game:

$${\left\{ \begin{array}{ll} q^{qp}_{0} \left( p_{1} \right) = \left[ \alpha _{0} - c \left( 1 - \delta \right) - \alpha _{1} \delta + \theta _{0} \right] / \beta \left( 1 - \delta ^{2}\right) , \\ p^{qp}_{1} \left( q_{0} \right) = \left( c + \alpha _{1} - q0 \beta \delta - \theta _{1} \right) / 2, \end{array}\right. }$$

yielding

$$\begin{aligned} q^{qp}_{0} \left( \theta _{0}, \theta _{1} \right)&= \left[ \alpha _{0} - c \left( 1 - \delta \right) - \alpha _{1} \delta + \theta _{0} \right] / \beta \left( 1 - \delta ^{2}\right) , \\ p^{qp}_{1} \left( \theta _{0}, \theta _{1} \right)&= \left( c + \alpha _{1} + c \delta - \alpha _{0} \delta - 2 c \delta ^{2} - \delta \theta _{0} - \theta _{1} + \delta ^{2} \theta _{1}\right) / \left( 2 - 2 \delta ^{2}\right) . \end{aligned}$$

Moreover, we have

$$\begin{aligned}&\pi ^{qp}_{1} \left( \theta _{0}, \theta _{1} \right) = \left[ \alpha _{1} - c (1 - \delta ) - \alpha 0 \delta - \delta \theta _{0} + \theta _{1} - \delta ^{2} \theta _{1} \right] \\&\left[ \alpha _{1} - c (1 - \delta ) - \alpha _{0} \delta - \delta \theta _{0} - \theta _{1} + \delta ^{2} \theta _{1} \right] / 4 \beta (1 - \delta ^{2})^{2}, \\&V^{qp}_{1} \left( \theta _{0}, \theta _{1} \right) = (c - \alpha _{1} - c \delta + \alpha _{0} \delta + \delta \theta _{0} - \theta _{1} + \delta ^{2} \theta _{1})^{2} / 4 \beta (1 - \delta ^{2})^{2}. \end{aligned}$$

In the second stage, the owner maximizes social welfare, which is equal to his objective function, within firm 0, and the bargaining problem between the manager and owner within firm 1 are given as follows:

$${\left\{ \begin{array}{ll} \partial W^{qp} \left( \theta _{0}, \theta _{1} \right) / \partial \theta _{0} = 0 \iff \theta ^{qp}_{0} \left( \theta _{1} \right) = \delta \left[ \alpha _{0} \delta + \theta _{1} - \delta ^{2} \theta _{1} - \alpha _{1} + c \left( 1 - \delta \right) \right] / \left( 4 - 3 \delta ^{2}\right) , \\ \partial B^{qp}_{1} \left( \theta _{0}, \theta _{1} \right) / \partial \theta _{1} = 0 \iff \theta ^{qp}_{1} \left( \theta _{0} \right) = \left[ \delta ^{2} + \gamma \left( 2 - \delta ^{2}\right) \right] \left[ \alpha _{1} - c \left( 1 - \delta \right) - \delta \left( \alpha _{0} + \theta 0\right) \right] / 2 \left( 1 - \delta ^{2}\right) . \end{array}\right. }$$

Note that \(B^{qp}_{1} \left( \theta _{0}, \theta _{1} \right) = \left[ V^{qp}_{1} \left( \theta _{0}, \theta _{1} \right) \right] ^{\gamma } \cdot \left[ \pi ^{qp}_{1} \left( \theta _{0}, \theta _{1} \right) \right] ^{1 - \gamma }\).Footnote 30 The delegation parameters of firms 0 and 1 in the q–p game are given as follows:

$$\begin{aligned} \theta ^{qp}_{0} = \frac{\left( 1 - \gamma \right) \delta \left[ \alpha _{1} - c \left( 1 - \delta \right) - \alpha _{0} \delta \right] }{4 - \left( 3 + \gamma \right) \delta ^{2}}, \quad \theta ^{qp}_{1} = - \frac{4 \gamma \left( c - \alpha _{1} - c \delta + \alpha _{0} \delta \right) }{4 - \left( 3 + \gamma \right) \delta ^{2}}. \end{aligned}$$

Moreover, we obtain the following equilibrium market outcomes in the q–p game:

$$\begin{aligned} q^{qp}_{0}&= \frac{4 \alpha _{0} - \alpha _{1} \left( 3 + \gamma \right) \delta - c \left[ 4 - \left( 3 + \gamma \right) \delta \right] }{\beta \left[ 4 - \left( 3 + \gamma \right) \delta ^{2} \right] }, \\ p^{qp}_{1}&= \frac{2 \left( 1 - \gamma \right) \left( \alpha _{1} - \alpha _{0} \delta \right) + c \left[ 2 + 2 \delta - 3 \delta ^{2} + \gamma \left( 2 - 2 \delta - \delta ^{2}\right) \right] }{4 - \left( 3 + \gamma \right) \delta ^{2}}, \\ \pi ^{qp}_{0}&= \frac{\left( 1 - \gamma \right) \delta \left[ \alpha _{1} - c \left( 1 - \delta \right) - \alpha _{0} \delta \right] \left\{ 4 \alpha _{0} - \alpha _{1} \left( 3 + \gamma \right) \delta - c \left[ 4 - \left( 3 + \gamma \right) \delta \right] \right\} }{\beta \left[ 4 - \left( 3 + \gamma \right) \delta ^{2} \right] ^{2}}, \\ CS^{qp}&= \frac{4 \alpha _{0}^{2} QP^{CS}_{1} + \alpha _{1}^{2} QP^{CS}_{2} - 4 \alpha _{0} \alpha _{1} \delta QP_{3} + c^{2} QP_{4} - 2 c \left( 2 \alpha _{0} QP_{5} + \alpha _{1} QP^{CS}_{6} \right) }{2 \beta \left[ 4 - \left( 3 + \gamma \right) \delta ^{2} \right] ^{2}}, \\ PS^{qp}&= \frac{\left( 1 - \gamma \right) \left[ \alpha _{0} \delta - \alpha _{1} + c \left( 1 - \delta \right) \right] \left\{ 4 \alpha _{0} \gamma \delta - \alpha _{1} \left[ 4 - 3 \delta ^{2} + \gamma \left( 4 - \delta ^{2}\right) \right] + c \left[ 4 - 3 \delta ^{2} + \gamma \left( 4 - 4 \delta - \delta ^{2}\right) \right] \right\} }{\beta \left[ 4 - \left( 3 + \gamma \right) \delta ^{2} \right] ^{2}}, \end{aligned}$$

where

$${\left\{ \begin{array}{ll} QP^{CS}_{1} = \left[ 4 - \left( 3 + 2 \gamma - \gamma ^{2}\right) \delta ^{2} \right] , \\ QP^{CS}_{2} = \left[ 4 - 3 \delta ^{2} + \gamma \left( 8 - 10 \delta ^{2}\right) + \gamma ^{2} \left( 4 - 3 \delta ^{2}\right) \right] , \\ QP^{CS}_{3} = \left[ 4 - 3 \delta ^{2} + \gamma ^{2} \left( 2 - \delta ^{2}\right) + \gamma \left( 2 - 4 \delta ^{2}\right) \right] , \\ QP^{CS}_{4} = \left[ 20 - 16 \delta - 15 \delta ^{2} + 12 \delta ^{3} + 2 \gamma \left( 4 - 4 \delta - 9 \delta ^{2} + 8 \delta ^{3}\right) + \gamma ^{2} \left( 4 - 8 \delta + \delta ^{2} + 4 \delta ^{3}\right) \right] , \\ QP^{CS}_{5} = \left[ 8 - 2 \left( 2 + \gamma + \gamma ^{2}\right) \delta + 2 \left( -3 - 2 \gamma + \gamma ^{2}\right) \delta ^{2} + \left( 3 + 4 \gamma + \gamma ^{2}\right) \delta ^{3} \right] , \\ QP^{CS}_{6} = \left[ 4 - 8 \delta - 3 \delta ^{2} + 6 \delta ^{3} + \gamma ^{2} \left( 4 - 4 \delta - 3 \delta ^{2} + 2 \delta ^{3}\right) + 2 \gamma \left( 4 - 2 \delta - 5 \delta ^{2} + 4 \delta ^{3}\right) \right] . \end{array}\right. }$$

1.2.4 q–q game

In the third stage, the reaction functions of firms 0 and 1 are given in the q–q game:

$$\begin{aligned} {\left\{ \begin{array}{ll} q^{qq}_{0} \left( q_{1} \right) = \left( \alpha _{0} - c - q_{1} \beta \delta + \theta _{0} \right) / \beta , \\ q^{qq}_{1} \left( q_{0} \right) = \left( \alpha _{1} - c - q_{0} \beta \delta + \theta _{1} \right) / 2 \beta , \end{array}\right. } \end{aligned}$$

yielding

$$\begin{aligned} q^{qq}_{0} \left( \theta _{0}, \theta _{1} \right)&= \left[ 2 \alpha _{0} - c \left( 2 - \delta \right) - \alpha _{1} \delta + 2 \theta _{0} - \delta \theta _{1} \right] / \beta \left( 2 - \delta ^{2}\right) , \\ q^{qq}_{1} \left( \theta _{0}, \theta _{1} \right)&= \left( \alpha _{1} - c + c \delta - \alpha _{0} \delta - \delta \theta _{0} + \theta _{1} \right) / \beta \left( 2 - \delta ^{2}\right) . \end{aligned}$$

Moreover, we have

$$\begin{aligned}&\pi ^{qq}_{1} \left( \theta _{0}, \theta _{1} \right) = \left[ \alpha _{1} - c (1 - \delta ) - \alpha _{0} \delta - \delta \theta _{0} + \theta _{1} \right] \\&\left[ \alpha _{1} - c (1 - \delta ) - \alpha _{0} \delta - \delta \theta _{0} - \theta _{1} + \delta ^{2} \theta _{1} \right] / \beta (2 - \delta ^{2})^{2}, \\&V^{qq}_{1} \left( \theta _{0}, \theta _{1} \right) = \left[ \alpha _{1} - c (1 - \delta ) - \alpha _{0} \delta - \delta \theta _{0} + \theta _{1} \right] ^{2} /\beta (2 - \delta ^{2})^{2}. \end{aligned}$$

In the second stage, the owner maximizes social welfare, which is equal to his objective function, within firm 0, while the bargaining problem between the manager and owner within firm 1 are given as follows:

$${\left\{ \begin{array}{ll} \partial W^{qq} \left( \theta _{0}, \theta _{1} \right) / \partial \theta _{0} = 0 \iff \theta ^{qq}_{0} \left( \theta _{1} \right) = - \delta \left[ \alpha _{1} - c (1 - \delta ) - \alpha _{0} \delta - \theta _{1} + \delta ^{2} \theta _{1} \right] / (4 - 3 \delta ^{2}), \\ \partial B^{qq}_{1} \left( \theta _{0}, \theta _{1} \right) / \partial \theta _{1} = 0 \iff \theta ^{qq}_{1} \left( \theta _{0} \right) = \left[ \delta ^{2} + \gamma (2 - \delta ^{2}) \right] \left[ \alpha _{1} - c (1 - \delta ) - \delta (\alpha _{0} + \theta _{0}) \right] / 2 (1 - \delta ^{2}), \end{array}\right. }$$

Note that \(B^{qq}_{1} \left( \theta _{0}, \theta _{1} \right) = \left[ V^{qq}_{1} \left( \theta _{0}, \theta _{1} \right) \right] ^{\gamma } \cdot \left[ \pi ^{qq}_{1} \left( \theta _{0}, \theta _{1} \right) \right] ^{1 - \gamma }\).Footnote 31 The delegation parameters of firms 0 and 1 in the q–q game are given as follows:

$$\begin{aligned} \theta ^{qq}_{0} = - \frac{\left( 1 - \gamma \right) \delta \left[ \alpha _{1} - c \left( 1 - \delta \right) - \alpha _{0} \delta \right] }{4 - \left( 1 - \gamma \right) \delta ^{2}}, \quad \theta ^{qq}_{1} = \frac{2 \left[ \alpha _{1} - c \left( 1 - \delta \right) - \alpha _{0} \delta \right] \left[ \delta ^{2} + \gamma \left( 2 - \delta ^{2}\right) \right] }{\left( 1 - \delta ^{2}\right) \left[ 4 - \left( 1 - \gamma \right) \delta ^{2} \right] }. \end{aligned}$$

Moreover, we obtain the following equilibrium market outcomes in the q–q game:

$$\begin{aligned} q^{qq}_{0}&= \frac{2 \alpha _{0} \left( 2 - \left( 1 - \gamma \right) \delta ^{2}\right) - c \left( 1 - \delta \right) \left[ 4 + \delta - \gamma \delta - \left( 1 - \gamma \right) \delta ^{2} \right] - \alpha _{1} \delta \left( 3 + \gamma - \delta ^{2} + \gamma \delta ^{2}\right) }{\beta \left( 1 - \delta ^{2}\right) \left[ 4 - \left( 1 - \gamma \right) \delta ^{2} \right] }, \\ q^{qq}_{1}&= \frac{2 \left( 1 + \gamma \right) \left[ \alpha _{1} - c \left( 1 - \delta \right) - \alpha _{0} \delta \right] }{\beta \left( 1 - \delta ^{2}\right) \left[ 4 - \left( 1 - \gamma \right) \delta ^{2} \right] }, \\ \pi ^{qq}_{0}&= \frac{ \begin{array}{c} \left( 1 - \gamma \right) \delta \left[ \alpha _{1} - c \left( 1 - \delta \right) - \alpha _{0} \delta \right] \left\{ 2 \alpha _{0} \left[ 2 - \left( 1 - \gamma \right) \delta ^{2} \right] - c \left( 1 - \delta \right) \left[ 4 + \delta - \gamma \delta - \left( 1 - \gamma \right) \delta ^{2}\right] - \alpha _{1} \delta \left( 3 + \gamma - \delta ^{2} + \gamma \delta ^{2}\right) \right\} \end{array} }{\beta \left( 1 - \delta ^{2}\right) \left[ 4 - \left( 1 - \gamma \right) \delta ^{2} \right] ^{2}}, \\ CS^{qq}&= \frac{4 \alpha _{0}^{2} QQ^{CS}_{1} - 8 \alpha _{0} \alpha _{1} QQ^{CS}_{2} + \alpha _{1}^{2} QQ^{CS}_{3} + c^{2} \left( 1 - \delta \right) QQ^{CS}_{4} - 2 c \left( 1 - \delta \right) \left( 4 \alpha _{0} QQ^{CS}_{5} + \alpha _{1} QQ^{CS}_{6} \right) }{2 \beta \left( 1 - \delta ^{2}\right) \left[ 4 - \left( 1 - \gamma \right) \delta ^{2} \right] ^{2}}, \\ PS^{qq}&= \frac{\left( 1 - \gamma \right) \left[ \alpha _{0} \delta - \alpha _{1} + c \left( 1 - \delta \right) \right] \left\{ \begin{array}{c} 2 \alpha _{0} \delta \left[ \delta ^{2} + \gamma \left( 2 - \delta ^{2}\right) \right] + c \left( 1 - \delta \right) \left[ 4 + 4 \delta + \delta ^{2} - \delta ^{3} + \gamma \left( 4 - \delta ^{2} + \delta ^{3}\right) \right] - \alpha _{1} \left[ 4 - 3 \delta ^{2} + \delta ^{4} + \gamma \left( 4 - \delta ^{2} - \delta ^{4}\right) \right] \end{array} \right\} }{\beta \left( 1 - \delta \right) \left( 1 + \delta \right) \left[ 4 - \left( 1 - \gamma \right) \delta ^{2} \right] ^{2}}, \end{aligned}$$

where

$$\begin{aligned} {\left\{ \begin{array}{ll} QQ^{CS}_{1} = \left[ 4 - \left( 3 - 2 \gamma - \gamma ^{2}\right) \delta ^{2} \right] , \\ QQ^{CS}_{2} = \delta \left( 2 + \gamma + \gamma ^{2} - \delta ^{2} + \gamma \delta ^{2}\right) , \\ QQ^{CS}_{3} = \left[ 4 + \delta ^{2} - \delta ^{4} + 2 \gamma \left( 4 - \delta ^{2} + \delta ^{4}\right) + \gamma ^{2} \left( 4 + \delta ^{2} - \delta ^{4}\right) \right] , \\ QQ^{CS}_{4} = \left[ 20 + 4 \delta - 7 \delta ^{2} + \delta ^{3} + \gamma \left( 8 + 6 \delta ^{2} - 2 \delta ^{3}\right) + \gamma ^{2} \left( 4 - 4 \delta + \delta ^{2} + \delta ^{3}\right) \right] , \\ QQ^{CS}_{5} = \left[ 4 + \left( 2 - \gamma - \gamma ^{2}\right) \delta - \left( 1 - \gamma \right) \delta ^{2} \right] , \\ QQ^{CS}_{6} = \left[ 4 - 4 \delta - 3 \delta ^{2} + \delta ^{3} + \gamma \left( 8 + 4 \delta + 2 \delta ^{2} - 2 \delta ^{3}\right) + \gamma ^{2} \left( 4 + \delta ^{2} + \delta ^{3}\right) \right] . \end{array}\right. } \end{aligned}$$

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Nakamura, Y. Endogenous market structures in a mixed oligopoly with a public firm whose managerial contract is based on welfare and bargaining over the managerial contract of a private firm. Econ Polit 34, 189–209 (2017). https://doi.org/10.1007/s40888-017-0065-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40888-017-0065-3

Keywords

JEL Classification

Search

Navigation