Skip to main content
SpringerLink
Log in

Fractional Analysis of Unsteady Slip Flow of Viscous Fluid Confined to the Boundaries of an Annulus Driven by Exponentially Decaying/Growing Time-Dependent Pressure Gradient

  • Original Paper
  • Published:
International Journal of Applied and Computational Mathematics Aims and scope Submit manuscript

Abstract

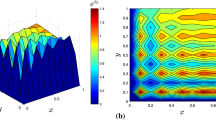
A theoretical investigation highlighting the effect of fractional-order parameter and slip boundaries on the motion of a viscous fluid in an annular domain induced by exponential time-dependent pressure gradient has been reported. The action of decaying/growing exponential time-dependent pressure gradient in the circumferential direction and fractional-order on the fractionalized governing momentum equation based on the Caputo-Fabrizio fractional model has been carried out. For proper insight into the physical problem, the fractionalized model was first transformed and solved in the Laplace domain. Afterwards, a numerical Laplace inversing scheme based on Tzou’s algorithm has been utilized to comparatively analyze the solution for the flow field, shear stresses and flow vortex for the model under scrutiny. It was found that the fluid flow can be made faster by applying a growing pressure gradient, time and wall slippage on both walls. The effect of the fractional-order parameter is to decrease the flow and shear stresses. The instability of the flow can be controlled by enhancing the memory effect.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24
Fig. 25

Similar content being viewed by others

Data Availability

The present paper has no data available.

Abbreviations

\({\mathrm{r}}_{1}\) :

Radius of the inner cylinder \((\mathrm{m})\)

\({\mathrm{r}}_{2}\) :

Radius of the outer cylinder \((\mathrm{m})\)

\(\mathrm{P}\) :

Static pressure \((\mathrm{Kg}/{\mathrm{ms}}^{2})\)

\(\mathrm{R}\) :

Dimensionless radius

\(\mathrm{s}\) :

Laplace parameter

\({\mathrm{t}}^{\prime}\) :

Dimensional time

\(\mathrm{r}^{\prime}\) :

Dimensional radial distance

\(\mathrm{t}\) :

Dimensionless time \((\mathrm{s})\)

\({\mathrm{U}}_{0}\) :

Reference velocity \((\mathrm{m}/\mathrm{s})\)

\({\mathrm{u}}_{\mathrm{r}^{\prime}}\) :

Radial velocity \((\mathrm{m}/\mathrm{s})\)

\(\mathrm{u}^{\prime}\) :

Circumferential velocity \((\mathrm{m}/\mathrm{s})\)

\(\mathrm{U}\) :

Dimensionless velocity

\(\upalpha \) :

Fractional-order parameter

\({\upbeta }_{1}\) :

Slip coefficient of inner cylinder

\({\upbeta }_{2}\) :

Slip coefficient of outer cylinder

\(\updelta \) :

Decaying/growing parameter of time-dependent pressure gradient

\({\upvarphi }\) :

Circumferential direction

\(\mathcal{L}\) :

Laplace parameter

\(\uplambda \) :

Radii ratio \(({\mathrm{r}}_{2}/{\mathrm{r}}_{1})\)

\(\uprho \) :

Fluid density \((\mathrm{Kg}/{\mathrm{m}}^{3})\)

\(\upomega \) :

Dean vortices

\(\uptau \) :

Skin friction

\(\upupsilon \) :

Dynamic viscosity of the fluid \((\mathrm{Kg}/\mathrm{ms})\)

References

  1. Dean, W.R.: XVI. Note on the motion of fluid in a curved pipe. London, Edinburgh Dublin Philos. Mag. J. Sci. 4(20), 208–223 (1927)

    Article  MATH  Google Scholar 

  2. Dean, W.R.: Fluid motion in a curved channel. Proc. R. Soc. A Math. Phys. Eng. Sci. 121(787), 402–420 (1928)

    MATH  Google Scholar 

  3. Richardson, E.G., Tyler, E.: The transverse velocity gradient near the mouths of pipes in which an alternating flow is established. Proc. Phys. Soc. 42(1), 1–15 (1929)

    Article  MATH  Google Scholar 

  4. Goldstein, S.: Modern Developments in Fluid Dynamics. Clarendon Press, Oxford, Vol. 1, pp. 315-316 (1997)

    Google Scholar 

  5. Uchida, S.: The pulsating viscous flow superposed on the steady laminar motion of incompressible fluid in a circular pipe. J. Appl. Math. Phys. 7, 403–422 (1956)

    MathSciNet  MATH  Google Scholar 

  6. Bhatnagar, R.K.: Flow of an oldroyd fluid in a circular pipe with time dependent pressure gradient. Appl. Sci. Res. 30(4), 241–267 (1975)

    Article  MATH  Google Scholar 

  7. Ioannou, I., Georgiou, G.C.: Annular pressure-driven flow of Bingham plastic with pressure-dependent rheological parameters. Rheol. Acta 58, 699–707 (2019)

    Article  Google Scholar 

  8. Womersley, J.R.: Method for the calculation of velocity, rate of flow and viscous drag in arteries when the pressure gradient is known. J. Physiol. 127(3), 553–563 (1995)

    Article  Google Scholar 

  9. Sud, V.K., Sekhon, G.S.: Arterial flow under periodic body acceleration. Bull. Math. Biol. 47(1), 35–52 (1985)

    Article  MATH  Google Scholar 

  10. Sud, V.K., Sekhon, G.S.: Analysis of blood flow through a model of the human arterial system under periodic body acceleration. J. Biomech. 19(11), 929–941 (1986)

    Article  Google Scholar 

  11. Tu, C., Deville, M., Dheur, L., Vanderschuren, L.: Finite element simulation of pulse tile flow through arterial stenosis. J. Biomech. 25(10), 1141–1152 (1992)

    Article  Google Scholar 

  12. Sinha, A., Shit, G.C., Kundu, P.K.: Slip effects on pulsatile flow of blood through a stenosed arterial segment under periodic body acceleration. ISRN Biomed. Eng. 2013, 1–10 (2013)

    Article  Google Scholar 

  13. Gupta, S., Poulikakos, D, Kurtcuoglu, V.: Analytical solution for pulsatile viscous flow in a straight elliptic annulus and application to the motion of the cerebrospinal fluid. Phys. Fluids, 20(9) (2008)

  14. Gambo, D., Gambo, J. J.: Role of suction/injection and slip flow on hydromagnetic free convective flow in a vertical coaxial cylinder under the influence of radial magnetic field. Heat Transf., pp. 1–13 (2021)

  15. Jha, B.K., Danjuma, Y.J.: Unsteady Dean flow formation in an annulus with partial slippage: a Riemann-sum approximation approach. Results Eng. 5, 1–10 (2020)

    Article  Google Scholar 

  16. Avramenko, A.A., Kuznetsov, A.V.: Instability of a slip flow in a curved channel formed by two concentric cylindrical surfaces. Eur. J. Mech. - B/Fluids 28(6), 722–727 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  17. Ramzan, M., Chung, J.D., Ullah, N.: Partial slip effect in the flow of MHD micropolar nanofluid flow due to a rotating disk: a numerical approach. Results Phys. 7, 3557–3566 (2017)

    Article  Google Scholar 

  18. Jha, B.K. Gambo, D.: Hydrodynamic effect of slip boundaries and exponentially decaying/growing time-dependent pressure gradient on Dean flow. J. Egypt. Math. Soc., 29, (11) (2021)

  19. Tsangaris, S. Oscillatory flow of an incompressible, viscous-fluid in a straight annular pipe. Journal de Mecanique Theorique et Appliquee, 3(3) (1984)

  20. Tsangaris, S., Kondaxakis, D., Vlachakis, N.W.: Exact solution of the Navier-Stokes equations for pulsating Dean flow in a channel with porous walls. Int. J. Eng. Sci. 44(11), 67–79 (2006)

    MATH  Google Scholar 

  21. Tsangaris, S., Vlachakis, N.W.: Exact solution for the pulsating finite gap dean flow. Appl. Math. Model. 31(9), 1899–1906 (2007)

    Article  MATH  Google Scholar 

  22. .

  23. Engheta, N.: On fractional calculus and fractional multi poles in electromagnetism. IEEE Trans. Antennas Propag. 44(4), 554–566 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  24. Imran, M.A.: Fractional mechanism with power law (Singular) and exponential (Non-singular) kernels and its applications in bio heat transfer model. Int. J. Heat Technol. 37(3), 846–852 (2019)

    Article  Google Scholar 

  25. Imran, M.A., Aleem, M., Riaz, M.B., Ali, R., Khan, I.: A comparative report on convective flow of fractional (ABC) and (CF) MHD viscous fluid subject to generalized boundary conditions. Chaos, Solitons Fractals 118, 274–289 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  26. Abdeljawad, T., Riaz, M.B., Saeed, S.T., Iftikhar, N.: MHD Maxwell fluid with heat transfer analysis under ramp velocity and ramp temperature subject to non-integer differential operators. Comput. Model. Eng. Sci. 126(2), 821–841 (2021)

    Google Scholar 

  27. Saeed, S.T., Riaz, M.B., Baleanu, D.: A fractional study of generalized Oldroyd-B fluid with ramped conditions via local and non-local kernals. Nonlinear Eng 10, 177–186 (2021)

    Article  Google Scholar 

  28. Safdar, R., Imran, M., Khalique, C.M.: Time-dependent flow model of a generalized Burger’s fluid with fractional derivatives through a cylindrical domain: an exact and numerical approach. Results Phys. 9, 237–245 (2018)

    Article  Google Scholar 

  29. Jha, B.K., Gambo, D.: Combined effects of suction/injection and exponentially decaying/growing time-dependent pressure gradient on unsteady Dean flow: a semi-analytical approach. GEM Int. J. Geomath. 11(28), 1–22 (2020)

    MathSciNet  MATH  Google Scholar 

  30. Jha, B.K., Gambo, D.: Role of exponentially decaying/growing time-dependent pressure gradient on unsteady Dean flow: a Riemann-sum approximation approach. Arab J. Basic Appl. Sci. 28(1), 1–10 (2021)

    Article  Google Scholar 

  31. Jha, B.K., Gambo, D.: Theoretical investigation on the impact of an oscillating time-dependent pressure gradient on Dean flow in a porous annulus. Propuls. Power Res. 10(3), 294–302 (2021)

    Article  Google Scholar 

  32. Jha, B.K., Gambo, D.: Effect of an oscillating time-dependent pressure gradient on Dean flow: transient solution. Beni-Suef Uni. J. Basic Appl. Sci. 9(39), 1–9 (2020)

    Google Scholar 

  33. Jafari, H., Mahmoudi, M., Noori Skandari, M.N.: "A new numerical method to solve pantograph delay differential equations with convergence analysis. Adv. Contin. Discrete Models 129, 1–12 (2021)

    MATH  Google Scholar 

  34. Kameli, A., Jafari, H., Moradi, A.: A new approach to solve linear systems. Int. J. Appl. Comput. Math., 6(180) (2021)

  35. Jafari, H., Jassim, H.K., Baleanu, D., Chu, Y.-M.: On the approximate solutions for a system of coupled Korteweg-de Vries equations with local fractional derivative. Fractals 29(5), 1–7 (2021)

    Article  MATH  Google Scholar 

  36. Ibrahim, R.W., Jafari, H., Jalab, H.A., Hadid, S.B.: Local fractional system for economic order quantity using entropy solution. Adv. Differ. Equ. 2019(96), 1–11 (2019)

    MathSciNet  MATH  Google Scholar 

  37. Hosseini, V.R., Koushki, M., Zou, W.-N.: The meshless approach for solving 2D variable-order time-fractional advection-diffusion equation arising in anomalous transport. Eng. Comput. 38(3), 2289–2307 (2022)

    Article  Google Scholar 

  38. Tzou, D.Y.: Macro to Microscale Heat Transfer: The Lagging Behavior. Taylor and Francis, London (1997)

    Google Scholar 

Download references

Funding

We declare that the authors received no funding in the course of this research.

Author information

Authors and Affiliations

  1. Department of Mathematics, Ahmadu Bello University, Zaria, Nigeria

    Basant K. Jha

  2. Department of Mathematics, Iowa State University, Ames, USA

    Dauda Gambo

  3. Department of Mathematics, Federal University Dutse, Dutse, Nigeria

    Umar M. Adam

Authors
  1. Basant K. Jha
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Dauda Gambo
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Umar M. Adam
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Dauda Gambo.

Ethics declarations

Conflict of interest

The authors have not disclosed any competing interests.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix

Appendix

$${\mathrm{A}}_{1}=\uplambda {\mathrm{K}}_{1}\left(\upgamma \right)-{\upbeta }_{2}{\mathrm{K}}_{1}\left(\upgamma \right)-{\uplambda }^{2}{\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right)+{\upbeta }_{2}\uplambda {\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right)-{\upbeta }_{1}{\upbeta }_{2}{\mathrm{K}}_{1}\left(\upgamma \right)+{\upbeta }_{1}\uplambda {\mathrm{K}}_{1}\left(\upgamma \right)-\upbeta {\uplambda }^{2}{\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right);$$
$${\mathrm{A}}_{2}={\upbeta }_{2}\mathrm{\alpha }{\mathrm{K}}_{1}\left(\upgamma \right)-\mathrm{\lambda \alpha }{\mathrm{K}}_{1}\left(\upgamma \right)+{\uplambda }^{2}\mathrm{\alpha }{\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right)+{\upbeta }_{1}{\upbeta }_{2}\mathrm{\alpha }{\mathrm{K}}_{1}\left(\upgamma \right)-{\upbeta }_{1}{\upbeta }_{2}\upgamma {\mathrm{K}}_{0}\left(\upgamma \right)-{\upbeta }_{1}\mathrm{\lambda \alpha }{\mathrm{K}}_{1}\left(\upgamma \right);$$
$${\mathrm{A}}_{3}={\upbeta }_{1}\mathrm{\lambda \gamma }{\mathrm{K}}_{0}\left(\upgamma \right)+{\upbeta }_{1}{\uplambda }^{2}\mathrm{\alpha }{\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right)+{\upbeta }_{2}{\uplambda }^{2}\upgamma {\mathrm{K}}_{0}\left(\mathrm{\lambda \gamma }\right);$$
$${\mathrm{A}}_{4}=\left(\frac{{\upbeta }_{2}\mathrm{\alpha }{\mathrm{K}}_{1}\left(\upgamma \right)}{\mathrm{s}}+\frac{\mathrm{\lambda \alpha }{\mathrm{K}}_{1}\left(\upgamma \right)}{\mathrm{s}}\right);$$
$${\mathrm{A}}_{5}=\left(\frac{{\uplambda }^{2}\mathrm{\alpha }{\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right)}{\mathrm{s}}\right);$$
$${\mathrm{A}}_{6}={\upbeta }_{1}{\upbeta }_{2}\uplambda {\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right)-{\upbeta }_{2}\mathrm{\lambda \alpha }{\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right)-{\upbeta }_{1}{\upbeta }_{2}\mathrm{\lambda \alpha }{\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right)+\left(\frac{{\upbeta }_{2}\mathrm{\lambda \alpha }{\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right)}{\mathrm{s}}\right)+{\upbeta }_{1}{\upbeta }_{2}{\uplambda }^{2}\upgamma {\mathrm{K}}_{0}\left(\mathrm{\lambda \gamma }\right);$$
$${\mathrm{A}}_{7}={\upbeta }_{1}{\upbeta }_{2}\mathrm{\alpha \gamma }{\mathrm{K}}_{0}\left(\upgamma \right)-{\upbeta }_{2}{\uplambda }^{2}\mathrm{\alpha \gamma }{\mathrm{K}}_{0}\left(\mathrm{\lambda \gamma }\right)-\left(\frac{{\upbeta }_{1}{\upbeta }_{2}\mathrm{\alpha }{\mathrm{K}}_{1}\left(\upgamma \right)}{\mathrm{s}}\right);$$
$${\mathrm{A}}_{8}=\left(\frac{{\upbeta }_{1}\mathrm{\lambda \alpha }{\mathrm{K}}_{1}\left(\upgamma \right)}{\mathrm{s}}\right)-\left(\frac{{\upbeta }_{1}{\uplambda }^{2}\mathrm{\alpha }{\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right)}{\mathrm{s}}\right)-\left(\frac{{\upbeta }_{1}{\upbeta }_{2}\mathrm{\alpha \gamma }{\mathrm{K}}_{0}\left(\upgamma \right)}{\mathrm{s}}\right)+\left(\frac{{\upbeta }_{1}\mathrm{\lambda \alpha \gamma }{\mathrm{K}}_{0}\left(\upgamma \right)}{\mathrm{s}}\right);$$
$${\mathrm{A}}_{9}=\left(\frac{{\upbeta }_{2}{\uplambda }^{2}\mathrm{\alpha \gamma }{\mathrm{K}}_{0}\left(\mathrm{\lambda \gamma }\right)}{\mathrm{s}}\right)+\left(\frac{{\upbeta }_{1}{\upbeta }_{2}\mathrm{\lambda \alpha }{\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right)}{\mathrm{s}}\right)-{\upbeta }_{1}{\upbeta }_{2}{\uplambda }^{2}\mathrm{\alpha \gamma }{\mathrm{K}}_{0}\left(\mathrm{\lambda \gamma }\right)+\left(\frac{{\upbeta }_{1}{\upbeta }_{2}{\uplambda }^{2}\mathrm{\alpha \gamma }{\mathrm{K}}_{0}\left(\mathrm{\lambda \gamma }\right)}{\mathrm{s}}\right);$$
$${\mathrm{A}}_{10}={\upbeta }_{2}{\mathrm{I}}_{1}\left(\upgamma \right)-\uplambda {\mathrm{I}}_{1}\left(\upgamma \right)+{\uplambda }^{2}{\mathrm{I}}_{1}\left(\mathrm{\lambda \gamma }\right)-{\upbeta }_{2}\uplambda {\mathrm{I}}_{1}\left(\mathrm{\lambda \gamma }\right)+{\upbeta }_{1}{\upbeta }_{2}{\mathrm{I}}_{1}\left(\upgamma \right)-{\upbeta }_{1}\uplambda {\mathrm{I}}_{1}\left(\upgamma \right)+\upbeta {\uplambda }^{2}{\mathrm{I}}_{1}\left(\mathrm{\lambda \gamma }\right)-{\upbeta }_{2}\mathrm{\alpha }{\mathrm{I}}_{1}\left(\upgamma \right);$$
$${\mathrm{A}}_{11}=\mathrm{\lambda \alpha }{\mathrm{I}}_{1}\left(\upgamma \right)-{\uplambda }^{2}\mathrm{\alpha }{\mathrm{I}}_{1}\left(\mathrm{\lambda \gamma }\right)-{\upbeta }_{1}{\upbeta }_{2}\mathrm{\alpha }{\mathrm{I}}_{1}\left(\upgamma \right)-{\upbeta }_{1}{\upbeta }_{2}\upgamma {\mathrm{I}}_{0}\left(\upgamma \right)+{\upbeta }_{1}\mathrm{\lambda \alpha }{\mathrm{I}}_{1}\left(\upgamma \right)+{\upbeta }_{1}\mathrm{\lambda \gamma }{\mathrm{I}}_{0}\left(\upgamma \right)-{\upbeta }_{1}{\uplambda }^{2}\mathrm{\alpha }{\mathrm{I}}_{1}\left(\mathrm{\lambda \gamma }\right);$$
$${\mathrm{A}}_{12}={\upbeta }_{2}{\uplambda }^{2}\upgamma {\mathrm{I}}_{0}\left(\mathrm{\lambda \gamma }\right)+\left(\frac{{\upbeta }_{2}\mathrm{\alpha }{\mathrm{I}}_{1}\left(\upgamma \right)}{\mathrm{s}}-\frac{\mathrm{\lambda \alpha }{\mathrm{I}}_{1}\left(\upgamma \right)}{\mathrm{s}}\right)+\left(\frac{{\uplambda }^{2}\mathrm{\alpha }{\mathrm{I}}_{1}\left(\mathrm{\lambda \gamma }\right)}{\mathrm{s}}\right)-{\upbeta }_{1}{\upbeta }_{2}\uplambda {\mathrm{I}}_{1}\left(\mathrm{\lambda \gamma }\right)+{\upbeta }_{2}\mathrm{\lambda \alpha }{\mathrm{I}}_{1}\left(\mathrm{\lambda \gamma }\right);$$
$${\mathrm{A}}_{13}={\upbeta }_{1}{\upbeta }_{2}\mathrm{\lambda \alpha }{\mathrm{I}}_{1}\left(\mathrm{\lambda \gamma }\right)-\left(\frac{{\upbeta }_{2}\mathrm{\lambda \alpha }{\mathrm{I}}_{1}\left(\mathrm{\lambda \gamma }\right)}{\mathrm{s}}\right)+{\upbeta }_{1}{\upbeta }_{2}{\uplambda }^{2}\upgamma {\mathrm{I}}_{0}\left(\mathrm{\lambda \gamma }\right)+{\upbeta }_{1}{\upbeta }_{2}\mathrm{\alpha \gamma }{\mathrm{I}}_{0}\left(\upgamma \right)-{\upbeta }_{2}{\uplambda }^{2}\mathrm{\alpha \gamma }{\mathrm{I}}_{0}\left(\mathrm{\lambda \gamma }\right);$$
$${\mathrm{A}}_{14}=\left(\frac{{\upbeta }_{1}{\upbeta }_{2}\mathrm{\alpha }{\mathrm{I}}_{1}\left(\upgamma \right)}{\mathrm{s}}\right)-\left(\frac{{\upbeta }_{1}\mathrm{\lambda \alpha }{\mathrm{I}}_{1}\left(\upgamma \right)}{\mathrm{s}}\right)+\left(\frac{{\upbeta }_{1}{\uplambda }^{2}\mathrm{\alpha }{\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right)}{\mathrm{s}}\right)-\left(\frac{{\upbeta }_{1}{\upbeta }_{2}\mathrm{\alpha \gamma }{\mathrm{I}}_{0}\left(\upgamma \right)}{\mathrm{s}}\right);$$
$${\mathrm{A}}_{15}=\left(\frac{{\upbeta }_{1}\mathrm{\lambda \alpha \gamma }{\mathrm{I}}_{0}\left(\upgamma \right)}{\mathrm{s}}\right)+\left(\frac{{\upbeta }_{2}{\uplambda }^{2}\mathrm{\alpha \gamma }{\mathrm{I}}_{0}\left(\mathrm{\lambda \gamma }\right)}{\mathrm{s}}\right)-\left(\frac{{\upbeta }_{1}{\upbeta }_{2}\mathrm{\lambda \alpha }{\mathrm{I}}_{1}\left(\mathrm{\lambda \gamma }\right)}{\mathrm{s}}\right)-{\upbeta }_{1}{\upbeta }_{2}{\uplambda }^{2}\mathrm{\alpha \gamma }{\mathrm{I}}_{0}\left(\mathrm{\lambda \gamma }\right)+\left(\frac{{\upbeta }_{1}{\upbeta }_{2}{\uplambda }^{2}\mathrm{\alpha \gamma }{\mathrm{I}}_{0}\left(\mathrm{\lambda \gamma }\right)}{\mathrm{s}}\right);$$
$${\mathrm{A}}_{16}=\mathrm{\lambda s}\left(\updelta +\mathrm{s}\right);$$
$${\mathrm{A}}_{17}={\upbeta }_{2}{\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right){\mathrm{I}}_{1}\left(\upgamma \right)-{\upbeta }_{2}{\mathrm{I}}_{1}\left(\mathrm{\lambda \gamma }\right){\mathrm{K}}_{1}\left(\upgamma \right)+\uplambda {\mathrm{I}}_{1}\left(\mathrm{\lambda \gamma }\right){\mathrm{K}}_{1}\left(\upgamma \right)-\uplambda {\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right){\mathrm{I}}_{1}\left(\upgamma \right)-{\upbeta }_{1}{\upbeta }_{2}{\mathrm{I}}_{1}\left(\mathrm{\lambda \gamma }\right){\mathrm{K}}_{1}\left(\upgamma \right);$$
$${\mathrm{A}}_{18}={\upbeta }_{1}{\upbeta }_{2}{\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right){\mathrm{I}}_{1}\left(\upgamma \right)+{\upbeta }_{1}\uplambda {\mathrm{I}}_{1}\left(\mathrm{\lambda \gamma }\right){\mathrm{K}}_{1}\left(\upgamma \right)-{\upbeta }_{1}\uplambda {\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right){\mathrm{I}}_{1}\left(\upgamma \right)-{\upbeta }_{1}{\upbeta }_{2}\upgamma {\mathrm{I}}_{1}\left(\mathrm{\lambda \gamma }\right){\mathrm{K}}_{0}\left(\upgamma \right)-{\upbeta }_{1}{\upbeta }_{2}\upgamma {\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right){\mathrm{I}}_{0}\left(\mathrm{\lambda \gamma }\right);$$
$${\mathrm{A}}_{19}={\upbeta }_{1}\mathrm{\lambda \gamma }{\mathrm{I}}_{1}\left(\mathrm{\lambda \gamma }\right){\mathrm{K}}_{0}\left(\upgamma \right)+{\upbeta }_{1}\mathrm{\lambda \gamma }{\mathrm{K}}_{1}\left(\mathrm{\lambda \gamma }\right){\mathrm{I}}_{0}\left(\upgamma \right)+{\upbeta }_{2}\mathrm{\lambda \gamma }{\mathrm{I}}_{0}\left(\mathrm{\lambda \gamma }\right){\mathrm{K}}_{1}\left(\upgamma \right)+{\upbeta }_{2}\mathrm{\lambda \gamma }{\mathrm{K}}_{0}\left(\mathrm{\lambda \gamma }\right){\mathrm{I}}_{1}\left(\upgamma \right);$$
$${\mathrm{A}}_{20}={\upbeta }_{1}{\upbeta }_{2}\uplambda {\upgamma }^{2}{\mathrm{I}}_{0}\left(\mathrm{\lambda \gamma }\right){\mathrm{K}}_{0}\left(\upgamma \right)-{\upbeta }_{1}{\upbeta }_{2}\uplambda {\upgamma }^{2}{\mathrm{K}}_{0}\left(\mathrm{\lambda \gamma }\right){\mathrm{I}}_{0}\left(\upgamma \right)+{\upbeta }_{1}{\upbeta }_{2}\mathrm{\lambda \gamma }{\mathrm{I}}_{0}\left(\mathrm{\lambda \gamma }\right){\mathrm{K}}_{1}\left(\upgamma \right)+{\upbeta }_{1}{\upbeta }_{2}\mathrm{\lambda \gamma }{\mathrm{K}}_{0}\left(\mathrm{\lambda \gamma }\right){\mathrm{I}}_{1}\left(\upgamma \right);$$
$${\mathrm{C}}_{1}=\frac{{-(\mathrm{A}}_{1}+{\mathrm{A}}_{2}+{\mathrm{A}}_{3}-{\mathrm{A}}_{4}-{\mathrm{A}}_{5}+{\mathrm{A}}_{6}+{\mathrm{A}}_{7}+{\mathrm{A}}_{8}+{\mathrm{A}}_{9})}{{\mathrm{A}}_{16}({\mathrm{A}}_{17}+{\mathrm{A}}_{18}+{\mathrm{A}}_{19}+{\mathrm{A}}_{20})};$$
$${\mathrm{C}}_{2}=\frac{{-(\mathrm{A}}_{10}+{\mathrm{A}}_{11}+{\mathrm{A}}_{12}+{\mathrm{A}}_{13}+{\mathrm{A}}_{14}+{\mathrm{A}}_{15})}{{\mathrm{A}}_{16}({\mathrm{A}}_{17}+{\mathrm{A}}_{18}+{\mathrm{A}}_{19}+{\mathrm{A}}_{20})};$$

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Jha, B.K., Gambo, D. & Adam, U.M. Fractional Analysis of Unsteady Slip Flow of Viscous Fluid Confined to the Boundaries of an Annulus Driven by Exponentially Decaying/Growing Time-Dependent Pressure Gradient. Int. J. Appl. Comput. Math 9, 16 (2023). https://doi.org/10.1007/s40819-022-01486-z

Download citation

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s40819-022-01486-z

Keywords

Search

Navigation