Strategic Delegation with Differentiated Products

Abstract

This paper contributes to the analytical research on the profit implications of delegating production decisions to managers in the context of cost-asymmetric competing firms. We generalize previous studies by relaxing the assumption of product homogeneity. We demonstrate that the interplay between strategic delegation and the degree of product differentiation may lead to the reversal of the classic profit-ranking of Cournot equilibria. We discuss the drivers beyond the pure marginal cost asymmetry, which lead to this reversal: specifically, how the abilities to delegate production decisions and to choose the extent of such delegation further expand the parameter space where the high-cost firm is earning higher equilibrium profits compared to its more efficient rival.

Appendix

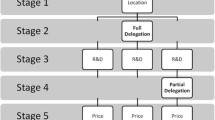
To prove proposition 1 and 2, we need to calculate the results in Table 1. By using backward induction, we solve the manager’s profit maximization problem first. The manager’s profit is ${M}_{i}=\left({p}_{i}-{\alpha }_{i}{c}_{i}\right){q}_{i}=\left(1-{{q}_{i}-\gamma }_{i}{q}_{-i}-{\alpha }_{i}{c}_{i}\right){q}_{i}$. If ${\alpha }_{i}=1,$ then the manager is not allowed to delegate otherwise s/he can delegate. To maximize the manager’s profits we take the derivative of their profit function ${M}_{i}({q}_{i},{q}_{i})$ with respect to ${q}_{i}$, simultaneously solve two FOCs, and obtain the optimal order quantities: ${q}_{i}^{*}({\alpha }_{A},{\alpha }_{B})=\frac{2-2{\alpha }_{i}{c}_{i}-{\gamma }_{i}+{\alpha }_{-i}{c}_{-i}{\gamma }_{i}}{4-{\gamma }_{A}{\gamma }_{B}}$. Next, we substitute ${q}_{i}^{*}({\alpha }_{A},{\alpha }_{B})$ into the firms’ profit functions ${\Pi }_{i}=\left({p}_{i}-{c}_{i}\right){q}_{i}$, which results in the following expression:

$${\Pi }_{i}^{*}({\alpha }_{A},{\alpha }_{B})=\frac{\left(2(1-{\alpha }_{i}{c}_{i})-{\gamma }_{i}(1-{\alpha }_{-i}{c}_{-i})\right)\left(2-{\gamma }_{i}(1-{\alpha }_{-i}{c}_{-i})-{c}_{i}\left(4-{\gamma }_{A}{\gamma }_{B}-{\alpha }_{i}\left(2-{\gamma }_{A}{\gamma }_{B}\right)\right)\right)}{{\left(4-{\gamma }_{A}{\gamma }_{B}\right)}^{2}}$$

Subgame $({\varvec{D}},{\varvec{D}})$

If both firms delegate, then the firm’s profit maximization problem is $\underset{{\mathrm{\alpha }}_{\mathrm{i}}\in (\mathrm{0,1})}{\mathrm{max}}{\Pi }_{i}^{*}$, and the profit maximizing degree of delegation ${\alpha }_{i}^{D/D}$ is listed in Table 1. By substituting ${\alpha }_{i}^{D/D}$ into ${q}_{i}^{*}({\alpha }_{A},{\alpha }_{B})$ and ${\Pi }_{i}^{*}\left({\alpha }_{A},{\alpha }_{B}\right),$ we obtain the corresponding optimal quantities ${q}_{i}^{D/D}$ and profits ${\Pi }_{i}^{D/D}$, also listed in Table 1.

Note that parameters ${c}_{i}$ and ${\gamma }_{i}$ must belong to a certain subset of parameter space, denoted the feasibility region${F}^{D/D}$, to ensure that optimal prices and quantities are positive in this subgame (${q}_{i}^{D/D}>0 ,{p}_{i}^{D/D}>0)$. The inequalities defining ${F}^{D/D}$ are lengthy but are available from the authors upon request.

Subgame $\left({\varvec{N}}{\varvec{D}},{\varvec{D}}\right)$ or $({\varvec{D}},{\varvec{N}}{\varvec{D}})$

If one firm delegates and the other does not then ${\alpha }_{i}\in (\mathrm{0,1})$ and ${\alpha }_{-i}=1$. Let us focus on $(ND,D)$ with ${\alpha }_{A}=1,{\alpha }_{B}\in (\mathrm{0,1})$ (the derivations for subgame $(ND,D)$ are similar). The optimal degree of delegation, ${\alpha }_{B}^{ND/D}$, which solves $\underset{{\alpha }_{B}\in (\mathrm{0,1})}{\mathrm{max}}{\Pi }_{B}$, is listed in Table 1 along with corresponding optimal quantities ${q}_{i}^{ND/D}$ and profits ${\Pi }_{i}^{ND/D}$. The feasibility region for parameters ${c}_{i}$ and ${\gamma }_{i}$ (resulting in positive prices and quantities in this subgame) denoted ${F}^{ND/D}({F}^{D/ND})$ (available upon request).

Subgame $\left({\varvec{N}}{\varvec{D}},{\varvec{N}}{\varvec{D}}\right)$

If neither firm delegates, then ${\alpha }_{A}={\alpha }_{B}=1,$ and the corresponding optimal production quantities ${q}_{i}^{ND/ND}$ and profits ${\Pi }_{i}^{ND/ND}$ are listed in Table 1. The set of feasible parameter values in this subgame is denoted ${F}^{ND/ND}$.

Subgame Perfect Nash Equilibrium

It is straightforward to see that (1) ${\Pi }_{A}^{ND/ND}<{\Pi }_{A}^{D/ND}$, and therefore subgame (ND,ND) is never an equilibrium; (2) ${\Pi }_{A}^{D/D}>{\Pi }_{A}^{ND/D}$ and ${\Pi }_{B}^{D/D}>{\Pi }_{B}^{D/ND}$, and therefore the subgame (D,D) is the unique SPNE. Throughout the paper, we focus on the feasible set of cost parameters: ${c}_{i}\in F={\cap }_{j\in \{D/D,ND/ND,D/ND,ND/D\}}{F}^{j}$.

• Proof of Proposition 1: Using the profit expressions presented in Table 1, we find that$({\Pi }_{A}^{ND/ND}\ge {\Pi }_{B}^{ND/ND}$ and ${\Pi }_{A}^{D/D}\le {\Pi }_{B}^{D/D})$ $\mathrm{\acute{o} } (\frac{{c}_{B}\left(4+{\gamma }_{A}\left(2-{\gamma }_{B}\right)\right)-2\left({\gamma }_{A}-{\gamma }_{B}\right)}{4+\left(2-{\gamma }_{A}\right){\gamma }_{B}}\le {c}_{A}\le \frac{{c}_{B}\left(2+{\gamma }_{A}\right)+{\gamma }_{B}-{\gamma }_{A}}{2+{\gamma }_{B}})$. Denote ${f}_{L}\left({c}_{B}\right)=\frac{{c}_{B}\left(4+{\gamma }_{A}\left(2-{\gamma }_{B}\right)\right)-2\left({\gamma }_{A}-{\gamma }_{B}\right)}{4+\left(2-{\gamma }_{A}\right){\gamma }_{B}}\mathrm{ and }{f}_{U}\left({c}_{B}\right)=\frac{{c}_{B}\left(2+{\gamma }_{A}\right)+{\gamma }_{B}-{\gamma }_{A}}{2+{\gamma }_{B}}$ ∎

• Proof of Proposition 2: Using the profit expressions presented in Table 1, we find that ${(\Pi }_{A}^{ND/ND}\le {\Pi }_{B}^{ND/ND})$$\left({c}_{B}-{c}_{A}\le {\frac{\left(1-{\mathrm{c}}_{\mathrm{A}}\right)\left({\upgamma }_{\mathrm{A}}-{\upgamma }_{\mathrm{B}}\right)}{2+{\upgamma }_{\mathrm{A}}}=G}^{ND/ND}\left({\gamma }_{A},{\gamma }_{B},{c}_{A}\right)\right);$

• (${\Pi }_{A}^{D/D}\le {\Pi }_{B}^{D/D}$)$\left({c}_{B}-{c}_{A}\le \frac{2\left(1-{\mathrm{c}}_{\mathrm{A}}\right)\left({\upgamma }_{\mathrm{A}}-{\upgamma }_{\mathrm{B}}\right)}{4+{\upgamma }_{\mathrm{A}}\left(2-{\upgamma }_{\mathrm{B}}\right)}{=G}^{D/D}\left({\gamma }_{A},{\gamma }_{B},{c}_{A}\right)\right).$

• A straightforward comparison demonstrates that ${G}^{D/D}\left({\gamma }_{A},{\gamma }_{B},{c}_{A}\right)\ge {G}^{ND/ND}({\gamma }_{A},{\gamma }_{B},{c}_{A})$. ∎

• Proof of Proposition 3: Using the degree of delegation expressions presented in Table 1, it is straightforward to demonstrate that

  • (a) ${\alpha }_{B}^{D/D}<{\alpha }_{A}^{D/D}$$\gamma_{B} < \frac{{2c_{B} \left( {2 - \left( {1 - c_{B} } \right)\gamma_{A} } \right) - 4c_{A} }}{{2c_{A}^{2} + c_{B} \gamma_{A} - c_{A} \left( {2 + \gamma_{A} } \right)}}$, and.

  • (b)${c}_{B}{\alpha }_{B}^{D/D}<{c}_{A}{\alpha }_{A}^{D/D }$$c_{B} < c_{A} + \frac{{\left( {1 - c_{A} } \right)\left( {\gamma_{A} - \gamma_{B} } \right)\gamma_{A} \gamma_{B} }}{{8 - 4\gamma_{A} \gamma_{B} + \gamma_{A}^{2} \gamma_{B} }}$.∎

• Proof of Lemma 1: Using the profit expressions presented in Table 1, we find that.

  • (1) ${\Pi }_{B}^{D/D}\le {\Pi }_{B}^\frac{ND}{ND}$ always holds; and

  • (2) $\begin{array}{l}{(\Pi}_A^{D/D}\geq\Pi_A^{ND/ND})\;\Leftrightarrow c_B-c_A>\left(1-c_A\right)\left[{(\gamma}_A-2\right)\left(\left(4-\gamma_A\gamma_B\right)^2-4\gamma_A\gamma_B\right)+\\\sqrt{4-2\gamma_A\gamma_B}(\left(4-\gamma_A\gamma_B\right)^2-2\gamma_A(4-\gamma_A\gamma_B)\rbrack/\lbrack\gamma_A(\left(4-\gamma_A\gamma_B\right)^2-\\4\gamma_A\gamma_B-2\sqrt{4-2\gamma_A\gamma_B}({4-\gamma}_A\gamma_B))\rbrack\end{array}$ ∎