Adaptive robust nonlinear control of spacecraft formation flying: a novel disturbance observer-based control approach

Abstract

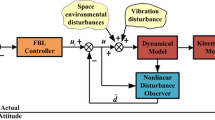
This paper proposes a novel nonlinear disturbance observer (NDO)-based control approach for spacecraft formation flying (SFF) subject to non-vanishing disturbances. The external disturbance from the space environment and modeled/unmodeled uncertainties are aggregated as a lumped unknown external disturbance. A novel NDO is designed to provide an estimation of unknown external disturbance. The adaptive robust control approach is combined with the NDO-based control approach to get a composite adaptive controller. In the resulting controller, the disturbance estimation is utilized as feed-forward to attenuate the disturbance effects. The asymptotic stability of the proposed composite adaptive controller is proved using the Lyapunov theorem. In previous NDO-based controllers designed for nonlinear systems, either the time derivative of external disturbance is assumed to be equal to zero, or the knowledge of external disturbance upper-bound is required to be known. However, the proposed controller neither makes presumption on the magnitude of the disturbance nor its time derivatives. Furthermore, compared with some existing NDO-based controllers, the conditions of previous methods on the magnitudes of controller parameters are relaxed. Simulation results, along with comparisons, are included to verify the effectiveness of the proposed control scheme. Compared to the previous NDO-based control methods, the proposed method provides better tracking performance in the presence of external disturbances, besides relaxing the restrictions of previous NDO control methods.

Appendices

Appendix A

Differentiating the definition of ${\varvec{z}}_{1}$ by Eq. (8), we obtain

$$ \dot{\varvec{z}}_{1} = \dot{\varvec{\rho }} - \dot{\varvec{\rho }}_{d} . $$

(47)

Considering the definition of ${\varvec{e}}_{2}$ by Eq. (8), we can rewrite Eq. (47) as

$$ \dot{\varvec{z}}_{1} = {\varvec{e}}_{2} . $$

(48)

Considering the definition of ${\varvec{z}}_{2}$ by Eq. (9), we can rewrite Eq. (48) as

$$ \dot{\varvec{z}}_{1} = {\varvec{z}}_{2} - {{\varvec{\Lambda}}} {\varvec{z}}_{1} . $$

(49)

Differentiating the definition of ${\varvec{z}}_{2}$ by Eq. (9), we obtain

$$ \dot{\varvec{z}}_{2} = \ddot{\varvec{\rho }} - \ddot{\varvec{\rho }}_{d} + {{\varvec{\Lambda}}} \dot{\varvec{z}}_{1} , $$

(50)

Substituting for $\ddot{\varvec{\rho }}$ from Eq. (1) in Eq. (50) and substituting for $\dot{\varvec{z}}_{1}$ from Eq. (49), we obtain

$$ \dot{\varvec{z}}_{2} = - {\varvec{C}}\left( \omega \right)\dot{\varvec{\rho }} - {\varvec{N}}\left( {{\varvec{\rho}},\omega ,{\varvec{r}}_{c} ,{\varvec{u}}_{l} } \right) - {\varvec{d}} + {\varvec{u}}_{f} - \ddot{\varvec{\rho }}_{d} + {{\varvec{\Lambda}}} \left( {{\varvec{z}}_{2} - {{\varvec{\Lambda}}} {\varvec{z}}_{1} } \right). $$

(51)

Considering ${\varvec{f}}\left( . \right)$ calculated by Eq. (11), the Eq. (51) can be rewritten as

$$ \dot{\varvec{z}}_{2} = {\varvec{f}}\left( {{\varvec{z}}_{1} ,{\varvec{z}}_{2} , \omega ,{\varvec{r}}_{c} ,{\varvec{u}}_{l} } \right) - {\varvec{d}} + {\varvec{u}}_{f} . $$

(52)

Appendix B

As mentioned in [15], the right-hand side of Eq. (11) can be linearly parameterized as

$$ {\varvec{f}}\left( {{\varvec{z}}_{1} ,{\varvec{z}}_{2} , \omega ,{\varvec{r}}_{c} ,{\varvec{u}}_{l} } \right) = - \ddot{\varvec{\rho }}_{d} - {\varvec{C}}\left( \omega \right)\dot{\varvec{\rho }} - {\varvec{N}}\left( {{\varvec{\rho}},\omega ,{\varvec{r}}_{c} ,{\varvec{u}}_{l} } \right) + {{\varvec{\Lambda}}}\left( {{\varvec{z}}_{2} - {{\varvec{\Lambda}}}{\varvec{z}}_{1} } \right) = {\varvec{W}}\left( {{\varvec{\xi}},{\varvec{z}}_{1} ,{\varvec{z}}_{2} , \omega ,{\varvec{r}}_{c} ,{\varvec{u}}_{l} } \right){\varvec{\theta}}, $$

(53)

where ${\varvec{\xi}} \in {\mathbb{R}}^{3}$ is the dummy variable which is a function of the desired position, velocity and acceleration of the follower spacecraft with respect to the leader one, ${\varvec{W}}\left( {{\varvec{\xi}},{\varvec{z}}_{1} ,{\varvec{z}}_{2} , \omega ,{\varvec{r}}_{c} ,{\varvec{u}}_{l} } \right) \in {\mathbb{R}}^{3 \times 3}$ represents the regression matrix, and ${\varvec{\theta}} \in {\mathbb{R}}^{3}$ the system parameters. Consequently, $\overline{\varvec{d}}\left( t \right)$, calculated by Eq. (10), can be rearranged by

$$ \overline{\varvec{d}}\left( t \right) = {\varvec{W}}\left( {{\varvec{z}}_{1} ,{\varvec{z}}_{2} , \omega ,{\varvec{r}}_{c} ,{\varvec{u}}_{l} } \right){{\varvec{\Gamma}}} - {\varvec{d}}, $$

(54)

where ${{\varvec{\Gamma}}} = {\varvec{\theta}} - \overline{\varvec{\theta }}$ represents the deviation of system parameters (i.e., ${\varvec{\theta}}$) from their nominal magnitudes (i.e.,$\varvec{ \overline{\theta }}$). In other words, ${{\varvec{\Gamma}}}$ represents uncertainties in system parameters.

Equation (54) can be upper-bounded as

$$ \left\| {\overline{\varvec{d}}} \right\| = \left\| {{\varvec{W}}\left( . \right)} \right\|\left\| {{\varvec{\Gamma}}} \right\| + \left\| {\varvec{d}} \right\|. $$

(55)

The regression matrix ${\varvec{W}}$, as defined in Eq. (53), is the function of leader spacecraft control input, position, and rotation rate (i.e., ${\varvec{u}}_{l} , {\varvec{r}}_{c} , \omega$), and the desired trajectories (i.e., ${\varvec{\rho}}_{d} , \dot{\varvec{\rho }}_{d} , \ddot{\varvec{\rho }}_{d}$), and the position and velocity tracking errors of the leader spacecraft with respect to follower one (i.e., ${\varvec{z}}_{1} ,{\varvec{z}}_{2}$).

The position, rate of orientation, and control input of the leader spacecraft are bounded inputs that are set by the leader spacecraft, and the desired trajectories are bounded vectors that are prescribed based on the desired demand. Let’s define the augmented vector ${\varvec{z}} = \left[ {\begin{array}{*{20}c} {{\varvec{z}}_{1} } \\ {{\varvec{z}}_{2} } \\ \end{array} } \right]$. As mentioned in [15], it can be stated that if the position and velocity tracking error of the follower spacecraft remain in the bounded set $\Omega_{3} = \left\{ {\left. {\varvec{z}} \right|\varvec{ }\left\| {\varvec{z}} \right\| \le \ell } \right\}$, we have $\left\| {{\varvec{W}}\left( . \right)} \right\| \le \mu$. where $\mu$ is a positive constant. As long as the external disturbance and parametric uncertainties remain bounded, i.e. $\left\| {\varvec{d}} \right\| \le \gamma$ and $\left\| {{\varvec{\Gamma}}} \right\| \le \varphi$, it can be stated that

$$ \left\| {\overline{\varvec{d}}} \right\| \le \mu \varphi + \gamma \le \delta , $$

(56)

where $\gamma , \varphi , \delta$ are positive constants. In Sect. 4, it is proved that any trajectories initialized within $\Omega_{1} = \left\{ {{\varvec{X}}{|} \left\| {{\varvec{X}}\left( t \right)} \right\| \le \frac{{A_{m} }}{{A_{M} }}\ell } \right\} \subset \Omega_{3}$ will remain within $\Omega_{2} = \left\{ {{\varvec{X}}{|} \left\| {{\varvec{X}}\left( t \right)} \right\| \le \ell } \right\} \subset \Omega_{3}$ and consequently the assumption (56) is consistent.

It is worth highlighting that the proposed control method does not require the knowledge of $\delta$ and just requires its existence. In fact, the adaptive robust estimator estimates the upper-bound $\delta .$

Appendix C

Substituting Eqs. (22), (25), (29), (18) in Eq. (31) we obtain

$$ \dot{V} = {\varvec{z}}_{1}^{T} \left( {{\varvec{z}}_{2} - {{\varvec{\Lambda}}}{\varvec{z}}_{1} } \right) + {\varvec{z}}_{2}^{T} \left( { - {\varvec{K}}_{1} {\varvec{z}}_{2} + {\dot{\tilde{\varvec{\beta} }}}} \right) + \tilde{\varvec{\beta }}^{T} {\dot{\tilde{\varvec{\beta} }}} + k_{\delta }^{ - 1} \tilde{\delta } \left( { - k_{\delta } \left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\|} \right) + k_{\varepsilon }^{ - 1} \varepsilon \left( { - k_{\varepsilon } \varepsilon } \right) . $$

(57)

Equation (57) can be rearranged by

$$ \dot{V} = {\varvec{z}}_{1}^{T} \left( {{\varvec{z}}_{2} - {{\varvec{\Lambda}}}{\varvec{z}}_{1} } \right) + {\varvec{z}}_{2}^{T} \left( { - {\varvec{K}}_{1} {\varvec{z}}_{2} } \right) + ({\varvec{z}}_{2} + \tilde{\varvec{\beta }})^{T} {\dot{\tilde{\varvec{\beta} }}} - \tilde{\delta }\left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| - \varepsilon^{2} . $$

(58)

Substituting Eq. (27) in the result to obtain

$$ \dot{V} = {\varvec{z}}_{1}^{T} \left( {{\varvec{z}}_{2} - {\Lambda }{\varvec{z}}_{1} } \right) + {\varvec{z}}_{2}^{T} \left( { - {\varvec{K}}_{1} {\varvec{z}}_{2} } \right) + ({\varvec{z}}_{2} + \tilde{\varvec{\beta }})^{T} \left[ {\dot{\varvec{\beta }} - {\varvec{K}}_{2} \left( {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right) - \frac{{\hat{\delta }^{2} \left( {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right)}}{{\hat{\delta }\left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| + \varepsilon^{2} }}} \right] - \tilde{\delta }\left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| - \varepsilon^{2} . $$

(59)

Considering that for any arbitrary vectors ${\varvec{a}}$ and ${\varvec{b}}$, we have ${\varvec{a}}^{T} {\varvec{b}} \le \left\| {\varvec{a}} \right\| \left\| {\varvec{b}} \right\| \le \frac{1}{2}\left( {\left\| {\varvec{a}} \right\|^{2} + \left\| {\varvec{b}} \right\|^{2} } \right)$, and ${\varvec{a}}^{T} {\varvec{a}} = \left\| {\varvec{a}} \right\|^{2}$, we have

$$ \begin{aligned} & {\varvec{z}}_{1}^{T} \left( { - {{\varvec{\Lambda}}}{\varvec{z}}_{1} } \right) \le - {\Lambda }_{m} \left\| {{\varvec{z}}_{1} } \right\|^{2} , \\ & {\varvec{z}}_{2}^{T} \left( { - {\varvec{K}}_{1} {\varvec{z}}_{2} } \right) \le - k_{1m} \left\| {{\varvec{z}}_{2} } \right\|^{2} , \\ & ({\varvec{z}}_{2} + \tilde{\varvec{\beta }})^{T} \left( { - K_{2} \left( {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right)} \right) \le - k_{2m} \left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\|^{2} , \\ & ({\varvec{z}}_{2} + \tilde{\varvec{\beta }})^{T} \dot{\varvec{\beta }} \le \left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\|{ }\left\| {\overline{\varvec{d}}} \right\| \le \delta \left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\|, \\ & \left( {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right)^{T} \left[ { - \frac{{\hat{\delta }^{2} \left( {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right)}}{{\hat{\delta }\left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| + \varepsilon^{2} }}} \right] \le - \frac{{\hat{\delta }^{2} \left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\|^{2} }}{{\hat{\delta }\left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| + \varepsilon^{2} }}. \\ \end{aligned} $$

(60)

where ${\Lambda }_{m} ,k_{1m} , k_{2m}$ represent the minimum eigenvalues of matrices ${{\varvec{\Lambda}}},\varvec{ K}_{1} ,\varvec{ K}_{2}$. Considering Eq. (60), the Eq. (59) can be upper-bounded as

$$ \dot{V} \le \frac{1}{2}\left( {\left\| {{\varvec{z}}_{1} } \right\|^{2} + \left\| {{\varvec{z}}_{2} } \right\|^{2} } \right) - {\Lambda }_{{\text{m}}} \left\| {{\varvec{z}}_{1} } \right\|^{2} - k_{1m} \left\| {{\varvec{z}}_{2} } \right\|^{2} + \delta \left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| - k_{2m} \left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\|^{2} - \frac{{\hat{\delta }^{2} \left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\|^{2} }}{{\hat{\delta }\left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| + \varepsilon^{2} }} - \tilde{\delta }\left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| - \varepsilon^{2} . $$

(61)

Considering the definition given in Eq. (28), we have

$$ - \tilde{\delta }\left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| + \delta \left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| = \hat{\delta }\left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\|, $$

(62)

moreover, it can be easily verified that

$$ - \frac{{\hat{\delta }^{2} \left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\|^{2} }}{{\hat{\delta }\left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| + \varepsilon^{2} }} + \hat{\delta }\left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| - \varepsilon^{2} = \frac{{ - \hat{\delta }^{2} \left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\|^{2} + \hat{\delta }^{2} \left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\|^{2} + \varepsilon^{2} \hat{\delta }\left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| - \varepsilon^{2} \hat{\delta }\left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| - \varepsilon^{4} }}{{\hat{\delta }\left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| + \varepsilon^{2} }} = \frac{{ - \varepsilon^{4} }}{{\hat{\delta }\left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| + \varepsilon^{2} }}. $$

(63)

Considering Eqs. (62) and (63), we can simplify Eq. (61) as

$$ \dot{V} \le \left[ {\frac{1}{2} - {\Lambda }_{{\text{m}}} } \right]\left\| {{\varvec{z}}_{1} } \right\|^{2} + \left[ {\frac{1}{2} - k_{1m} } \right]\left\| {{\varvec{z}}_{2} } \right\|^{2} - k_{2m} \left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\|^{2} - \frac{{\varepsilon^{4} }}{{\hat{\delta }\left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| + \varepsilon^{2} }}. $$

(64)

Consequently, if the control gains are selected such that conditions in Eq. (33) are satisfied, we have

$$ \dot{V} \le - \lambda_{1} \left\| {{\varvec{z}}_{1} } \right\|^{2} - \lambda_{2} \left\| {{\varvec{z}}_{2} } \right\|^{2} - k_{2m} \left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\|^{2} - \frac{{\varepsilon^{4} }}{{\hat{\delta }\left\| {{\varvec{z}}_{2} + \tilde{\varvec{\beta }}} \right\| + \varepsilon^{2} }} . $$

(65)

where $\lambda_{1}$ and $\lambda_{2}$ are positive constants.