Traveling wave solution and Painleve’ analysis of generalized fisher equation and diffusive Lotka–Volterra model

Abstract

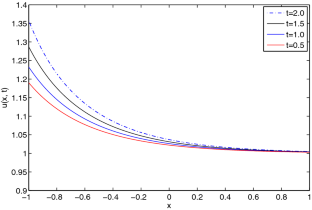
In this paper, we have obtained the traveling wave solution for generalized Fisher equation and Lotka–Volterra (L-V) model with diffusion using hyperbolic function method. The Painleve’ analysis has been used to check both of the system’s integrability. Obtained solutions have also been plotted to represent their spatio-temporal dependence. The three dimensional plot shows a monotonic profile of the solutions.

Appendices

Appendix-A

$$\begin{aligned} 3a_2^2b_2p+b_2^3p=0, \end{aligned}$$

(A.1)

$$\begin{aligned} 3a_2^2b_1p+6a_1a_2b_2p+3b_1b_2^2p=0, \end{aligned}$$

(A.2)

$$\begin{aligned}&a_2^2p-3a_0a_2^2p-6a_1a_2b_1p-3a_1^2b_2p+b_2^2p\nonumber \\&\quad -3a_0b_2^2p-3a_2^2b_2p-3b_1^2b_2p\nonumber \\&\quad -a_2^2pq-b_2^2pq+6b_2Dk^2=0, \end{aligned}$$

(A.3)

$$\begin{aligned}&2a_1a_2p-6a_0a_1a_2p-3a_1^2b_1p-3a_2^2b_1p-b_1^3p\nonumber \\&\quad -6a_1a_2b_2p+2b_1b_2p\nonumber \\&\quad -6a_0b_1b_2p-2a_1a_2pq-2b_1b_2pq+2b_1Dk^2\nonumber \\&\quad +2a_2k\lambda =0, \end{aligned}$$

(A.4)

$$\begin{aligned}&a_1^2p-3a_0a_1^2p+a_2^2p-3a_0a_2^2p-6a_1a_2b_1p+b_1^2p\nonumber \\&\quad +3a_0b_1^2p+2a_0b_2p-3a_0^2b_2p\nonumber \\&\quad -3a_1^2b_2p-a_1^2pq-a_2^2pq-b_1^2pq+b_2pq\nonumber \\&\quad -2a_0b_2pq+4b_2Dk^2+a_1k\lambda =0, \end{aligned}$$

(A.5)

$$\begin{aligned}&2a_1a_2p-6a_0a_1a_2p+2a_0b_1p+3a_0^2b_1p-3a_1^2b_1p\nonumber \\&\quad -2a_1a_2pq+b_1pq\nonumber \\&\quad -2a_0b_1pq+b_1Dk^2+a_2k\lambda =0, \end{aligned}$$

(A.6)

$$\begin{aligned} 2a_0a_1p-3a_0^2a_1p-a_1^3p+a_1pq-2a_0a_1pq=0, \end{aligned}$$

(A.7)

$$\begin{aligned}&2a_0a_2p-3a_0^2a_2p-3a_1^2a_2p+2a_1b_1p-6a_0a_1b_1p\nonumber \\&\quad +a_2pq-2a_0a_2pq\nonumber \\&\quad -2a_1b_1pq+a_2Dk^2+b_1k\lambda =0, \end{aligned}$$

(A.8)

$$\begin{aligned}&-a_1^3p-3a_1a_2^2+2a_2b_1p-6a_0a_2b_1p-3a_1b_1^2p\nonumber \\&\quad +2a_1b_2p-6a_0a_1b_2p\nonumber \\&\quad -2a_2b_1pq-2a_1b_2pq+2a_1Dk^2+2b_2k\lambda =0, \end{aligned}$$

(A.9)

$$\begin{aligned}&-3a_1^2a_2p-a_2^3p-3a_2b_1^2p+2a_2b_2p-6a_0a_2b_2p\nonumber \\&\quad -6a_1b_1b_2p-2a_2b_2pq+6a_2Dk^2=0, \end{aligned}$$

(A.10)

$$\begin{aligned} 3a_1a_2^2p+6a_2b_1b_2p+3a_1b_2^2p=0, \end{aligned}$$

(A.11)

$$\begin{aligned} a_2^3p+3a_2b_2^2p=0, \end{aligned}$$

(A.12)

$$\begin{aligned} a_0^2p-a_0^3p+a_1^2p-3a_0a_1^2p+a_0pq-a_0^2pq-a_1^2pq=0. \end{aligned}$$

(A.13)

Appendix-B

$$\begin{aligned} 6b_2Dk^2-b_2B_2-a_2A_2=0, \end{aligned}$$

(B.1)

$$\begin{aligned} 2b_1Dk^2+2a_2k\lambda -b_1B_2-a_1A_2-b_2B_1-a_2A_1=0, \end{aligned}$$

(B.2)

$$\begin{aligned} 4b_2Dk^2+a_1k\lambda -b_1B_1-a_1A_1+b_2-b_2A_0-a_2A_2-a_0B_2=0, \end{aligned}$$

(B.3)

$$\begin{aligned} b_1Dk^2+a_2k\lambda +b_1-b_1A_0-a_1A_2-a_2A_1-a_0B_1=0, \end{aligned}$$

(B.4)

$$\begin{aligned} a_2Dk^2+b_1k\lambda -b_1A_1-a_1B_1+a_2-a_2A_0-a_0A_2=0, \end{aligned}$$

(B.5)

$$\begin{aligned} 2a_1Dk^2+2b_2k\lambda -b_1A_2-a_1B_2-b_2A_1-a_2B_1=0, \end{aligned}$$

(B.6)

$$\begin{aligned} 6a_2Dk^2-b_2A_2-a_2B_2=0, \end{aligned}$$

(B.7)

$$\begin{aligned} a_1-a_1A_0-a_0A_1=0, \end{aligned}$$

(B.8)

$$\begin{aligned} a_0-a_0A_0-a_1A_1=0, \end{aligned}$$

(B.9)

$$\begin{aligned} 6B_2k^2+b_2B_2\rho +a_2A_2\rho =0, \end{aligned}$$

(B.10)

$$\begin{aligned} 2B_1k^2+2A_2k\lambda +b_2B_1\rho +b_1B_2\rho +a_2A_1\rho +a_1A_2\rho =0, \end{aligned}$$

(B.11)

$$\begin{aligned}&4B_2k^2+A_1k\lambda +\rho b_1B_1+a_0B_2\rho -B_2\rho +A_0b_2\rho \nonumber \\&\quad +a_1A_1\rho +a_2A_2\rho =0, \end{aligned}$$

(B.12)

$$\begin{aligned} B_1k^2+A_2k\lambda +a_0B_1\rho -B_1\rho +A_0b_1\rho +a_2A_1\rho +a_1A_2\rho =0, \end{aligned}$$

(B.13)

$$\begin{aligned} A_2k^2+B_1k\lambda +a_1B_1\rho +b_1A_1\rho +a_0A_2\rho -A_2\rho +A_0a_2\rho =0, \end{aligned}$$

(B.14)

$$\begin{aligned} 2A_1k^2+2B_2k\lambda +a_2B_1\rho +b_2A_1\rho +a_1B_2\rho +b_1A_2\rho =0, \end{aligned}$$

(B.15)

$$\begin{aligned} 6A_2k^2+a_2B_2\rho +b_2A_2\rho =0, \end{aligned}$$

(B.16)

$$\begin{aligned} a_0A_1\rho -A_1\rho +A_0a_1\rho =0, \end{aligned}$$

(B.17)

$$\begin{aligned} a_1A_1+a_0A_0-A_0=0. \end{aligned}$$

(B.18)