Stabilization principle in dynamics: a tutorial

Abstract

This paper introduces and illustrates the stabilization principle that provides a strategy for modeling post instability behavior in dynamics, including turbulence and chaos. It starts with investigation of different types of instability with the objective to demonstrate that stability is not a physical invariant since it depends upon the frame to which the motion is referred, upon the class of functions in which the governing equations are derived. The application of the stabilization principle to the Navier–Stokes equations is illustrated by closure of the Reynolds equations for the Poiseuille flow.

Appendix: Comments to Lagrangian turbulents

The Lagrangian turbulence is defined as postinstability motion of individualized trajectories of a fluid generated by a laminar flow. A Lagrangian description of turbulence (L-turbulence) has advantage over Eulerian description of turbulence (E-turbulence) in case of studies of mixing and dispersion. That includes mixing of passive scalars and the dispersion of contaminants.

The Lagrangian description of motion starts with the frame of reference that is frozen at the fluid and moves with it. Initially, at $t_{0} = 0$, such frame can be represented by Cartesian axes $X_{0}$, $\hbox {Y}_{0}$, and $\hbox {Z}_{0}$.

For t > 0, these axes, in general, transform into a non-orthogonal curvilinear system. Any individualized particle of the fluid with the coordinates x$_{0}$,y$_{0}$, and z$_{0}$ will have the same coordinates in the moving frame, but different coordinates in the initial frame

$$\begin{aligned} x= & {} x(x_{0} ,y_{0} ,z_{0} ,t) \end{aligned}$$

(104)

$$\begin{aligned} y= & {} y(x_{0} ,y_{0} ,z_{0} ,t)\end{aligned}$$

(105)

$$\begin{aligned} z= & {} z(x_{0} ,y_{0} ,z_{0} ,t) \end{aligned}$$

(106)

Equations (104)–(106) represent the Lagrangian description of a continuum, including fluid. These equations can be obtained as the solution of the governing equations of the fluid

$$\begin{aligned}&\frac{\partial u}{\partial t}+u\frac{\partial u}{\partial x}+v\frac{\partial u}{\partial y}+w\frac{\partial u{}}{\partial z}=F_x -\frac{1}{\uprho }\frac{\partial p}{\partial x}\end{aligned}$$

(107)

$$\begin{aligned}&\frac{\partial v}{\partial t}+u\frac{\partial v}{\partial x}+v\frac{\partial v}{\partial y}+w\frac{\partial v{}}{\partial z}=F_y -\frac{1}{\uprho }\frac{\partial p}{\partial x}\end{aligned}$$

(108)

$$\begin{aligned}&\frac{\partial w}{\partial t}+u\frac{\partial w}{\partial x}+v\frac{\partial w}{\partial y}+w\frac{\partial w{}}{\partial z}=F_z -\frac{1}{\uprho }\frac{\partial p}{\partial x}\end{aligned}$$

(109)

$$\begin{aligned}&\frac{\partial }{\partial x}u+\frac{\partial }{\partial y}v+\frac{\partial }{\partial z}w=0, \end{aligned}$$

(110)

presented in the Lagrangian form

$$\begin{aligned}&\left( F_x -\frac{\partial ^{2}x}{\partial t^{2}}\right) \frac{\partial x}{\partial x_{0} }+\left( F_y -\frac{\partial ^{2}y}{\partial t^{2}}\right) \frac{\partial y}{\partial x_{0} }\nonumber \\&\quad +\left( F_z -\frac{\partial ^{2}z}{\partial t^{2}}\right) \frac{\partial z}{\partial x_{0} } =\frac{1}{\uprho }\frac{\partial p}{\partial x_{0} }\end{aligned}$$

(111)

$$\begin{aligned}&\left( F_x -\frac{\partial ^{2}x}{\partial t^{2}}\right) \frac{\partial x}{\partial y_{0} }+\left( F_y -\frac{\partial ^{2}y}{\partial t^{2}}\right) \frac{\partial y}{\partial y_{0} }\nonumber \\&\quad +\left( F_z -\frac{\partial ^{2}z}{\partial t^{2}}\right) \frac{\partial z}{\partial y_{0} } =\frac{1}{\uprho }\frac{\partial p}{\partial y_{0} }\end{aligned}$$

(112)

$$\begin{aligned}&\left( F_x -\frac{\partial ^{2}x}{\partial t^{2}}\right) \frac{\partial x}{\partial z_{0} }+\left( F_y -\frac{\partial ^{2}y}{\partial t^{2}}\right) \frac{\partial y}{\partial z_{0} }\nonumber \\&\quad +\left( F_z -\frac{\partial ^{2}z}{\partial t^{2}}\right) \frac{\partial z}{\partial z_{0} } =\frac{1}{\uprho }\frac{\partial p}{\partial z_{0} }\end{aligned}$$

(113)

$$\begin{aligned}&\;\left| {\begin{array}{l} \frac{\partial x}{\partial x_{0} }\;\frac{\partial y}{\partial x_{0} }\;\frac{\partial z}{\partial z_{0} } \\ \frac{\partial x}{\partial y_{0} }{}{}{}\frac{\partial y}{\partial y_{0} }{}\;{}{}\frac{\partial z}{\partial y_{0} } \\ \frac{\partial x}{\partial z_{0} }\;\frac{\partial y}{\partial z_{0} }\;\;\frac{\partial z}{\partial z_{0} } \\ \end{array}} \right| =1 \end{aligned}$$

(114)

This is a system of four PDE with respect to four unknowns: x,y,z and p as functions of $\hbox {x}_{0}, \hbox { y}_{0,}\hbox { z}_{0}$ and t.

However if the Euler’s equations (107)–(110) are already solved in the form

$$\begin{aligned} u= & {} u(x,y,z,t) \nonumber \\ v= & {} v(x,y,z,t) \nonumber \\ w= & {} w(x,y,z,t) \end{aligned}$$

(115)

then the Lagrangian description of the same motion reduces to three kinematical ODE

$$\begin{aligned} \frac{{ dx}}{{ dt}}= & {} u(x,y,z,t) \nonumber \\ \frac{{ dy}}{{ dt}}= & {} v(x,y,z,t) \nonumber \\ \frac{{ dz}}{{ dt}}= & {} w(x,y,z,t) \end{aligned}$$

(116)

to be solved subject to the following initial conditions

$$\begin{aligned} x=x_{0} ,\quad y=y_{0} ,\quad z=z_{0} \quad at\quad t=0 \end{aligned}$$

(117)

The solution of this system can be written in the form (104)–(106).

It should be emphasized again that Eq. (116) are kinematical, and they are valid not only for the Euler incompressible fluid, but for a viscose compressible fluid as well. The only difference is in the form in which the velocities as known functions of the coordinates are presented in Eqs. (116).

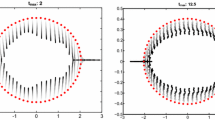
Comparing the Eulerian and Lagrangian descriptions of fluid motion, one sees that Lagrangian description requires an additional system of differential equations (116), and that can change the condition of stability of the solution (116) even if the solutions (115) are stable. Indeed the chaos in the systems of the first order ODE can appear when $n\ge 3$,where n is the number of equations. Since $n =3$ in Eq. (116), a laminar flow of a fluid can have chaotic trajectories, and that we will call Lagrangian turbulence.

The first theoretical example of Lagrangian turbulence was introduced by Arnold [7] who proved that an inviscid stationary flow with a smooth velocity field

$$\begin{aligned} u= & {} A\sin z+C\cos y, \quad v=B\sin x+A\cos z, \nonumber \\ w= & {} C\sin y+b\cos x \end{aligned}$$

(118)

has chaotic trajectories that represent Lagrangian turbulence. It means that this flow is stable in the Eulerian coordinates, but is unstable in the Lagrangian coordinates. Actually this result is another evidence of the statement made in Sect. 2 that stability is not a physical invariant: it depends upon the frame of reference.