Abstract
This work analyzes the use of Equivalent Polynomials (EqP) in the context of the eXtended Finite Element Method (XFEM). Special attention is devoted to the modeling of weak interfaces, here studied as bi-material interfaces, in the framework of the weak form of elastostatic boundary value problems. The well-known pathological oscillations due to the loss of partition of unity in blending elements are also treated by using a local hierarchical enrichment of the approximation space. In order to assess the properties of the studied approximation spaces, some key examples are selected, for which numerical result comparisons are provided by allowing the standard form to also represent the interface. Conventional numerical integration techniques perform the integration of the weak form: the standard Gauss–Legendre quadrature method and the sub-elements (or integration cells, subcells) conformed to the interface. The EqP version of the XFEM investigated here takes advantage of the standard Gauss–Legendre quadrature method and can reproduce the convergence rates of the integration technique with sub-elements, especially for regular meshes. It is an alternative for modeling static or mobile interfaces where the use of the sub-elementation technique presents issues.






















Similar content being viewed by others
Notes
The term “conform” is employed to assign whether the interface was considered during the meshing process. It does not refer to finite element spaces conformity or the presence of hanging nodes.
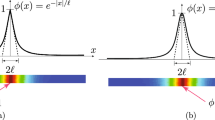
For a more details see Ventura [25], pages 775 and 776, and the subsequent development for each element.
References
Belytschko T, Black T (1999) Elastic crack growth in finite elements with minimal remeshing. Int J Numer Methods Eng 45(5):601–620. https://doi.org/10.1002/(SICI)1097-0207(19990620)45:5<601::AID-NME598>3.0.CO;2-S
Belytschko T, Parimi C, Moës N et al (2003) Structured extended finite element methods for solids defined by implicit surfaces. Int J Numer Methods Eng 56(4):609–635. https://doi.org/10.1002/nme.686
Belytschko T, Gracie R, Ventura G (2009) A review of extended/generalized finite element methods for material modeling. Modell Simul Mater Sci Eng 17(4):1–24. https://doi.org/10.1088/0965-0393/17/4/043001
Bordas SPA, Natarajan S, Kerfriden P et al (2011) On the performance of strain smoothing for quadratic and enriched finite element approximations (xfem/gfem/pufem). Int J Numer Methods Eng 86(4–5):637–666. https://doi.org/10.1002/nme.3156
Chessa J, Wang H, Belytschko T (2003) On the construction of blending elements for local partition of unity enriched finite elements. Int J Numer Methods Eng 57(7):1015–1038. https://doi.org/10.1002/nme.777
Fries TP (2008) A corrected XFEM approximation without problems in blending elements. Int J Numer Methods Eng 75(5):503–532. https://doi.org/10.1002/nme.2259
Fries TP (2008) A corrected XFEM approximation without problems in blending elements. Int J Numer Methods Eng 75(5):503–532. https://doi.org/10.1002/nme.2259
Fries TP, Belytschko T (2006) The intrinsic XFEM: a method for arbitrary discontinuities without additional unknowns. Int J Numer Methods Eng 68(13):1358–1385. https://doi.org/10.1002/nme.1761
Fries TP, Belytschko T (2010) The extended/generalized finite element method: an overview of the method and its applications. Int J Numer Methods Eng 84(3):253–304. https://doi.org/10.1002/nme.2914
Gracie R, Wang H, Belytschko T (2008) Blending in the extended finite element method by discontinuous Galerkin and assumed strain methods. Int J Numer Methods Eng 74(11):1645–1669. https://doi.org/10.1002/nme.2217
Kästner M, Müller S, Goldmann J et al (2013) Higher-order extended fem for weak discontinuities-level set representation, quadrature and application to magneto-mechanical problems. Int J Numer Methods Eng 93(13):1403–1424. https://doi.org/10.1002/nme.4435
Khoei AR (2015) Extended finite element method: theory and applications. Wiley series in computational mechanics. Wiley, New York. https://doi.org/10.1002/9781118869673
Lim I, Johnston I, Choi S et al (1992) An improved numerical inverse isoparametric mapping technique for 2d mesh rezoning. Eng Fract Mech 41(3):417–435. https://doi.org/10.1016/0013-7944(92)90082-P
Mariggiò G, Fichera S, Corrado M et al (2020) Eqp—a 2d/3d library for integration of polynomials times step function. SoftwareX 12(100):636. https://doi.org/10.1016/j.softx.2020.100636
Moës N, Dolbow J, Belytschko T (1999) A finite element method for crack growth without remeshing. Int J Numer Methods Eng 46(1):131–150. https://doi.org/10.1002/(SICI)1097-0207(19990910)46:1<131::AID-NME726>3.0.CO;2-J
Moës N, Cloirec M, Cartraud P et al (2003) A computational approach to handle complex microstructure geometries. Comput Methods Appl Mech Eng 192(28–30):3163–3177. https://doi.org/10.1016/S0045-7825(03)00346-3
Murti V, Valliappan S (1986) Numerical inverse isoparametric mapping in remeshing and nodal quantity contouring. Comput Struct 22(6):1011–1021. https://doi.org/10.1016/0045-7949(86)90161-6
Natarajan S, Mahapatra DR, Bordas SPA (2010) Integrating strong and weak discontinuities without integration subcells and example applications in an XFEM/GFEM framework. Int J Numer Methods Eng 83(3):269–294. https://doi.org/10.1002/nme.2798
Perić D, Vaz M, Owen D (1999) On adaptive strategies for large deformations of elasto-plastic solids at finite strains: computational issues and industrial applications. Comput Methods Appl Mech Eng 176(1):279–312. https://doi.org/10.1016/S0045-7825(98)00342-9
Seabra MRR, de Sa JMAC, Šuštarič P et al (2012) Some numerical issues on the use of XFEM for ductile fracture. Comput Mech 50:611–629. https://doi.org/10.1007/s00466-012-0694-x
Strouboulis T, Babuška I, Copps K (2000) The design and analysis of the generalized finite element method. Comput Methods Appl Mech Eng 181(1):43–69. https://doi.org/10.1016/S0045-7825(99)00072-9
Sukumar N, Chopp D, Moës N et al (2001) Modeling holes and inclusions by level sets in the extended finite element method. Comput Methods Appl Mech Eng 190(46):6183–6200. https://doi.org/10.1016/S0045-7825(01)00215-8
Tarancón JE, Vercher A, Giner E et al (2009) Enhanced blending elements for XFEM applied to linear elastic fracture mechanics. Int J Numer Methods Eng 77(1):126–148. https://doi.org/10.1002/nme.2402
Tavares JMA (2021) Análise de métodos de integração numérica para problemas com descontinuidade fraca no XFEM. Master thesis, UFRGS, sabi.001127211
Ventura G (2006) On the elimination of quadrature subcells for discontinuous functions in the extended finite element method. Int J Numer Methods Eng 66(5):761–795. https://doi.org/10.1002/nme.1570
Ventura G, Benvenuti E (2015) Equivalent polynomials for quadrature in heaviside function enriched elements. Int J Numer Methods Eng 102(3–4):688–710. https://doi.org/10.1002/nme.4679
Wan D, Hu D, Natarajan S et al (2017) A fully smoothed XFEM for analysis of axisymmetric problems with weak discontinuities. Int J Numer Methods Eng 110(3):203–226. https://doi.org/10.1002/nme.5352
Wang M, Liu Y (2010) Analytical solution for bi-material beam with graded intermediate layer. Compos Struct 92(10):2358–2368. https://doi.org/10.1016/j.compstruct.2010.03.013
Acknowledgements
The authors wish to acknowledge the support of CNPq, Conselho Nacional de Desenvolvimento Científico e Tecnológico of Brazil, Grant Number 309430/2021-6, and of FAPERGS, Fundação de Amparo à Pesquisa do Estado do Rio Grande do Sul, Grant Number 19/2551-0001954-8-1.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
Not applicable
Additional information
Technical Editor: Aurelio Araujo.
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
A Numerical integration using sub-elements
The triangulation of elements crossed by a discontinuity is one of the most used strategies in the XFEM numerical integration. It consists of dividing the regions of a element crossed by interface into triangles and applying a numerical integration procedure, like the Gauss–Legendre procedure, within each sub-element. The triangular sub-elements are easily obtained for convex regions using the Delaunay triangulation.
Given an element with a crossing interface, what is the case of interest, the division into triangular sub-elements was given by the Delaunay triangulation, where each sub-triangle coordinate vertex at the Cartesian plane \(\left( x,y\right)\) and the integration points, say the Gauss–Legendre integration quadrature, in the parametric system \(\left( r,s\right)\) are known, so the coordinates of the integration points can be easily determined in the Cartesian plane by the use of the shape functions of the triangular element, Eqs. 55 and 56:
Figure 23 shows an element with triangular sub-elements and the coordinates transformation.
Once the integration points in the Cartesian plane were known, it was necessary to transform them for the quadrilateral element’s parametric system \(\left( \xi ,\eta \right)\). The mapping from local coordinates \(\left( \xi ,\eta \right)\) to the Cartesian plane\(\left( x,y\right)\) is performed by
The inverse of this mapping, \(\left( x,y\right) \rightarrow \left( \xi ,\eta \right)\), is not simple because it involves a nonlinear system of equations that cannot be solved analytically, so a numerical approximation is necessary. There are several ways to solve this problem. In this paper, we have implemented the method proposed by Murti and Valliappan[17].
In Murti and Valliappan [17], the iteration procedure to determine the inverse mapping has been improved by the intersection of a defined line segment that passes through a point M, defined at the interior of the element, whose Cartesian coordinates are \(\varvec{x}_{M}\) and a point P whose the local parametric coordinate, \(\xi _{p}\) is known, such that a node of the vertex of the element.
For this characterization, the point M is assumed to be an integration point that needs to be determined in terms of the parametric coordinates, \(\xi _{m}\), corresponding to the point \(M'\), see Fig. 24.
The segment \(\overline{PQ}\) in the Cartesian plane can be easily determined by the linear equation of the line passing by the points P and M. This segment is associated with the curve \(P'Q'\) on the local coordinate plane and passing through the point \(M'\). Thus, the coordinate of the point \(M'\), \(\xi _{m}\), can be determined more efficiently when compared to a numerical method without any improvement condition.
The false position method was employed, Lim et al. [13], to determine the point \(M'\) on the curve \(P'Q'\). In Murti and Valliappan [17], the authors used the bisection method, but the number of iterations for this method was higher.
The point P cannot be any vertex of the element. It must be chosen so that the curve \(P'Q'\) be defined in the element; that is, it must cover the entire axis \(\eta\) at the interval \([-1,1]\). In this paper, when this occurs, the point P is redefined as the vertex opposite to the previous one, while in Murti and Valliappan [17], the authors choose to renumber the element nodes, so there is a transformation of the axis \(\eta\) to \(\xi\).
Once the integration points are obtained on the parametric system \(\left( \xi ,\eta \right)\) the stiffness matrix can be integrated into each triangular sub-element using:
where, n is the number of integration points, \(w_{i}\) is the weight. The matrices \(\textbf{B}\) and \(\textbf{E}\) depend on the variables \(\eta\) and \(\xi\). They are integrated at the points \(\left( \xi _{i},\eta _{i}\right)\), the integration points of the triangular sub-element transformed into this coordinate system.
The element stiffness matrix \(\textbf{K}_{e}\) is the summation of the sub-elements stiffness matrices \(\textbf{K}_{\text {sub-e}}\), that is,
where nk is the number of sub-elements in the element \(\Omega _{e}\).
B Coefficients for the equivalent polynomials
The coefficients of the polynomial \(\tilde{H}\) are the same presented by Ventura [25] and will not be presented in this paper. However, the coefficients for the equivalent polynomial \(\tilde{Q}\) are different, see also [24]. When the interface intercepts adjacent sides of the element, the coefficients to the polynomial \(\tilde{Q}\), are:
And when the interface intercepted opposite sides of the element, the coefficients to the polynomial \(\tilde{Q}\), are
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
da Rosa Rodriguez, E., Rossi, R. Assessment of EqP in XFEM for weak discontinuities. J Braz. Soc. Mech. Sci. Eng. 45, 312 (2023). https://doi.org/10.1007/s40430-023-04211-z
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s40430-023-04211-z