Force on a spherical particle oscillating in a viscous fluid perpendicular to an impermeable planar wall

Abstract

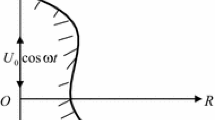
The slow motion of a hard spherical particle embedded in a semi-infinite viscous fluid bounded by an impermeable plane wall is considered. The particle oscillates with small amplitude along a diameter perpendicular to the wall. At both surfaces of the particle and plane wall, the no-slip kinematic condition is used. An analytical procedure with a numerical solution based on collocation technique is considered. The solution is found to be accurate for the low and high frequency of oscillations. The drag force coefficients acting on the particle are plotted and tabulated against the frequency and the separation distance. The drag coefficients are found in good agreement with the corresponding problem of a steady case and with the oscillation of a particle embedded in an infinite viscous fluid.

Appendix

The functions in Eqs. (60) and (61) are defined as

$$ A_{1n} (r,\theta ) = - (n + 1)r^{ - n - 1} \,G_{n + 1} \left( {\cos \theta } \right)\csc \theta , $$

(56)

$$ B_{1n} (r,\theta ) = - r^{{ - \tfrac{3}{2}}} \left( {\alpha r\cos \theta K_{{n - \tfrac{3}{2}}} (\alpha r)G_{n} \left( {\cos \theta } \right) + (n + 1)\,K_{{n - \tfrac{1}{2}}} (\alpha r)G_{n + 1} \left( {\cos \theta } \right)} \right)\csc \theta , $$

(57)

$$ A_{2n} (r,\theta ) = - r^{ - n - 1} P_{n} (\cos \theta ), $$

(58)

$$ B_{2n} (r,\theta ) = r^{{ - \tfrac{3}{2}}} \left[ {\alpha rK_{{n - \tfrac{3}{2}}} (\alpha r)G_{n} (\cos \theta )} \right.\left. { - K_{{n - \tfrac{1}{2}}} P_{n} (\cos \theta )} \right], $$

(59)

where $P_{n} (.)$ is the Legendre Polynomial of order n.

The functions in Eqs. (68) and (69) are defined as

$$ e_{1n} (\tau , - \sigma ) = - \int\limits_{0}^{\infty } t A_{1n} (t, - \sigma )J_{1} (t\tau )\,{\text{d}}t, $$

(60)

$$ f_{1n} (\tau , - \sigma ) = - \int\limits_{0}^{\infty } t B_{1n} (t, - \sigma )J_{1} (t\tau )\,{\text{d}}t, $$

(61)

$$ e_{2n} (\tau , - \sigma ) = - \int\limits_{0}^{\infty } t A_{2n} (t, - \sigma )J_{0} (t\tau )\,dt, $$

(62)

$$ f_{2n} (\tau , - \sigma ) = - \int\limits_{0}^{\infty } t \,B_{2n} (t, - \sigma )J_{0} (t\tau )\,{\text{d}}t. $$

(63)

The integrals required in (60)–(63) are performed analytically as follows: Using the polynomial representations of the Gegenbauer and Legendre functions together with the result given by Erdelyi et al. [32]:

$$ \begin{aligned} \int\limits_{0}^{\infty } {\frac{{x^{{\nu + \tfrac{1}{2}}} }}{{(x^{2} + a^{2} )^{\mu + 1} }}\,J_{\nu } (xy)(xy)^{{\tfrac{1}{2}}} \,{\text{d}}x} & = \frac{{a^{\nu - \mu } y^{{\mu + \tfrac{1}{2}}} }}{{2^{\mu } \varGamma (\mu + 1)}}K_{\nu - \mu } (ay), \\ & \quad \text{Re} \,a > 0,\,y > 0,\quad - 1 < \text{Re} \nu < 2\text{Re} \mu + 1, \\ \end{aligned} $$

$$ \begin{aligned} & \int\limits_{0}^{\infty } {\frac{{x^{{\nu + \tfrac{1}{2}}} K_{\mu } (a(x^{2} + y^{2} )^{{\tfrac{1}{2}}} )}}{{(x^{2} + \beta^{2} )^{{\tfrac{\mu }{2}}} }}J_{\nu } (xy)(xy)^{{\tfrac{1}{2}}} {\text{d}}x} \\ & \quad = \frac{{\beta^{\nu + 1 - \mu } y^{{\nu + \tfrac{1}{2}}} }}{{a^{\mu } }}(y^{2} + a^{2} )^{{\tfrac{\mu - \nu - 1}{2}}} K_{\mu - \nu - 1} (\beta (y^{2} + a^{2} )^{{\tfrac{1}{2}}} ),\quad \text{Re} \,a > 0,\,\,\text{Re} \beta > 0, \\ \end{aligned} $$

where $K_{\nu }$ is the modified Bessel function of the second kind, one can show by induction that

$$ e_{1n} (\tau , - \sigma ) = ( - 1)^{n - 1} \frac{{\tau^{n - 1} }}{n!}e^{ - \sigma \tau } , $$

(64)

$$ f_{1n} (\tau , - \sigma ) = ( - 1)^{n} \frac{{\tau^{n - 1} }}{n!}e^{ - \sigma \tau } , $$

(65)

$$ e_{2n} (\tau , - \sigma ) = ( - 1)^{n} \sqrt {\frac{\pi \alpha }{{2\tau^{2} }}} \,\,e^{ - \sigma \,\xi } G_{n} \left( {\frac{\xi }{\alpha }} \right), $$

(66)

$$ f_{2n} (\tau , - \sigma ) = ( - 1)^{n - 1} \sqrt {\frac{\pi \alpha }{{2\xi^{2} }}} \,\,e^{ - \sigma \,\xi } G_{n} \left( {\frac{\xi }{\alpha }} \right). $$

(67)

The functions in Eqs. (20) and (21) are defined as

$$ \left[ \begin{aligned} a_{1n} (r,\theta ) \hfill \\ b_{1n} (r,\theta ) \hfill \\ \end{aligned} \right] = \int\limits_{0}^{\infty } {\tau \left\{ {H_{1} \left[ \begin{aligned} e_{1n} (\tau , - \sigma ) \hfill \\ f_{1n} (\tau , - \sigma ) \hfill \\ \end{aligned} \right] + H_{2} \left[ \begin{aligned} e_{2n} (\tau , - \sigma ) \hfill \\ f_{2n} (\tau , - \sigma ) \hfill \\ \end{aligned} \right]} \right\}\,\,J_{1} (\tau r\sin \theta ){\text{d}}\tau } , $$

(68)

$$ \left[ \begin{aligned} a_{2n} (r,\theta ) \hfill \\ b_{2n} (r,\theta ) \hfill \\ \end{aligned} \right] = \int\limits_{0}^{\infty } {\tau \left\{ {\,\,H_{3} \left[ \begin{aligned} e_{1n} (\tau , - \sigma ) \hfill \\ f_{1n} (\tau , - \sigma ) \hfill \\ \end{aligned} \right] + H_{4} \left[ \begin{aligned} e_{2n} (\tau , - \sigma ) \hfill \\ f_{2n} (\tau , - \sigma ) \hfill \\ \end{aligned} \right]} \right\}J_{0} (\tau r\sin \theta )\,\,{\text{d}}\tau } , $$

(69)

where

$$ H_{1} = \left( {\tau - \xi } \right)^{ - 1} \left( {\tau e^{{ - \tau \tilde{z}}} - \xi e^{{ - \xi \tilde{z}}} } \right), $$

(70)

$$ H_{2} = \xi \left( {\tau - \xi } \right)^{ - 1} \left( {e^{{ - \xi \tilde{z}}} - e^{{ - \tau \tilde{z}}} } \right), $$

(71)

$$ H_{3} = \tau \left( {\tau - \xi } \right)^{ - 1} \left( {e^{{ - \tau \tilde{z}}} - \,e^{{ - \xi \tilde{z}}} } \right), $$

(72)

$$ H_{4} = \left( {\tau - \xi } \right)^{ - 1} \left( {\tau e^{{ - \xi \tilde{z}}} - \xi e^{{ - \tau \tilde{z}}} } \right). $$

(73)