Nonlinear aeroelastic analysis of high-aspect-ratio wings using indicial aerodynamics

Abstract

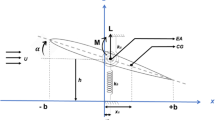
A new approach for calculating the unsteady aerodynamic loads based upon the indicial functions concept in combination with a fully third-order nonlinear structural model has been developed to analyze the aeroelastic behavior of high-aspect-ratio wings over the entire range of subsonic flow. The resulting aeroelastic equations including all structural geometric nonlinearities associated with large deformations and mass distributions, along with nonlinear terms due to mass imbalance at wing’s cross section, are then rewritten in the state-space form, introducing an efficient and appropriate approach to use in both eigenvalue and time response analysis. To validate the developed aeroelastic equations, the linear and nonlinear aeroelastic behaviors of a specified wing are compared with those presented for an incompressible aerodynamic case. Quantitative and qualitative agreement between the present results and available ones confirms the unsteady indicial aerodynamics, nonlinear structural modeling, and, consequently, the developed nonlinear aeroelastic model. By changing the wing model and applying the unsteady compressible aerodynamic loads, the nonlinear aeroelastic behavior of Goland wing is then investigated, including the flutter boundary, limit cycle oscillations, pre-flutter, flutter, and post-flutter time responses, phase plane diagrams, and also the effect of flight conditions such as altitude and air speed on the aeroelastic behavior. The results represent the necessity of applying appropriate Mach-dependent aerodynamic loads to provide reasonable description of the aeroelastic analysis in the compressible flight speed regime.

Appendix

The nonlinear terms that have been defined as $G_{v}$ and $G_{\alpha }$, and elements of structural and aerodynamic matrices are given in this section. Note that $D_{\xi }$, $D_{\eta }$, and $D_{\zeta }$ are torsional, out-of-plane, and in-plane rigidity, respectively, $j_{\xi }$, $j_{\eta }$, and $j_{\zeta }$ denote moment of inertia per length about the axes of the deformed coordinate system. Moreover, $m$ and $n$ denote the number of the out-of-plane and torsional modes, respectively.

$$ \begin{aligned} G_{v} = & - D_{\zeta } \left[ {v^{{\prime }} \left( {v^{{\prime \prime }} v^{{\prime }} } \right)^{{\prime }} } \right]^{{\prime }} - \left( {D_{\eta } - D_{\zeta } } \right)\left( {v^{{\prime \prime }} \alpha^{2} } \right)^{{\prime \prime }} - \frac{1}{2}m\left[ {v^{{\prime }} \mathop \int \limits_{L}^{s} \frac{{\partial^{2} }}{{\partial t^{2} }}\left( {\mathop \int \limits_{0}^{s} v^{{{\prime }2}} {\text{d}}s} \right){\text{d}}s} \right]^{{\prime }} + \left( {j_{\eta } - j_{\zeta } } \right)\left[ {\frac{\partial }{\partial t}\left( {\dot{v}^{{\prime }} \alpha^{2} } \right)} \right]^{{\prime }} + \left[ {j_{\zeta } v^{{\prime }} \frac{\partial }{\partial t}\left( {v^{{\prime }} \dot{v}^{{\prime }} } \right)} \right]^{{\prime }} \\ \quad + me_{z} \left[ {v^{\prime } \frac{{\partial^{2} }}{{\partial t^{2} }}\left( {\mathop \int \limits_{L}^{s} v^{\prime}\alpha \,{\text{d}}s} \right)} \right]^{'} + me_{z} \left\{ {\alpha \left[ { - \frac{1}{2}\frac{{\partial^{2} }}{{\partial t^{2} }}\left( {\mathop \int \limits_{0}^{s} v^{\prime 2} \,{\text{d}}s} \right) + v^{\prime}\ddot{v}} \right]} \right\}^{\prime } - \frac{1}{2}me_{z} \frac{{\partial^{2} }}{{\partial t^{2} }}\left( {v^{\prime 2} \alpha + \frac{1}{3}\alpha^{3} } \right) + j_{\zeta } \ddot{v}^{\prime \prime } \\ \end{aligned} $$

$$ G_{\alpha } = \left( {D_{\zeta } - D_{\eta } } \right)v^{{{\prime \prime }2}} \alpha + \left( {j_{\eta } - j_{\zeta } } \right)\dot{v}^{{{\prime }2}} \alpha + me_{z} \left[ {\frac{1}{2}v^{{\prime }} \frac{{\partial^{2} }}{{\partial t^{2} }}\left( {\mathop \int \limits_{0}^{s} v^{{{\prime }2}} {\text{d}}s} \right) - \frac{1}{2}\left( {v^{{{\prime }2}} + \alpha^{2} } \right)\ddot{v}} \right] $$

$$ \begin{array}{*{20}l} {M_{i,j} = \mathop \int \limits_{0}^{1} V_{i} \left( s \right)V_{j} \left( s \right){\text{d}}s = \delta_{ij} } \hfill & {1 \le i \le m, 1 \le j \le m} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {M_{i,m + j} = - e\mathop \int \limits_{0}^{1} V_{i} \left( s \right)A_{j} \left( s \right){\text{d}}s,} \hfill & {i \le i \le m, 1 \le j \le n} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {M_{m + i,j} = - e\mathop \int \limits_{0}^{1} A_{i} \left( s \right)V_{j} \left( s \right){\text{d}}s,} \hfill & {1 \le i \le n, 1 \le j \le m} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {M_{m + i,m + j} = j_{1} \mathop \int \limits_{0}^{1} A_{i} \left( s \right)A_{j} \left( s \right){\text{d}}s = j_{1} \delta_{ij} } \hfill & {1 \le i \le n, 1 \le j \le n} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {K_{i,j} = \beta_{z} \mathop \int \limits_{0}^{1} V_{i} \left( s \right)V_{j}^{iv} \left( s \right){\text{d}}s = \beta_{z} z_{j}^{4} \delta_{ij} } \hfill & {1 \le i \le m, 1 \le j \le m} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {K_{m + i,m + j} = - \beta_{y} \mathop \int \limits_{0}^{1} A_{i} \left( s \right)A_{j}^{''} \left( s \right){\text{d}}s = \beta_{y} \gamma_{j}^{2} \delta_{ij} , \quad {\text{where}}\, \gamma_{j} \, is\, a\, {\text{root}} \,{\text{of}}\,\sin \left( {\gamma_{j} } \right) = 0} \hfill & {1 \le i \le n, 1 \le j \le n} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {C_{i,j} = c_{{v_{i} }} \mathop \int \limits_{0}^{1} V_{i} \left( s \right)V_{j} \left( s \right){\text{d}}s = c_{{v_{i} }} \delta_{ij} } \hfill & {1 \le i \le m, 1 \le j \le n} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {C_{m + i,m + j} = c_{{\alpha_{i} }} \mathop \int \limits_{0}^{1} A_{i} \left( s \right)A_{j} \left( s \right){\text{d}}s = c_{{\alpha_{i} }} \delta_{ij} } \hfill & {1 \le i \le n, 1 \le j \le n} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {M_{i,j}^{nl} = j_{3} \mathop \int \limits_{0}^{1} V_{i} V_{j}^{{\prime \prime }} \, {\text{d}}s - \mathop \sum \limits_{p = 1}^{m} \mathop \sum \limits_{q = 1}^{m} \mathop \int \limits_{0}^{1} V_{i} \left( {V_{p}^{{\prime }} \mathop \int \limits_{1}^{s} \mathop \int \limits_{0}^{s} V_{j}^{{\prime }} V_{q}^{{\prime }} \,{\text{d}}s{\text{d}}s} \right)^{'} {\text{d}}s\,v_{p} v_{q} - e_{z} \mathop \sum \limits_{p = 1}^{m} \mathop \sum \limits_{k = 1}^{n} \mathop \int \limits_{0}^{1} V_{i} \left[ {\left( {{\text{A}}_{k} \mathop \int \limits_{0}^{s} V_{j}^{{\prime }} V_{p}^{{\prime }} \,{\text{d}}s - {\text{A}}_{k} V_{p}^{'} V_{j} - V_{p}^{{\prime }} \mathop \int \limits_{1}^{s} V_{j}^{{\prime }} {\text{A}}_{k} \,{\text{d}}s} \right)^{{\prime }} + V_{j}^{{\prime }} V_{p}^{{\prime }} {\text{A}}_{k} } \right] {\text{d}}s\,v_{p} \alpha_{k} } \hfill & {1 \le i \le m, 1 \le j \le m} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {M_{i,m + j}^{nl} = \frac{1}{2}e_{z} \mathop \sum \limits_{p = 1}^{m} \mathop \sum \limits_{q = 1}^{m} \mathop \int \limits_{0}^{1} \left[ {2V_{i} \left( {V_{p}^{{\prime }} \mathop \int \limits_{1}^{s} V_{q}^{{\prime }} {\text{A}}_{j} \,{\text{d}}s} \right)^{{\prime }} - V_{i} V_{p}^{{\prime }} V_{q}^{{\prime }} {\text{A}}_{j} } \right] {\text{d}}s\,v_{p} v_{q} - \frac{1}{2}e_{z} \mathop \sum \limits_{p = 1}^{n} \mathop \sum \limits_{q = 1}^{n} \mathop \int \limits_{0}^{1} V_{i} {\text{A}}_{j} {\text{A}}_{p} {\text{A}}_{q} \,{\text{d}}s\alpha_{p} \alpha_{q} } \hfill & {1 \le i \le m, 1 \le j \le n} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {M_{m + i,j}^{nl} = \frac{1}{2}e_{z} \mathop \sum \limits_{p = 1}^{m} \mathop \sum \limits_{q = 1}^{m} \mathop \int \limits_{0}^{1} A_{i} V_{p}^{{\prime }} \left[ {2\mathop \int \limits_{0}^{s} V_{j}^{{\prime }} V_{q}^{{\prime }} \,{\text{d}}s - V_{q}^{{\prime }} V_{j} } \right]{\text{d}}s\,v_{p} v_{q} \ddot{v}_{j} - \frac{1}{2}e_{z} \mathop \sum \limits_{k = 1}^{n} \mathop \sum \limits_{p = 1}^{n} \mathop \int \limits_{0}^{1} A_{i} A_{k} A_{p} V_{j} {\text{d}}s\,\alpha_{k} \alpha_{p} } \hfill & {1 \le i \le n, 1 \le j \le m} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {C_{i,j}^{nl} = - \mathop \sum \limits_{p = 1}^{m} \mathop \sum \limits_{q = 1}^{m} \mathop \int \limits_{0}^{1} V_{i} \left( {V_{p}^{'} \mathop \int \limits_{1}^{s} \mathop \int \limits_{0}^{s} V_{j}^{'} V_{q}^{'} \,{\text{d}}s\,{\text{d}}s} \right)^{'} {\text{d}}sv_{p} \dot{v}_{q} - e_{z} \mathop \sum \limits_{p = 1}^{m} \mathop \sum \limits_{k = 1}^{n} \mathop \int \limits_{0}^{1} \left[ {V_{i} \left( {{\text{A}}_{k} \mathop \int \limits_{0}^{s} V_{j}^{{\prime }} V_{p}^{{\prime }} {\text{d}}s} \right)^{{\prime }} + V_{i} V_{j}^{{\prime }} V_{p}^{{\prime }} {\text{A}}_{k} } \right] {\text{d}}s\,\dot{v}_{p} \alpha_{k} \, + 2e_{z} \mathop \sum \limits_{p = 1}^{m} \mathop \sum \limits_{k = 1}^{n} \mathop \int \limits_{0}^{1} \left[ {V_{i} \left( {V_{p}^{{\prime }} \mathop \int \limits_{1}^{s} V_{j}^{{\prime }} {\text{A}}_{k} \,{\text{d}}s} \right)^{{\prime }} - V_{i} V_{j}^{{\prime }} V_{p}^{{\prime }} {\text{A}}_{k} } \right] {\text{d}}s\,v_{p} \dot{\alpha }_{k} } \hfill & {1 \le i \le m, 1 \le j \le m} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {C_{i,m + j}^{nl} = - e_{z} \mathop \sum \limits_{p = 1}^{n} \mathop \sum \limits_{q = 1}^{n} \mathop \int \limits_{0}^{1} V_{i} {\text{A}}_{j} {\text{A}}_{p} {\text{A}}_{q} \,{\text{d}}s\,\dot{\alpha }_{p} \alpha_{q} } \hfill & {1 \le i \le m, 1 \le j \le n} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {C_{m + i,j}^{nl} = \left( {j_{2} - j_{3} } \right)\mathop \sum \limits_{p = 1}^{m} \mathop \sum \limits_{k = 1}^{n} \mathop \int \limits_{0}^{1} A_{i} V_{j}^{{\prime }} V_{p}^{{\prime }} A_{k} {\text{d}}s\,\dot{v}_{p} \alpha_{k} + e_{z} \mathop \sum \limits_{p = 1}^{m} \mathop \sum \limits_{q = 1}^{m} \mathop \int \limits_{0}^{1} A_{i} V_{p}^{{\prime }} \mathop \int \limits_{0}^{s} V_{j}^{{\prime }} V_{q}^{{\prime }} \,{\text{d}}s \,{\text{d}}sv_{p} \dot{v}_{q} } \hfill & {1 \le i \le n, 1 \le j \le m} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {K_{i,j}^{nl} = - \mathop \sum \limits_{p = 1}^{m} \mathop \sum \limits_{q = 1}^{m} \mathop \int \limits_{0}^{1} V_{i} \left[ {V_{j}^{{\prime \prime }} \left( {V_{p}^{{\prime }} V_{q}^{{\prime \prime }} } \right)^{'} } \right]^{'} {\text{d}}s\,v_{p} v_{q} - \left( {\beta_{z} - 1} \right)\mathop \sum \limits_{k = 1}^{n} \mathop \sum \limits_{p = 1}^{n} \mathop \int \limits_{0}^{1} V_{i} \left( {V_{j}^{{\prime \prime }} {\text{A}}_{k} {\text{A}}_{p} } \right)^{{\prime \prime }} {\text{d}}s\,\alpha_{k} \alpha_{p} } \hfill & {1 \le i \le m, 1 \le j \le m} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {K_{m + i,j}^{nl} = \left( {1 - \beta_{z} } \right)\mathop \sum \limits_{p = 1}^{m} \mathop \sum \limits_{k = 1}^{n} \mathop \int \limits_{0}^{1} A_{i} V_{j}^{''} V_{p}^{''} A_{k} {\text{d}}sv_{p} \alpha_{k} } \hfill & {1 \le i \le n, 1 \le j \le m} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {M_{m + i,m + j}^{a} = - \mu b^{2} \left( {\frac{1}{8} + a^{2} } \right)\mathop \int \limits_{0}^{1} A_{m} \left( s \right)A_{k} \left( s \right) } \hfill & {1 \le i \le n, 1 \le j \le m} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {C_{i,j}^{a} = c_{a} \phi_{c\alpha } \left( 0 \right)\mathop \int \limits_{0}^{1} V_{i} \left( s \right)V_{j} \left( s \right){\text{d}}s} \hfill & {1 \le i \le m, 1 \le j \le m} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {C_{i,m + j}^{a} = \left[ {\mu U + 2c_{a} b\phi_{cq} \left( 0 \right)} \right]\mathop \int \limits_{0}^{1} V_{i} \left( s \right)A_{j} \left( s \right){\text{d}}s} \hfill & {1 \le i \le m, 1 \le j \le n} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {C_{m + i,j}^{a} = 2bc_{a} \phi_{cm} \left( 0 \right)\mathop \int \limits_{0}^{1} A_{i} \left( s \right)V_{j} \left( s \right){\text{d}}s} \hfill & {1 \le i \le n, 1 \le j \le m} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {C_{m + i,m + j}^{a} = \left[ {4b^{2} c_{a} \phi_{cmq} \left( 0 \right) - \mu bU\left( {\frac{1}{2} - a} \right)} \right]\mathop \int \limits_{0}^{1} A_{i} \left( s \right)A_{j} \left( s \right){\text{d}}s} \hfill & {1 \le i \le n, 1 \le j \le n} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {K_{i,j}^{a} = c_{a} \dot{\phi }_{c\alpha } \left( 0 \right)\mathop \int \limits_{0}^{1} V_{i} \left( s \right)V_{j} \left( s \right){\text{d}}s,} \hfill & {1 \le i \le m, 1 \le j \le m} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {K_{i,m + j}^{a} = c_{a} \left[ {U\phi_{c\alpha } \left( 0 \right) + 2b\dot{\phi }_{cq} \left( 0 \right)} \right]\mathop \int \limits_{0}^{1} V_{i} \left( s \right)A_{j} \left( s \right){\text{d}}s} \hfill & {1 \le i \le m, 1 \le j \le n} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {K_{m + i,j}^{a} = 2bc_{a} \dot{\phi }_{cm} \left( 0 \right)\mathop \int \limits_{0}^{1} A_{i} \left( s \right)V_{j} \left( s \right){\text{d}}s} \hfill & {1 \le i \le n, 1 \le j \le m} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {K_{m + i,m + j}^{a} = 2bc_{a} \left[ {U\phi_{cm} \left( 0 \right) + 2b\dot{\phi }_{cmq} \left( 0 \right)} \right]\mathop \int \limits_{0}^{1} A_{i} \left( s \right)A_{j} \left( s \right){\text{d}}s} \hfill & {1 \le i \le n, 1 \le j \le n} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {IC_{i,j} = - c_{a} \dot{\phi }_{c\alpha } \mathop \int \limits_{0}^{1} V_{i} \left( s \right)V_{j} \left( s \right){\text{d}}s,} \hfill & {1 \le i \le m, 1 \le j \le m} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {IC_{i,m + j} = - 2c_{a} b\dot{\phi }_{cq} \mathop \int \limits_{0}^{1} V_{i} \left( s \right)A_{j} \left( s \right){\text{d}}s,} \hfill & {1 \le i \le m, 1 \le j \le n} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {IC_{m + i,j} = - 2bc_{a} \dot{\phi }_{cm} \mathop \int \limits_{0}^{1} A_{i} \left( s \right)V_{j} \left( s \right){\text{d}}s,} \hfill & {1 \le i \le n, 1 \le j \le m} \hfill \\ \end{array} $$

$$ \begin{array}{*{20}l} {IC_{m + i,m + j} = - 4b^{2} c_{a} \dot{\phi }_{cmq} \mathop \int \limits_{0}^{1} A_{i} \left( s \right)A_{j} \left( s \right){\text{d}}s} \hfill & {1 \le i \le n, 1 \le j \le n} \hfill \\ \end{array} $$

$M_{i,j}$, $C_{i,j}$ and $K_{i,j}$; $M_{i,j}^{nl}$, $C_{i,j}^{nl}$ and $K_{i,j}^{nl}$; $M_{i,j}^{a}$, $C_{i,j}^{a}$, and $K_{i,j}^{a}$ denote the elements of mass, damping, and stiffness matrices that have been categorized in terms of linear, nonlinear, and aerodynamic ones, respectively. The row and column positions of each element in the matrix are specified with their subscripts. Recall that each matrix is square on the order of $m + n$, and the elements of matrices not mentioned above are zero. The elements of the initial condition matrix are defined by $IC_{i,j}$.