A positivity-preserving edge-centred finite volume scheme for heterogeneous and anisotropic diffusion problems on polygonal meshes

Abstract

In many application problems such as the electromagnetics, the unknowns are usually defined at the edges to satisfy the continuity requirement. This paper develops the first positivity-preserving edge-centred finite volume scheme for diffusion problems on general unstructured polygonal meshes. The edge-centred unknowns are primary and have associated finite volume equations. The cell-vertex and cell-centred unknowns are treated as auxiliary ones and are interpolated by the primary unknowns, making the final scheme purely edge-centred. The scheme has a fixed stencil due to the fixed decomposition of the co-normal, which makes the scheme very easy to implement. The positivity-preserving property is rigorously proved. Numerical experiments indicate that the scheme has second-order accuracy and positivity for heterogeneous and anisotropic problems on highly distorted meshes.

Appendix

Lemma 1

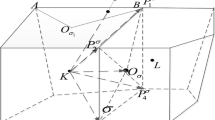
For arbitrary two triangles $\triangle \varvec{x}_E\varvec{x}_P\varvec{x}_K$ and $\triangle \varvec{x}_F\varvec{x}_P\varvec{x}_K$ with the common edge $\sigma$ (see Fig. 2), $\varvec{n}_{E,\sigma }$ is the unit outer vector normal to the edge $\sigma$ outward to the triangle $\triangle \varvec{x}_E\varvec{x}_P\varvec{x}_K$. $\Lambda$ is a positive definite tensor. For the decomposition (3) and (7), we have

$$\begin{aligned} d_{E,\sigma }(\alpha _{E,\sigma }+\beta _{E,\sigma })=d_{F,\sigma }(\alpha _{F,\sigma }+\beta _{F,\sigma })>0. \end{aligned}$$

(27)

where $d_{E,\sigma }$ (resp. $d_{F,\sigma }$) denotes the distance from the vertex ${\varvec{x}}_{E}$ (resp. ${\varvec{x}}_{F}$) to the edge ${\varvec{x}}_K{\varvec{x}}_P$.

Proof

The geometrical relations gives

$$\begin{aligned} {({\varvec{x}}_{K}{\varvec{x}}_{E})^T\mathcal {R}({\varvec{x}}_{P}{\varvec{x}}_{E}) =-({\varvec{x}}_{P}{\varvec{x}}_{E})^T\mathcal {R}({\varvec{x}}_{K}{\varvec{x}}_{E})} =-|{\varvec{x}}_K{\varvec{x}}_P|d_{E,\sigma }. \end{aligned}$$

Combined this with (4), we have

$$\begin{aligned} \alpha _{E,\sigma }+\beta _{E,\sigma } ={\frac{|{\varvec{x}}_K{\varvec{x}}_P|{\varvec{n}}_{E,\sigma }^T\Lambda \mathcal {R}({\varvec{x}}_P{\varvec{x}}_K)}{({\varvec{x}}_{K}{\varvec{x}}_{E})^T\mathcal {R}({\varvec{x}}_P{\varvec{x}}_{E})} }=\frac{|{\varvec{x}}_K{\varvec{x}}_P|{\varvec{n}}_{E,\sigma }^T\Lambda {\varvec{n}}_{E,\sigma }}{d_{E,\sigma }}, \end{aligned}$$

where the identity ${\mathcal {R}({\varvec{x}}_P{\varvec{x}}_K)}=-|{\varvec{x}}_K{\varvec{x}}_P|{\varvec{n}}_{E,\sigma }$ is used in second equality. Similarly, we obtain

$$\begin{aligned} \alpha _{F,\sigma }+\beta _{F,\sigma } =\frac{|{\varvec{x}}_K{\varvec{x}}_P|{\varvec{n}}_{F,\sigma }^T\Lambda {\varvec{n}}_{F,\sigma }}{d_{F,\sigma }}. \end{aligned}$$

Recalling that ${\varvec{n}}_{E,\sigma }=-{\varvec{n}}_{F,\sigma }$ and $\Lambda$ is positive definite, we immediately obtain (27). $\square$