Skip to main content
SpringerLink
Log in

Approximate solution of time-fractional fuzzy partial differential equations

  • Published:
Computational and Applied Mathematics Aims and scope Submit manuscript

Abstract

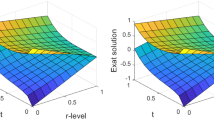
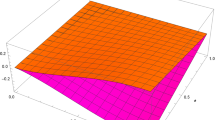
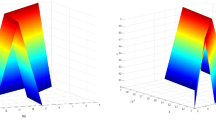
In this study, we develop perturbation–iteration algorithm (PIA) for numerical solutions of some types of fuzzy fractional partial differential equations (FFPDEs) with generalized Hukuhara derivative. We also present the convergence analysis of the method. The proposed approach reveals fast convergence rate and accuracy of the present method when compared with exact solutions of crisp problems. The main efficiency of this method is that while scaling support zone of uncertainty for the fractional partial differential equations, it eliminates over calculation and produces highly approximate and accurate results. Error analysis of the PIA for the FFPDEs is also illustrated within examples.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  • Abbas S, Benchohra M, N’Guerekata GM (2012) Topics in fractional differential equations, vol 27. Springer, New York

    Book  MATH  Google Scholar 

  • Ahmadian A, Salahshour S, Chan CS (2017) Fractional differential systems: a fuzzy solution based on operational matrix of shifted chebyshev polynomials and its applications. IEEE Trans Fuzzy Syst 25(1):218–236

    Article  Google Scholar 

  • Ahmad MZ, Hasan MK, Abbasbandy S (2013) Solving fuzzy fractional differential equations using Zadeh’s extension principle. Sci World J. https://doi.org/10.1155/2013/454969

  • Aksoy Y, Pakdemirli M (2010) New perturbation–iteration solutions for Bratu-type equations. Comput Math Appl 59:2802–2808

    Article  MathSciNet  MATH  Google Scholar 

  • Alquran M (2014) Analytical solutions of fractional foam drainage equation by residual power series method. Math Sci 8(4):153–160

    Article  MathSciNet  MATH  Google Scholar 

  • El-Ajou A, Arqub OA, Momani S (2015) Approximate analytical solution of the nonlinear fractional KdV-Burgers equation: a new iterative algorithm. J Comput Phys 293:81–95

    Article  MathSciNet  MATH  Google Scholar 

  • Atpinar S, Zararsiz Z, Sengonul M (2017) The entropy value for ECG of the sport horses. IEEE Trans Fuzzy Syst 25(5):1168–1174

    Article  Google Scholar 

  • Allahviranloo T, Taheri N (2009) An analytic approximation to the solution of fuzzy heat equation by adomian decomposition method. Int J Contemp Math Sci 4(3):105–114

    MathSciNet  MATH  Google Scholar 

  • Allahviranloo T, Armand A, Gouyandeh Z (2014) Fuzzy fractional differential equations under generalized fuzzy Caputo derivative. J Intell Fuzzy Syst 26(3):1481–1490

    Article  MathSciNet  MATH  Google Scholar 

  • Bagley RL, Torvik PJ (1983) A theoretical basis for the application of fractional calculus to viscoelasticity. J Rheol 27(3):201–210

    Article  MATH  Google Scholar 

  • Balachandran K, Park JY, Trujillo JJ (2012) Controllability of nonlinear fractional dynamical systems. Nonlinear Anal Theory Methods Appl 75(4):1919–1926

    Article  MathSciNet  MATH  Google Scholar 

  • Bede B, Gal SG (2005) Generalizations of the differentiability of fuzzy-number-valued functions with applications to fuzzy differential equations. Fuzzy Sets Syst 151(3):581–599

    Article  MathSciNet  MATH  Google Scholar 

  • Bildik N (2017) General convergence analysis for the perturbation iteration technique. Turk J Math Comput Sci 6:1–9

    Google Scholar 

  • Cooper K, Mickens R (2002) Generalized harmonic balance/numerical method for determining analytical approximations to the periodic solutions of the \(x^{4/3}\) potential. J Sound Vib 250:951–954

    Article  MATH  MathSciNet  Google Scholar 

  • Das AK, Roy TK (2017) Exact solution of some linear fuzzy fractional differential equation using Laplace transform method. Glob J Pure Appl Math 13(9):5427–5435

    Google Scholar 

  • Diamond P, Kloeden P (1994) Metric spaces of fuzzy sets: theory and applications. World Scientific, Singapore

    Book  MATH  Google Scholar 

  • Takaci D, Takaci A, Aleksandar T (2014) On the solutions of fuzzy fractional differential equation. TWMS J Appl Eng Math 4(1):98

    MathSciNet  MATH  Google Scholar 

  • Dolapci IT, Şenol M, Pakdemirli M (2013) New perturbation iteration solutions for Fredholm and Volterra integral equations. J Appl Math. https://doi.org/10.1155/2013/682537

  • Duan JS, Chaolu T, Rach R, Lu L (2013) The Adomian decomposition method with convergence acceleration techniques for nonlinear fractional differential equations. Comput Math Appl 66(5):728–736

    Article  MathSciNet  MATH  Google Scholar 

  • Hasan S, Alawneh A, Al-Momani M, Momani S (2017) Second order fuzzy fractional differential equations under caputo’s H-differentiability. Appl Math Inf Sci 11(6):1597–1608

    Article  MathSciNet  Google Scholar 

  • He J-H (2012) Homotopy perturbation method with an auxiliary term. Abstract and applied analysis. Hindawi Publishing Corporation, Cairo

    Book  MATH  Google Scholar 

  • Hu H, Xiong Z-G (2003) Oscillations in an \(x^{(2m+2)/(2n+1)}\) potential. J Sound Vib 259:977–980

    Article  MathSciNet  MATH  Google Scholar 

  • Hukuhara M (1967) Integration des applications mesurables dont la valeur est un compact convexe. Funkcial Ekvac 10:205–223

    MathSciNet  MATH  Google Scholar 

  • Iqbal S, Javed A (2011) Application of optimal homotopy asymptotic method for the analytic solution of singular Lane–Emden type equation. Appl Math Comput 217:7753–7761

    MathSciNet  MATH  Google Scholar 

  • Jameel AF (2014) Semi-analytical solution of heat equation in fuzzy environment. Int J Appl Phys Math 4(6):371

    Article  Google Scholar 

  • Jordan DW, Smith P (1999) Nonlinear ordinary differential equations: an introduction to dynamical systems, vol 2. Oxford University Press, Oxford

    MATH  Google Scholar 

  • Kurt A, Tasbozan O, Cenesiz Y (2016) Homotopy analysis method for conformable Burgers–Korteweg–de Vries equation. Bull Math Sci Appl 17:17–23

    Google Scholar 

  • Kaleva O (1987) Fuzzy differential equations. Fuzzy Sets Syst 24:301–317

    Article  MathSciNet  MATH  Google Scholar 

  • Liu Y, Wang X (2010) Contraction conditions with perturbed linear operators and applications. Math Commun 15(1):25–35

    MathSciNet  MATH  Google Scholar 

  • Matloka M (1986) Sequences of fuzzy numbers. Busefal 28(1):28–37

    MATH  Google Scholar 

  • Mizukoshi MT (2007) Fuzzy differential equations and the extension principle. Inf Sci 177(17):3627–3635

    Article  MathSciNet  MATH  Google Scholar 

  • Momani S, Odibat Z (2006) Analytical approach to linear fractional partial differential equations arising in fluid mechanics. Phys Lett A 355(4–5):271–279

    Article  MATH  Google Scholar 

  • Moore RE (1959) Automatic error analysis in digital computation. Technical Report LMSD-48421, Lockheed Missiles and Space Company

  • Nanda S (1989) On sequences of fuzzy numbers. Fuzzy Sets Syst 33(1):123–126

    Article  MathSciNet  MATH  Google Scholar 

  • Nayfeh AH (2011) Introduction to perturbation techniques. Wiley, Amsterdam

    MATH  Google Scholar 

  • Pakdemirli M, Boyacı H (2007) Generation of root finding algorithms via perturbation theory and some formulas. Appl Math Comput 184(2):783–788

    MathSciNet  MATH  Google Scholar 

  • Pakdemirli M, Boyacı H, Yurtsever H (2007) Perturbative derivation and comparisons of root-finding algorithms with fourth order derivatives. Math Comput Appl 12(2):117

    MathSciNet  MATH  Google Scholar 

  • Pakdemirli M, Boyacı H, Yurtsever H (2008) A root-finding algorithm with fifth order derivatives. Math Comput Appl 13(2):123

    MathSciNet  MATH  Google Scholar 

  • Pandey RK, Mishra HK (2017) Homotopy analysis Sumudu transform method for time–fractional third order dispersive partial differential equation. Adv Comput Math 43(2):365–383

    Article  MathSciNet  MATH  Google Scholar 

  • Puri ML, Ralescu DA (1983) Differentials of fuzzy functions. J Math Anal Appl 91(2):552–558

    Article  MathSciNet  MATH  Google Scholar 

  • Rahman NAA, Ahmad MZ (2016) Solution of fuzzy fractional differential equations using fuzzy Sumudu transform. In: AIP conference proceedings, 1775.1, AIP Publishing

  • Rivaz A, Fard OS, Bidgoli TA (2016) Solving fuzzy fractional differential equations by a generalized differential transform method. SeMA J 73(2):149–170

    Article  MathSciNet  MATH  Google Scholar 

  • Salahshour S, Ahmadian A, Chan CS (2016) A novel technique for solving fuzzy differential equations of fractional order using Laplace and integral transforms. In: 2016 IEEE international conference on fuzzy systems (FUZZ-IEEE), Vancouver, pp 1473–1477

  • Salahshour S, Ahmadian A, Chan CS, Baleanu D (2015) Toward the existence of solutions of fractional sequential differential equations with uncertainty. In: 2015 IEEE international conference on fuzzy systems (FUZZ-IEEE), Istanbul, pp 1–6

  • Şenol M, Dolapci IT, Aksoy Y, Pakdemirli M (2013) Perturbation-iteration method for first-order differential equations and systems. Abstract and applied analysis. Hindawi Publishing Corporation, Cairo

    Book  MATH  Google Scholar 

  • Şenol M, Dolapci IT (2016) On the perturbation–iteration algorithm for fractional differential equations. J King Saud Univ Sci 28(1):69–74

    Article  Google Scholar 

  • Skorokhod AV, Hoppensteadt FC, Salehi HD (2002) Random perturbation methods with applications in science and engineering, vol 150. Springer, New York

    Book  MATH  Google Scholar 

  • von Groll G, Ewins DJ (2001) The harmonic balance method with arc-length continuation in rotor/stator contact problems. J Sound Vib 241:223–233

    Article  Google Scholar 

  • Wang SQ, He JH (2008) Nonlinear oscillator with discontinuity by parameter-expansion method. Chaos Solit Fract 35:688–691

    Article  MATH  Google Scholar 

  • Wilansky A (2000) Summability through functional analysis, vol 85. Elsevier, Amsterdam

    MATH  Google Scholar 

  • Yeroglu C, Senol B (2013) Investigation of robust stability of fractional order multilinear affine systems: 2q-convex parpolygon approach. Syst Control Lett 62(10):845–855

    Article  MathSciNet  MATH  Google Scholar 

  • Zadeh LA (1965) Fuzzy sets. Inf Control 8(3):338–353

    Article  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Nevşehir Hacı Bektaş Veli University, Nevşehir, Turkey

    Mehmet Senol, Sevda Atpinar & Zarife Zararsiz

  2. Young Researchers and Elite Club, Mobarakeh Branch, Islamic Azad University, Mobarakeh, Iran

    Soheil Salahshour

  3. Institute for Mathematical Research, Universiti Putra Malaysia, 43400 UPM, Serdang, Selangor, Malaysia

    Ali Ahmadian

Authors
  1. Mehmet Senol
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Sevda Atpinar
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Zarife Zararsiz
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Soheil Salahshour
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Ali Ahmadian
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Mehmet Senol.

Additional information

Communicated by Marcos Eduardo Valle.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Senol, M., Atpinar, S., Zararsiz, Z. et al. Approximate solution of time-fractional fuzzy partial differential equations. Comp. Appl. Math. 38, 18 (2019). https://doi.org/10.1007/s40314-019-0796-6

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s40314-019-0796-6

Keywords

Mathematics Subject Classification

Search

Navigation