Approximate Models of Singularly Perturbed Time-Varying Systems: A Bond Graph Approach

Abstract

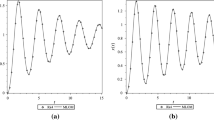
A bond graph model in an integral causality assignment (BGI) for a singularly perturbed linear time-varying (LTV) system is proposed. The LTV constitutive relations of the elements and MTF and MGY elements modulated by LTV functions of the BGI are considered. A new bond graph called singularly perturbed varying bond graph (SPVBG) for determining the quasi-steady-state model is presented. This SPVBG has the property that the storage elements for the slow and fast dynamics have an integral and derivative causality assignment, respectively. In order to apply the proposed methodology, a case study of an electromechanical system is modelled by bond graphs and approximated models are obtained. Finally, simulation results for the exact and approximated solutions are shown.

Appendices

Proof of Lemma 1

Proof. From the second line of (33) with (32)

$$\begin{aligned} D_\mathrm{in}^{h}= & {} \left[ I-H_{22}\left( t\right) L^{h}\left( t\right) \right] ^{-1} \nonumber \\&\times \left[ H_{21}^{11}\left( t\right) z_{1}+H_{21}^{12}\left( t\right) \overset{ \bullet }{x}_{2}+H_{21}^{13}\left( t\right) \overset{\bullet }{x} _{2}^{d}+H_{23}\left( t\right) u\right] \nonumber \\ \end{aligned}$$

(70)

from the derivative of the fifth line of (33) with (21) and (22)

$$\begin{aligned} \overset{\bullet }{x}_{1}^{d}= & {} \left\{ \frac{\mathrm{d}\left[ F_{1}^{d}\left( t\right) \right] ^{-1}}{\mathrm{d}t}H_{41}^{11}\left( t\right) +\left[ F_{1}^{d}\left( t\right) \right] ^{-1}\overset{\bullet }{H}_{41}^{11}\left( t\right) \right\} F_{1}\left( t\right) x_{1} \nonumber \\&+\left[ F_{1}^{d}\left( t\right) \right] ^{-1}H_{41}^{11}\overset{\bullet }{ F}_{1}\left( t\right) x_{1}+\left[ F_{1}^{d}\left( t\right) \right] ^{-1}H_{41}^{11}F_{1}\left( t\right) \overset{\bullet }{x}_{1} \nonumber \\ \end{aligned}$$

(71)

by substituting (21), (70) and (71) into the first line of (33) with (43),

(72)

simplifying (72) with (42) we have

$$\begin{aligned} \widetilde{E_{1}}\left( t\right) \overset{\bullet }{x}_{1}= & {} \left[ H_{11}^{11}\left( t\right) +H_{12}^{11}M_{h}\left( t\right) H_{21}^{11}\left( t\right) \right] F_{1}\left( t\right) x_{1}\nonumber \\&+\left[ H_{11}^{12}\left( t\right) +H_{12}^{11}\left( t\right) +M_{h}\left( t\right) H_{21}^{12}\left( t\right) \right] \overset{\bullet }{x}_{2} \nonumber \\&+\left[ H_{11}^{13}\left( t\right) +H_{12}^{11}\left( t\right) M_{h}\left( t\right) H_{21}^{13}\left( t\right) \right] \overset{\bullet }{x} _{2}^{d}\nonumber \\&+H_{14}^{11}\left( t\right) \left[ F_{1}^{d}\left( t\right) \right] ^{-1}H_{41}^{11}F_{1}\left( t\right) \overset{\bullet }{x}_{1}\nonumber \\&+\left[ H_{13}^{11}\left( t\right) +H_{12}^{11}\left( t\right) M_{h}\left( t\right) H_{23}\left( t\right) \right] u\nonumber \\&+H_{14}^{11}\left( t\right) \left\{ \frac{\mathrm{d}\left[ F_{1}^{d}\left( t\right) \right] ^{-1}}{\mathrm{d}t}H_{41}^{11}\left( t\right) \right. \nonumber \\&+\left. \left[ F_{1}^{d}\left( t\right) \right] ^{-1}\overset{\bullet }{H} _{41}^{11}\left( t\right) F_{1}\left( t\right) x_{1}\right\} \end{aligned}$$

(73)

with (38)–(40) and (41), (73) being written by

$$\begin{aligned} \overset{\bullet }{x}_{1}=\widetilde{A_{11}}\left( t\right) x_{1}+\widetilde{ A_{12}}\left( t\right) \overset{\bullet }{x}_{2}+\widetilde{B_{1}}\left( t\right) u+\widetilde{A_{13}}\left( t\right) \overset{\bullet }{x}_{2}^{d} \end{aligned}$$

(74)

where

$$\begin{aligned} \widetilde{A_{12}}\left( t\right)= & {} \left[ \widetilde{E_{1}}\left( t\right) \right] ^{-1}\left[ H_{11}^{12}\left( t\right) +H_{12}^{11}\left( t\right) M_{h}\left( t\right) H_{21}^{12}\left( t\right) \right] \nonumber \\\end{aligned}$$

(75)

$$\begin{aligned} \widetilde{A_{13}}\left( t\right)= & {} \left[ \widetilde{E_{1}}\left( t\right) \right] ^{-1}\left[ H_{11}^{13}\left( t\right) +H_{12}^{11}\left( t\right) M_{h}\left( t\right) H_{21}^{13}\left( t\right) \right] \nonumber \\ \end{aligned}$$

(76)

From the second line of (33) and taking (70) with (43),

$$\begin{aligned} z_{2}= & {} \left[ H_{11}^{21}\left( t\right) +H_{12}^{21}\left( t\right) M_{h}\left( t\right) H_{21}^{11}\left( t\right) \right] F_{1}\left( t\right) x_{1}\left( t\right) \nonumber \\&+\left[ H_{11}^{22}\left( t\right) +H_{12}^{21}\left( t\right) M_{h}\left( t\right) H_{21}^{12}\left( t\right) \right] \overset{ \bullet }{x}_{2} \nonumber \\&+\left[ H_{11}^{23}\left( t\right) +H_{12}^{21}\left( t\right) M_{h}\left( t\right) H_{21}^{13}\left( t\right) \right] \overset{\bullet }{x}_{2}^{d}\nonumber \\&+\left[ H_{13}^{21}\left( t\right) +H_{12}^{21}\left( t\right) M_{h}\left( t\right) H_{23}\left( t\right) \right] u \end{aligned}$$

(77)

with (23), (35) and (36), (77) being written by

$$\begin{aligned} x_{2}=\widetilde{A_{21}}\left( t\right) x_{1}+\widetilde{A_{22}}\left( t\right) \overset{\bullet }{x}_{2}+\widetilde{A_{23}}\left( t\right) \overset{\bullet }{x}_{2}^{d}+\widetilde{B_{2}}u \end{aligned}$$

(78)

where

$$\begin{aligned} \widetilde{A_{22}}\left( t\right)= & {} F_{2}^{-1}\left( t\right) \left[ H_{11}^{22}\left( t\right) +H_{12}^{21}\left( t\right) M_{h}\left( t\right) H_{21}^{12}\left( t\right) \right] \end{aligned}$$

(79)

$$\begin{aligned} \widetilde{A_{23}}\left( t\right)= & {} F_{2}^{-1}\left( t\right) \left[ H_{11}^{23}\left( t\right) +H_{12}^{21}\left( t\right) M_{h}\left( t\right) H_{21}^{13}\left( t\right) \right] \end{aligned}$$

(80)

For the steady-state response, $\overset{\bullet }{x}_{2}=0$ and $\overset{ \bullet }{x}_{2}^{d}=0$; from (78), the real roots of (34) for the fast dynamics are proved.

From (78) with the linearly independent state variables

$$\begin{aligned} \overset{\bullet }{x}_{2}=-\widetilde{A_{22}}^{-1}\left( t\right) \widetilde{ A_{21}}\left( t\right) x_{1}+\widetilde{A_{22}}^{-1}\left( t\right) x_{2}- \widetilde{A_{22}}^{-1}\left( t\right) \widetilde{B_{2}}u \end{aligned}$$

(81)

and comparing with (8), the relationships between these models are given by

$$\begin{aligned}&\widetilde{A_{22}}\left( t\right) =A_{22}^{-1}\left( t\right) \varepsilon \end{aligned}$$

(82)

$$\begin{aligned}&\widetilde{A_{21}}\left( t\right) =-A_{22}^{-1}\left( t\right) A_{21}\left( t\right) \end{aligned}$$

(83)

$$\begin{aligned}&\widetilde{B_{2}}\left( t\right) =-A_{22}^{-1}\left( t\right) B_{2}\left( t\right) \end{aligned}$$

(84)

by substituting (81) into (78)

$$\begin{aligned} \overset{\bullet }{x}_{1}= & {} \left[ \widetilde{A_{11}}\left( t\right) - \widetilde{A_{12}}\left( t\right) \widetilde{A_{22}}^{-1}\left( t\right) \widetilde{A_{21}}\left( t\right) \right] x_{1}\nonumber \\&+\left[ \widetilde{A_{12}} \left( t\right) \widetilde{A_{22}}^{-1}\left( t\right) \right] x_{2}\nonumber \\&+\left[ \widetilde{B_{1}}\left( t\right) -\widetilde{A_{12}}\left( t\right) \widetilde{A_{22}}^{-1}\left( t\right) \widetilde{B_{2}}\left( t\right) \right] u \end{aligned}$$

(85)

comparing (7) with (85)

$$\begin{aligned} \widetilde{A_{12}}\left( t\right)= & {} A_{12}\left( t\right) A_{22}^{-1}\left( t\right) \varepsilon \end{aligned}$$

(86)

$$\begin{aligned} \widetilde{A_{11}}\left( t\right)= & {} A_{11}\left( t\right) -A_{12}\left( t\right) A_{22}^{-1}\left( t\right) A_{21}\left( t\right) =A_{0}\left( t\right) \end{aligned}$$

(87)

$$\begin{aligned} \widetilde{B_{1}}\left( t\right)= & {} B_{1}\left( t\right) -A_{12}\left( t\right) A_{22}^{-1}\left( t\right) B_{2}\left( t\right) =B_{0}\left( t\right) \end{aligned}$$

(88)

it can be seen that the quasi-steady-state model is determined by $x_{2}=0$ in (85) with (86)–(88) and (10), (37) being proved.

Proof of Assumptions

1.1 Assumption 4

From the second line of (27)

$$\begin{aligned} A_{22}\left( t\right)= & {} \left[ E_{2}\left( t\right) F_{2}\left( t\right) \right] ^{-1}\nonumber \\&\times \left\{ \left[ S_{11}^{22}\left( t\right) F_{2}\left( t\right) +S_{11}^{21}\left( t\right) M\left( t\right) S_{21}^{12}\left( t\right) F_{2}\left( t\right) \right] \right. \nonumber \\&\left. +S_{14}^{22}\left( t\right) \frac{\mathrm{d}}{\mathrm{d}t}\left\{ \left[ F_{2}^{d}\left( t\right) \right] ^{-1}S_{31}^{22}\left( t\right) F_{2}\left( t\right) \right\} \right\} \end{aligned}$$

(89)

and

$$\begin{aligned} A_{22}^{T}\left( t\right)= & {} \left\{ F_{2}^{T}\left( t\right) \left[ S_{11}^{21}\left( t\right) \right] ^{T}\right. \nonumber \\&+F_{2}^{T}\left( t\right) \left[ S_{21}^{12}\left( t\right) \right] ^{T}M^{T}\left( t\right) \left[ S_{11}^{21}\left( t\right) \right] ^{T} \nonumber \\&\times \left. \frac{\mathrm{d}}{\mathrm{d}t}\left\{ F_{2}\left( t\right) \left[ S_{31}^{22}\left( t\right) \right] ^{T}\left[ F_{2}^{d}\left( t\right) \right] ^{-1}\right\} \left[ S_{14}^{22}\left( t\right) \right] ^{T}\right\} \nonumber \\&\times \left[ E_{2}\left( t\right) F_{2}\left( t\right) \right] ^{-T} \end{aligned}$$

(90)

the system will be stable if

$$\begin{aligned} A_{22}\left( t\right) +A_{22}^{T}\left( t\right) \le 0 \end{aligned}$$

(91)

the properties for the junction structure are established as

$$\begin{aligned} S_{11}^{22}\left( t\right)= & {} -\left[ S_{11}^{22}\left( t\right) \right] ^{T};\\ S_{14}^{22}\left( t\right)= & {} -\left[ S_{31}^{22}\left( t\right) \right] ^{T}\\ S_{12}^{21}\left( t\right)= & {} -\left[ S_{21}^{12}\left( t\right) \right] ^{T}\\ S_{22}\left( t\right)= & {} -\left[ S_{22}\left( t\right) \right] ^{T} \end{aligned}$$

and

$$\begin{aligned} F_{2}=F_{2}^{T};\text { }L=L^{T};\text { }F_{2}^{d}=\left( F_{2}^{d}\right) ^{T} \end{aligned}$$

by substituting (89) and (90) into (91)

$$\begin{aligned}&-F_{2}^{-1}\left( t\right) E_{2}^{-1}\left( t\right) \left[ S_{11}^{22}\left( t\right) \right] ^{T}-F_{2}\left( t\right) \left[ S_{11}^{22}\left( t\right) \right] \nonumber \\&\quad \times F_{2}^{-1}\left( t\right) E_{2}^{-1}\left( t\right) -F_{2}^{-1}\left( t\right) E_{2}^{-1}\left( t\right) \left[ S_{11}^{22}\left( t\right) \right] ^{T}\nonumber \\&\quad \times \left[ L^{-1}\left( t\right) +S_{22}^{T}\left( t\right) \right] ^{-1} S_{21}^{12}\left( t\right) F_{2}\left( t\right) \nonumber \\&\quad -F_{2}\left( t\right) \left[ S_{12}^{21}\left( t\right) \right] \left[ L^{-1}\left( t\right) +S_{22}^{T}\left( t\right) \right] ^{-1} \nonumber \\&\quad \times \left[ S_{12}^{21}\left( t\right) \right] ^{T}-F_{2}^{-1}\left( t\right) E_{2}^{-1}\left( t\right) \left[ S_{31}^{22}\left( t\right) \right] ^{T} \nonumber \\&\quad \times \frac{\mathrm{d}}{\mathrm{d}t}\left\{ \left[ F_{2}^{d}\left( t\right) \right] ^{-1}S_{31}^{22}\left( t\right) F_{2}\left( t\right) \right\} \nonumber \\&\quad -\frac{\mathrm{d}}{\mathrm{d}t}\left\{ F_{2}\left( t\right) \left[ S_{31}^{22}\left( t\right) \right] ^{T}\left[ F_{2}^{d}\left( t\right) \right] ^{-1}\right\} \nonumber \\&\quad \times \left[ S_{14}^{22}\left( t\right) \right] ^{T}F_{2}^{-1}\left( t\right) E_{2}^{-1}\left( t\right) \le 0 \end{aligned}$$

(92)

from (92), the stability conditions given in (44) are proved.

1.2 Assumption 5

From the second line of (27) and applying the norm to this expression

$$\begin{aligned} \left\| A_{2}\left( t\right) \right\|= & {} \left\| \left[ E_{2}\left( t\right) F_{2}\left( t\right) \right] ^{-1}\right. \nonumber \\&\times \left. \left\{ \left[ S_{11}^{22}\left( t\right) F_{2}\left( t\right) +S_{11}^{21}\left( t\right) M\left( t\right) S_{21}^{12}\left( t\right) F_{2}\left( t\right) \right] \right. \right. \nonumber \\&\left. \left. +S_{14}^{22}\left( t\right) \frac{\mathrm{d}}{\mathrm{d}t}\left\{ \left[ F_{2}^{d}\left( t\right) \right] ^{-1}S_{31}^{22}\left( t\right) F_{2}\left( t\right) \right\} \right\} \right\| \end{aligned}$$

(93)

this can be written by

$$\begin{aligned}&\left\| A_{2}\left( t\right) \right\| \le \left\| \left[ E_{2}\left( t\right) F_{2}\left( t\right) \right] ^{-1}\right\| \nonumber \\&\quad \times \left\{ \left[ \left\| S_{11}^{22}\left( t\right) \right\| +\left\| S_{11}^{21}\left( t\right) \right\| \times \left\| M\left( t\right) \right\| \right. \right. \nonumber \\&\quad \left. \times \left\| S_{21}^{12}\left( t\right) \right\| \right] \times \left\| F_{2}\left( t\right) \right\| \nonumber \\&\quad \times \left. \left\| S_{14}^{22}\left( t\right) \right\| \times \left\| \frac{\mathrm{d}}{\mathrm{d}t}\left\{ \left[ F_{2}^{d}\left( t\right) \right] ^{-1}S_{31}^{22}\left( t\right) F_{2}\left( t\right) \right\} \right\| \right\} \end{aligned}$$

(94)

from (94) with (45), Assumption 5 is proved.

1.3 Assumption 6

By obtaining the derivative of the second line of (27)

$$\begin{aligned} \overset{\bullet }{A}_{22}\left( t\right)= & {} \frac{\mathrm{d}\left[ E_{2}\left( t\right) F_{2}\left( t\right) \right] ^{-1}}{\mathrm{d}t}\left\{ \left[ S_{11}^{22}\left( t\right) F_{2}\left( t\right) \right. \right. \nonumber \\&\left. +\,S_{11}^{21}\left( t\right) M\left( t\right) S_{21}^{12}\left( t\right) F_{2}\left( t\right) \right] \nonumber \\&\left. +\,S_{14}^{22}\left( t\right) \frac{\mathrm{d}}{\mathrm{d}t}\left\{ \left[ F_{2}^{d}\left( t\right) \right] ^{-1}S_{31}^{22}\left( t\right) F_{2}\left( t\right) \right\} \right\} \nonumber \\&+\left[ E_{2}\left( t\right) F_{2}\left( t\right) \right] ^{-1} \left\{ \frac{\mathrm{d}S_{11}^{22}\left( t\right) F_{2}\left( t\right) }{\mathrm{d}t}\right. \nonumber \\&+\frac{ dS_{11}^{21}\left( t\right) M\left( t\right) S_{21}^{12}\left( t\right) F_{2}\left( t\right) }{dt}\nonumber \\&\left. +\frac{\mathrm{d}}{\mathrm{d}t}S_{14}^{22}\left( t\right) \frac{\mathrm{d}}{\mathrm{d}t}\left\{ \left[ F_{2}^{d}\left( t\right) \right] ^{-1}S_{31}^{22}\left( t\right) F_{2}\left( t\right) \right\} \right\} \nonumber \\ \end{aligned}$$

(95)

and applying the norm

$$\begin{aligned}&\left\| \overset{\bullet }{A}_{22}\left( t\right) \right\| \le \left\| \frac{\mathrm{d}\left[ E_{2}\left( t\right) F_{2}\left( t\right) \right] ^{-1}}{\mathrm{d}t}\right\| \nonumber \\&\quad \times \left[ \left\| S_{11}^{22}\left( t\right) \right\| +\left\| S_{11}^{21}\left( t\right) \right\| \times \left\| M\left( t\right) \right\| \times \left\| S_{21}^{12}\left( t\right) \right\| \right] \nonumber \\&\quad \times \left\| F_{2}\left( t\right) \right\| +\left\| \left[ E_{2}\left( t\right) F_{2}\left( t\right) \right] ^{-1}\right\| \nonumber \\&\quad \times \left[ \left\| \frac{\mathrm{d}S_{11}^{22}\left( t\right) F_{2}\left( t\right) }{\mathrm{d}t}\right\| +\left\| \frac{\mathrm{d}S_{11}^{21}\left( t\right) M\left( t\right) S_{21}^{12}\left( t\right) F_{2}\left( t\right) }{ \mathrm{d}t}\right\| \right. \nonumber \\&\quad +\left\| \frac{\mathrm{d}S_{14}^{22}\left( t\right) }{\mathrm{d}t}\right\| \times \left\| \frac{\mathrm{d}\left[ F_{2}^{d}\left( t\right) \right] ^{-1}S_{31}^{22} \left( t\right) F_{2}\left( t\right) }{\mathrm{d}t}\right\| \nonumber \\&\quad +\left\| S_{14}^{22}\left( t\right) \right\| \times \left\| \frac{ d^{2}\left[ F_{2}^{d}\left( t\right) \right] ^{-1}S_{31}^{22}\left( t\right) F_{2}\left( t\right) }{dt^{2}}\right\| \nonumber \\&\quad \left. +\left\| S_{14}^{22}\left( t\right) \right\| \times \left\| \frac{\mathrm{d}^{2}\left[ F_{2}^{d}\left( t\right) \right] ^{-1}S_{31}^{22}\left( t\right) F_{2}\left( t\right) }{\mathrm{d}t^{2}}\right\| \right] \end{aligned}$$

(96)

from (96) and (46), Assumption 6 is proved.

1.4 Assumption 7

By obtaining the derivative of the first line of (27) corresponding to $A_{12}\left( t\right)$

$$\begin{aligned}&\overset{\bullet }{A}_{12}\left( t\right) \nonumber \\&\quad =\frac{\mathrm{d}E_{1}^{-1}\left( t\right) }{\mathrm{d}t}\left[ S_{11}^{12}\left( t\right) +S_{12}^{11}\left( t\right) M\left( t\right) S_{21}^{12}\left( t\right) \right] F_{2}\left( t\right) \nonumber \\&\qquad +E_{1}^{-1}\left( t\right) \frac{\mathrm{d}\left[ S_{11}^{12}\left( t\right) +S_{12}^{11}\left( t\right) M\left( t\right) S_{21}^{12}\left( t\right) \right] F_{2}\left( t\right) }{\mathrm{d}t}\nonumber \\ \end{aligned}$$

(97)

and applying the norm to $\overset{\bullet }{A}_{22}\left( t\right)$ giving

$$\begin{aligned}&\left\| \overset{\bullet }{A}_{12}\left( t\right) \right\| \le \left\| \frac{\mathrm{d}E_{1}^{-1}\left( t\right) }{\mathrm{d}t}\right\| \left[ \left\| S_{11}^{12}\left( t\right) \right\| +\left\| S_{12}^{11}\left( t\right) \right\| \right. \nonumber \\&\qquad \left. \times \left\| M\left( t\right) \right\| \times \left\| S_{21}^{12}\left( t\right) \right\| \right] \times \left\| F_{2}\left( t\right) \right\| \nonumber \\&\qquad +\left\| E_{1}^{-1}\left( t\right) \right\| \times \left\| \frac{ d\left[ S_{11}^{12}\left( t\right) +S_{12}^{11}\left( t\right) M\left( t\right) S_{21}^{12}\left( t\right) \right] }{dt}\right\| \nonumber \\&\qquad \times \left\| F_{2}\left( t\right) \right\| +\left\| E_{1}^{-1}\left( t\right) \right\| \times \left[ \left\| S_{11}^{12}\left( t\right) \right\| +\left\| S_{12}^{11}\left( t\right) \right\| \right. \nonumber \\&\qquad \left. \times \left\| M\left( t\right) \right\| \times \left\| S_{21}^{12}\left( t\right) \right\| \right] \times \left\| \frac{\mathrm{d}F_{2}\left( t\right) }{\mathrm{d}t}\right\| \end{aligned}$$

(98)

from (98) and (47), Assumption 7 is proved.

1.5 Assumption 8

The derivative of the second line of (27) corresponding to $A_{21}\left( t\right)$ is

$$\begin{aligned} \overset{\bullet }{A}_{21}\left( t\right)= & {} \frac{\mathrm{d}\left[ E_{2}\left( t\right) F_{2}\left( t\right) \right] ^{-1}}{\mathrm{d}t}\nonumber \\&\times \left[ S_{11}^{21}\left( t\right) +S_{12}^{21}\left( t\right) M\left( t\right) S_{21}^{11}\left( t\right) \right] F_{1}\left( t\right) \nonumber \\&+\left[ E_{2}\left( t\right) F_{2}\left( t\right) \right] ^{-1}\nonumber \\&\times \frac{\mathrm{d}\left[ S_{11}^{21}\left( t\right) +S_{12}^{21}\left( t\right) M\left( t\right) S_{21}^{11}\left( t\right) \right] F_{1}\left( t\right) }{\mathrm{d}t} \end{aligned}$$

(99)

and the norm of this component is given by

$$\begin{aligned} \left\| \overset{\bullet }{A}_{21}\left( t\right) \right\|= & {} \left\| \frac{\mathrm{d}\left[ E_{2}\left( t\right) F_{2}\left( t\right) \right] ^{-1}}{\mathrm{d}t}\right\| \nonumber \\&\times \left[ \left\| S_{11}^{21}\left( t\right) \right\| +\left\| S_{12}^{21}\left( t\right) \right\| \times \left\| M\left( t\right) \right\| \times \left\| S_{21}^{11}\left( t\right) \right\| \right] \nonumber \\&\times \left\| F_{1}\left( t\right) \right\| +\left\| \left[ E_{2}\left( t\right) F_{2}\left( t\right) \right] ^{-1}\right\| \nonumber \\&\times \left\| \frac{\mathrm{d}\left[ S_{11}^{21}\left( t\right) +S_{12}^{21}\left( t\right) M\left( t\right) S_{21}^{11}\left( t\right) \right] }{\mathrm{d}t}\right\| \times \left\| F_{1}\left( t\right) \right\| \nonumber \\&+\left\| \left[ E_{2}\left( t\right) F_{2}\left( t\right) \right] ^{-1}\right\| \times \left[ \left\| S_{11}^{21}\left( t\right) \right\| +\left\| S_{12}^{21}\left( t\right) \right\| \right. \nonumber \\&\left. \times \left\| M\left( t\right) \right\| \times \left\| S_{21}^{11}\left( t\right) \right\| \right] \times \left\| \frac{\mathrm{d}F_{1}\left( t\right) }{\mathrm{d}t}\right\| \end{aligned}$$

(100)

from (100) and (48), Assumption 8 is proved.

Proof of Theorem 3

Assumptions 4–7 are equivalent to Assumptions 1–3; then, we can consider a continuously differentiable and bounded linear time-varying system on T modelled by bond graphs (BGI). This BGI represents a LTV system with singular perturbations. Also, the reduced fast model in a bond graph approach is stable according to Assumption 4. Theorem 1 gives the conditions in order to have the result for first-order approximations and Theorem 2 proposes the state approximations for a LTV singularly perturbed system. From (10) with (11) and (12), $\overline{x_{1}}$ is obtained, in a bond graph approach Lemma 1; from (37) with (38) and (41), $\overline{x_{1}}$ is determined and (49) is proved.

From the reduced fast model $\overset{\bullet }{x}_{2}^{f}=A_{22}\left( t\right) x_{2}^{f}+B_{2}\left( t\right) u$, the state $x_{2}^{f}$ is obtained and from (9) the approximation state for the fast dynamics is got by (20). In a bond graph approach, the model for the fast reduced RFBGI is built by removing the elements, bonds and junctions of the slow dynamics for the complete bond graph (BGI). Hence, RFBGI determines $x_{2}^{f}$, and from SVPBG and (35) of Lemma 1, (50) is proved.