Stability Analysis of Indirect Binary Model Reference Adaptive Controller for Plants with Relative Degree One

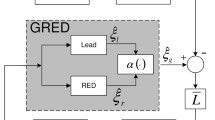
Abstract

This paper presents the Lyapunov-based stability analysis of the Indirect Binary Model Reference Adaptive Controller (IB-MRAC) for relative-degree-one systems. The motivation for indirect adaptive control approaches relies on their capabilities of providing an easier and more intuitive controller design. IB-MRAC is a mixed controller, acting as an Indirect MRAC or as an Indirect Variable Structure MRAC depending on a design parameter. The main result herein described states that the overall system error tends exponentially fast to some small residual set.

Appendices

Appendix A

From (19) and knowing that ${\hat{\theta }_{p}}^T = \left[ \begin{array}{cccc} \hat{k}_{p}&\hat{\beta }^{T}&\hat{\alpha }_{1}&\hat{\alpha }^{T} \end{array} \right]$, one has:

$$\begin{aligned} \displaystyle V(\hat{\theta }_{p}) = {{1} \over {2}} \left\{ {\hat{k}_{p}}^2 + \hat{\beta }^{T}\hat{\beta } + {\hat{\alpha }_{1}}^2 + \hat{\alpha }^{T}\hat{\alpha } \right\} . \end{aligned}$$

(40)

Deriving (40), we obtain:

$$\begin{aligned} \displaystyle \dot{V}(\hat{\theta }_{p}) = {{1} \over {2}} \left\{ 2{\hat{k}_{p}}\dot{\hat{k}}_{p} \!+\! \dot{\hat{\beta }}^{T}\hat{\beta } + \hat{\beta }^{T}\dot{\hat{\beta }}\! +\! 2{\hat{\alpha }_{1}}\dot{\hat{\alpha }}_{1} + \dot{\hat{\alpha }}^{T}\hat{\alpha } + \hat{\alpha }^{T}\dot{\hat{\alpha }} \right\} \!.\nonumber \\ \end{aligned}$$

(41)

Replacing $\dot{\hat{\theta }}_{p}$ by IB-MRAC adaptive laws (16), one has:

$$\begin{aligned} \displaystyle \dot{V}(\hat{\theta }_{p})= & {} {{1} \over {2}} \{ 2{\hat{k}_{p}} [ -\sigma _{k_{p}}\hat{k}_{p} - \gamma _{p}e_{o}\zeta _{p} ]\nonumber \\&+\, [ -\hat{\beta }^{T}\sigma _{\beta }^{T} + e_{o}{v_{1}}^{T}{\varGamma _{\beta }}^{T} ]\hat{\beta }\nonumber \\&+\, \hat{\beta }^{T} [ -\sigma _{\beta }\hat{\beta } + \varGamma _{\beta }e_{o}v_{1} ] \nonumber \\&+\, \displaystyle 2{\hat{\alpha }_{1}} \left[ -\sigma _{\alpha _{1}} \hat{\alpha }_{1} - \gamma _{1}e_{o}\zeta _{1} \right] \nonumber \\&+\, [ -\hat{\alpha }^{T}{\sigma _{\alpha }}^{T} - e_{o}{v_{2}}^{T}{\varGamma _{\alpha }}^{T} ]\hat{\alpha }\nonumber \\&+\, \hat{\alpha }^{T} [ -{\sigma _{\alpha }}\hat{\alpha } - \varGamma _{\alpha }e_{o}{v_{2}} ] \}. \end{aligned}$$

(42)

Performing some simplifications, we obtain the following equation for $\dot{V}$:

$$\begin{aligned} \displaystyle \dot{V}(\hat{\theta }_{p})= & {} \hat{k}_{p} [ -\sigma _{k_{p}}\hat{k}_{p} - \gamma _{p}e_{o}\zeta _{p} ] + \hat{\beta }^{T} [ -\sigma _{\beta }\hat{\beta } + \varGamma _{\beta }e_{o}v_{1} ]\nonumber \\&+\,\displaystyle \hat{\alpha }_{1} \left[ -\sigma _{\alpha _{1}} \hat{\alpha }_{1} - \gamma _{1}e_{o}\zeta _{1} \right] + \hat{\alpha }^{T} [ -{\sigma _{\alpha }}\hat{\alpha } - \varGamma _{\alpha }e_{o}{v_{2}} ].\nonumber \\ \end{aligned}$$

(43)

Developing (43), one has:

$$\begin{aligned} \displaystyle \dot{V}(\hat{\theta }_{p})= & {} -\,\sigma _{k_{p}}{\hat{k}}^2_{p} - \hat{k}_{p}\gamma _{p}e_{o}\zeta _{p} -\sigma _{\beta }\hat{\beta }^{T}\hat{\beta } + \hat{\beta }^{T}\varGamma _{\beta }e_{o}v_{1} \nonumber \\&\displaystyle -\,\sigma _{\alpha _{1}}{\hat{\alpha }}^2_{1} - \hat{\alpha }_{1}\gamma _{1}e_{o}\zeta _{1} -{\sigma _{\alpha }}\hat{\alpha }^{T}\hat{\alpha } - \hat{\alpha }^{T}\varGamma _{\alpha }e_{o}{v_{2}}.\nonumber \\ \end{aligned}$$

(44)

From (18), and knowing that $\hat{\beta }^{T}\hat{\beta } = {||\hat{\beta }||}^2$ and $\hat{\alpha }^{T}\hat{\alpha } = {||\hat{\alpha }||}^2$, one has:

$$\begin{aligned} \displaystyle \dot{V}(\hat{\theta }_{p})= & {} -\sigma _{k_{p}}{\hat{k}}^2_{p} + \sigma _{eq\_k_{p}}{\hat{k}}^2_{p} -\sigma _{\beta }{||\hat{\beta }||}^2 + \sigma _{eq\_\beta }{||\hat{\beta }||}^2 \nonumber \\&\displaystyle -\sigma _{\alpha _{1}}{\hat{\alpha }}^2_{1} + \sigma _{eq\_\alpha _{1}}{\hat{\alpha }}^2_{1} -{\sigma _{\alpha }}{||\hat{\alpha }||}^2 + \sigma _{eq\_\alpha }{||\hat{\alpha }||}^2.\nonumber \\ \end{aligned}$$

(45)

Appendix B

Calculating the derivative of (21), one has:

$$\begin{aligned}&\dot{V}(e, \tilde{\theta }_{p}) = {{1} \over {2}} \left( {\dot{e}^{T}Pe + e^{T}P\dot{e}} \right) +\displaystyle {{1} \over {k_{m}}} \nonumber \\&\quad \times \left( { {2 \tilde{k}_{p} \dot{\tilde{k}}_{p}} \over {2 \gamma _{p}} } + {{{\dot{\tilde{\beta }}}^{T}\varGamma _{\beta }^{-1}{\tilde{\beta }} k_{p} + {\tilde{\beta }}^{T}\varGamma _{\beta }^{-1}\dot{\tilde{\beta }} k_{p} + {\tilde{\beta }}^{T}\varGamma _{\beta }^{-1}\tilde{\beta } \dot{k}_{p}} \over {2 \gamma }} \right. \nonumber \\&\quad \left. +\, {{2{\tilde{\alpha }_{1}}\dot{\tilde{\alpha }}_{1}} \over {2 \gamma _{1}}} + {{\dot{\tilde{\alpha }}^{T}\varGamma _{\alpha }^{-1}{\tilde{\alpha }} + \tilde{\alpha }^{T}\varGamma _{\alpha }^{-1}{\dot{\tilde{\alpha }}}} \over {2 \gamma }} \right) . \end{aligned}$$

(46)

Knowing that $k_{p}$ is a constant, one has that ${\tilde{\beta }}^{T}\varGamma _{\beta }^{-1}\tilde{\beta } \dot{k}_{p} = 0$. Performing some cancellations and replacing $\dot{e}$ by (13), we obtain:

$$\begin{aligned} \displaystyle \dot{V}(e, \tilde{\theta }_{p})= & {} {{1} \over {2}} \left\{ \left( {e}^{T}{A_{c}}^{T} + {{{b_{c}}^{T}} \over {k_{m}}} \left[ \tilde{k}_{p}\zeta _{p} + k_{p}{\tilde{\beta }}^{T}\zeta _{\beta }\right. \right. \right. \nonumber \\&\left. \left. \left. +\, \tilde{\alpha }_{1}\zeta _{1} + {\tilde{\alpha }}^{T}\zeta _{\alpha } \right] \right) Pe \right\} \nonumber \\&+\, \displaystyle {{1} \over {2}} \left\{ e^{T}P\left( A_{c}e + {{b_{c}} \over {k_{m}}} \left[ \tilde{k}_{p}\zeta _{p} + k_{p}{\tilde{\beta }}^{T}\zeta _{\beta }\right. \right. \right. \nonumber \\&\left. \left. \left. +\, \tilde{\alpha }_{1}\zeta _{1} + {\tilde{\alpha }}^{T}\zeta _{\alpha } \right] \right) \right\} \nonumber \\&+\,\displaystyle {{1} \over {k_{m}}} \left( { {\tilde{k}_{p} \dot{\tilde{k}}_{p}} \over {\gamma _{p}} } + {{{\tilde{\beta }}^{T}\varGamma _{\beta }^{-1}\dot{\tilde{\beta }} k_{p}} \over {\gamma }} \right. \nonumber \\&\left. +\, {{{\tilde{\alpha }_{1}}\dot{\tilde{\alpha }}_{1}} \over {\gamma _{1}}} + {{\tilde{\alpha }^{T}\varGamma _{\alpha }^{-1}{\dot{\tilde{\alpha }}}} \over {\gamma }} \right) . \end{aligned}$$

(47)

Reorganizing (47), one has:

$$\begin{aligned}&\displaystyle \dot{V}(e, \tilde{\theta }_{p})= {{1} \over {2}} \left\{ e^{T} \left( {A_{c}}^{T}P + PA_{c} \right) e \right. \nonumber \\&\quad \left. + {{2e^{T}Pb_{c}} \over {k_{m}}} \left[ \tilde{k}_{p}\zeta _{p} + k_{p}{\tilde{\beta }}^{T}\zeta _{\beta } + \tilde{\alpha }_{1}\zeta _{1} + {\tilde{\alpha }}^{T}\zeta _{\alpha } \right] \right\} \nonumber \\&\quad +\displaystyle {{1} \over {k_{m}}} \left( { {\tilde{k}_{p} \dot{\tilde{k}}_{p}} \over {\gamma _{p}} } + {{{\tilde{\beta }}^{T}\varGamma _{\beta }^{-1}\dot{\tilde{\beta }} k_{p}} \over {\gamma }} + {{{\tilde{\alpha }_{1}}\dot{\tilde{\alpha }}_{1}} \over {\gamma _{1}}} + {{\tilde{\alpha }^{T}\varGamma _{\alpha }^{-1}{\dot{\tilde{\alpha }}}} \over {\gamma }} \!\right) .\nonumber \\ \end{aligned}$$

(48)

Considering the Kalman–Yakubovich Lemma ($A_{c}^{T}P + PA_{c} = -2Q, Pb_{c} = h_{c}, P = P^{T} > 0, Q = Q^{T} > 0$), one has that $e^{T}Pb_{c} = e^{T}h_{c} = h_{c}^{T}e = e_{o}$. Applying the changes in (48):

$$\begin{aligned}&\dot{V}(e, \tilde{\theta }_{p})\nonumber \\&\quad = -e^{T}Qe + {{e_{o}} \over {k_{m}}} \left[ \tilde{k}_{p}\zeta _{p} + k_{p}{\tilde{\beta }}^{T}\zeta _{\beta } + \tilde{\alpha }_{1}\zeta _{1} + {\tilde{\alpha }}^{T}\zeta _{\alpha } \right] \nonumber \\&\qquad +\,\displaystyle {{1} \over {k_{m}}} \left( { {\tilde{k}_{p} \dot{\tilde{k}}_{p}} \over {\gamma _{p}} } + {{{\tilde{\beta }}^{T}\varGamma _{\beta }^{-1}\dot{\tilde{\beta }} k_{p}} \over {\gamma }} + {{{\tilde{\alpha }_{1}}\dot{\tilde{\alpha }}_{1}} \over {\gamma _{1}}} + {{\tilde{\alpha }^{T}\varGamma _{\alpha }^{-1}{\dot{\tilde{\alpha }}}} \over {\gamma }} \right) .\nonumber \\ \end{aligned}$$

(49)

From (12), we can observe that $\tilde{\theta }_{p} = \hat{\theta }_{p} - \theta _{p}$, and, consequently, $\dot{\tilde{\theta }}_{p} = \dot{\hat{\theta }}{p} - \dot{\theta }_{p}$. As $\theta _{p}$ is a constant vector, $\dot{\theta }_{p} = 0$ and, thus, $\dot{\tilde{\theta }}_{p} = \dot{\hat{\theta }}_{p}$. Therefore, (49) can be replaced by:

$$\begin{aligned}&\dot{V}(e, \tilde{\theta }_{p})\nonumber \\&\quad = -e^{T}Qe + {{e_{o}} \over {k_{m}}} \left[ \tilde{k}_{p}\zeta _{p} + k_{p}{\tilde{\beta }}^{T}\zeta _{\beta } + \tilde{\alpha }_{1}\zeta _{1} +\, {\tilde{\alpha }}^{T}\zeta _{\alpha } \right] \nonumber \\&\qquad +\, \displaystyle {{1} \over {k_{m}}} \left( { {\tilde{k}_{p} \dot{\hat{k}}_{p}} \over {\gamma _{p}} } + {{{\tilde{\beta }}^{T}\varGamma _{\beta }^{-1}\dot{\hat{\beta }} k_{p}} \over {\gamma }} + {{{\tilde{\alpha }_{1}}\dot{\hat{\alpha }}_{1}} \over {\gamma _{1}}} + {{\tilde{\alpha }^{T}\varGamma _{\alpha }^{-1}{\dot{\hat{\alpha }}}} \over {\gamma }} \right) .\nonumber \\ \end{aligned}$$

(50)

Replacing $\dot{\hat{\theta }}_{p}$ by (16), one has:

$$\begin{aligned}&\dot{V}(e, \tilde{\theta }_{p})\nonumber \\&\quad = -e^{T}Qe + {{e_{o}} \over {k_{m}}} \left[ \tilde{k}_{p}\zeta _{p} + k_{p}{\tilde{\beta }}^{T}\zeta _{\beta } + \tilde{\alpha }_{1}\zeta _{1} + {\tilde{\alpha }}^{T}\zeta _{\alpha } \right] \nonumber \\&\qquad +\, \displaystyle {\tilde{k}_{p}{\left( -\sigma _{k_{p}}\hat{k}_{p} -\gamma _{p}e_{o}\zeta _{p} \right) } \over {k_{m}\gamma _{p}}}\nonumber \\&\qquad +\, {{{\tilde{\beta }}^{T}\varGamma _{\beta }^{-1}k_{p}{\left( -\sigma _{\beta }\hat{\beta } + \varGamma _{\beta }e_{o}v_{1} \right) }} \over {{k_{m}}\gamma }} \nonumber \\&\qquad +\, \displaystyle {{\tilde{\alpha }_{1}{\left( -\sigma _{\alpha _{1}}\hat{\alpha }_{1} -\gamma _{1}e_{o}\zeta _{1} \right) }} \over {{k_{m}}\gamma _{1}}} \nonumber \\&\qquad + {{{\tilde{\alpha }}^{T}\varGamma _{\alpha }^{-1}{\left( -\sigma _{\alpha }\hat{\alpha } - \varGamma _{\alpha }e_{o}v_{2} \right) }} \over {{k_{m}}\gamma }}. \end{aligned}$$

(51)