Analytical Probabilistic Methods to Analyze the Stability of the Rock Wedge with Multiple Failure Modes

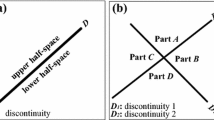
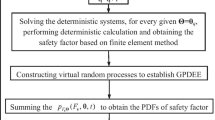
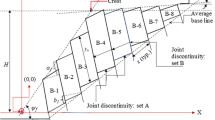
Abstract

Wedge failures are frequently observed in rock slopes. However, these conventional probability analysis methods for rock wedge stability analysis suffer from a known critic of poor efficiency and accuracy. This paper proposes two analytical probabilistic methods (JDRV method and SAPM) to address these issues. The proposed methods are illustrated through a practical rock wedge example. By the comparison with the Monte Carlo simulation (MCs), it can be concluded that the JDRV method is reliable and accurate for the random variables with normal and lognormal distribution, but SAPM is only reliable and accurate for those with normal distribution. The results of the JDRV method and SAPM also indicate that the failure probability of rock wedge will decrease for the random variables with normal distribution with the reduction of correlation coefficient, while the failure probability will increase for the random variables with lognormal distribution. Moreover, the failure probability of rock wedge with biplane sliding mode can greatly be reduced by improving the strength of the discontinuity plane whose effective normal load or area is larger in the two discontinuity planes.

Appendix A

For the random variables c₁, c₂, tanφ₁, and tanφ₂ with the lognormal distribution, we can rewrite Eq. (3) as:

$$ \ln F_{s} = \ln \frac{{e^{{\ln c_{1} }} A_{1} + e^{{\ln c_{2} }} A_{2} + N_{1} e^{{\ln \tan \varphi_{1} }} + N_{2} e^{{\ln \tan \varphi_{2} }} }}{G\sin \beta } $$

(A1)

Similar to normal distribution, F_s, c₁, c₂, tanφ₁, and tanφ₂ can be written as follows:

$$ \ln F_{s} = \mu_{{\ln F_{s} }} + \Delta \ln F_{s} \;E\left[ {\ln F_{s} } \right] = \mu_{{\ln F_{s} }} \;E\left[ {\Delta \ln F_{s} } \right] = 0 $$

(A2)

$$ \ln c_{1} = \mu_{{\ln c_{1} }} + \Delta \ln c_{1} \;\;E\left[ {\ln c_{1} } \right] = \mu_{{\ln c_{1} }} \;\;E\left[ {\Delta \ln c_{1} } \right] = 0 $$

(A3)

$$ \ln \tan \varphi_{1} = \mu_{{\ln \tan \varphi_{1} }} + \Delta \ln \tan \varphi_{1} \;\;E\left[ {\ln \tan \varphi_{1} } \right] = \mu_{{\ln \tan \varphi_{1} }} \;\;E\left[ {\Delta \ln \tan \varphi_{1} } \right] = 0 $$

(A4)

$$ \ln c_{2} = \mu_{{\ln c_{2} }} + \Delta \ln c_{2} \;E\left[ {\ln c_{2} } \right] = \mu_{{\ln c_{2} }} \;E\left[ {\Delta \ln c_{2} } \right] = 0 $$

(A5)

$$ \ln \tan \varphi_{2} = \mu_{{\ln \tan \varphi_{2} }} + \Delta \ln \tan \varphi_{2} \;\;E\left[ {\ln \tan \varphi_{2} } \right] = \mu_{{\ln \tan \varphi_{2} }} \;\;E\left[ {\Delta \ln \tan \varphi_{2} } \right] = 0 $$

(A6)

where ∆lnF_s, ∆lnc₁, ∆lnc₂, ∆lntanφ₁, and ∆lntanφ₂ are the perturbations of the lnF_s, lnc₁, lnc₂, lntanφ₁, and lntanφ₂. After a Taylor expansion for Eq. (A1) is carried out at the mean and the expansion is retained the first-order term, the following formula can be obtained.

$$ \begin{aligned} \ln \mu_{{F_{s} }} + \Delta \ln F_{s} = & \frac{{A_{1} e^{{\mu_{{\ln c_{1} }} }} + A_{2} e^{{\mu_{{\ln c_{2} }} }} + N_{1} e^{{\mu_{{\ln \tan \varphi_{1} }} }} + N_{2} e^{{\mu_{{\ln \tan \varphi_{2} }} }} }}{G\sin \beta } \\ & + \frac{{\Delta \ln c_{1} A_{1} e^{{\mu_{{\ln c_{1} }} }} + \Delta \ln c_{2} A_{2} e^{{\mu_{{\ln c_{2} }} }} + \Delta \ln \tan \varphi_{1} N_{1} e^{{\mu_{{\ln \tan \varphi_{1} }} }} + \Delta \ln \tan \varphi_{2} N_{2} e^{{\mu_{{\ln \tan \varphi_{2} }} }} }}{{A_{1} e^{{\mu_{{\ln c_{1} }} }} + A_{2} e^{{\mu_{{\ln c_{2} }} }} + N_{1} e^{{\mu_{{\ln \tan \varphi_{1} }} }} + N_{2} e^{{\mu_{{\ln \tan \varphi_{2} }} }} }} \\ \end{aligned} $$

(A7)

Furthermore, the mean of F_s can be estimated by:

$$ \ln \mu_{{F_{s} }} = \frac{{A_{1} e^{{\mu_{{\ln c_{1} }} }} + A_{2} e^{{\mu_{{\ln c_{2} }} }} + N_{1} e^{{\mu_{{\ln \tan \varphi_{1} }} }} + N_{2} e^{{\mu_{{\ln \tan \varphi_{2} }} }} }}{G\sin \beta } $$

(A8)

And the variance of F_s can be determined by:

$$ \sigma_{{F_{s} }}^{2} = \frac{{\sigma_{{\ln c_{1} }} \left( {A_{1} e^{{\mu_{{\ln c_{1} }} }} } \right)^{2} + \sigma_{{\ln c_{2} }} \left( {A_{2} e^{{\mu_{{\ln c_{2} }} }} } \right)^{2} + \sigma_{{\ln \tan \varphi_{1} }} \left( {N_{1} e^{{\mu_{{\ln \tan \varphi_{1} }} }} } \right)^{2} + \sigma_{{\ln \tan \varphi_{2} }} \left( {N_{2} e^{{\mu_{{\ln \tan \varphi_{2} }} }} } \right)^{2} }}{{\left( {A_{1} e^{{\mu_{{\ln c_{1} }} }} + A_{2} e^{{\mu_{{\ln c_{2} }} }} + N_{1} e^{{\mu_{{\ln \tan \varphi_{1} }} }} + N_{2} e^{{\mu_{{\ln \tan \varphi_{2} }} }} } \right)^{2} }} $$

(A9)

Due to the linearity of Eq. (A7), it can be known that lnF_s obeys normal distribution with mean μ_lnFs and standard deviation σ_lnFs. In this case, the failure probability of rock wedge can be calculated by the following formula.

$$ P_{f} = P\left[ {\ln F_{s} < \ln 1} \right] \approx \Phi \left[ {\frac{{\ln 1 - \mu_{{\ln F_{s} }} }}{{\sigma_{{\ln F_{s} }} }}} \right] $$

(A10)