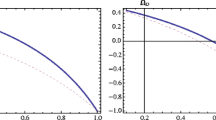
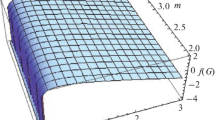
Abstract
Purpose of this paper is to study generalized quantum chromodynamics ghost dark energy (GDE) in the frame work of Horava–Lifshitz cosmology. Considering interacting and non-interacting scenario of GDE with dark matter in a spatially non-flat universe, we investigate the cosmological implications of this model in detail. We obtain equation of state parameter, deceleration parameter and the evolution of dark energy density to explain the expansion of the universe. Also, we show that the results we calculate have a good compatibility with previous work and restore it in limiting case. Further, we investigate validity of generalized second law of thermodynamics in this scenario. Finally, we find out a cosmological application of our work by evaluating a relation for the equation of state of dark energy for law redshifts.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
Introduction
The discovery that universe is in an accelerating expansion phase is one of the most remarkable advancement in modern cosmology. Several cosmological observations clearly indicate that the present day universe is experiencing a phase of accelerated expansion [1–4]. This current accelerating expansion of the universe is dominated by an unknown energy termed as dark energy with negative pressure. Numerous models have been proposed to understand the dark energy phenomena and evolution of the universe. Comprehensive reviews of these models have been widely reported [5, 6]. However, nature and cosmological origin of dark energy still remain enigmatic at present.
In most of recent dark energy models, normally we need to consider a new degree of freedom or a new parameter, to explain the cosmic acceleration of Universe. However, our prior choice to handle the dark energy problem without introducing new degrees of freedom beyond what are already known. An interesting and successful attempt, in this direction is the so called “ghost dark energy” (GDE), which has been motivated from Veneziano ghost of chromodynamics. The Veneziano ghost field was proposed to resolve the \(U(1)\) problem in quantum chromodynamics (QCD) [7–9]. It is well known that QCD ghost fields have positive and negative norms and cancel with each other, leaving no trace in the physical subspace, but it was argued that they have small contribution to the vacuum energy in the curved space or time-dependent background [10]. The ghost field has no contribution in the vacuum energy in Minkowski space-time but it has a non-vanishing contribution to the vacuum energy, in a dynamical background such as our universe, is proportional to \(\Lambda _\mathrm{QCD}^{3}H\), where \(H\) is Hubble parameter and \(\Lambda _\mathrm{QCD}^{3}\) is QCD mass scale [10]. Taking into account the fact that \(\Lambda _\mathrm{QCD}\sim 100\, \mathrm{MeV}\) and \(H\sim 10^{-33}\,\mathrm{eV}\) for present time, this gives the right order of magnitude \(\rho _\mathrm{D}\sim (3\times 10^{-3}\,\mathrm{eV})^{4}\) for the ghost energy density [10]. Thus GDE model can solve the fine tuning problem [10–12]. Energy density of ghost dark energy reads as [10–12]
where \(H\) is Hubble parameter \(H = \frac{\dot{a}}{a}\) and \(\alpha \) is constant parameter of the model, which should be determined. Author of Ref. [13] discussed that the contribution of the Veneziano QCD ghost field to the vacuum energy is not exactly of order \(H\) and a sub-leading term \(H^{2}\) appears due to the fact that the vacuum expectation value of the energy–momentum tensor is conserved in isolation [14]. It was argued that the vacuum energy of the ghost field can be written as \(H +O(H^{2})\), where the sub-leading term \(H^{2}\) in the GDE model might play a crucial role in the early stage of the universe evolution, acting as the early DE [15]. It was shown [15, 16] that taking the second term into account can give better agreement with observational data compared to the usual GDE. The density of this generalized ghost dark energy (GGDE) reads [15]
with \(\beta \) another constant parameter of the model with [energy]\(^{2}\).
The cosmological implications of GDE model in a flat universe were considered in [17]. The generalization to the non-flat Friedmann–Robertson–Walker (FRW) universe and its consistency check with recent observational data were studied in [18]. The GDE model in the framework of Brans–Dicke cosmology and its stability against perturbations were also investigated in [19, 20]. The correspondence between this model and scalar field models of dark energy has been widely extended by many authors in the recent years [21]. Furthermore GDE has been studied and extended in the frame work of modified gravity [22, 23].
It is a well-known fact that the biggest challenge towards a theory of quantum gravity is the question of renormalizability whose lack, in effect, would imply loss of theoretical control and predictability at high energies. General relativity although performs fairly well at the solar system scale but fails at the cosmic scales at which it is unable to explain the origin of dark energy or presents accelerated cosmic acceleration properly. Therefore the classical generalization of general relativity is considered as the gravitational alternative for a unified description of the early-time inflation with late-time cosmic acceleration. Thus, to study the structure and cosmological properties of modified theories, the cosmological reconstruction of different modified gravities is provided in detail. In other words, the modified gravity, which represents a classical generalization of general relativity, becomes reasonable alternative. Scalar tensor theories are also modified theories of gravity. Lifshitz and Eksp [24] introduced a scalar theory in which the temporal dimension has weight equal to three if the space dimension has weight equal to one. Recently Horava [25–28] proposed a new quantizable theory of gravity, using the ideas from solid state physics, which is similar to Lifshitz theory, thus this theory is called Horava–Lifshitz gravity theory. This is a power-counting renormalizable theory with consistent ultraviolet (UV) behavior and the theory has one fixed point in the infrared limit (IR), namely general relativity [25–28]. Thus, this theory leads to a modification of the Einstein’s general relativity at high energies producing interesting features in cosmology. Furthermore Horava–Lifshitz gravity has been studied and extended in detail [29–36] and applied as a cosmological frame work of the universe [37–46]. Recently, different dark energy models have been studied in the framework of Horava–Lifshitz cosmology using different infrared cut-offs. For comprehensive reviews on the scenario where the cosmological evolution is governed by Horava–Lifshitz cosmology one can go through the references [47–53]. Moreover, the ghost energy density belongs to a dynamical cosmological constant, therefore we need a dynamical frame to accommodate it instead of general relativity. Also the original GDE is unstable in all range of the parameter spaces in standard [20]. So it is worthwhile to investigate the GGDE model in the framework of the Horava–Lifshitz cosmology.
This paper is organized as follows: in Sect. 2, we discuss briefly some important features of Horava–Lifshitz Cosmology. In Sect. 3, we study GGDE in Horava–Lifshitz cosmology for interacting and non-interacting scenario of dark energy with dark matter. Section 4 is devoted for a cosmological application of our work, where we obtain the equation of state of dark energy at low redshifts. Since, study of thermodynamic properties of ghost dark energy in Horava–Lifshitz cosmology is also an important direction, in Sect. 5, we investigate the validity of generalized second law of thermodynamics. Finally in the final section we summarize our results.
Horava–Lifshitz cosmology
In this section, we briefly review Horava–Lifshitz cosmology. Under the projectability condition, the full metric in the \(3+1\)-dimensional Arnowitt–Deser–Misner formalism is given by
where dynamical variables \(N\) and \(N^{i}\) are the lapse and shift functions, respectively. The indices are raised and lowered by using the spatial metric \(g_{ij}\). The scaling transformation of the co-ordinates for \(z=3\) is given by
Decomposing the gravitational action into a kinetic and a potential part, action of Horava–Lifshitz cosmology can be written as
Under the assumption of detailed balance [25] the full action of Horava–Lifshitz gravity is given by
where
are extrinsic curvature and cotton tensor, respectively. Also, \(\eta ^{ijk}\) is the totally antisymmetric unit tensor, \(\lambda \) is a dimensionless constant and \(\Lambda \) is a negative constant which is related to the cosmological constant in the IR limit. The variables, \(\kappa , \omega \) and \(\mu \) are constants with mass dimensions \(-1, 0\) and \( 1\), respectively. To include dark matter component in a universe governed by Horava gravity, two options can be considered: one is to introduce a scalar field \(\phi \) with action [54] and the second is add a cosmological stress–energy tensor to the gravitational field equations [55]. Here in our work we follow the second one.
In the cosmological contents, we consider FRW metric,
with
where, \(k=-1,0,+1\) refer to spatially open, flat and closed universe, respectively. Taking the variation of action with respect to the metric components \(N\) and \(g_{ij}\), one can obtain the equation of motion as
where \(H=\frac{\dot{a}}{a}\) is the Hubble parameter. Keeping in mind the Friedmann equations [given by Eqs. (11, 12)], we can define the energy density \(\rho _\mathrm{D}\) and pressure density \(p_\mathrm{D}\) of dark energy as
The term proportional to \(a^{-4}\) is the “dark radiation term” in Horava–Lifshitz cosmology. Rewriting Friedmann Eqs. (11) and (12), using Eqs. (13) and (14), we have
with
where \(G_\mathrm{c}\) represents the Newton’s cosmological constant. Additionally, we can define Newton’s gravitational constant as
It is interesting that in the IR limit \((\lambda =1)\), \(G_\mathrm{c}\) and \(G_{g}\) are the same.
Generalized ghost dark energy in Horava–Lifshitz cosmology
To study generalized ghost dark energy in the frame work of Horava–Lifshitz cosmology, we consider a spatially non-flat FRW Universe containing DE and DM. We define the critical energy density, \(\rho _\mathrm{cr}\), and the energy density of the curvature, \(\rho _{k}\), as
Then the dimensionless density parameter can also be defined as usual
Using Eqs. (21), (22) and (23), the first Friedmann Eq. (15), can be written as
Non-interacting scenario
In this section, we extend our study to the case where PLECHDE and dark matter are interaction free. In non-interacting scenario the energy conservation laws for spatially non-flat FRW universe are as follows
where \(w_\mathrm{D}=\frac{p_\mathrm{D}}{\rho _\mathrm{D}}\) is the equation of state parameter of PLECHDE.
Differentiating Eqs. (2) and (15) with respect to time, we obtain
where, \(u=\frac{\rho _{m}}{\rho _\mathrm{D}}=\frac{\Omega _{m}}{\Omega _\mathrm{D}}\).
Combining Eqs. (27) and (28) with continuity Eq. (25), we have
here, it is easy to see that at late time where \(\Omega _\mathrm{D}\longrightarrow 1\) (in limiting case \(\Omega _{k}\longrightarrow 0\)), we have \(w_\mathrm{D}\longrightarrow -1\), which means that GGDE mimics a cosmological constant. Also if \(\beta = 0\) or \(\frac{8\pi G_\mathrm{c}}{3}\longrightarrow 0\), Eq. (29) restores the EoS parameter of the original GDE model [18]
On the other hand, for flat universe \(\Omega _{k}=0\), the equation of state parameter of GGDE in Horava–Lifshitz cosmology is
It is clear from this relation also that if \(\beta = 0\) or \(\frac{8\pi G_\mathrm{c}}{3}\longrightarrow 0\), it restores its respective one in GDE [18].
Imposing the present observational values \(\Omega _{\Lambda }\approx 0.73\) and \(\Omega _{k}\approx 0.02\) in Eq. (29), we can see that \(w_\mathrm{D}< -1\) if \(\frac{8\pi G_\mathrm{c}\beta }{3} < \frac{2}{3}\). This implies that GGDE model in Horava–Lifshitz cosmology replicates the role of Phantom dark energy model at present time if \(\frac{8\pi G_\mathrm{c}\beta }{3} < \frac{2}{3}\).
We can also study the evolution behavior of the GGDE. For this, differentiating Eq. (22) with respect to time and using Eqs. (27), (28) and relation \(\dot{\Omega _\mathrm{D}}=H\Omega _\mathrm{D}'\) we obtain
Also using Eqs. (24) and (29), we have
Using Eq. (33) in Eq. (32), finally we obtain the expression that describes the evolution behavior of the non-interacting GGDE in the frame work of Horava–Lifshitz cosmology as
For completeness, we find the deceleration parameter ‘\(q\)’ which represents the decelerated or accelerated phase of the expansion of the universe. The positive value of deceleration parameter \((q>0)\) indicates the decelerated phase of expansion and the negative value \((q<0)\) represents the accelerated phase of expansion of the universe. Thus using Eq. (28) we obtain the decelerated parameter as
Combining Eqs. (29) and (35), finally we have the decelerated parameter is
Interacting scenario
To be more general, in this section we consider an interaction between both the dark components, the pressure-less dark matter and the ghost dark energy . The recent observational evidence provided by the galaxy clusters also supports the interaction between dark energy and dark matter [56]. In this case, the energy densities of dark energy and dark matter no longer satisfy independent conservation laws. They obey instead
where \(Q\) represents the interaction term. Following [57], we consider the simplest form of \(Q\) as
where \(b^{2}\) is a coupling constant. The positive \(b^{2}\) is responsible for the transition from dark energy to matter and vice-versa for negative \(b^{2}\). Note that the form of \(Q\) chosen is purely phenomenological, i.e. to obtain desirable cosmological findings although more general phenomenological interaction terms can be used. Following as above, we obtain expressions for \(w_\mathrm{D}\) and \(\Omega _\mathrm{D}'\) as
and
As in the non-interacting case here also if \(\beta = 0\) or \(\frac{8\pi G_\mathrm{c}}{3}\longrightarrow 0\), Eq. (40) restores the EoS parameter of the original GDE model [18] in interacting case. Also for flat universe, where \(\Omega _{k}=0\), the equation of state parameter of GGDE in interacting case takes the form
Using Eq. (40) in Eq. (35), we obtain the deceleration parameter in interacting case as
Equation of state of generalized ghost dark energy at low redshift
This section is devoted for cosmological applications of our work, using the differential expression that we obtained above, which determine the evolution of dark energy parameter. We will set up the Ghost dark energy equation of state parameter for low redshift at present time. Since we have derived the expression for \(\Omega _\mathrm{D}'\), hence we can calculate \(\omega (z)\) for low redshift \(z\). We can measure \(\omega \) as in [58], due to \(\rho _\mathrm{D}\). In this regard, since \(\rho _\mathrm{D} \sim \frac{1}{a^{3(1+\omega )}}\), we will use Taylor expansion for \(\rho _\mathrm{D}\)
Here derivatives are taken at present time \(a_{0}=1\), therefore \(\omega (\ln a)\) is
up to second order. Replacing \(\ln a= -\ln (1+z)=-z\), which is valid for small redshifts, we can calculate \(\omega (z)\) as
Using
we can find out linear equation of state parameter \(\omega (z)\) as
where,
and
Using Friedmann second Eq. (16), we can find \(\Omega _{k}'\) and \(\Omega _{k}''\) from Eq. (23) as
and
Also from Eq. (34), we have
Inserting Eqs. (34) and (51) in the expression of \(\omega _{0}\) given in Eq. (49), we obtain
Using Eqs. (34), (51), (52) and (53) into Eq. (50), we finally obtain the expression for \(\omega _{1}\) as
Equations (54) and (55) together determine the linear equation of state of dark energy for low redshift.
Validity of generalized second law of thermodynamics
In this section, we are going to investigate validity of generalized second law of thermodynamics of a universe bounded apparent horizon and governed by Horava–Lifshitz gravity. Bekenstein [59] introduced that, in view of the proportionality relation between entropy of black hole horizon and horizon area, the sum of black hole entropy and the background entropy must be an increasing quantity with time. Also, the thermodynamic interpretation of field equations can be applicable for any horizon, provided that the gravitational theory is diffeomorphism invariant [60], however, apparent horizon is widely used in the literature in FRW geometry [61–64]. In the case of Horava–Lifshitz gravity, the breaking of diffeomorphism invariance leaves us the apparent horizon as a reasonable choice, thus here we will use apparent horizon as black hole horizon. The dynamical apparent horizon, a marginally trapped surface with vanishing expansion can be determined by the equality \(h^{ij}\partial _{i}R_\mathrm{A}\partial _{j}R_\mathrm{A}=0\). We can find the radius of cosmological apparent horizon as [65],
Therefore the evolution of the apparent horizon follows,
The first law of thermodynamics gives
where \(T, S_{1},V, E_{1}\) and \(P_\mathrm{D}\) represent temperature, entropy, volume, energy of the matter distribution and pressure of the system, respectively. We consider that temperature of the source inside horizon is in equilibrium with temperature associated with horizon, so starting with volume and energy of the system bounded by apparent horizon as
and using Eqs. (57), (58) and (59), we obtain
To connect the entropy of the horizon to its radius \(R_\mathrm{A}\) in Horava–Lifshitz cosmology, we consider the expression as in [66]
Differentiating Eq. (61), we have
Also, temperature of the horizon is related to its radius for spherical geometry [60], and we have,
In view of the above equations, we can find the rate of change of total entropy as
From the above equation, it is difficult to give definite conclusion regarding validity of the generalized second law of thermodynamics, but if generalized ghost dark energy is not of phantom nature then the second law of thermodynamics is valid in the generalized ghost dark energy model if \((8\pi R_\mathrm{A}^{3}\rho _{m}+1-2\pi R_\mathrm{A})> 0\).
Conclusion
In this paper, we have investigated the GGDE scenario in the frame work of Horava–Lifshitz cosmology. Since GDE density corresponds to a dynamical cosmological constant, we need a dynamical framework, instead of general relativity, to consistently accommodate it. There fore we investigate the GDE in the framework of Horava–Lifshitz cosmology. Experimental data have implied that our universe is not a perfectly flat universe and it possesses a small positive curvature \((\Omega _{k}\sim 0.02)\) [47], so here we consider a non-flat FRW universe. We have extracted the exact differential equations that derive evolution of the dark energy parameter, which gives a suitable estimate for the state parameter of dark energy as well as the deceleration parameter to study an expansion of the universe for both interacting and non-interacting case. We have obtained that GGDE model in Horava–Lifshitz cosmology replicates the role of Phantom dark energy model at present time if \(\frac{8\pi G_\mathrm{c}\beta }{3} < \frac{2}{3}\). Furthermore, we have established that our work has a good compatibility with previous works and restores it in limiting cases. It is also interesting to see that we have found out a cosmological application of our work by evaluating a relation for the equation of state of dark energy for low redshifts. Moreover, we have obtained that generalized second law of thermodynamics valid in case of non-phantom nature of generalized ghost dark energy, but in general we can not give a definite conclusion regarding validity of generalized second law.
References
Riess, A.G., et al.: Observational evidence from supernovae for an accelerating universe and a cosmological constant. Astron. J. 116, 1009 (1998)
Perlmutter, S.J., et al.: Measurements of \(\Omega \) and \(\Lambda \) from 42 high-redshift supernovae. Astrophys. J. 517, 565 (1999)
Tegmark, M., et al.: (SDSS Collaboration), Cosmological parameters from SDSS and WMAP. Phys. Rev. D 69, 103501 (2004)
Spergel, D.N., et al.: [WMAP Collaboration], First year Wilkinson Microwave Anisotropy Probe observations : determination of cosmological parameters. Astrophys. J. Suppl. 148, 175 (2003)
Copland, E.J., Sami, M., Tsujikawa, S.: Dynamics of dark energy. Int. J. Mod. Phys. D 15, 1753 (2006)
Li, M., Li, X.D., Wang, S., Wang, Y.: Dark Energy. Commun. Theor. Phys. 56, 525 (2011)
Witten, E.: Current Algebra theorem for the U(1) “Goldstone Boson”. Nucl. Phys. B 156, 269 (1979)
Veneziano, G.: U(1) without instantons. Nucl. Phys. B 159, 213 (1979)
Rosenzweig, C., Schechter, J., Trahern, C.G.: Is the effective Lagrangian for quantum Chromodynamics a \(\sigma \) model. Phys. Rev. D 21, 3388 (1980)
Ohta, N.: Dark Energy and QCD Ghost. Phys. Lett. B 695, 41 (2011)
Urban, F.R., Zhitnitsky, A.R.: The cosmological constant from the QCD Veneziano Ghost. Phys. Lett. B 688, 9 (2010)
Urban, F.R., Zhitnitsky, A.R.: The Cosmological constant from the ghost: A Toy model. Phys. Rev. D 80, 063001 (2009)
Zhitnitsky, A.R.: Contact term, its holographic description in QCD and dark energy. Phys. Rev. D 86, 045026 (2012)
Maggiore, M.: Zero-point quantum fluctuations and dark energy. Phys. Rev. D 83, 063514 (2011)
Cai, R.G., Tuo, Z.L., Wu, Y.B., Zhao, Y.Y.: More on QCD Ghost Dark Energy. Phys. Rev. D 86, 023511 (2012)
Ebrahimi, E., Sheykhi, A.: Interacting generalized ghost dark energy in a non-flat universe. Central Eur. J. Phys. 11, 949 (2013)
Cai, R.G., Tuo, Z.L., Zhang, H.B.: Notes on Ghost Dark Energy. Phys. Rev. D 84, 123501 (2011)
Sheykhi, A., Sadegh, M.: Movahed, Interacting ghost dark energy in non-flat universe. Gen. Relativ. Gravit. 44, 449 (2012)
Ebrahimi, E., Sheykhi, A.: Interacting ghost dark energy in Brans-Dicke theory. Phys. Lett. B 705, 19 (2011)
Ebrahimi, E., Sheykhi, A.: Instability of QCD ghost dark energy model. Int. J. Mod. Phys. D 20, 2369 (2011)
Sheykhi, A., Bagheri, A.: Quintessence Ghost Dark Energy Model. Europhys. Lett. 95, 39001 (2011)
Khodam-Mohammadi, A., Malekjani, M., Monshizadeh, M.: Reconstruction of modified gravity with ghost dark energy models. Mod. Phys. Lett. A 27, 1250100 (2012)
Yousefi, L., Sheykhi, A.: QCD Ghost Dark Energy in RS II Braneworld with Bulk-Brane Interaction. Int. J. Theor. Phys. 53, 1472 (2014)
Lifshitz, E.M.: On the theory of second order phase transitions I and II. Zh. Eksp. Teor. Fiz. 11, 255 (1949)
Horava, P.: Membranes at quantum criticality. J. High Energy Phys. 3, 20 (2009)
Horava, P.: Quantum Gravity at a Lifshitz Point. Phys. Rev. D 79, 084008 (2009)
Horava, P.: Spectral Dimension of the Universe in Quantum Gravity at a Lifshitz Point. Phys. Rev. Lett. 102, 161301 (2009)
Horava, P.: Quantum criticality and YangMills gauge theory. Phys. Lett. B 694, 172 (2010)
Cai, R.G., Cao, L.M., Ohta, N.: Topological black holes in Hoava-Lifshitz gravity. Phys. Rev. D 80, 024003 (2009)
Germani, C., Kehagias, A., Sfetsos, K.: Relativistic Quantum Gravity at a Lifshitz Point. Journal of High Energy Physics 9, 60 (2009)
Bogdanos, C., Saridakis, E.N.: Perturbative instabilities in Hoava gravity. Classical and Quantum Gravity 27, 075005 (2010)
Myung, Y.S.: Generalized uncertainty principle and Hoava-Lifshitz gravity. Physics Letters B 679, 491 (2009)
Alexandre, J., Farakos, K., Pasipoularides, P., Tsapalis, A.: Schwinger-Dyson approach for a Lifshitz-type Yukawa model. Phys. Rev. D 81, 045002 (2010)
Capasso, D., Polychronakos, A.P.: Particle kinematics in Hoava-Lifshitz gravity. Journal of High Energy Physics 2, 68 (2010)
Koyama, K., Arroja, F.: Pathological behaviour of the scalar graviton in Hoava-Lifshitz gravity. Journal of High Energy Physics 3, 61 (2010)
Li, M., Pang, Y.: A trouble with Hoava-Lifshitz gravity. Journal of High Energy Physics 8, 15 (2009)
Kiritsis, E., Konas, G.: Hoava-Lifshitz cosmology. Nuclear Physics B 821, 467 (2009)
Wang, A., Wu, Y.: Cosmology in nonrelativistic general covariant theory of gravity. J. Cosmol. Astropart. Phys. 7, 12 (2009)
Sotiriou, T.P., Visser, M., Weinfurtner, S.: Quantum gravity without Lorentz invariance. Journal of High Energy Physics 10, 33 (2009)
Mukohyama, S.: Scale-invariant cosmological perturbations from Horava-Lifshitz gravity without inflation. J. Cosmol. Astropart. Phys. 6, 1 (2009)
Cai, Y.F., Zhang, X.: Primordial perturbations with a modified dispersion relation. Phys. Rev. D 80, 043520 (2009)
Gao, X., Wang, Y., Xue, W., Brandenberger, R.: Fluctuations in a Hoava-Lifshitz Bouncing Cosmology. J. Cosmol. Astropart. Phys. 2, 20 (2010)
Kim, S.S., Kim, T., Kim, Y.: Surplus solid angle as an imprint of Hoava-Lifshitz gravity. Phys. Rev. D 80, 124002 (2009)
Dutta, S., Saridakis, E.N.: Observational constraints on Hoava-Lifshitz cosmology. J. Cosmol. Astropart. Phys. 1, 13 (2010)
Kehagias, A., Sfetsos, K.: The black hole and FRW geometries of non-relativistic gravity. Physics Letters B 678, 123 (2009)
Greenwald, J., Papazoglou, A., Wang, A.: Black holes and stars in Horava-Lifshitz theory with projectability condition. Phys. Rev. D 81, 084046 (2010)
Jamil, M., Saridakis, E.N., Setare, M.R.: The generalized second law of thermodynamics in. Horava Lifshitz cosmology. J. Cosmol. Astropart. Phys. 11, 32 (2010)
Jamil, M., Saridakis, E.N.: New agegraphic dark energy in Hova-Lifshitz cosmology. J. Cosmol. Astropart. Phys. 7, 28 (2010)
Setare, M.R., Jamil, M.: Holographic dark energy with varying gravitational constant in Horava-Lifshitz cosmology. J. Cosmol. Astropart. Phys. 2, 10 (2010)
Pasqua, A., Chattopadhyay, S.: New Agegraphic Dark Energy model in chameleon Brans-Dicke cosmology for different forms of the scale factor. Astrophys. Space Sci. 348, 541 (2013)
Elizalde, E., Nojiri, S., Odintsov, S.D., Saez-Gomez, D.: Unifying inflation with dark energy in modified F(R) Horava-Lifshitz gravity. European Physical Journal C 70, 351 (2010)
Lepe, Samuel, Saavedra, Joel: On Horava-Lifshitz cosmology. Astrophys. Space Sci. 350, 839 (2014)
Setare, M.R., Momeni, D., Moayedi, S.K.: Interacting dark energy in Hoava-Lifshitz cosmology. Astrophys. Space Sci. 338, 405 (2012)
Calcagni, G.: Cosmology of the Lifshitz universe. J. High Energy Phys. 9, 112 (2009)
Chaichian, M., Nojiri, S., Odintsov, S.D., Oksanen, M., Tureanu, A.: Modified F(R) Horava-Lifshitz gravity: a way to accelerating FRW cosmology. Class. Quantum Gravity 27, 185021 (2010)
Bartolami, O., Gil, F.: Perdro and M. LeDelliou, Dark Energy-Dark Matter Interaction and putative violation of the Equivalence Principle from the Abell Cluster A586. Phys. Lett. B 654, 165 (2007)
Pavon, D., Zimdahl, W.: Holographic dark energy and cosmic coincidence. Phys. Lett. B 628, 206 (2005)
Riess, A.G., et al.: Type Ia Supernova Discoveries at \(z>1\) From the Hubble Space Telescope: Evidence for Past Deceleration and Constraints on Dark Energy Evolution. Astrophys. J. 607, 665 (2004)
Bekenstein, J.D.: Black Holes and Entropy. Phys. Rev. D 7, 2333 (1973)
Padmanabhan, T.: Thermodynamical Aspects of Gravity: New insights. Rept. Prog. Phys. 73, 046901 (2010)
Frolov, A.V., Kofman, L.: Inflation and de Sitter thermodynamics. J. Cosmol. Astropart. Phys. 05, 009 (2003)
Bousso, R.: Cosmology and the S matrix. Phys. Rev. D 71, 064024 (2005)
Cai, R.G., Kim, S.P.: First Law of Thermodynamics and Friedmann Equations of Friedmann-Robertson-Walker Universe. JHEP 0502, 050 (2005)
Akbar, M., Cai, R.G.: Thermodynamic behavior of the Friedmann equation at the apparent horizon of the FRW universe. Phys. Rev. D 75, 084003 (2007)
Bak, D., Rey, S.J.: Cosmic Holography. Class. Quant. Grav. 17, L83 (2000)
Jamil, M., Saridakis, E.N., Setare, M.R.: The generalized second law of thermodynamics in Horava-Lifshitz cosmology. J. Cosmol. Astropart. Phys. 11, 1475 (2010)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution License which permits any use, distribution, and reproduction in any medium, provided the original author(s) and the source are credited.
About this article
Cite this article
Borah, B., Ansari, M. Generalized ghost dark energy in Horava–Lifshitz cosmology. J Theor Appl Phys 9, 7–14 (2015). https://doi.org/10.1007/s40094-014-0153-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s40094-014-0153-5